Confidence intervals

Confidence coefficient (confidence level) is a probability with which the inequality takes place, i.e.
Remark.
The statistical methods do not allow us to say that the estimator satisfies the inequality
We can only say about probability with which this inequality holds.

The interval
which covers the unknown parameter with prescribed probability is called confidence interval (CI).
— estimation accuracy.
The construction of the CI:
1) point estimate calculation;
2) the choice of confidence level (0,95; 0,99; 0,995);
3) the calculation of the accuracy .

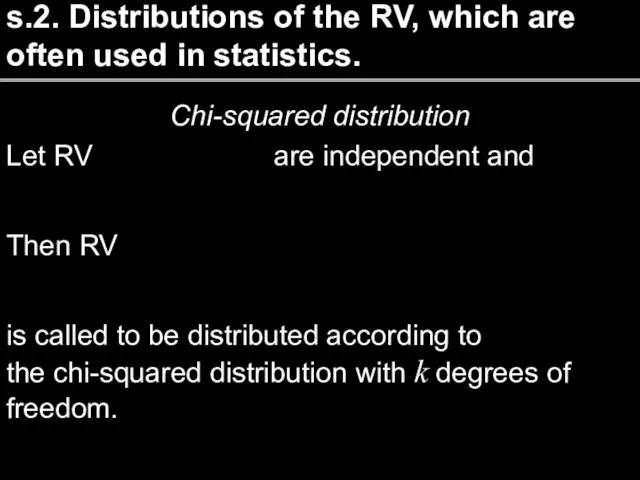
s.2. Distributions of the RV, which are often used in statistics.
Chi-squared distribution
Let RV are independent and
Then RV
is called to be distributed according to the chi-squared distribution with k degrees of freedom.

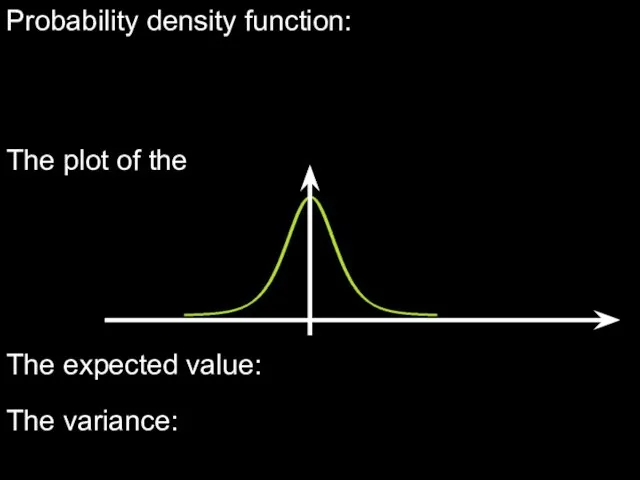
Probability density function:
where
— Gamma function.
The plot of

The expected value:
The variance:
The quantile of the distribution, which corresponds the statistical significance , is a such value that the following inequality holds:
Remark.
The values of the quantiles can be found in special tables.

Student’s t-distribution (t-distribution)
Let
RV has the chi-squared distribution with k degrees of freedom.
Then the RV
is called to be distributed according to the t- distribution with k degrees of freedom.

Probability density function:
The plot of the
The expected value:
The variance:

The quantile of the t-distribution, which corresponds the statistical significance , is a such value that the following inequality holds:
Remark.
The values of the quantiles can be found in special tables.

s.3. Confidence Intervals for Unknown Mean and Known Standard Deviation.
Let
We know
We should find the CI for the a with confidence level .
Let’s find accuracy .
The point estimate for the mean is

Let
is a sample obtained from the observations for the RV X.
The values
change from sample to sample.
Therefore, we can assume that
Besides, the sample mean is also a RV which has normal distribution, and

i.e.

Since
then
Let us denote
Then
and

Therefore
i.e. with confidence level we can assert than CI
covers unknown parameter a, and the accuracy of the estimation is

Example. Let we have sample of the RV
Find 95% confidence interval for the mean.
Solution.
Confidence interval:

s.4. Confidence Intervals for Unknown Mean and Unknown Standard Deviation.
Let
We know
We should find the CI for the a with confidence level .
Let’s find accuracy .
The point estimate for the mean is

Let S is a standard error.
Consider the following RV
We can prove that T has t-distribution with degrees of freedom.
Let’s find so that

Let us divide the both sides of the inequality in brackets on
or
Let us denote
Then
The value can be determined by the t-distribution table.

i.e. with confidence level we can assert than CI
Therefore
or
covers unknown parameter a, and the accuracy of the estimation is

Example. In the previous example find CI for the unknown mean, if standard deviation is unknown.
Solution. Let’s find S: