Integration by parts

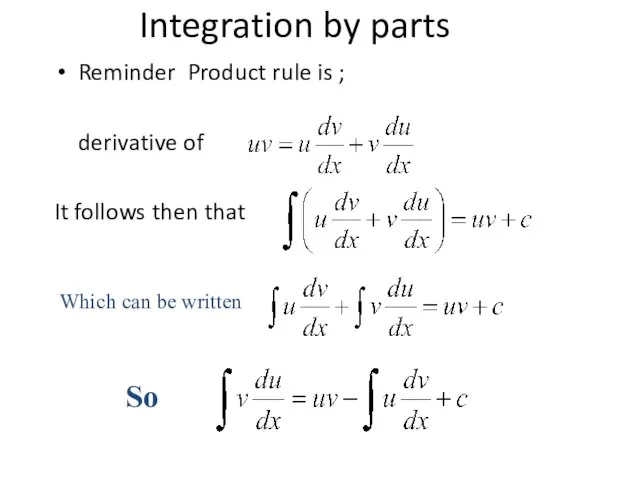
Integration by parts
Reminder Product rule is ;
derivative of
It follows then that
Which can be written

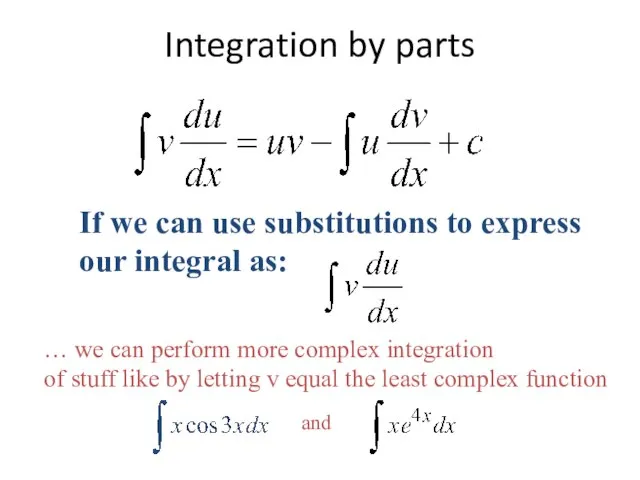
Integration by parts
… we can perform more complex integration
of stuff like by letting v equal the least complex function
and

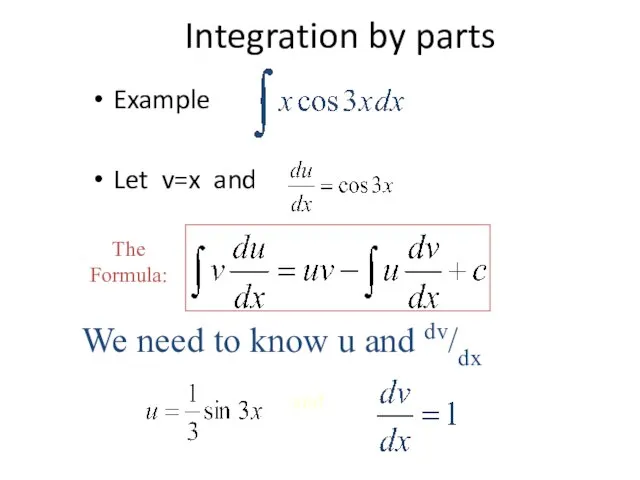
Integration by parts
Example
Let v=x and
We need to know u and dv/dx
The
Formula:
and

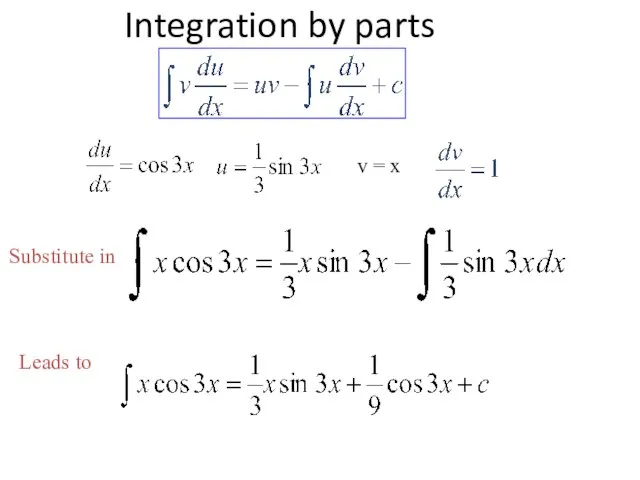
Integration by parts
Substitute in
Leads to

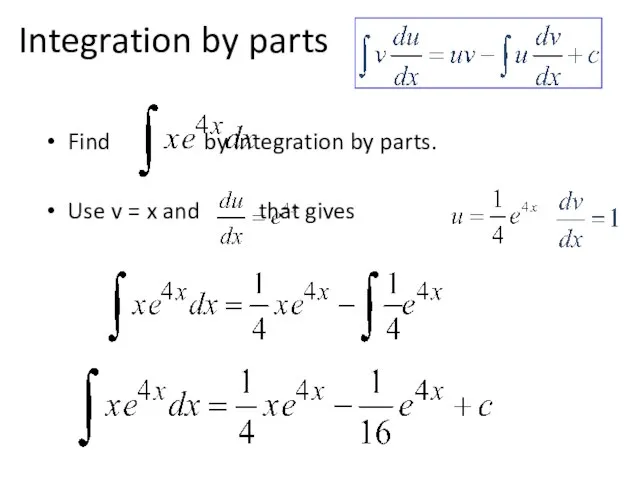
Integration by parts
Find by integration by parts.
Use v = x and that gives

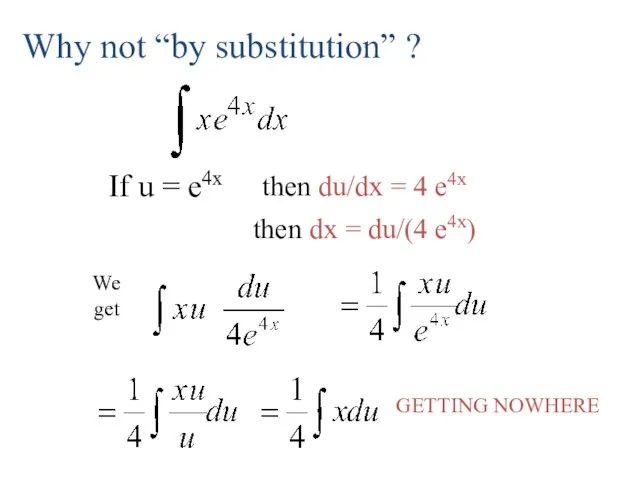
Why not “by substitution” ?
If u = e4x
then du/dx = 4 e4x
then dx = du/(4 e4x)
We
get
GETTING NOWHERE

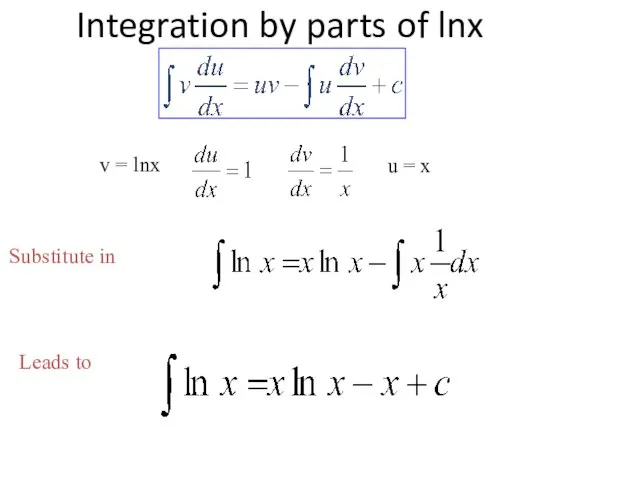
Integration by parts of lnx
Example
lnx is difficult to integrate so consider the function as 1lnx and use by parts.
Let v = lnx and
So and u = x

Integration by parts of lnx
Substitute in
Leads to

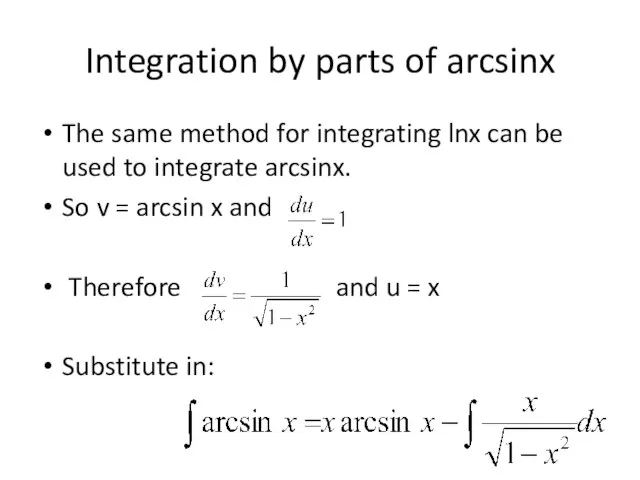
The same method for integrating lnx can be used to integrate arcsinx.
So v = arcsin x and
Therefore and u = x
Substitute in:
Integration by parts of arcsinx

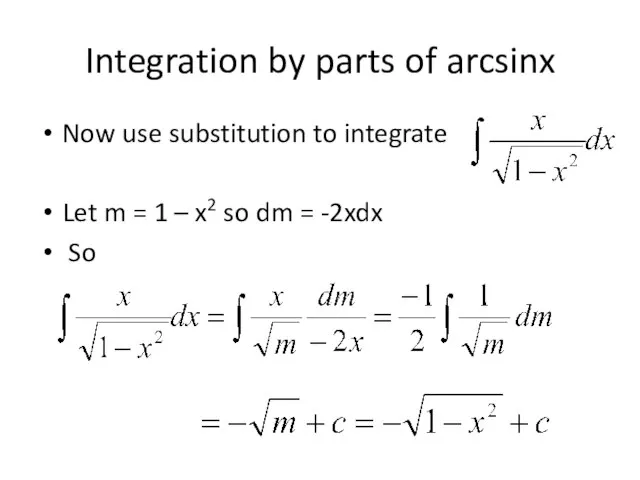
Integration by parts of arcsinx
Now use substitution to integrate
Let m = 1 – x2 so dm = -2xdx
So

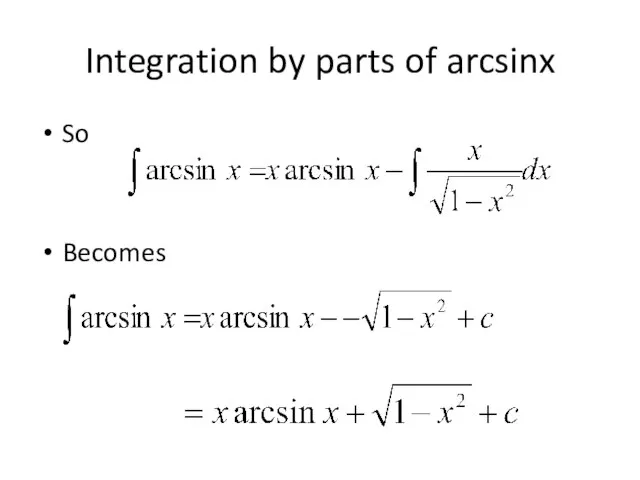
Integration by parts of arcsinx
So
Becomes

This involves some algebraic manipulation since the second integral does not resolve into an easily integratable function.
Let v = ex and
Therefore and u = sinx
So:
Integration by parts of excosx
[1]

Integrating by parts again for exsinx we get:
Rearranging:
Integration by parts of excosx
[2]