Содержание
- 2. Boolean functions
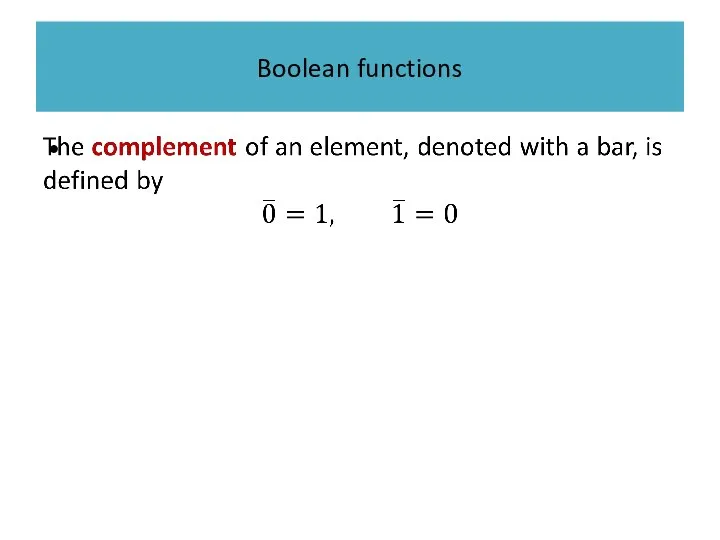
- 3. Boolean functions
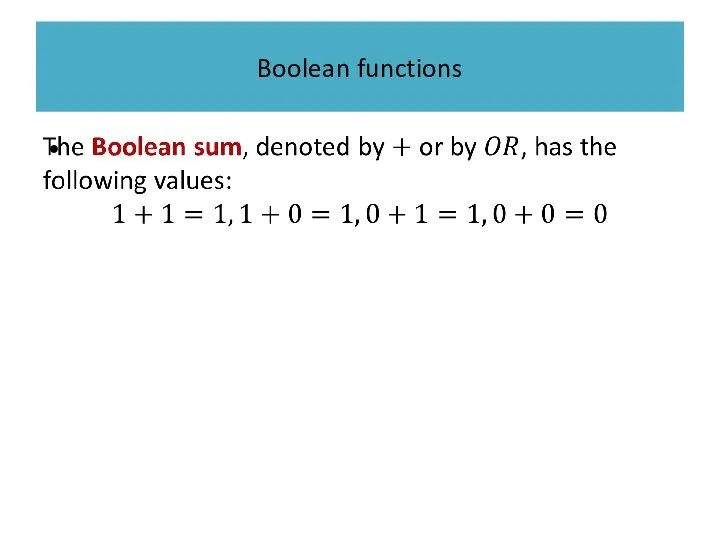
- 4. Boolean functions
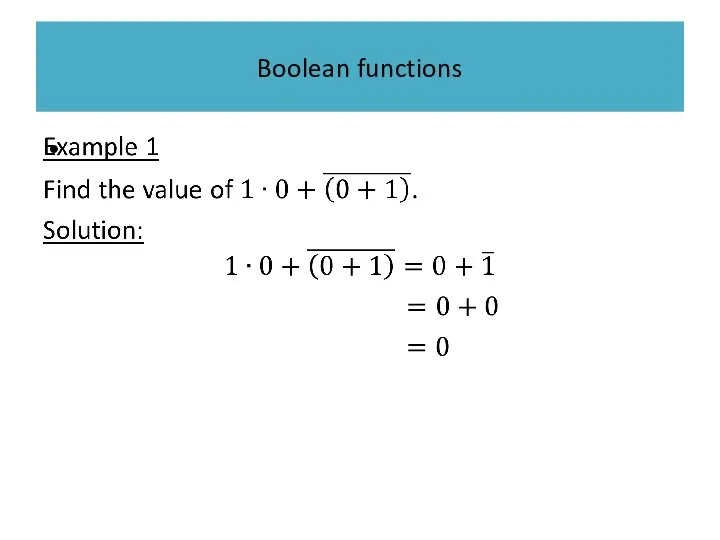
- 5. Boolean functions
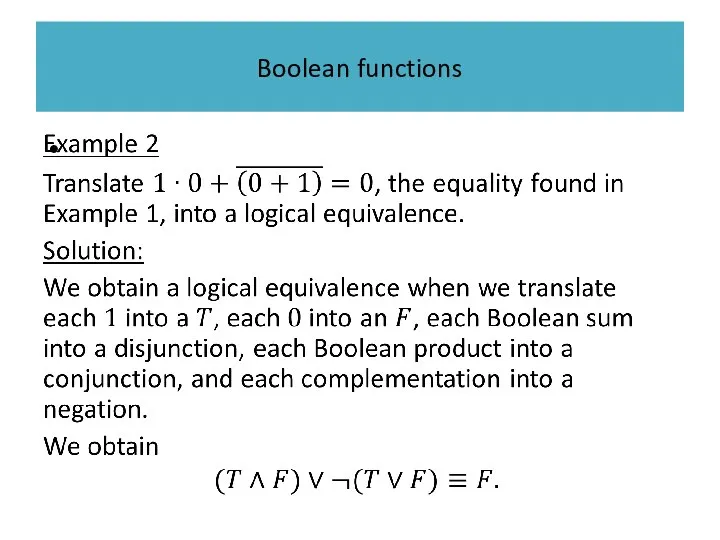
- 6. Boolean functions
- 7. Boolean functions
- 8. Boolean functions
- 9. Boolean functions
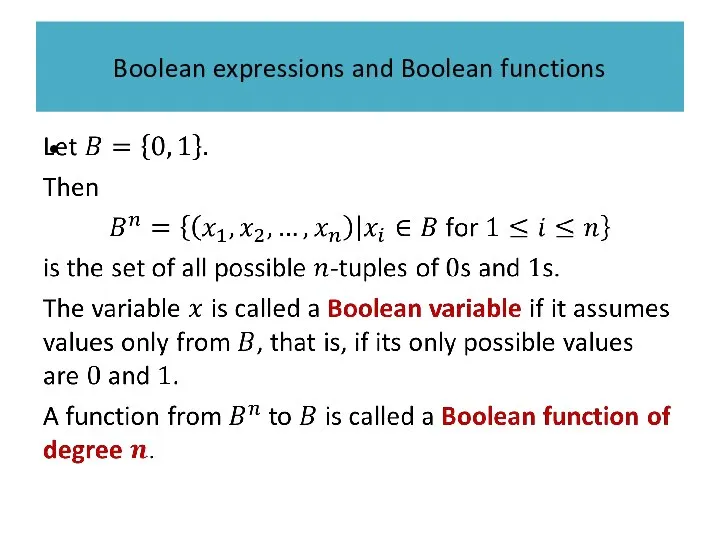
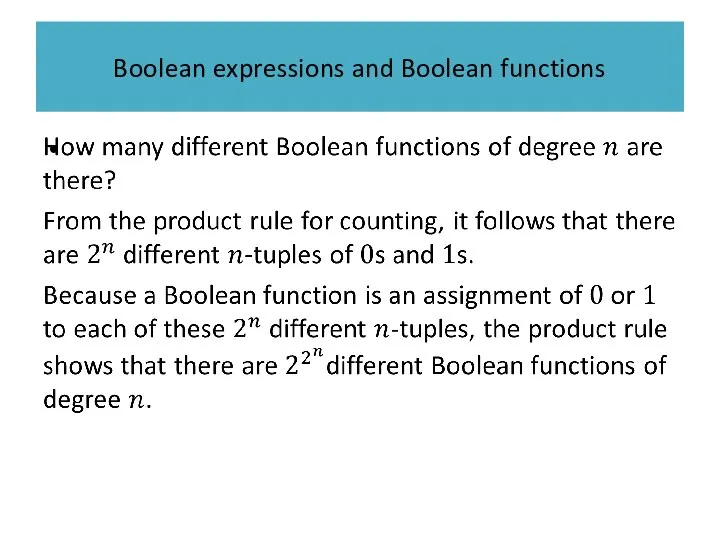
- 10. Boolean expressions and Boolean functions
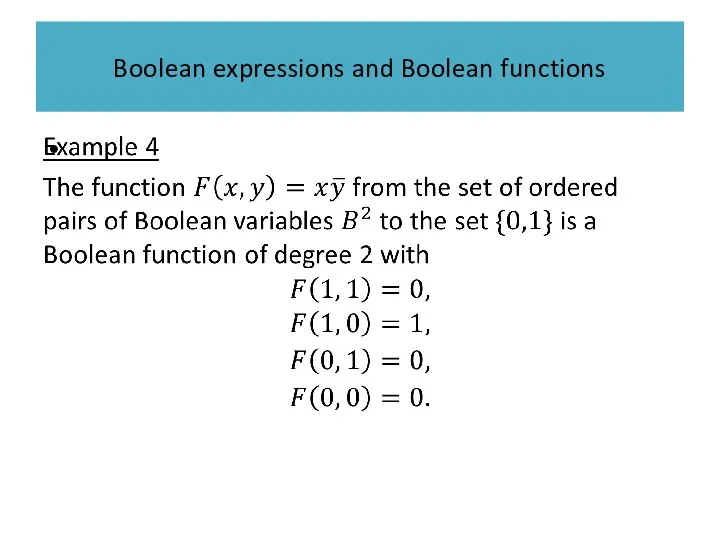
- 11. Boolean expressions and Boolean functions
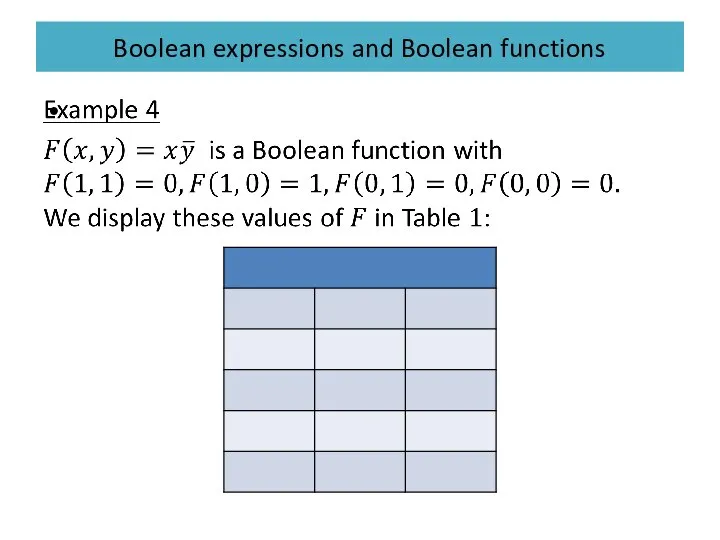
- 12. Boolean expressions and Boolean functions
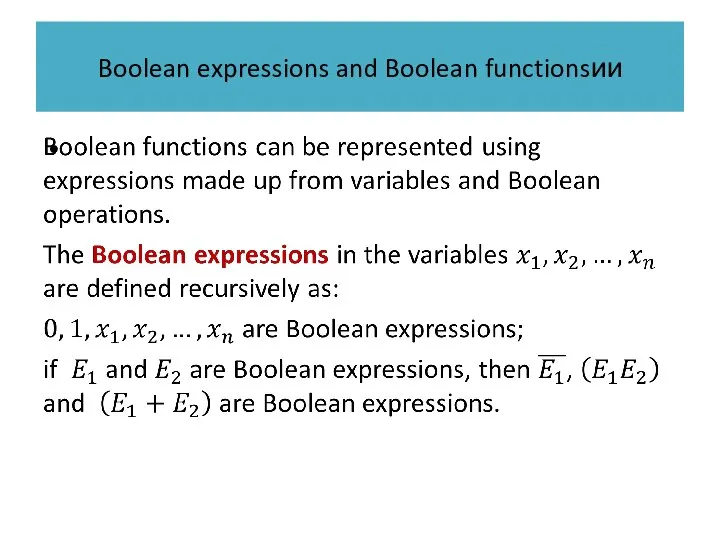
- 13. Boolean expressions and Boolean functionsии
- 14. Boolean expressions and Boolean functions
- 15. Boolean expressions and Boolean functions
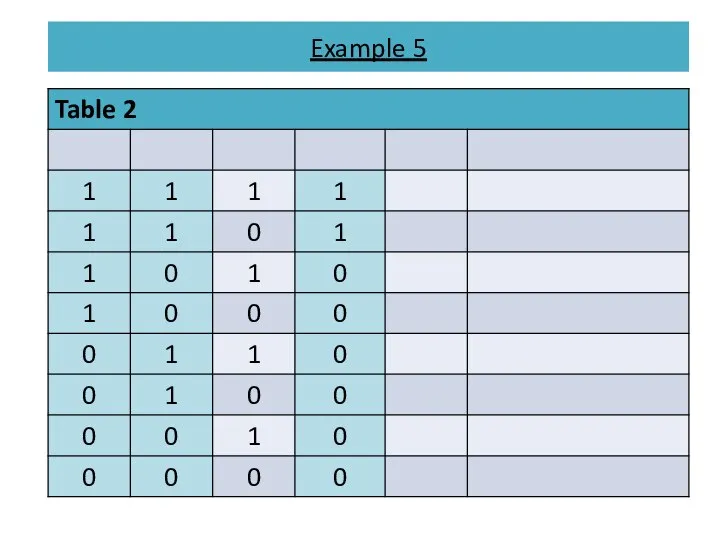
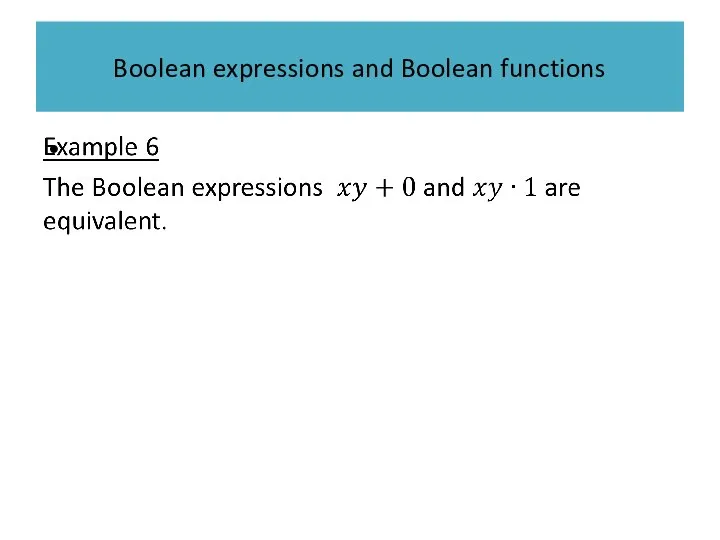
- 16. Example 5
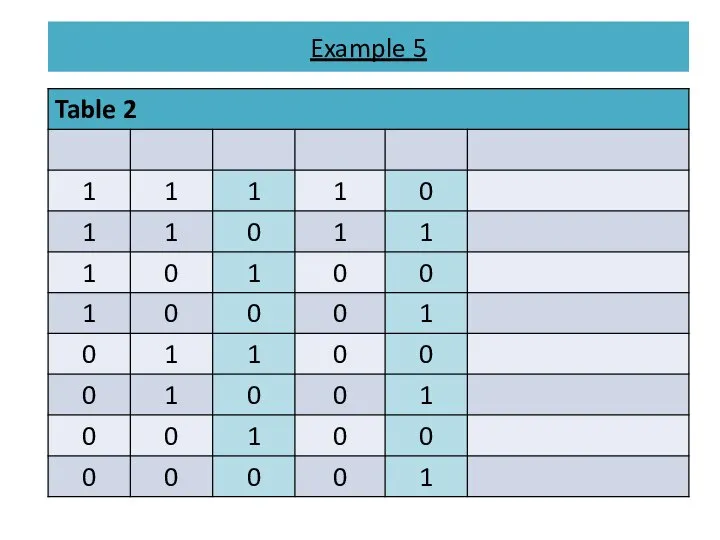
- 17. Example 5
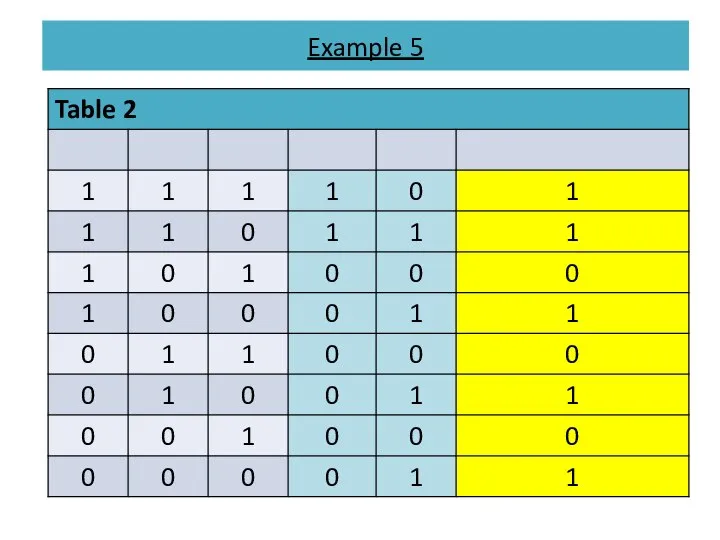
- 18. Example 5
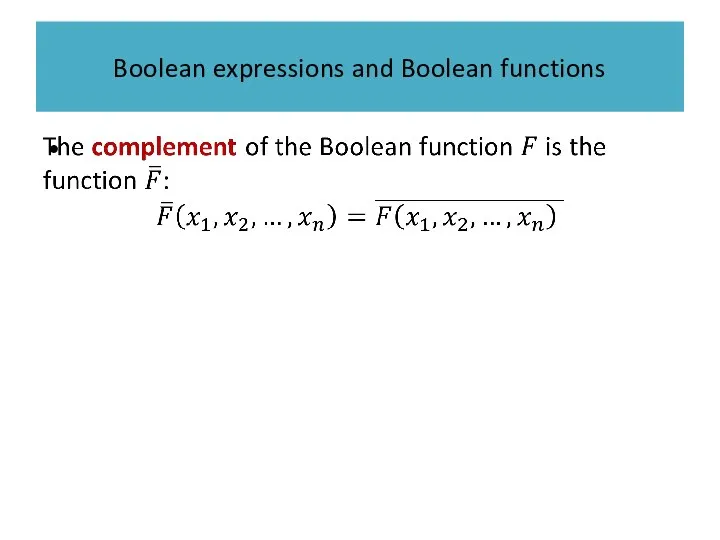
- 19. Boolean expressions and Boolean functions
- 20. Boolean expressions and Boolean functions
- 21. Boolean expressions and Boolean functions
- 22. Boolean expressions and Boolean functions
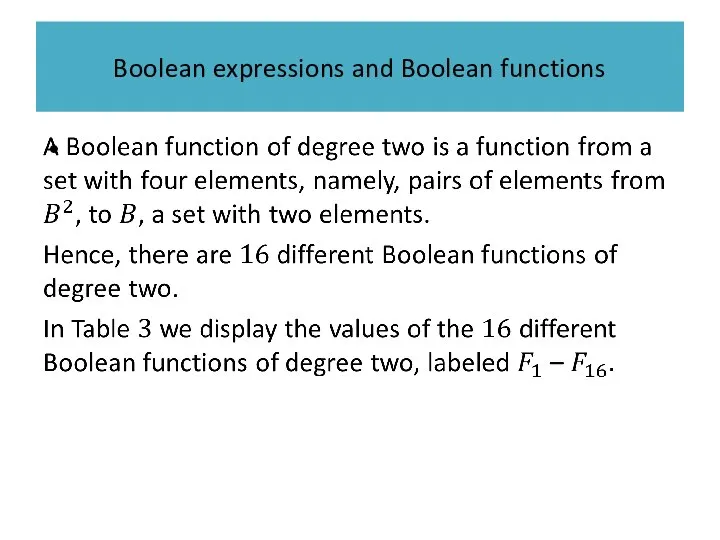
- 23. Boolean expressions and Boolean functions
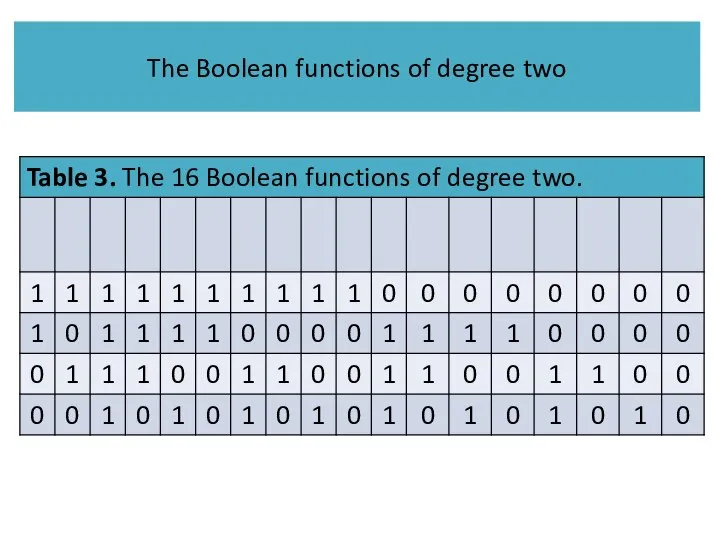
- 24. The Boolean functions of degree two
- 25. Boolean expressions and Boolean functions
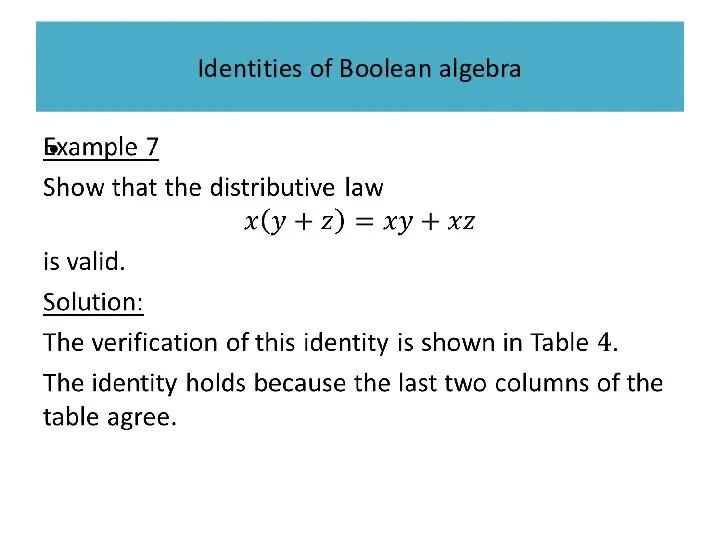
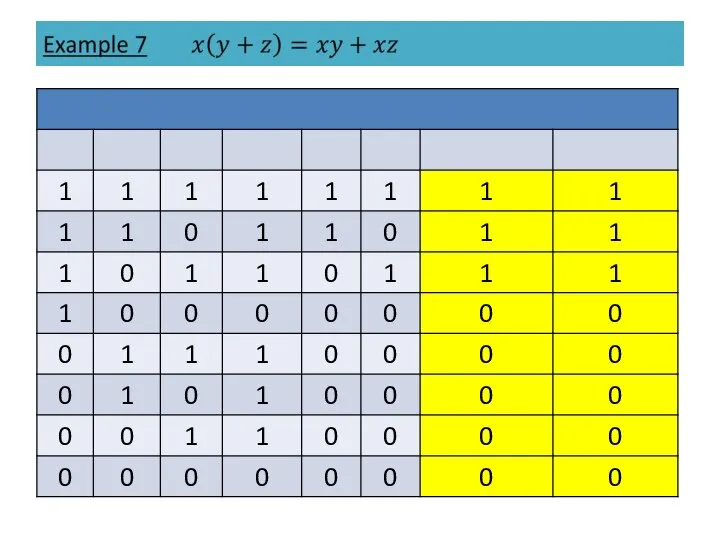
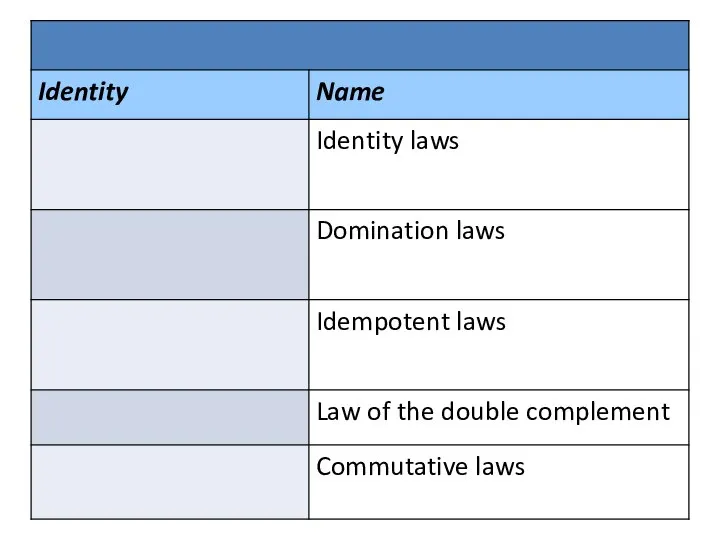
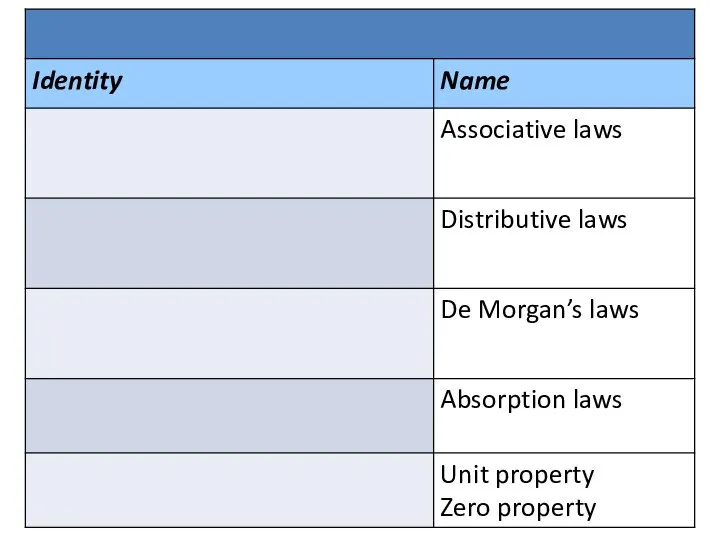
- 26. Identities of Boolean algebra
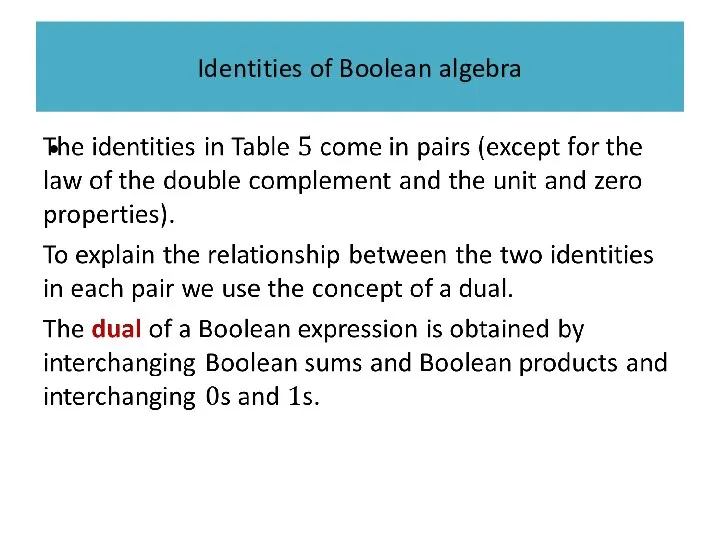
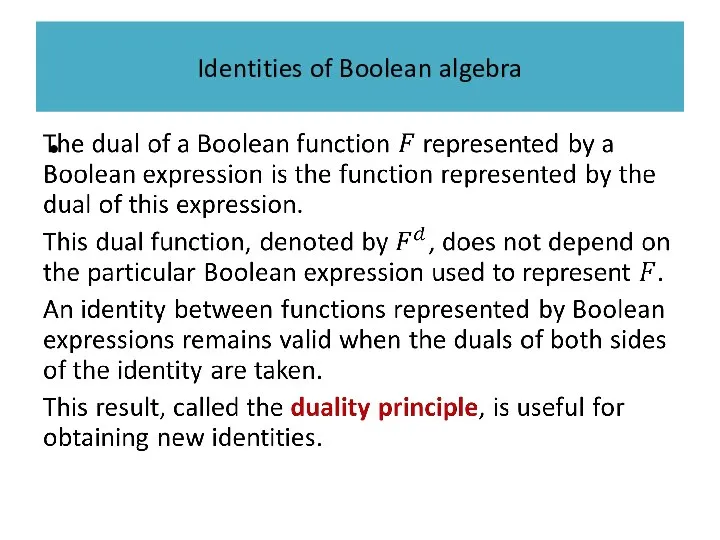
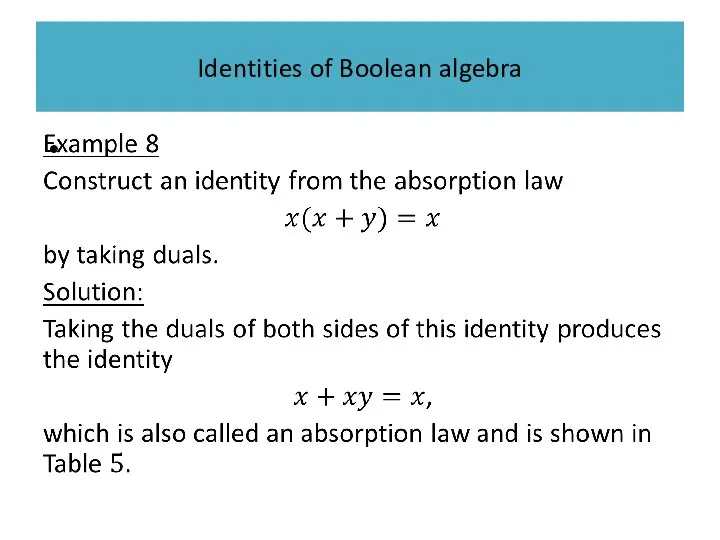
- 27. Identities of Boolean algebra
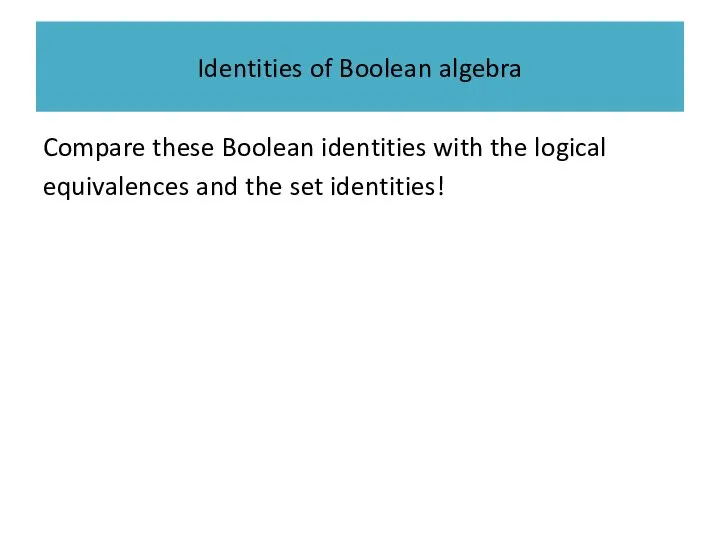
- 31. Identities of Boolean algebra Compare these Boolean identities with the logical equivalences and the set identities!
- 32. Identities of Boolean algebra
- 33. Identities of Boolean algebra
- 34. Identities of Boolean algebra
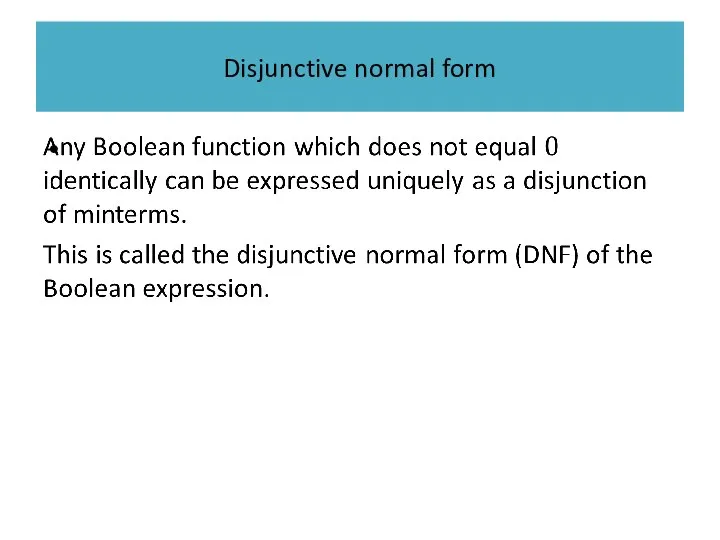
- 35. Disjunctive normal form We now show how any Boolean expression can be expressed in an equivalent
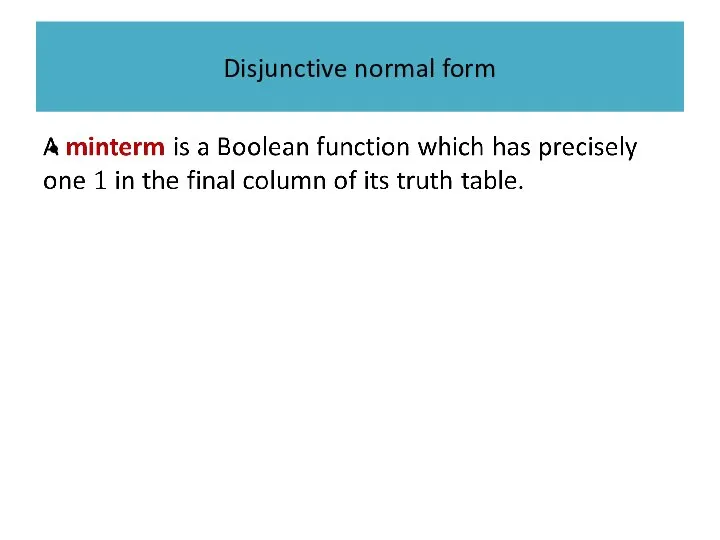
- 36. Disjunctive normal form
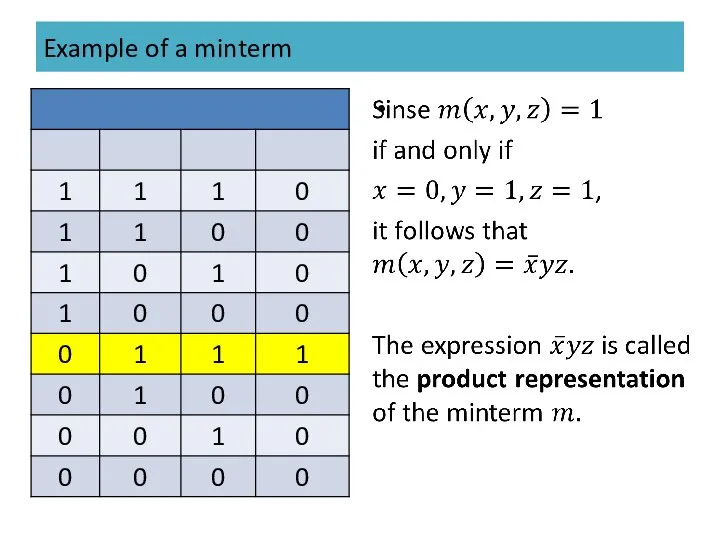
- 37. Example of a minterm
- 38. Disjunctive normal form
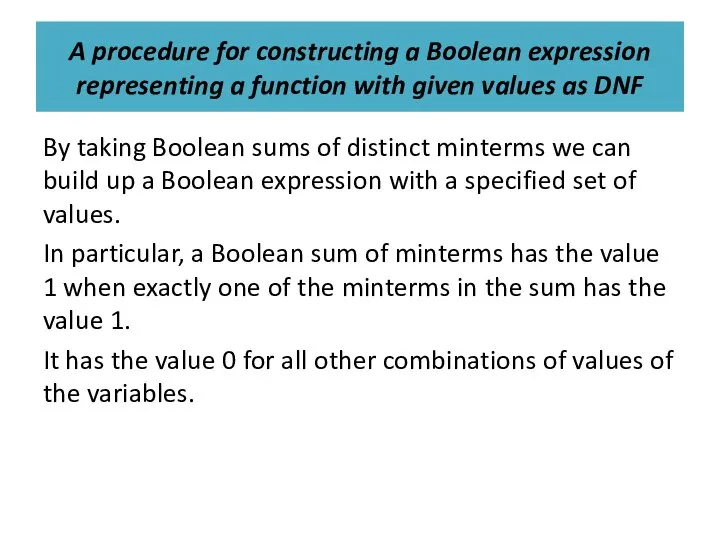
- 39. A procedure for constructing a Boolean expression representing a function with given values as DNF By
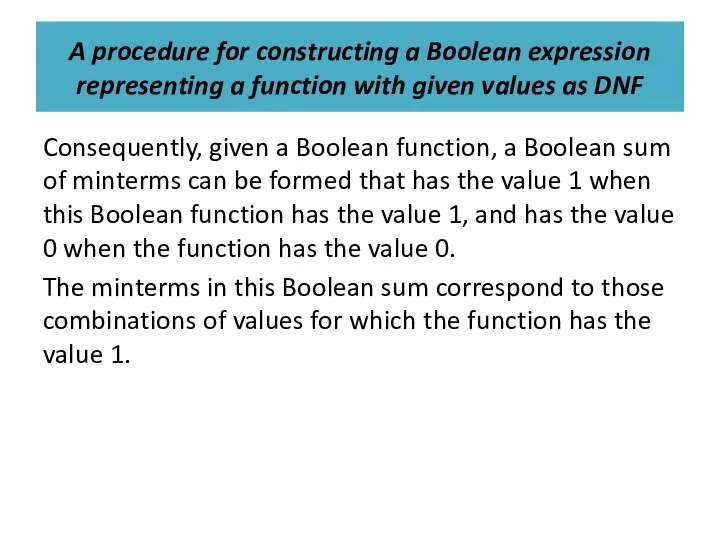
- 40. A procedure for constructing a Boolean expression representing a function with given values as DNF Consequently,
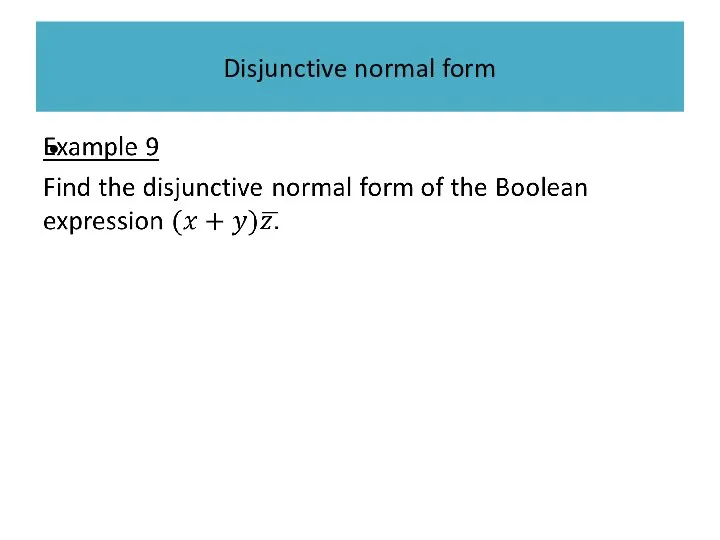
- 41. Disjunctive normal form
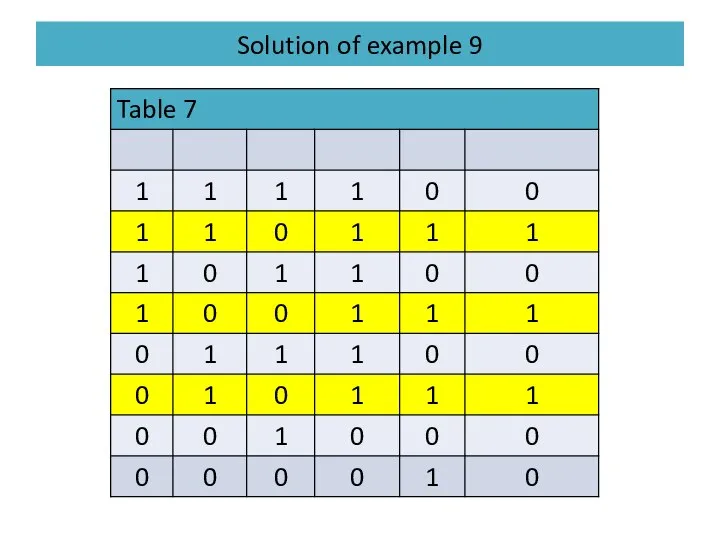
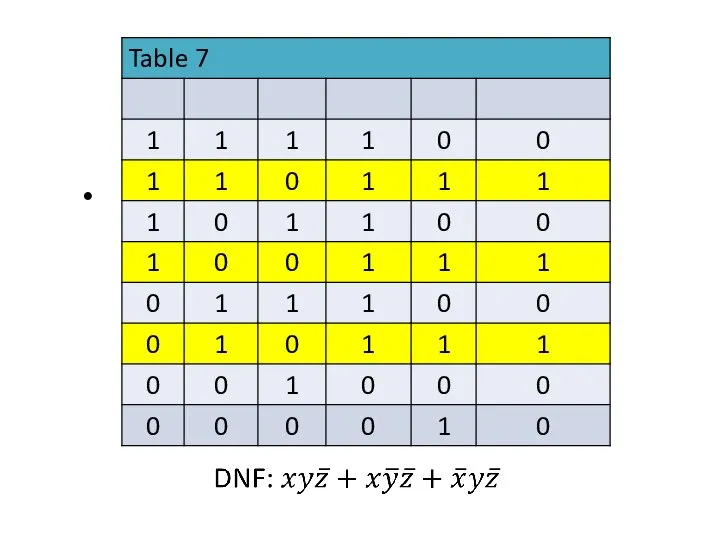
- 42. Solution of example 9
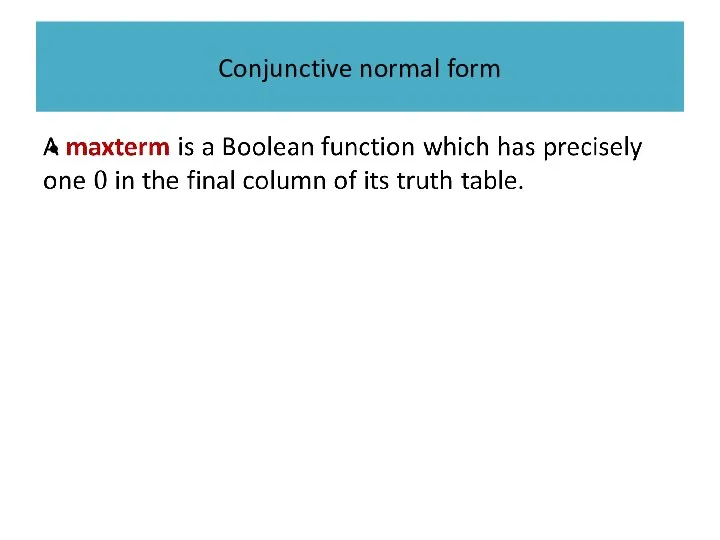
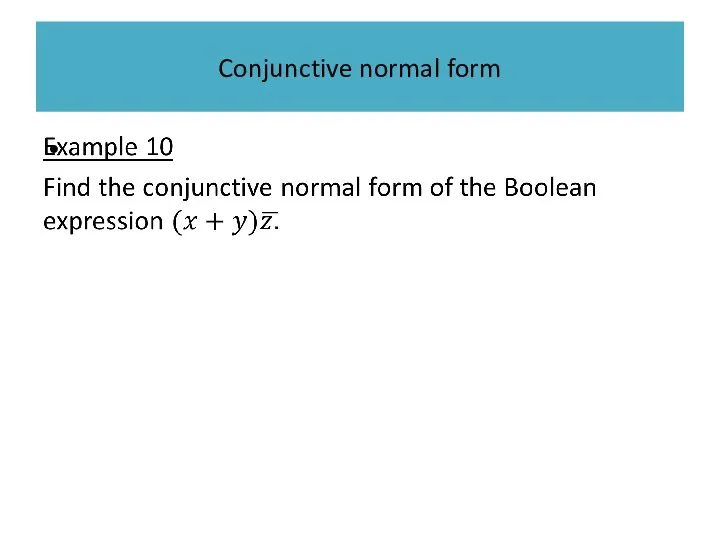
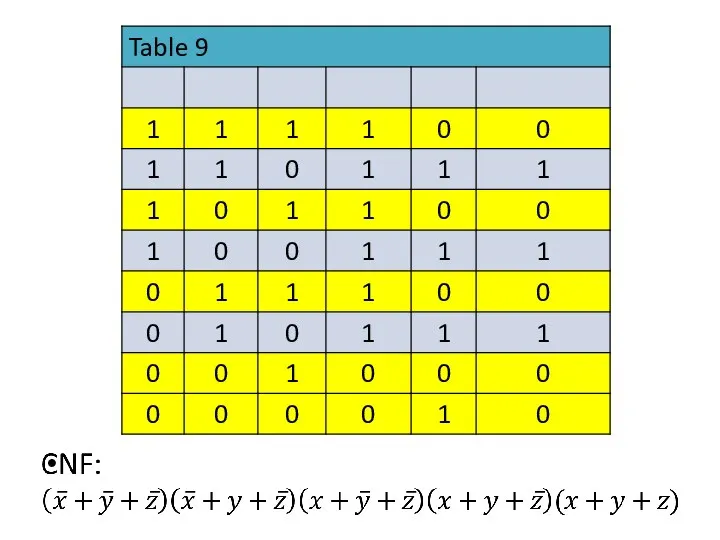
- 44. Conjunctive normal form
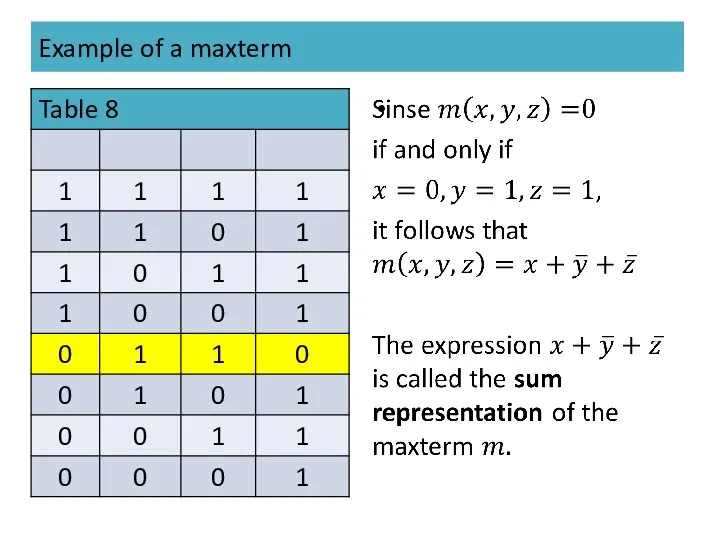
- 45. Example of a maxterm
- 46. Conjunctive normal form
- 47. A procedure for constructing a Boolean expression representing a function with given values as CNF By
- 48. A procedure for constructing a Boolean expression representing a function with given values as CNF Consequently,
- 49. Conjunctive normal form
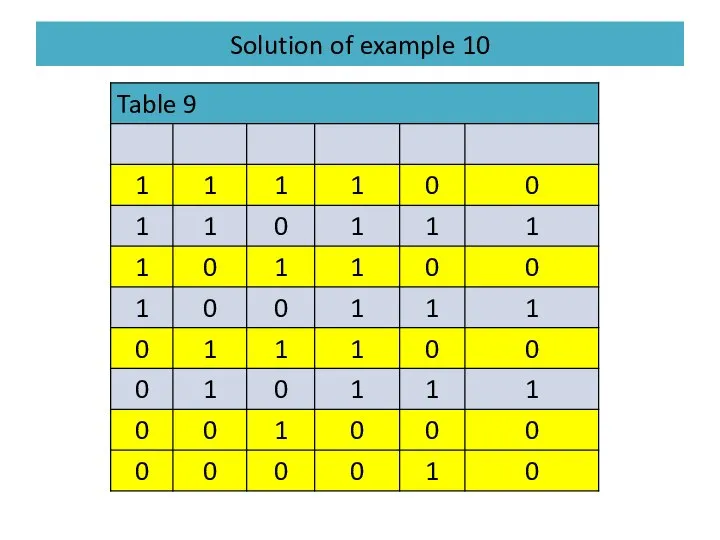
- 50. Solution of example 10
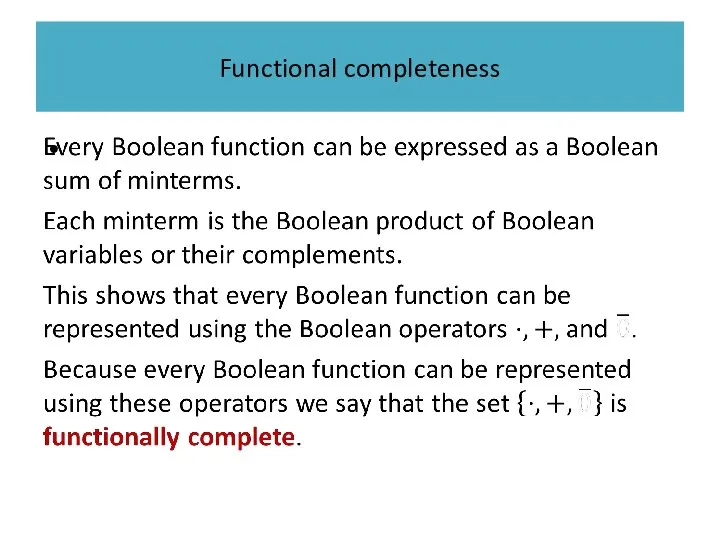
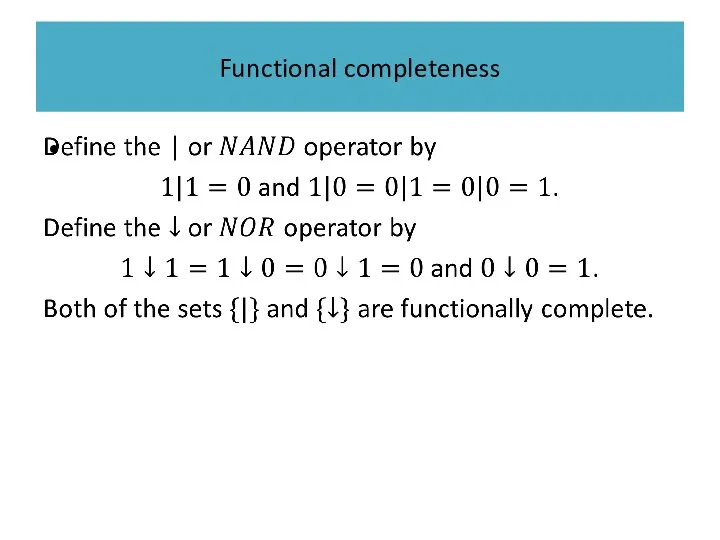
- 52. Functional completeness
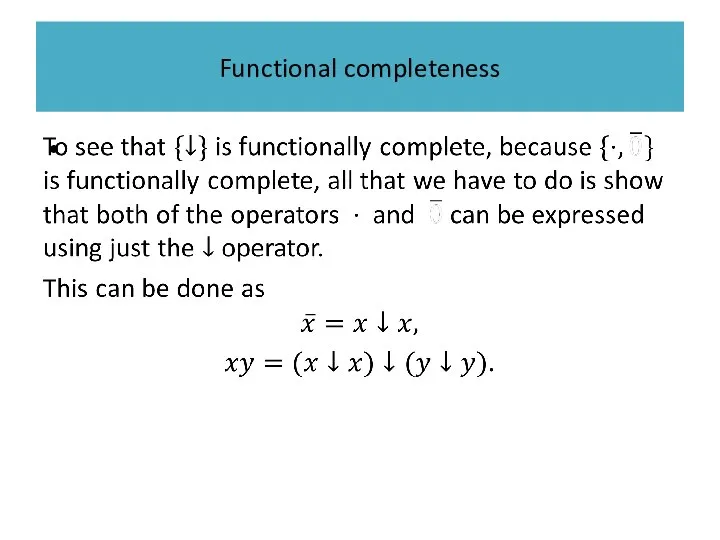
- 53. Functional completeness Can we find a smaller set of functionally complete operators? We can do so
- 54. Functional completeness
- 55. Functional completeness
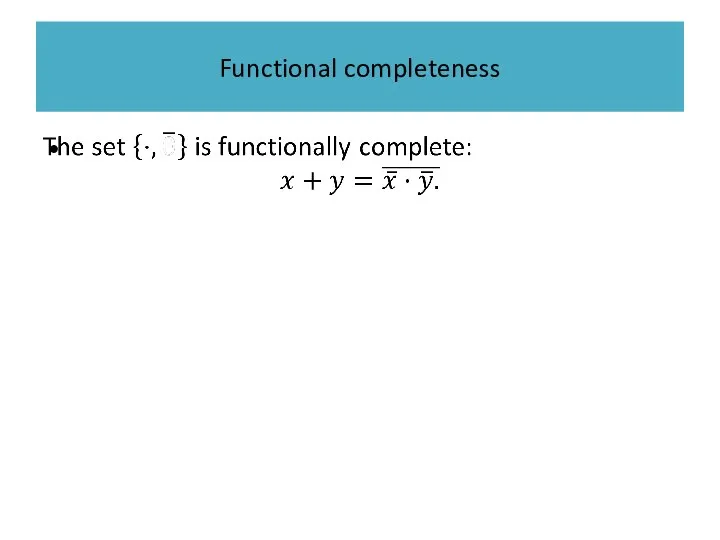
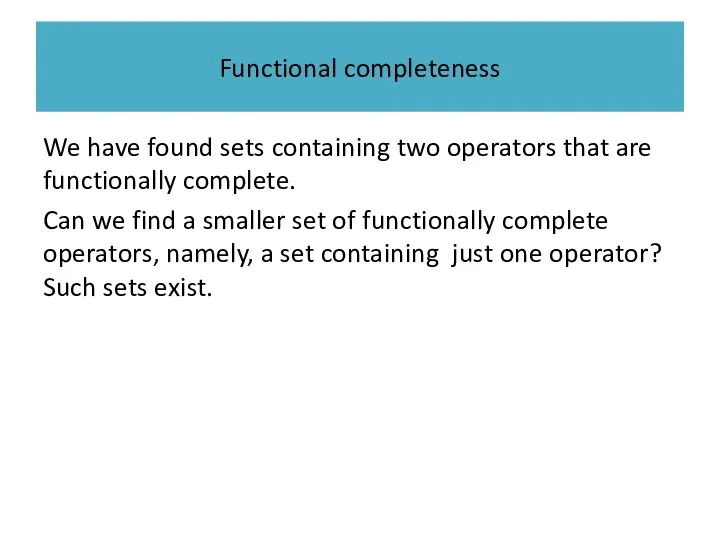
- 56. Functional completeness We have found sets containing two operators that are functionally complete. Can we find
- 57. Functional completeness
- 58. Functional completeness
- 59. Functional completeness
- 60. Biography George Boole, the son of a cobbler, was born in Lincoln, England, in November 1815.
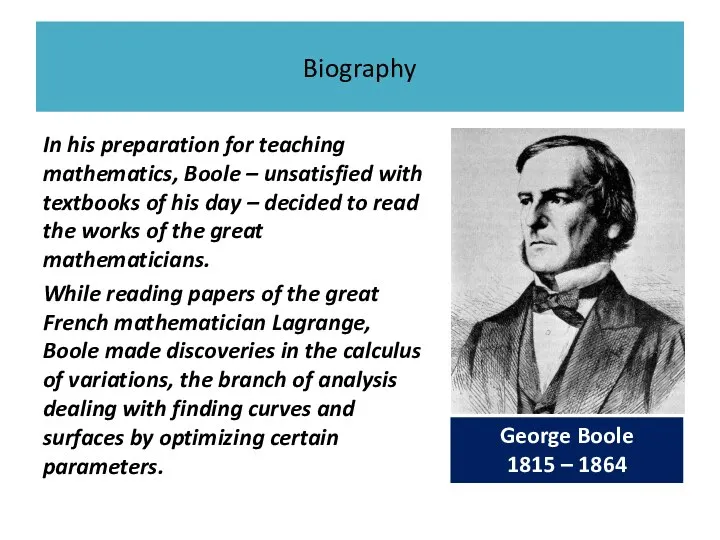
- 61. Biography In his preparation for teaching mathematics, Boole – unsatisfied with textbooks of his day –
- 62. Biography In 1848 Boole published The Mathematical Analysis of Logic, the first of his contributions to
- 64. Скачать презентацию












 Station. Theatre
Station. Theatre Native Americans
Native Americans Устная часть ЕГЭ
Устная часть ЕГЭ Present tenses
Present tenses At the circus! Spotlight 2
At the circus! Spotlight 2 Past simple tense
Past simple tense First conditionals sentences. Type 1, 2
First conditionals sentences. Type 1, 2 Cats Behavior
Cats Behavior Презентация на тему Jobs
Презентация на тему Jobs  Английские числительные 20-100
Английские числительные 20-100 Глагол To Have Got (иметь)
Глагол To Have Got (иметь) Solve puzzles
Solve puzzles Give a talk about music (12-15 sentences)
Give a talk about music (12-15 sentences) Veterans day
Veterans day Presentation on my favorite movie theme
Presentation on my favorite movie theme Economic Community of West African States (ECOWAS)
Economic Community of West African States (ECOWAS) Приложение Present Tenses
Приложение Present Tenses I can jump. Spotlight 2
I can jump. Spotlight 2 Australia Slang
Australia Slang How to build a dialog with English-speaking possible friend
How to build a dialog with English-speaking possible friend Plurals. Game
Plurals. Game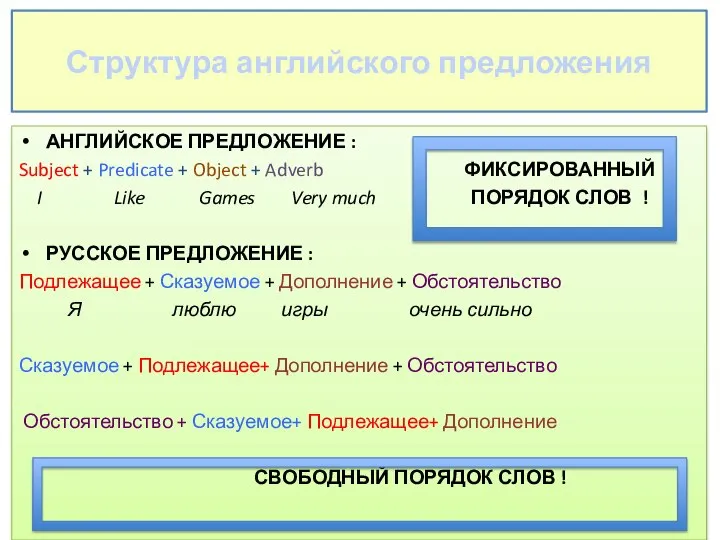 Структура английского предложения
Структура английского предложения I like the cat. Love
I like the cat. Love Konotop. The city in which we live
Konotop. The city in which we live Презентация на тему The Bronte sisters
Презентация на тему The Bronte sisters  My ideal neighbourhood
My ideal neighbourhood Grammar issues
Grammar issues Crimes and criminals
Crimes and criminals