Содержание
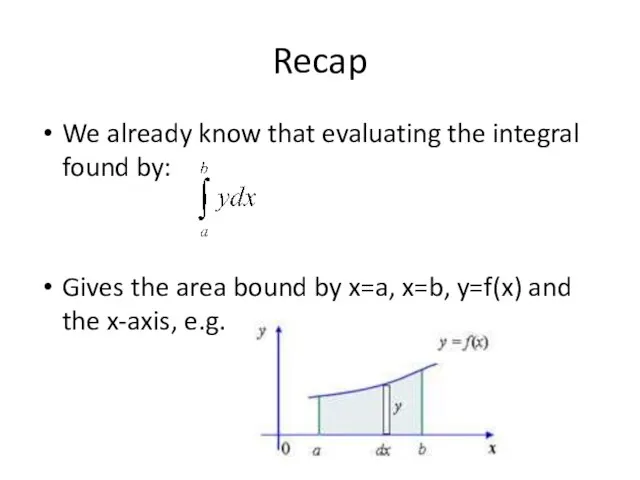
- 2. Recap We already know that evaluating the integral found by: Gives the area bound by x=a,
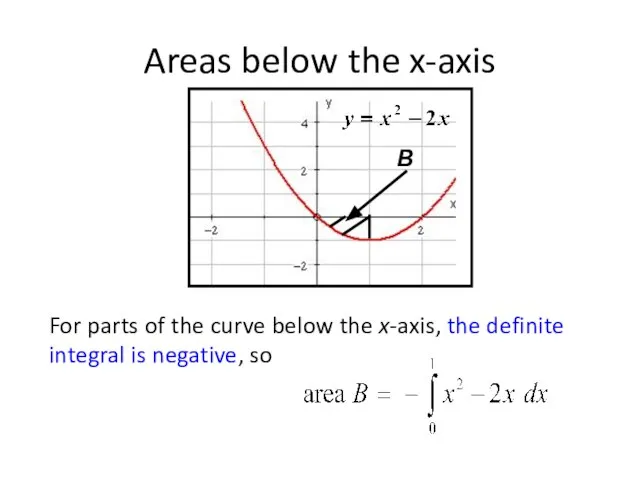
- 3. Areas below the x-axis
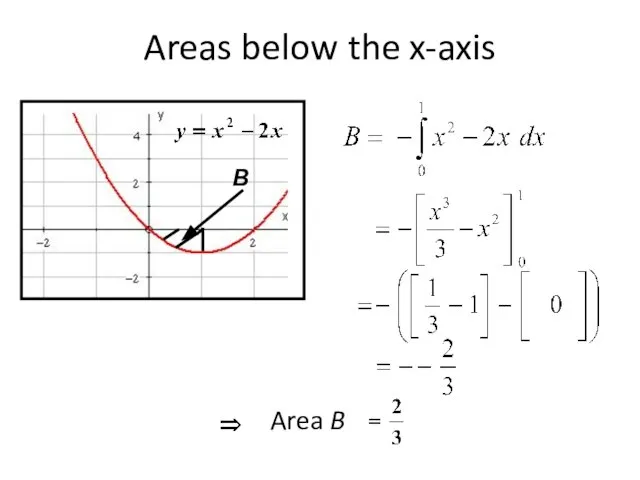
- 4. Areas below the x-axis
- 5. An area is always positive. The definite integral is positive for areas above the x-axis but
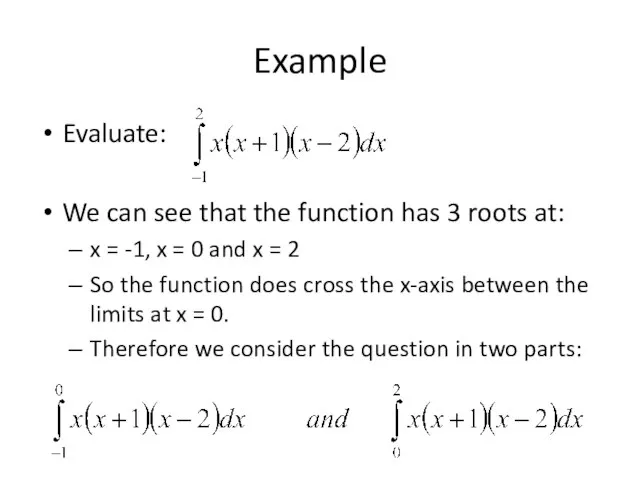
- 6. Example Evaluate: We can see that the function has 3 roots at: x = -1, x
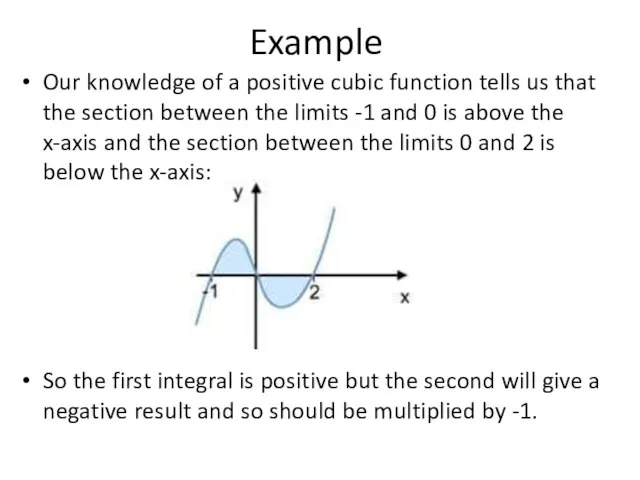
- 7. Example Our knowledge of a positive cubic function tells us that the section between the limits
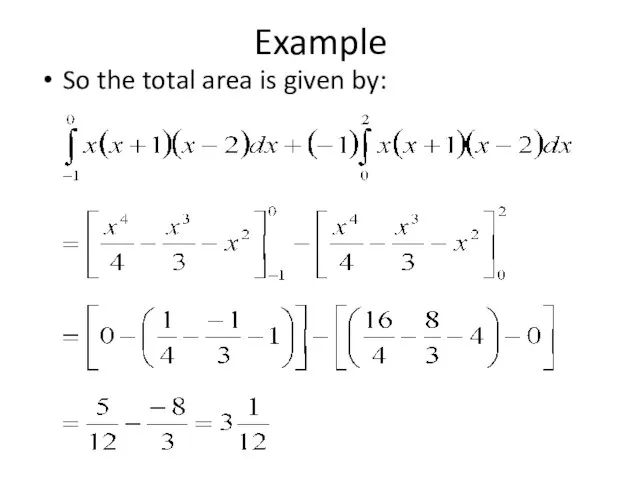
- 8. Example So the total area is given by:
- 9. Areas bound with the y-axis We can also evaluate areas bound by y=a, y=b, x=f(y) and
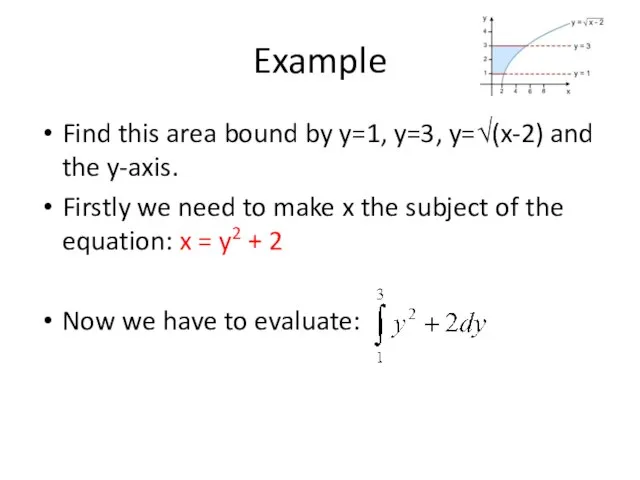
- 10. Example Find this area bound by y=1, y=3, y=√(x-2) and the y-axis. Firstly we need to
- 12. Скачать презентацию


 Конструирование прямой юбки
Конструирование прямой юбки Деятельность регионального органа управления образованием
Деятельность регионального органа управления образованием Название проекта. Дополнительная информация
Название проекта. Дополнительная информация Сергей Фёдорович Бондарчук
Сергей Фёдорович Бондарчук ITIL. ITSM. Введение
ITIL. ITSM. Введение Благодарность медицинским работникам
Благодарность медицинским работникам Исследование состояния здоровьесберегающей и здоровьеформирующей среды образовательного учреждения глазами учащихся школы.
Исследование состояния здоровьесберегающей и здоровьеформирующей среды образовательного учреждения глазами учащихся школы. Мир художественной культуры
Мир художественной культуры Политические партии
Политические партии  Путешествие в ледниковый период (часть 2)
Путешествие в ледниковый период (часть 2) gazprom (1)
gazprom (1) Предприятия с наибольшим выпуском мехов и меховых изделий: Татарское меховое ОАО «Мелита»; АООТ «Русский мех» (г.Москва); ООО «Бел
Предприятия с наибольшим выпуском мехов и меховых изделий: Татарское меховое ОАО «Мелита»; АООТ «Русский мех» (г.Москва); ООО «Бел Специальность 060101 «лечебное дело»Дисциплина «терапия» 4 курс 7 семестрПрезентацияпо терапиина тему:«Заболевания кровии крове
Специальность 060101 «лечебное дело»Дисциплина «терапия» 4 курс 7 семестрПрезентацияпо терапиина тему:«Заболевания кровии крове Т 3.1. Марш МВ
Т 3.1. Марш МВ Презентация на тему Природа в искусстве
Презентация на тему Природа в искусстве  Архитипический профиль Нижнего Новгорода
Архитипический профиль Нижнего Новгорода Кузьма Минин и Дмитрий Пожарский
Кузьма Минин и Дмитрий Пожарский «ЭЛЕКТРОННАЯ БОЛЬНИЦА»
«ЭЛЕКТРОННАЯ БОЛЬНИЦА» Modern epoch
Modern epoch  Лекция 2Стандарты ЕСКД. Оформление чертежей
Лекция 2Стандарты ЕСКД. Оформление чертежей Интерстициальное инфракрасное лазерное воздействие – эффективный органосохраняющий метод лечения доброкачественных узловых об
Интерстициальное инфракрасное лазерное воздействие – эффективный органосохраняющий метод лечения доброкачественных узловых об Социальная психология
Социальная психология Презентация на тему Лесная промышленность России
Презентация на тему Лесная промышленность России  Иван Бунин о любви
Иван Бунин о любви Психология корпоративного менеджмента. Новые модели человека
Психология корпоративного менеджмента. Новые модели человека Геометрия это наука
Геометрия это наука Георгий Алексеевич Скребицкий и Геннадий Яковлевич Снегирев
Георгий Алексеевич Скребицкий и Геннадий Яковлевич Снегирев Львова Наталья, руководитель проекта DOCFLOW
Львова Наталья, руководитель проекта DOCFLOW