Содержание
- 2. Rotating about a line parallel to the x or y axis The area to be rotated
- 3. Example 1 Consider the region bound by: y = 4x – x2 y = 3 Find
- 4. Rotating between two curves about the x or y axis We use the same theory we
- 5. Example 2 Consider the region bound by: y = 4x – x2 y = 3 Find
- 6. Rotating between two curves about a line parallel to the x or y axis We now
- 7. Example 3 Consider the region in the first quadrant bound by: y = x2 + 1
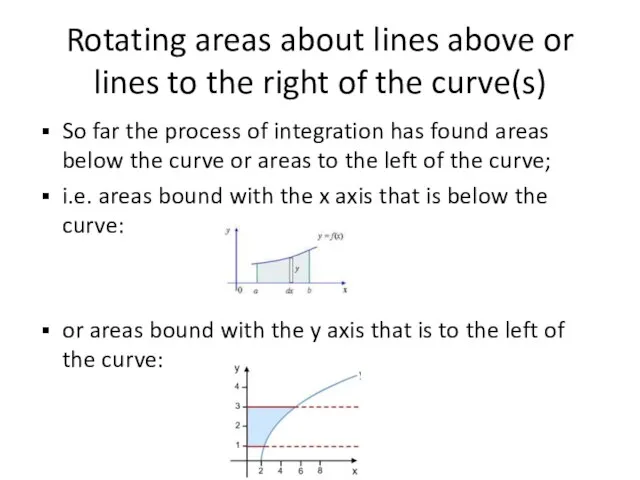
- 8. Rotating areas about lines above or lines to the right of the curve(s) So far the
- 9. Rotating areas about lines above or lines to the right of the curve(s) Following on to
- 10. Rotating areas about lines above or lines to the right of the curve(s) Now consider when
- 12. Скачать презентацию







 Сказочная птица
Сказочная птица Да здравствует спорт и свежий воздух
Да здравствует спорт и свежий воздух Презентация на тему Химия в косметике
Презентация на тему Химия в косметике Профориентационная работа Профориентационная работа с учащимися.
Профориентационная работа Профориентационная работа с учащимися. УСЛОВИЯПРОХОЖДЕНИЯ В.СПО КОНТРАКТУ. ТРЕБОВАНИЯ. СРОКИ В.С., ПРАВА И ЛЬГОТЫ.
УСЛОВИЯПРОХОЖДЕНИЯ В.СПО КОНТРАКТУ. ТРЕБОВАНИЯ. СРОКИ В.С., ПРАВА И ЛЬГОТЫ. Рейтинг группы 2743
Рейтинг группы 2743 Человек и закон. Конкурс лучших детективов имени Шерлока Холмса
Человек и закон. Конкурс лучших детективов имени Шерлока Холмса Презентация на тему Подготовка к ЕГЭ Комплексный анализ текста
Презентация на тему Подготовка к ЕГЭ Комплексный анализ текста  Корректировки мойки сатин
Корректировки мойки сатин Литература 30-х – начала 40-х годов
Литература 30-х – начала 40-х годов Спортивный праздник Мы близки к природе
Спортивный праздник Мы близки к природе Металлические поверхности
Металлические поверхности От социального заказа к построению системы муниципального образования инновационного характера
От социального заказа к построению системы муниципального образования инновационного характера Лекарственные растения
Лекарственные растения Этот удивительный чай!
Этот удивительный чай! Презентация на тему Компьютерные вирусы (8 класс)
Презентация на тему Компьютерные вирусы (8 класс) Мария I Тюдор
Мария I Тюдор Воздух, которым мы дышим
Воздух, которым мы дышим Монастырь
Монастырь вещества клетки
вещества клетки Реализация ФГОС. Стандарты второго поколения
Реализация ФГОС. Стандарты второго поколения Процессы и управление процессами
Процессы и управление процессами Основные потребители
Основные потребители Презентация на тему Рабдовирусы Возбудители бешенства
Презентация на тему Рабдовирусы Возбудители бешенства Ликвидация радиоактивного и химического заражения
Ликвидация радиоактивного и химического заражения Презентация на тему Барельеф Изобразительное искусство
Презентация на тему Барельеф Изобразительное искусство Образ Тома Сойера в романе Марка Твена «Приключения Тома Сойера»
Образ Тома Сойера в романе Марка Твена «Приключения Тома Сойера» Управление корпорацией
Управление корпорацией