Volumes of Revolution

Consider the line y=3x
Now rotate the line 360° about the x axis
.
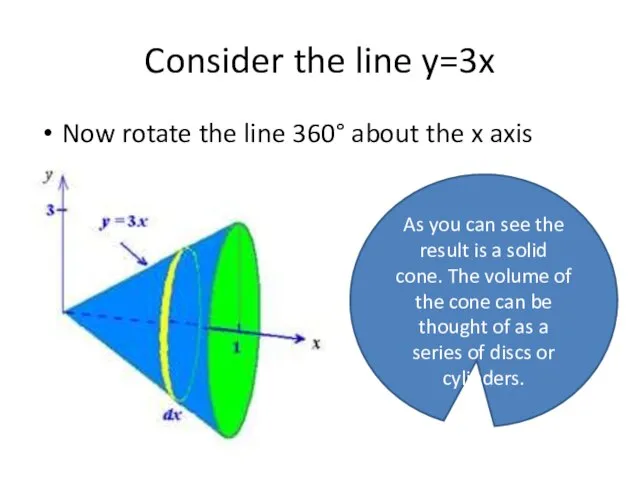
As you can see the result is a solid cone. The volume of the cone can be thought of as a series of discs or cylinders.

Volume of a cylinder
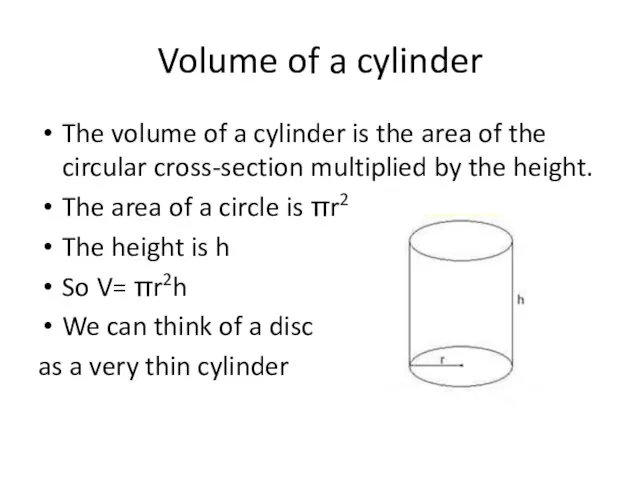
The volume of a cylinder is the area of the circular cross-section multiplied by the height.
The area of a circle is πr2
The height is h
So V= πr2h
We can think of a disc
as a very thin cylinder

Consider a small disc in the cone
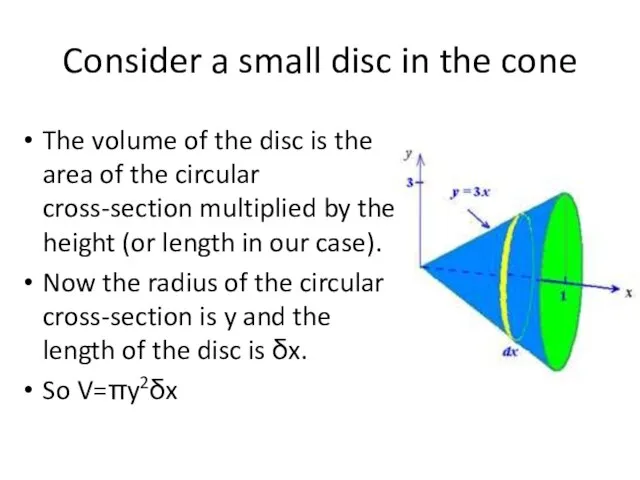
The volume of the disc is the area of the circular cross-section multiplied by the height (or length in our case).
Now the radius of the circular cross-section is y and the length of the disc is δx.
So V=πy2δx

Integration as a process of summation
We have seen that integration is a process of summation that adds a series of very small strips to give an area.
This process can also be used to add a series of very small discs.
As with areas, as δx→0, the limit of the sum gives the result that the volume of revolution about the x axis is given by

Volumes of revolution example
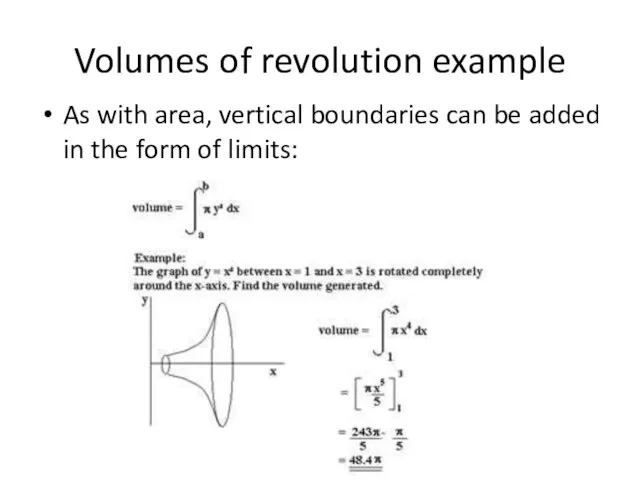
As with area, vertical boundaries can be added in the form of limits:

General formula
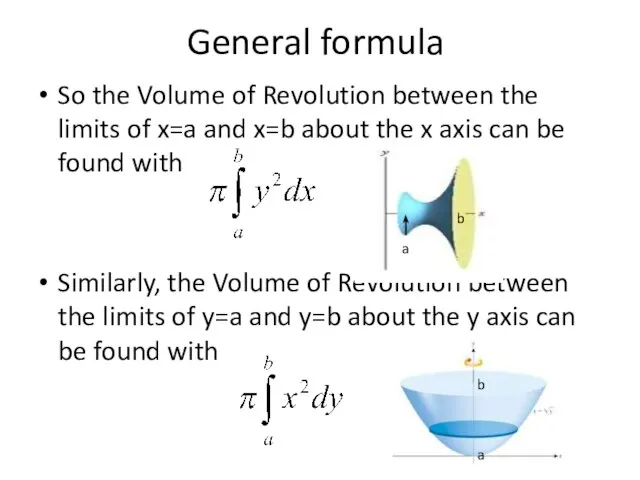
So the Volume of Revolution between the limits of x=a and x=b about the x axis can be found with
Similarly, the Volume of Revolution between the limits of y=a and y=b about the y axis can be found with

Example 1
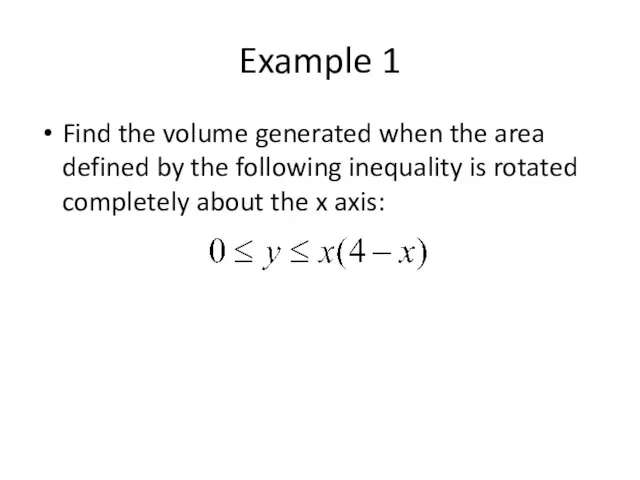
Find the volume generated when the area defined by the following inequality is rotated completely about the x axis:

Example 1 Solution
So our four boundary equations are:
x-axis
curve
x-axis intercept
x-axis intercept

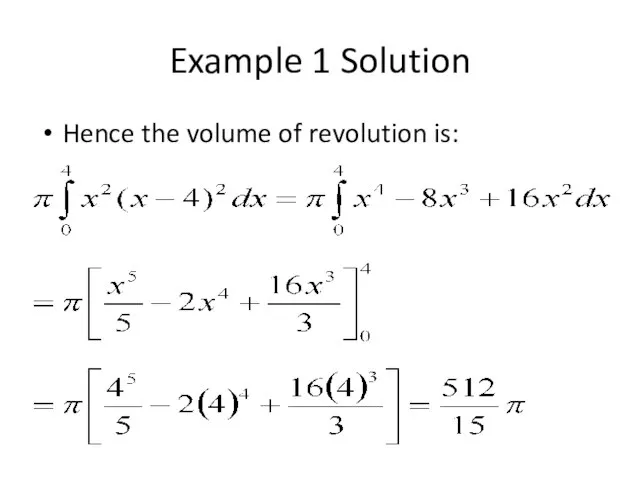
Example 1 Solution
Hence the volume of revolution is:

Example 2
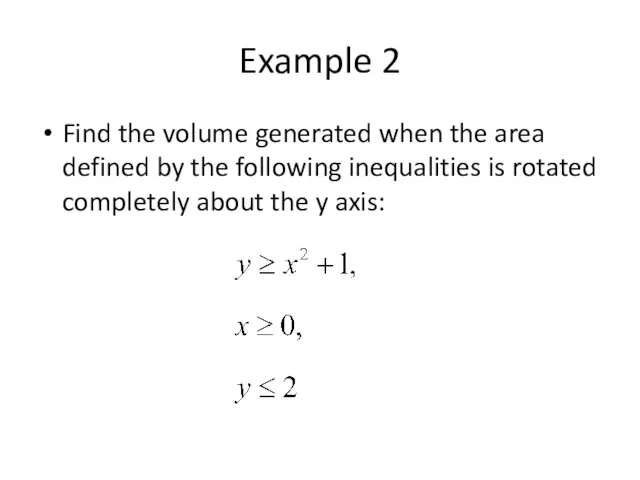
Find the volume generated when the area defined by the following inequalities is rotated completely about the y axis:

Example 2 Solution
So our four boundary equations are:
y-axis
curve
y-axis intercept
line
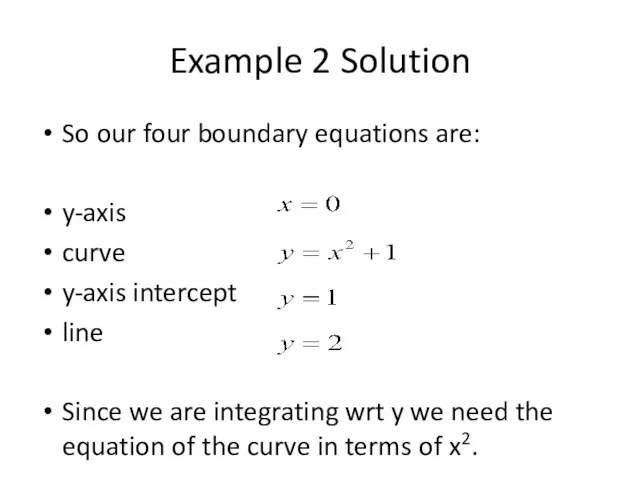
Since we are integrating wrt y we need the equation of the curve in terms of x2.