Macroeconomics 7

Let remember one important picture…
Potential output
Actual output
Economic growth can be treated as
an increase of potential GDP
Q
t (in years)

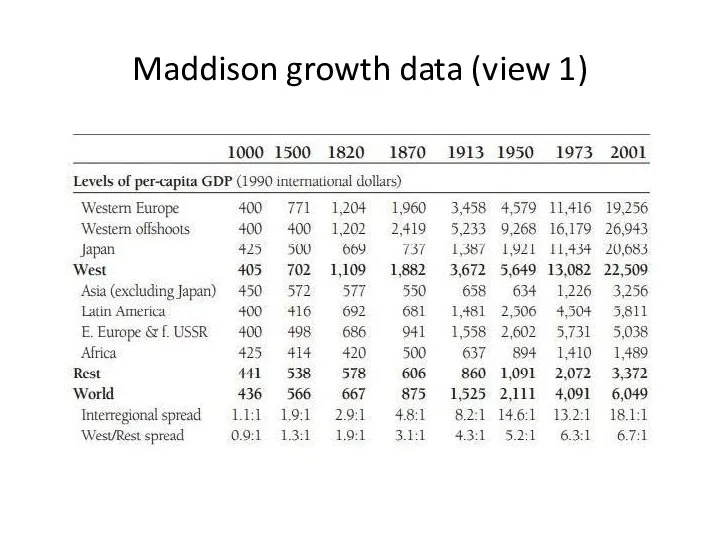
Maddison growth data (view 1)

Perhaps, you heard in the introductory course about…
hockey stick of economic progress!

Maddison growth data (view 2)
!

Economic Theory of the XIX Century: An example of “Outdated” View on Growth – part 1
Ricardo (1817) and Mill (1848) believed that rate of economic growth decreases in the course of time.
Ricardo and Mill developed theory based on “the Law of Diminishing Fertility of the Soil” or growth in the conditions of decreasing returns to scale.
As a result, an economy is characterized by a tendency to a stagnation.

Economic Theory of the XIX Century: An example of “Outdated” View on Growth – part 2
Marx (1867, 1885, 1894) believed also that rate of economic growth decreases in the course of time.
Marx developed theory that predicts enrichment of the large/successful capitalists and immiserization of all others. In particular, workers are displaced from the production process by “labor-augmented” technical progress.
As a result, the economy is characterized a tendency to the social revolution…

Towards the contemporary theory of growth – part 1
Harrod (1939) and Domar (1946) laid the foundation of the contemporary theory of growth:
They assumed away the Law of Diminishing Fertility of the Soil” and believed that population growth does not depend on the difference between the actual wage rate and the minimal wage rate.

Towards the contemporary theory of growth – part 2
So, Harrod and Domar created the models in which the main aggregate macroeconomic variables – output (GDP), capital, labor, consumption – grow with constant rate.
But they assumed an absence of substitutability between capital and labor.
So, in these models the equilibrium growth is unstable.

Kaldor’s (1961) stylised facts
Per capita output grows over time and its growth rate does not tend to diminish;
Physical capital per worker grows over time;
The rate of return to capital is nearly constant;
The ratio of physical capital to output is nearly constant;
The shares of labour and physical capital in national income are nearly constant;
The growth rate of output per worker differs substantially across countries.

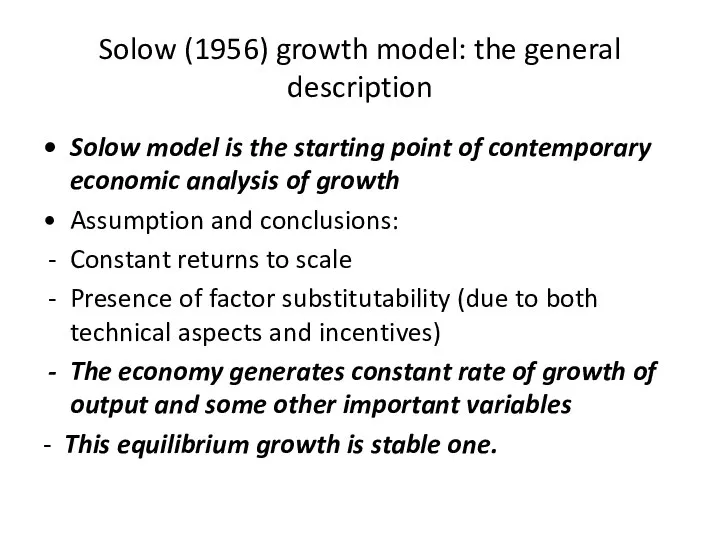
Solow (1956) growth model: the general description
Solow model is the starting point of contemporary economic analysis of growth
Assumption and conclusions:
Constant returns to scale
Presence of factor substitutability (due to both technical aspects and incentives)
The economy generates constant rate of growth of output and some other important variables
- This equilibrium growth is stable one.

A general production function in the Solow growth model
Consider a general production function
This is a “neoclassical” production function if there are positive and diminishing returns to K and L; if there are constant returns to scale (CRS); and if it obeys the Inada conditions:
with CRS, we have output per worker of
If we write K/L as k and Y/L as y, then in intensive form:

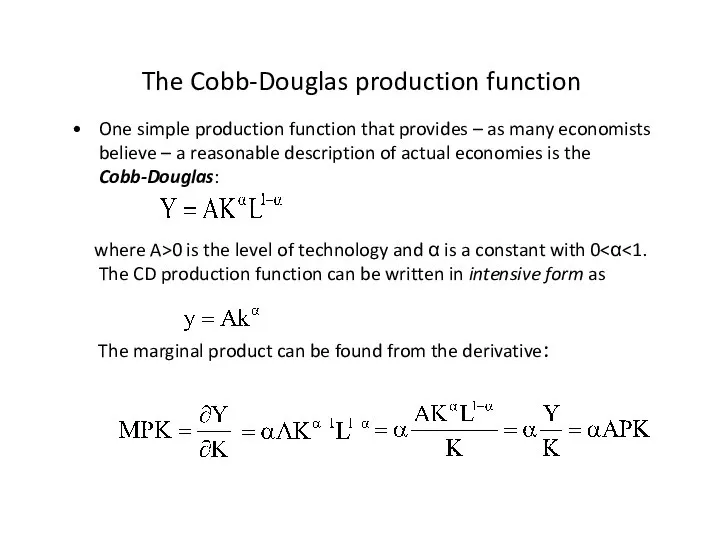
The Cobb-Douglas production function
One simple production function that provides – as many economists believe – a reasonable description of actual economies is the Cobb-Douglas:
where A>0 is the level of technology and α is a constant with 0<α<1. The CD production function can be written in intensive form as
The marginal product can be found from the derivative:

Results for distribution of income
If firms pay workers a wage of w, and pay r to rent a unit of capital for one period, profit-maximizing firms should maximise:
Under perfect competition firms are price-takers so they employ workers and rent capital until w and r are equal to the marginal products of labour and capital
Notice that wL+rK=Y, that is, payments to inputs completely exhaust output so economic profits are zero.

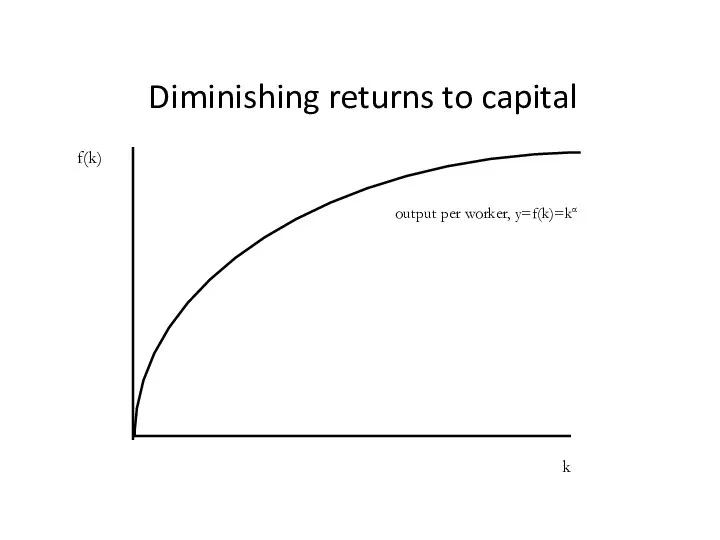
Diminishing returns to capital
output per worker, y=f(k)=kα
f(k)
k

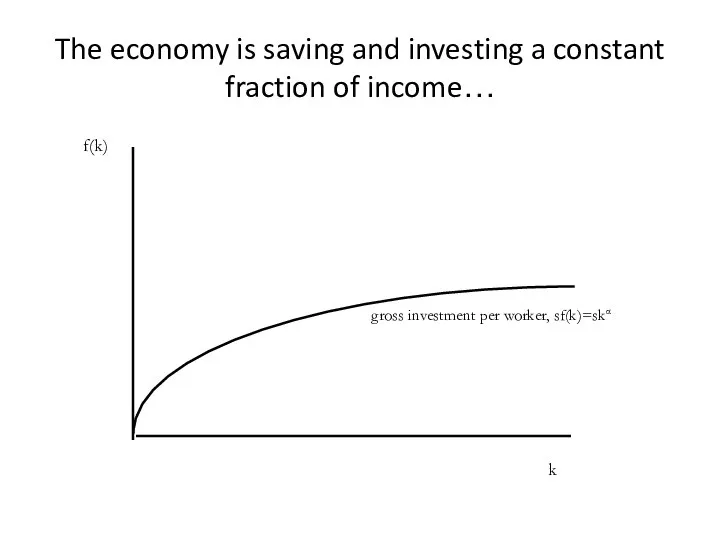
The economy is saving and investing a constant fraction of income…
gross investment per worker, sf(k)=skα
f(k)
k

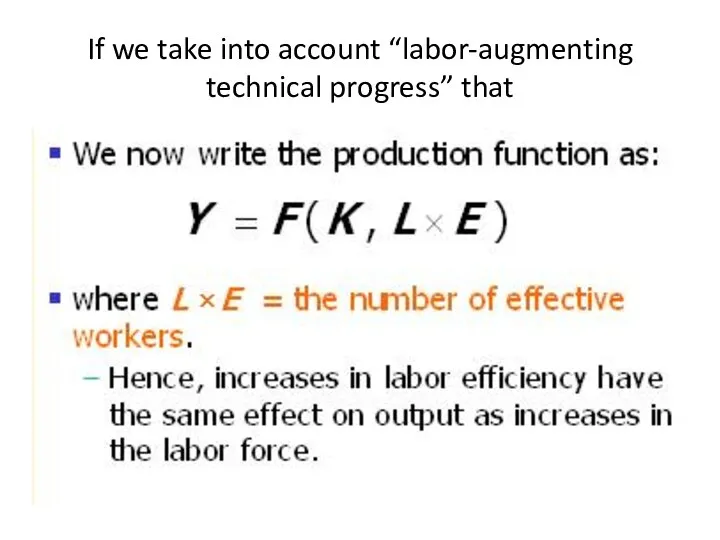
What is “labor-augmenting technical progress”?
This is technical progress that increases contribution of labor into output!

If we take into account “labor-augmenting technical progress” that

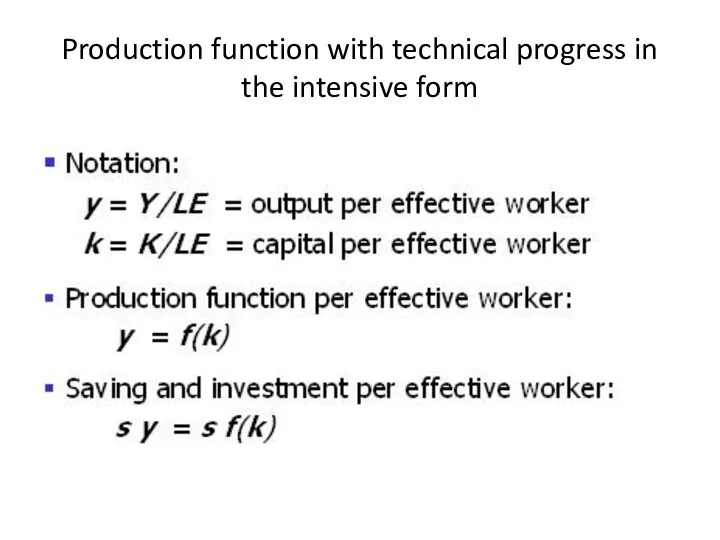
Production function with technical progress in the intensive form

What is break-even investment?

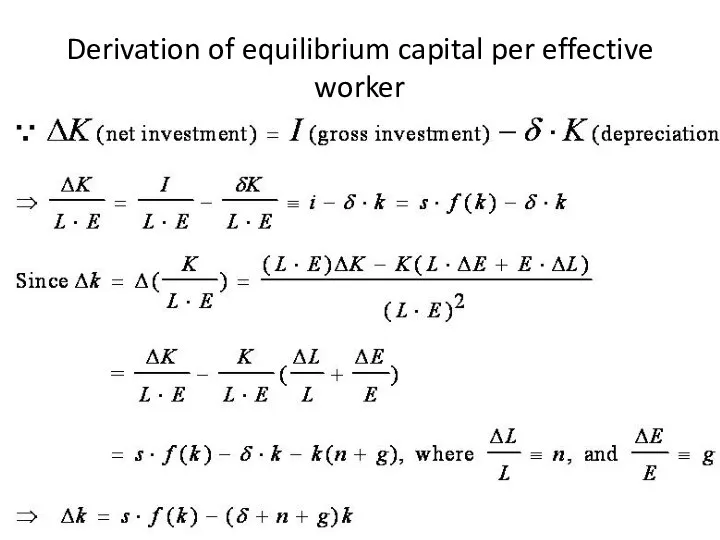
Derivation of equilibrium capital per effective worker

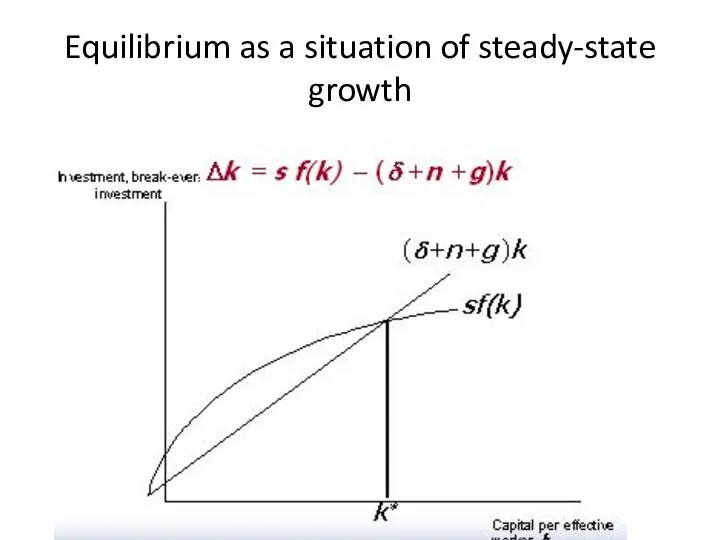
Equilibrium as a situation of steady-state growth

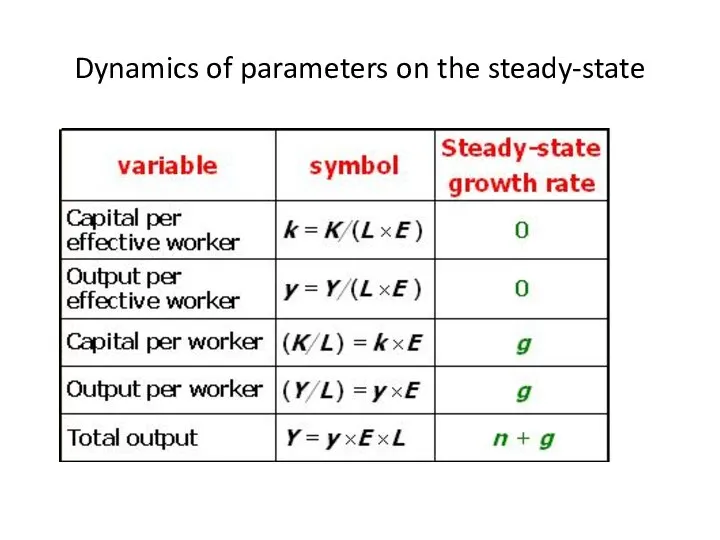
Dynamics of parameters on the steady-state

Balanced growth

Growth in steady state and outside steady state
In the steady state – when actual investment per “effective worker” = break-even investment - the rate of economic growth will be equal to the sum of rate of population growth and rate of technical progress = n+g.
If “initial” capital stock is less than steady state capital stock, then the rate of economic growth will be more than n+g.

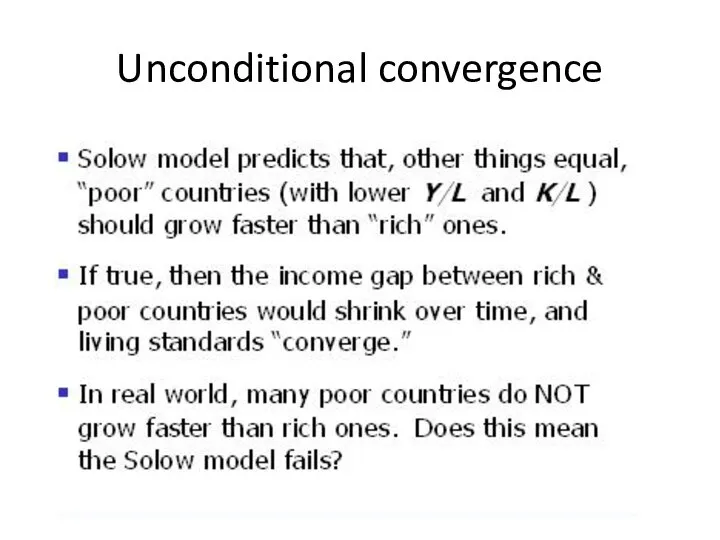
Unconditional convergence

Conditional convergence

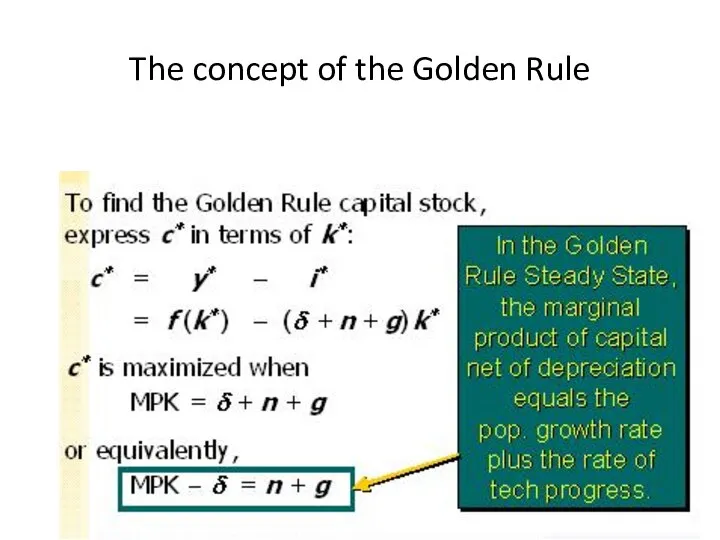
The concept of the Golden Rule

The Golden Rule – for what?

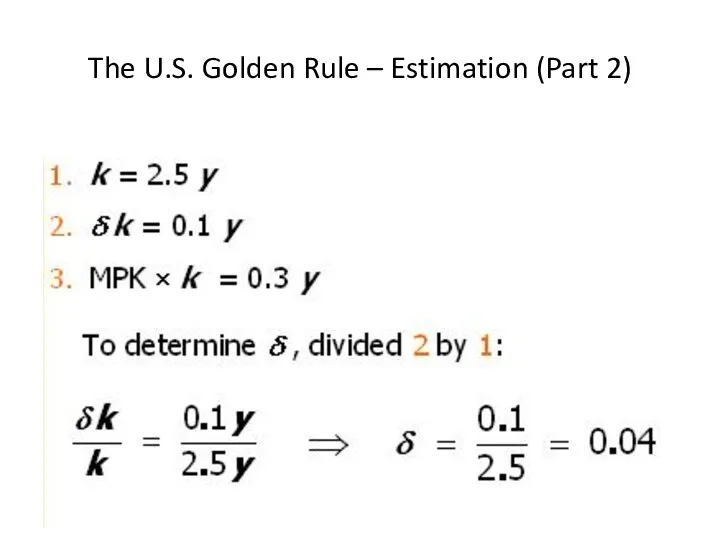
The U.S. Golden Rule – Estimation (Part 1)

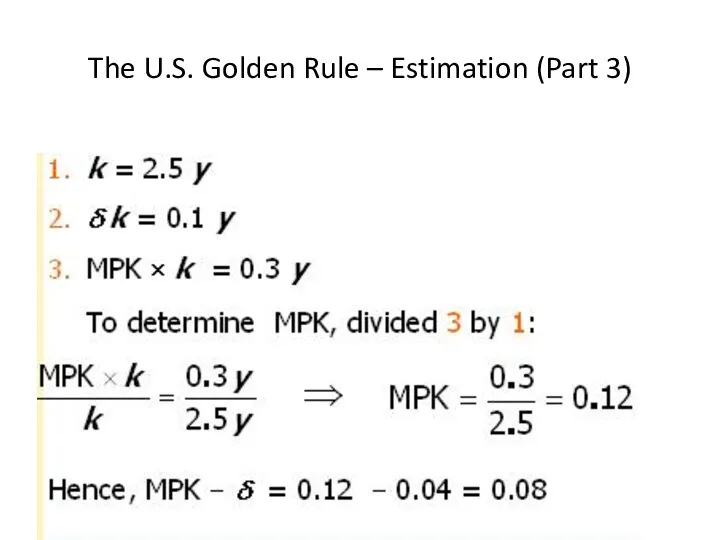
The U.S. Golden Rule – Estimation (Part 2)

The U.S. Golden Rule – Estimation (Part 3)

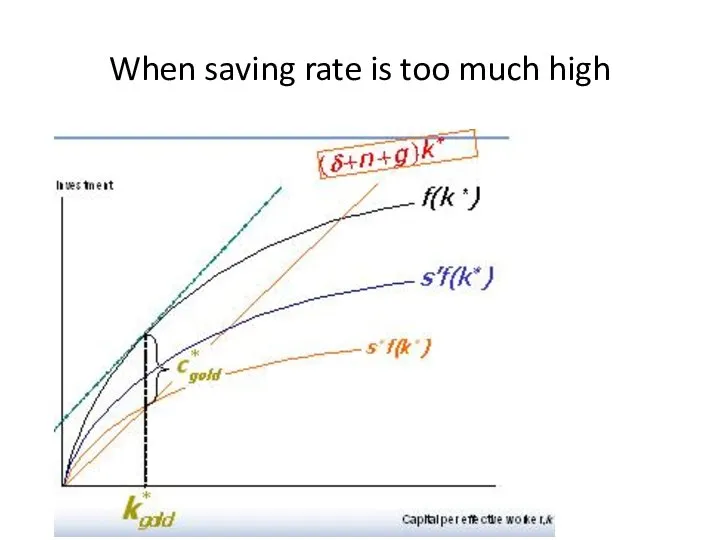
The U.S. Golden Rule – Estimation (Part 4)

When saving rate is too much high

Accounting of growth in Solow model (Part 1)

Accounting of growth in Solow model (Part 2)

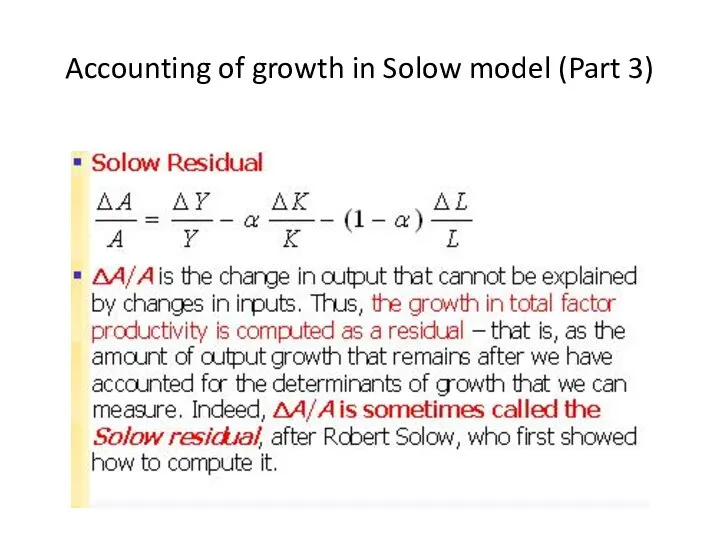
Accounting of growth in Solow model (Part 3)

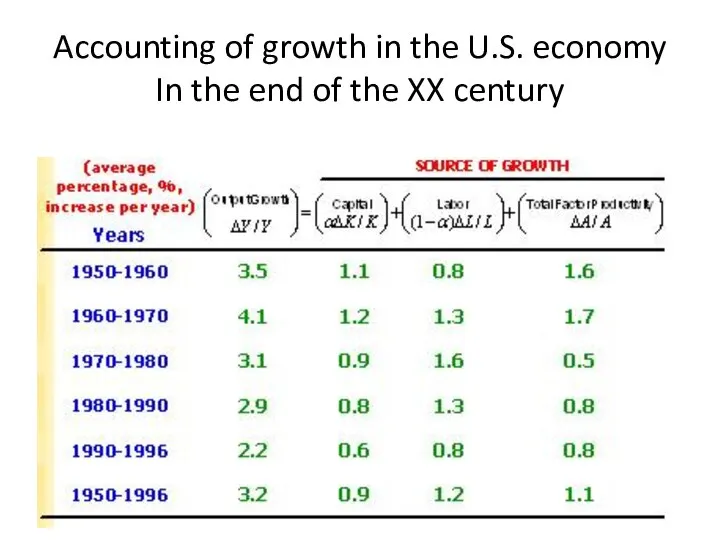
Accounting of growth in the U.S. economy In the end of the XX century

Accounting of growth among “Asian Tigers” In the end of the XX century