Слайд 2Variational Approach to the Fixed-Time, Free-Endpoint Problem

Слайд 3We now want to see how far a variational approach – i.e.

an approach based on analyzing the first (and second) variation of the cost functional – can take us in studying the optimal control problem formulated in the previous lecture.
Слайд 6
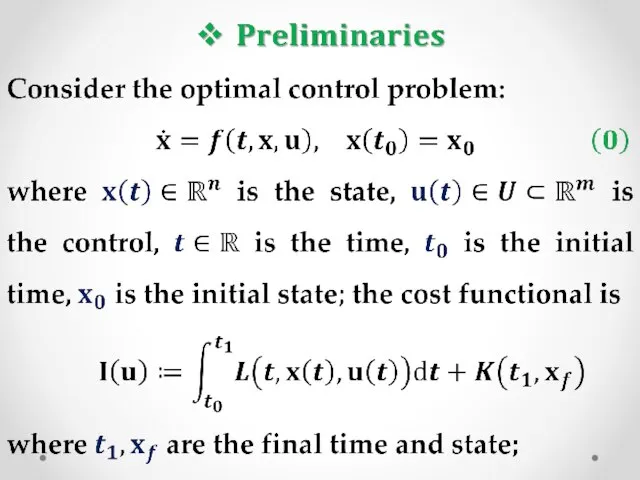
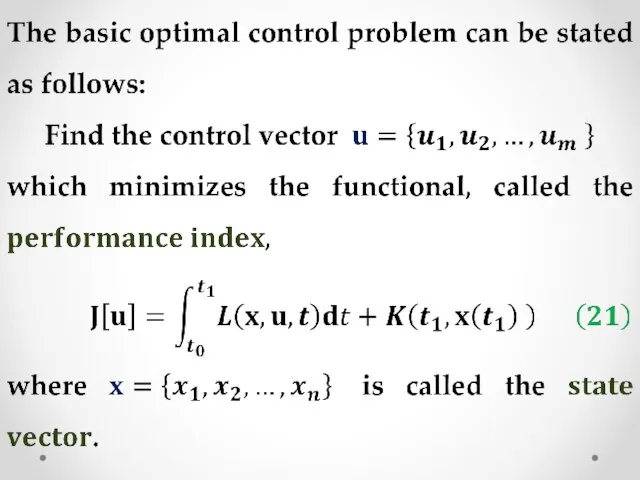
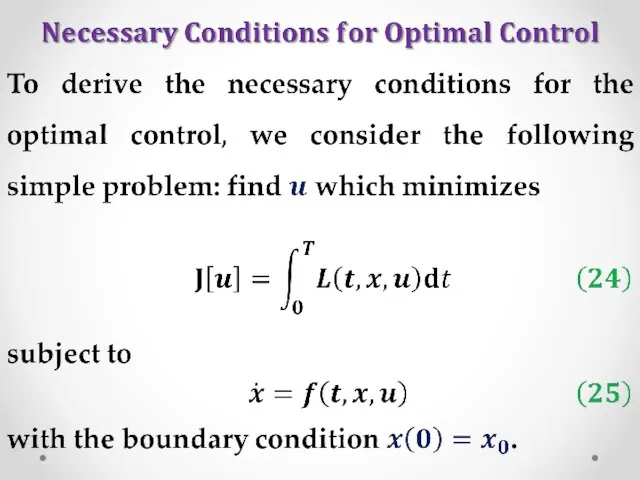
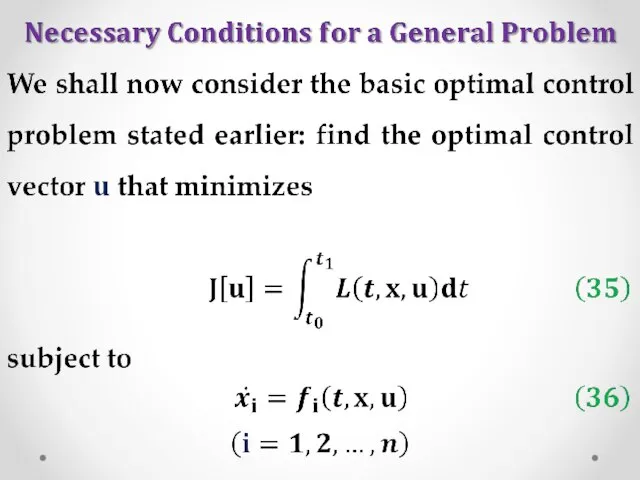
Our goal is to derive necessary conditions for optimality.

Слайд 8
Thus, in the optimal control context it is more natural to directly
perturb the control instead.
Слайд 9And then define perturbed state trajectories in terms of perturbed controls.

Слайд 16
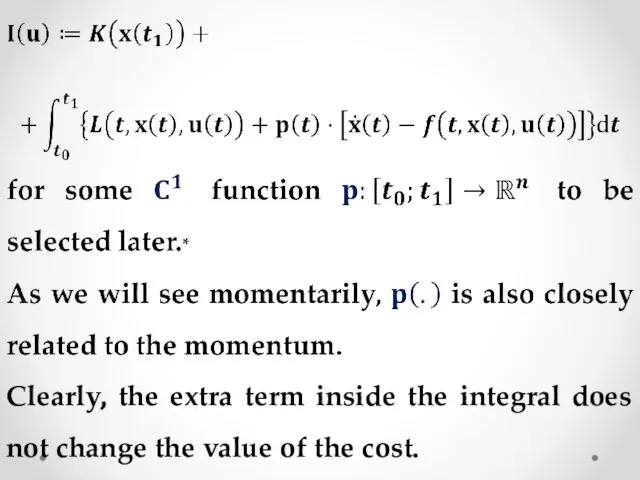
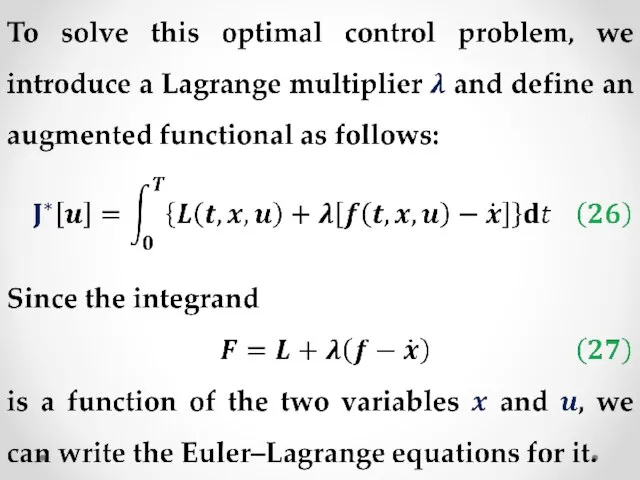
Motivated by Lagrange's idea for treating such constraints in calculus of variations,
expressed by an augmented cost, let us rewrite our cost as indicated below.
Слайд 17Clearly, the extra term inside the integral does not change the value
Слайд 31
This is just a reformulation of the property already discussed by us
in the context of calculus of variations.
Слайд 32
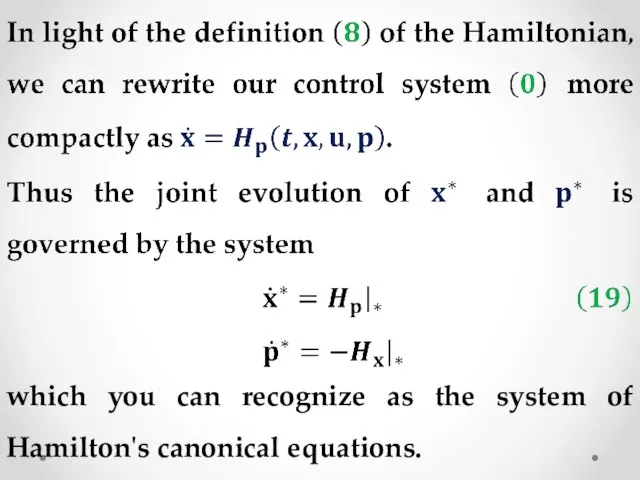
which you can recognize as the system of Hamilton's canonical equations.
Слайд 35
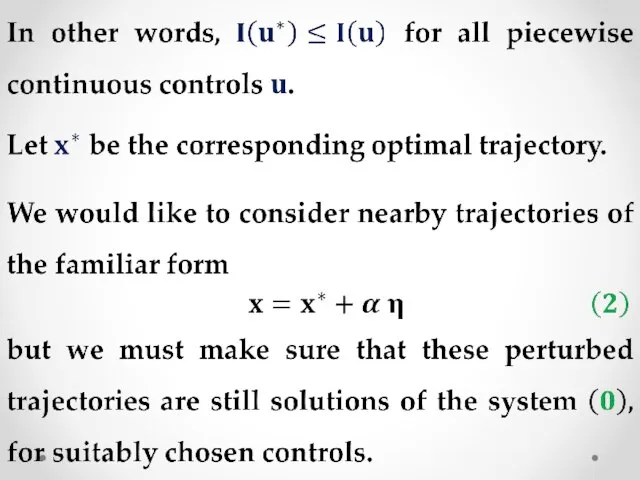
Let’s summarize the results obtained so far and see how to apply

them in practice.
Слайд 43
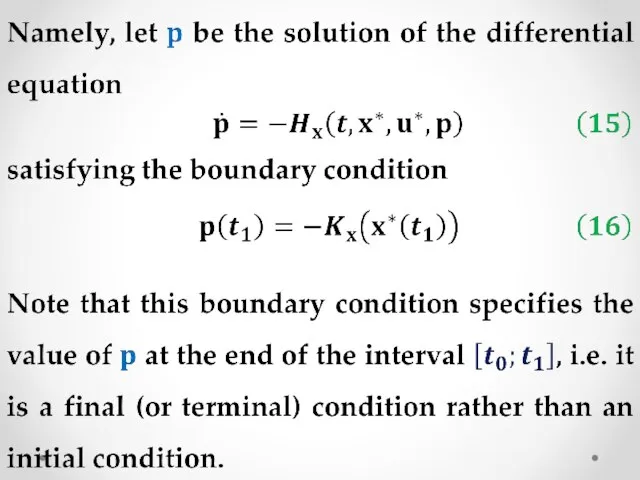
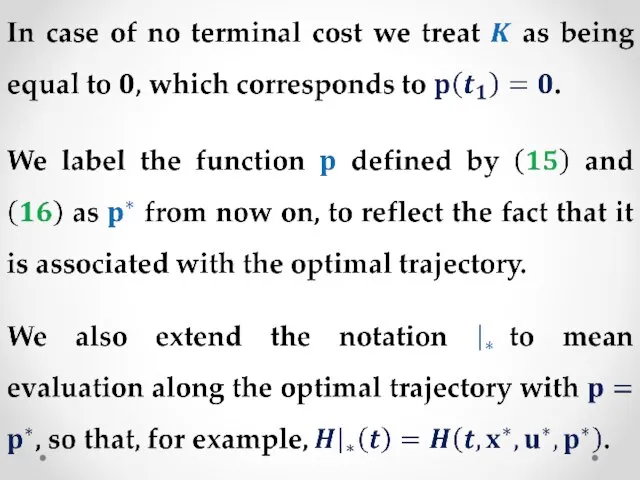
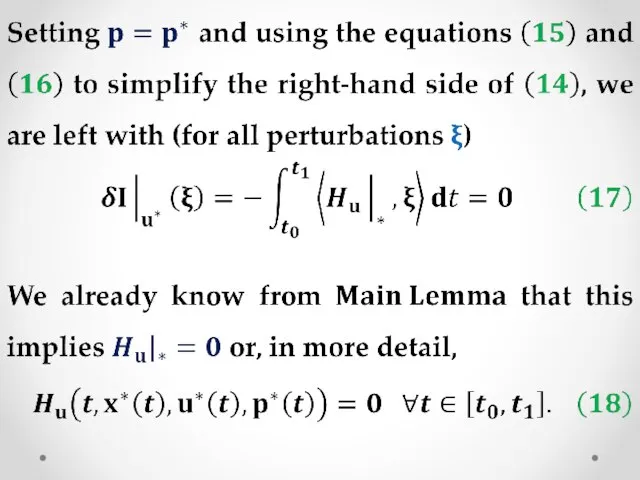
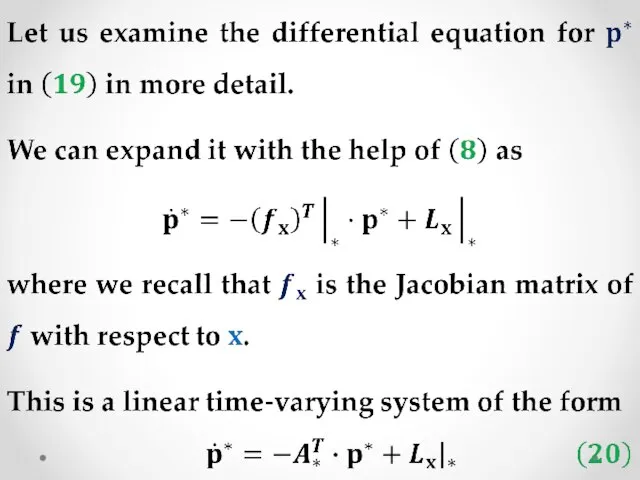
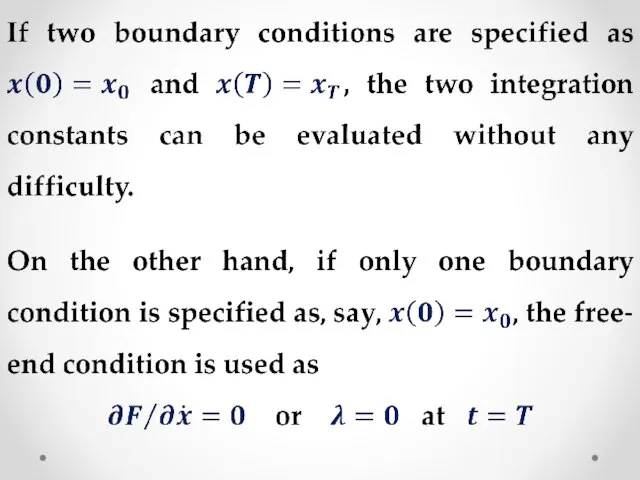
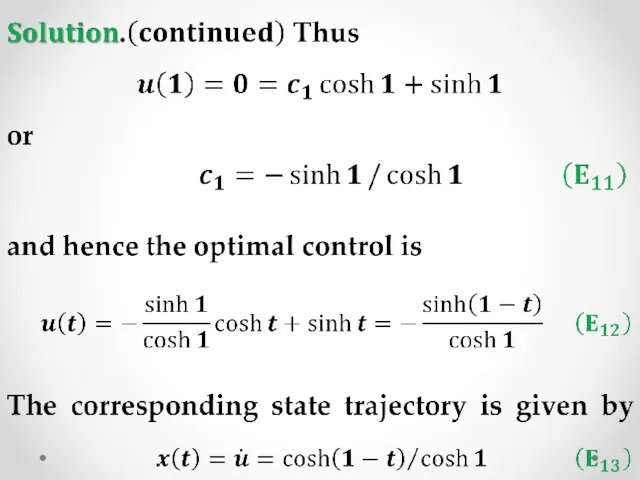
The integration of these equations leads to two constants whose values can
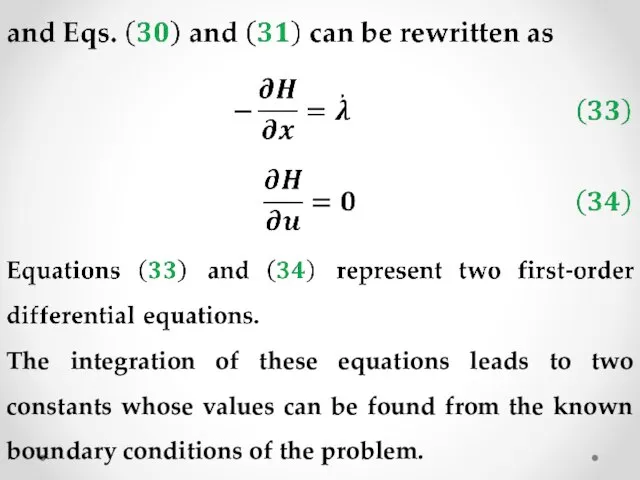
be found from the known boundary conditions of the problem.














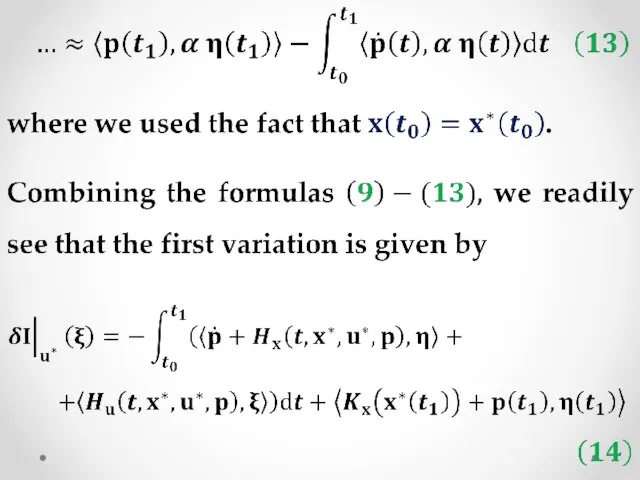























 Преобразование графиков функций. Математический диктант
Преобразование графиков функций. Математический диктант النشاط الثالث
النشاط الثالث Математический диктант по теме Сложение и вычитание. Числа от 11 до 20
Математический диктант по теме Сложение и вычитание. Числа от 11 до 20 Сложение векторов
Сложение векторов Понятие функции
Понятие функции Урок по алгебре и началам анализа. Форма проведения - деловая игра Выборы!
Урок по алгебре и началам анализа. Форма проведения - деловая игра Выборы! Проецирование на две плоскости проекций
Проецирование на две плоскости проекций Объем конуса
Объем конуса Развитие учебных навыков через применение наглядных пособий и перевода математических задач в практическую плоскость
Развитие учебных навыков через применение наглядных пособий и перевода математических задач в практическую плоскость Контрольная работа № 9
Контрольная работа № 9 формулы сокрощенного умножения
формулы сокрощенного умножения Окружность. Вписанные углы
Окружность. Вписанные углы Симметрия в пространстве
Симметрия в пространстве Отношение. Пропорции
Отношение. Пропорции Задача на тему Прогрессия
Задача на тему Прогрессия Отношения эквивалентности. Частичный порядок на множестве. Линейный порядок на множестве
Отношения эквивалентности. Частичный порядок на множестве. Линейный порядок на множестве Влияние математических действий на аликвоты
Влияние математических действий на аликвоты Движение: скорость, время, расстояние
Движение: скорость, время, расстояние Introduction to probability
Introduction to probability Презентация на тему НАХОЖДЕНИЕ НАИБОЛЬШЕГО И НАИМЕНЬШЕГО ЗНАЧЕНИЯ ФУНКЦИИ
Презентация на тему НАХОЖДЕНИЕ НАИБОЛЬШЕГО И НАИМЕНЬШЕГО ЗНАЧЕНИЯ ФУНКЦИИ  Уравнения и неравенства с двумя переменными
Уравнения и неравенства с двумя переменными Решение задач
Решение задач Прикладная математика. Системы уравнений
Прикладная математика. Системы уравнений Знакомая незнакомка. Парабола
Знакомая незнакомка. Парабола Ромб. Квадрат
Ромб. Квадрат Построение сечений многогранников
Построение сечений многогранников Интегральное исчисление
Интегральное исчисление Схема Горнера. Уильям Джордж Горнер
Схема Горнера. Уильям Джордж Горнер