Содержание
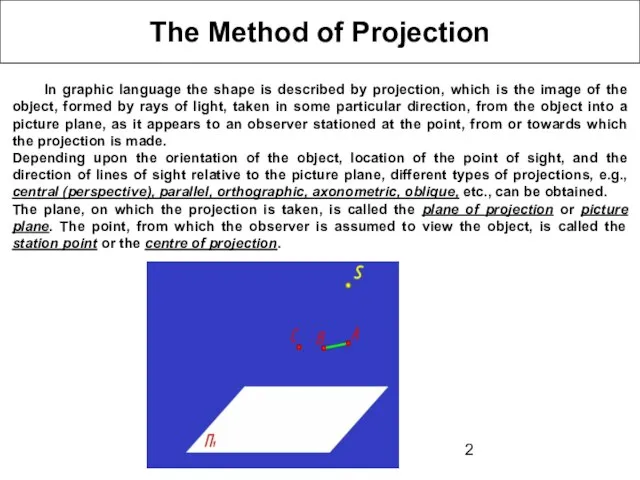
- 2. The Method of Projection In graphic language the shape is described by projection, which is the
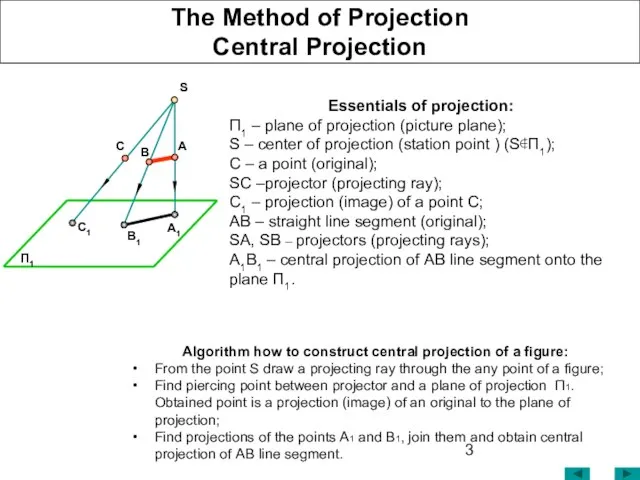
- 3. The Method of Projection Central Projection Essentials of projection: П1 – plane of projection (picture plane);
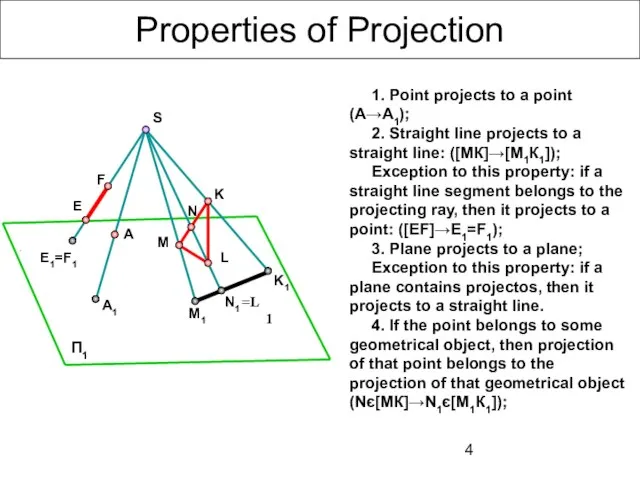
- 4. Properties of Projection 1. Point projects to a point (А→А1); 2. Straight line projects to a
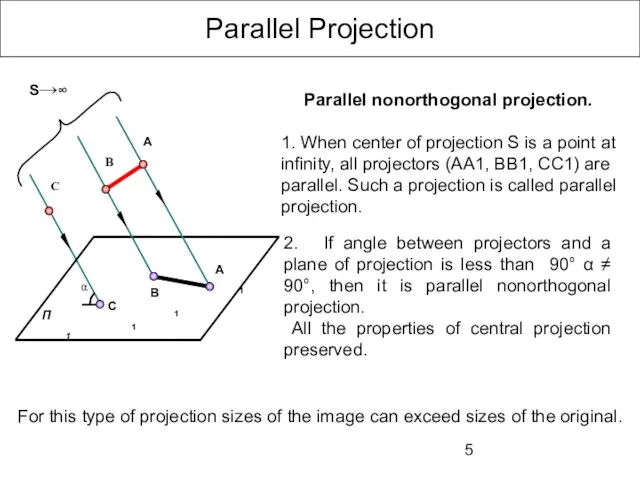
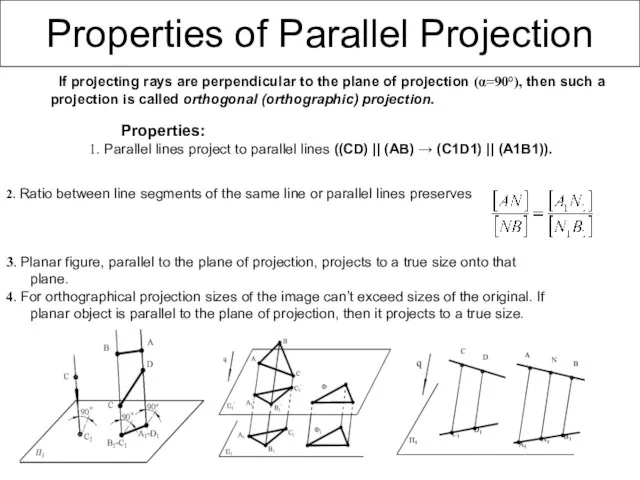
- 5. Parallel Projection 2. If angle between projectors and a plane of projection is less than 90°
- 6. Parallel Projection
- 7. 2. Ratio between line segments of the same line or parallel lines preserves 3. Planar figure,
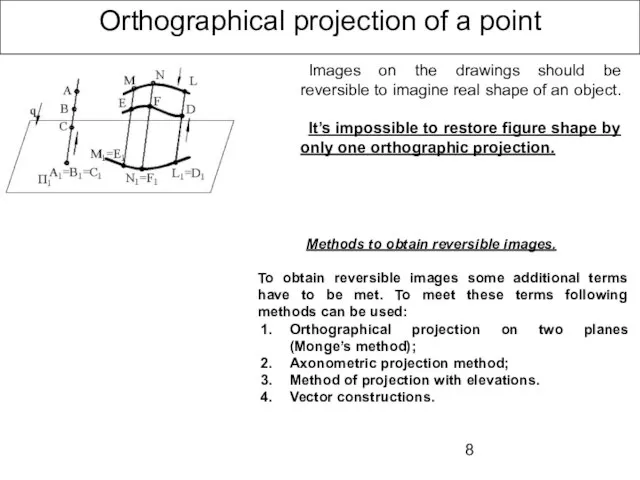
- 8. Orthographical projection of a point Images on the drawings should be reversible to imagine real shape
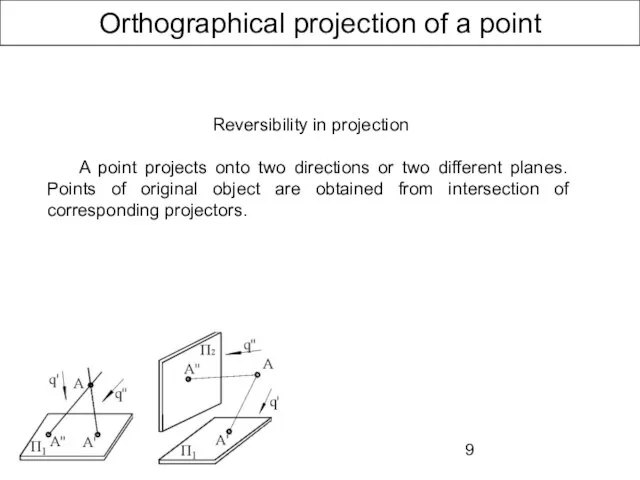
- 9. Orthographical projection of a point Reversibility in projection A point projects onto two directions or two
- 10. Monge’s method A point projects onto two mutually perpendicular principal planes (planes of projection). After that
- 11. Monge’s method Designations П1 – horizontal principal plane (plane of projection) - H; П2 – frontal
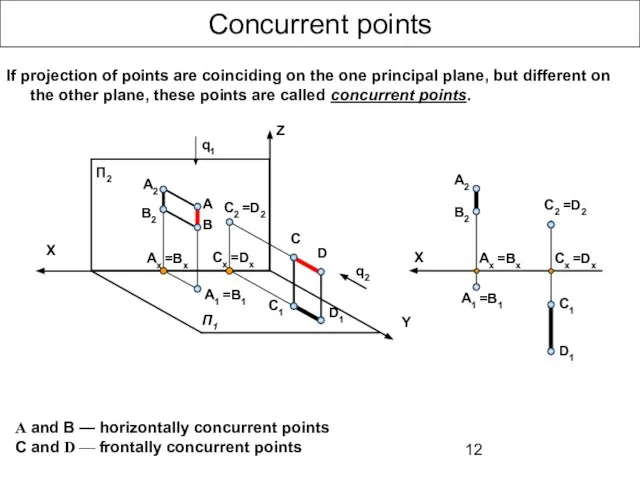
- 12. Concurrent points А and В — horizontally concurrent points C and D — frontally concurrent points
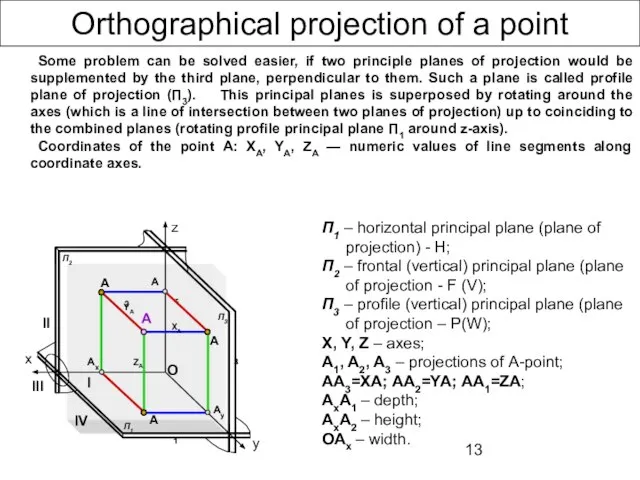
- 13. Orthographical projection of a point Some problem can be solved easier, if two principle planes of
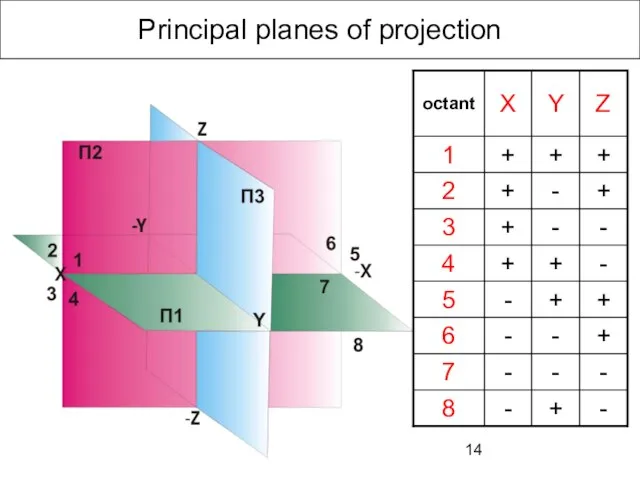
- 14. Principal planes of projection
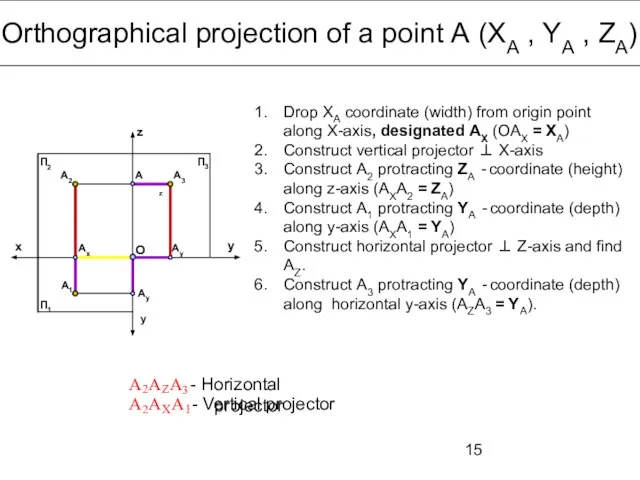
- 15. Orthographical projection of a point А (ХА , YA , ZA) x y z y П1
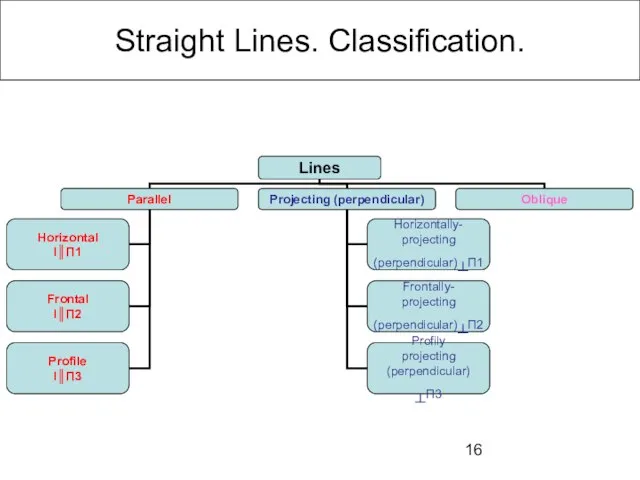
- 16. Straight Lines. Classification.
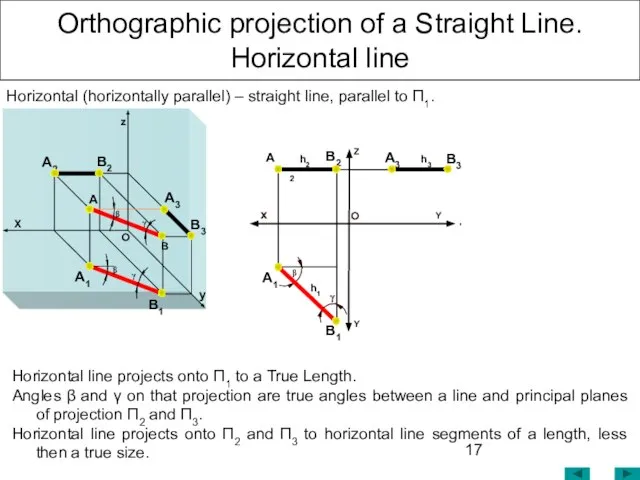
- 17. Orthographic projection of a Straight Line. Horizontal line Horizontal (horizontally parallel) – straight line, parallel to
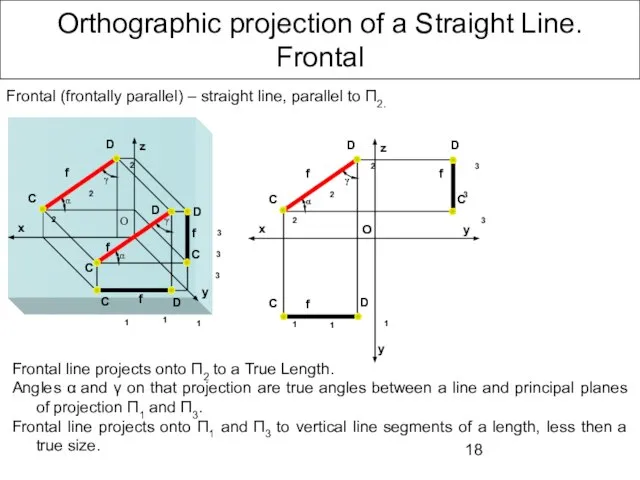
- 18. Orthographic projection of a Straight Line. Frontal Frontal line projects onto П2 to a True Length.
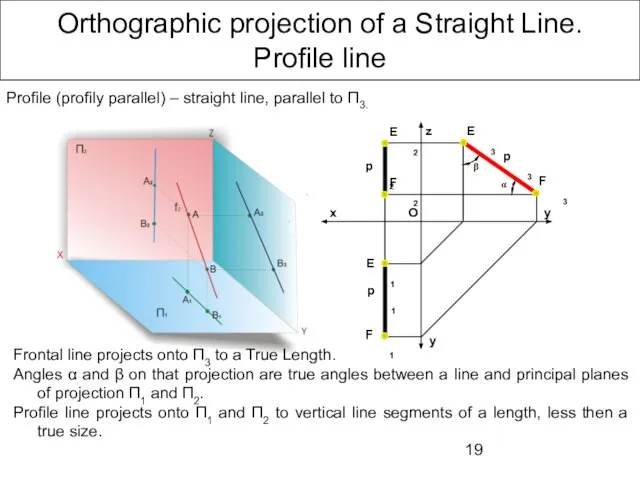
- 19. Orthographic projection of a Straight Line. Profile line Profile (profily parallel) – straight line, parallel to
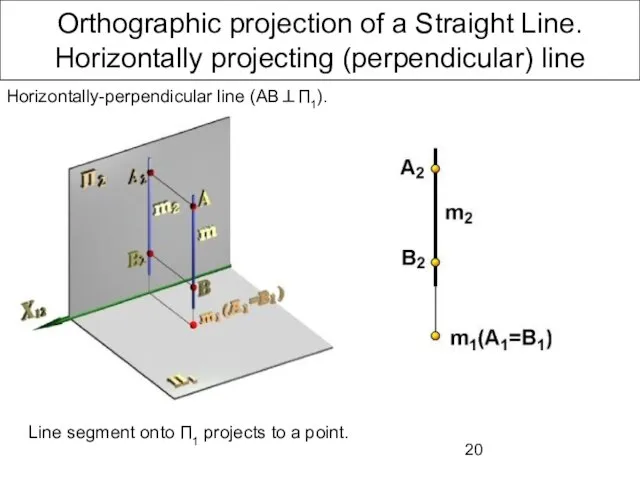
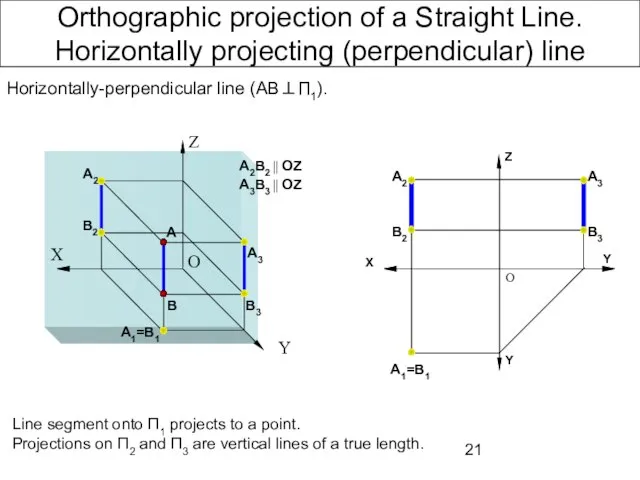
- 20. Orthographic projection of a Straight Line. Horizontally projecting (perpendicular) line Line segment onto П1 projects to
- 21. Orthographic projection of a Straight Line. Horizontally projecting (perpendicular) line Line segment onto П1 projects to
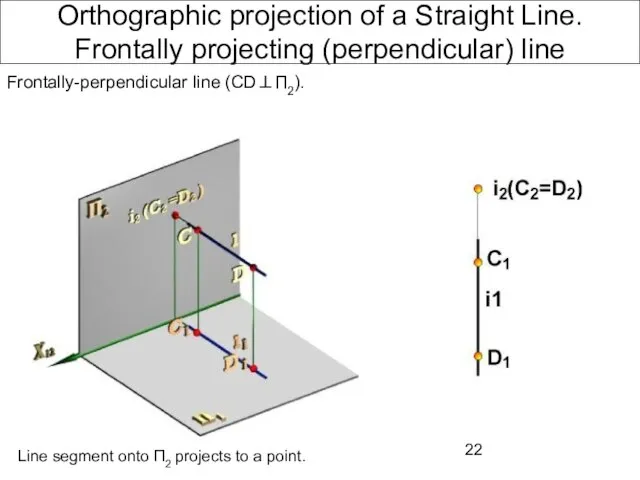
- 22. Orthographic projection of a Straight Line. Frontally projecting (perpendicular) line Frontally-perpendicular line (CD⊥П2). Line segment onto
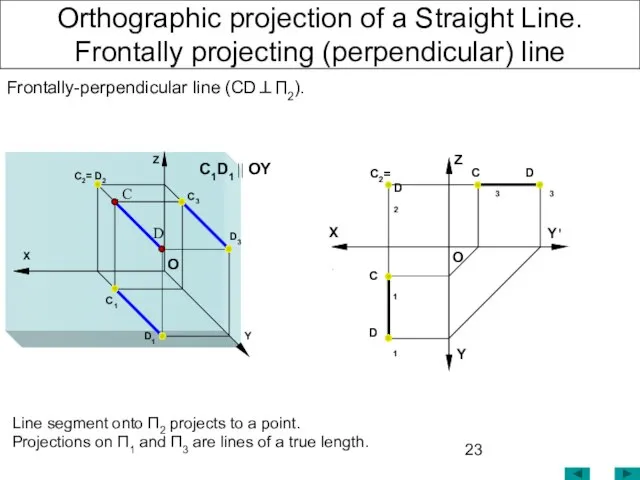
- 23. Orthographic projection of a Straight Line. Frontally projecting (perpendicular) line Frontally-perpendicular line (CD⊥П2). Line segment onto
- 24. Orthographic projection of a Straight Line. Profily projecting (perpendicular) line Profily-perpendicular line (MN⊥П3). Line segment onto
- 25. Orthographic projection of a Straight Line. Profily projecting (perpendicular) line Profily-perpendicular line (MN⊥П3). Line segment onto
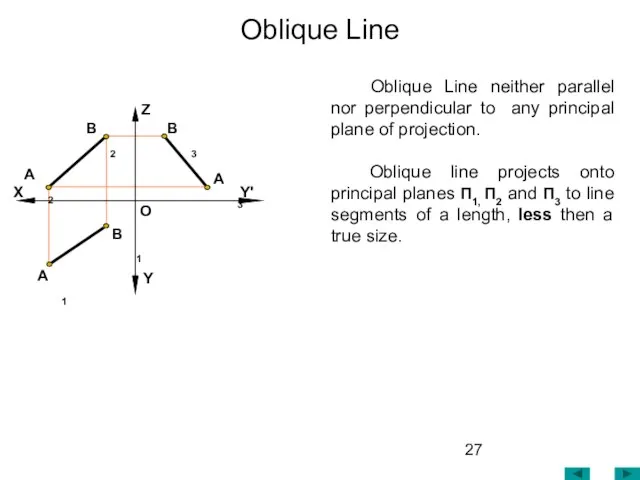
- 26. Oblique Line
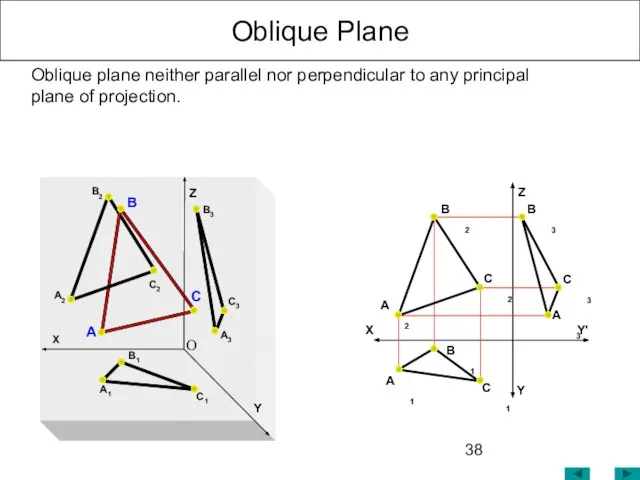
- 27. Oblique Line neither parallel nor perpendicular to any principal plane of projection. Oblique line projects onto
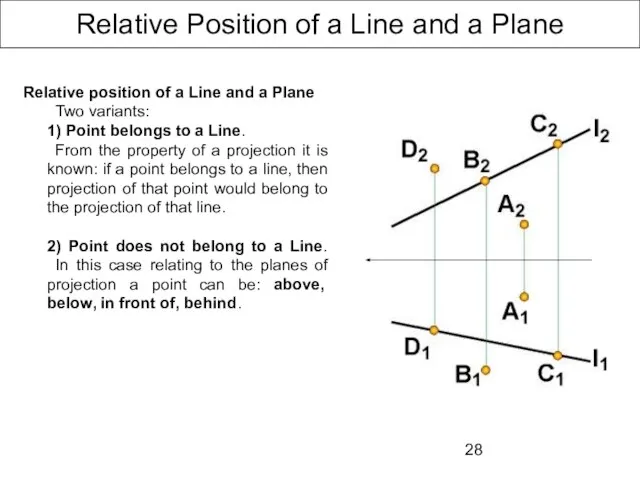
- 28. Relative Position of a Line and a Plane Relative position of a Line and a Plane
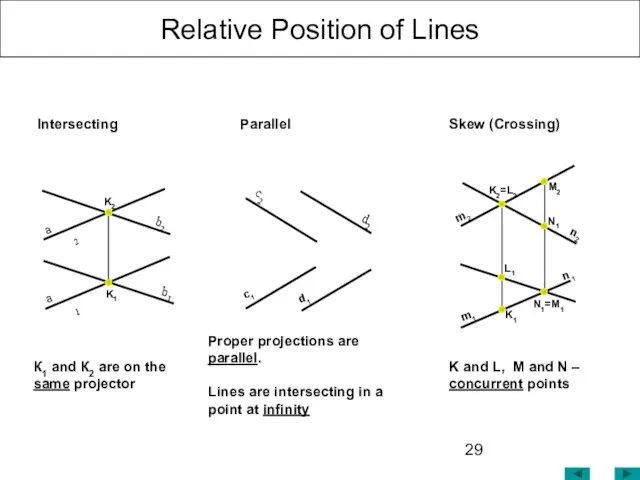
- 29. Relative Position of Lines K and L, M and N – concurrent points Proper projections are
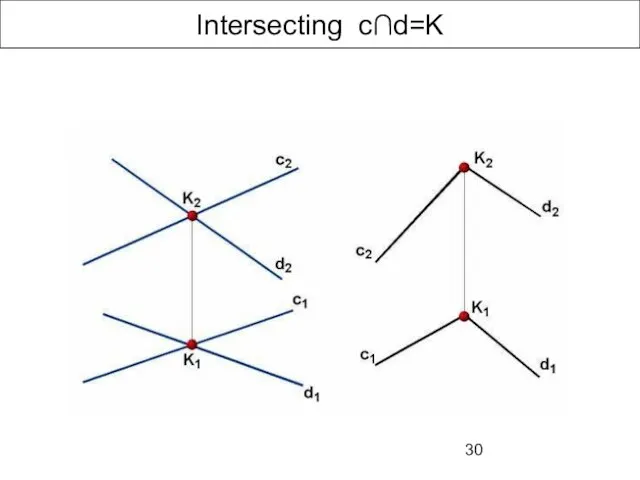
- 30. Intersecting с∩d=K
- 31. Parallel a║b
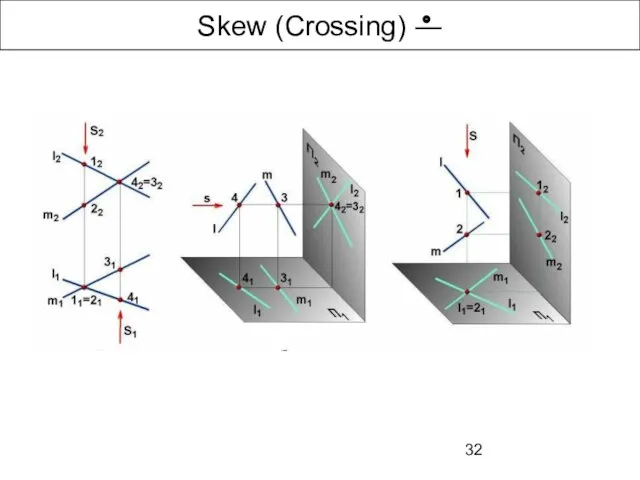
- 32. Skew (Crossing) ―
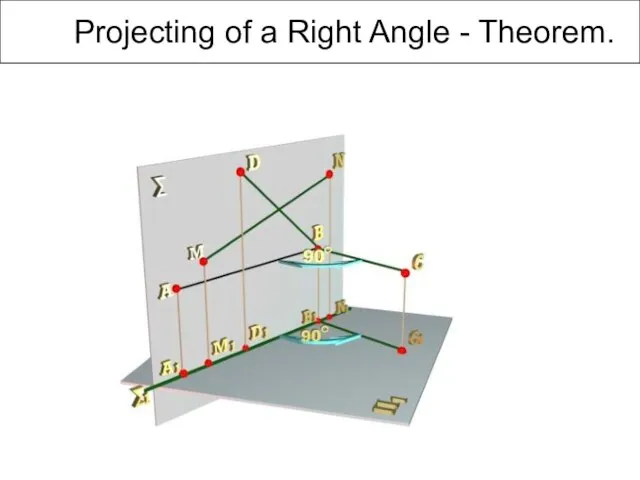
- 33. Projecting of a Right Angle - Theorem.
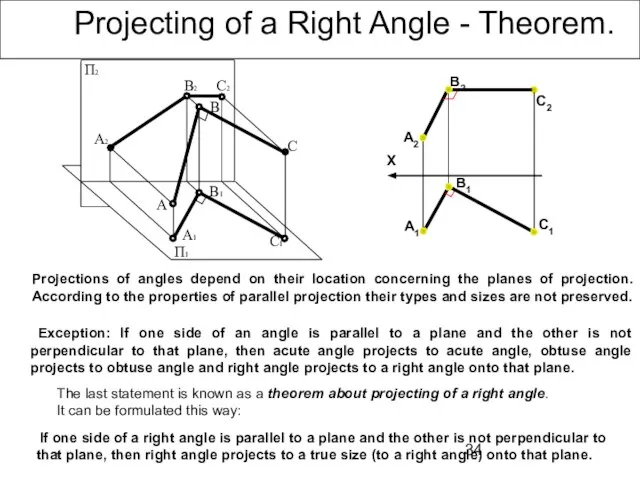
- 34. Projecting of a Right Angle - Theorem. Exception: If one side of an angle is parallel
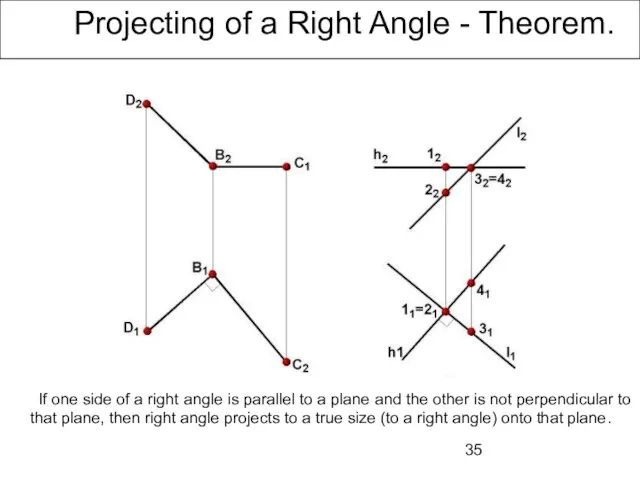
- 35. Projecting of a Right Angle - Theorem. If one side of a right angle is parallel
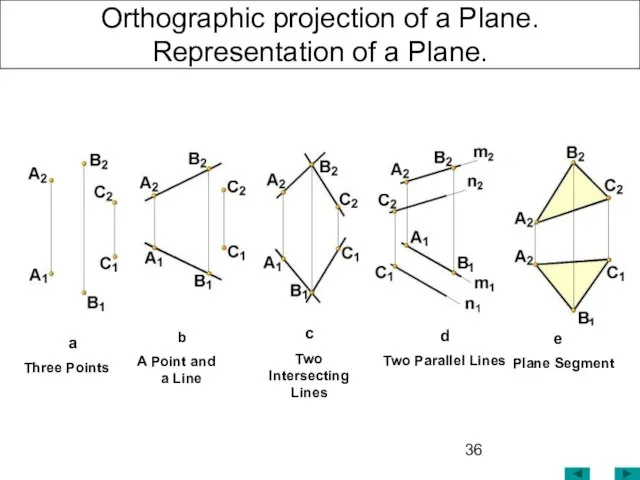
- 36. Orthographic projection of a Plane. Representation of a Plane. b A Point and a Line a
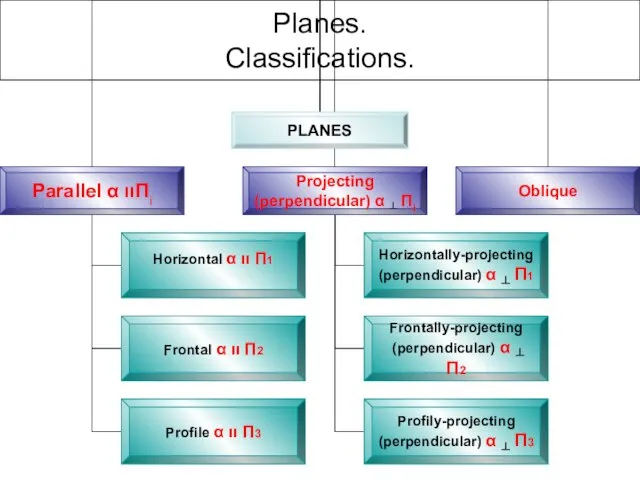
- 37. Planes. Classifications.
- 38. Oblique Plane Z Y' Y X Oblique plane neither parallel nor perpendicular to any principal plane
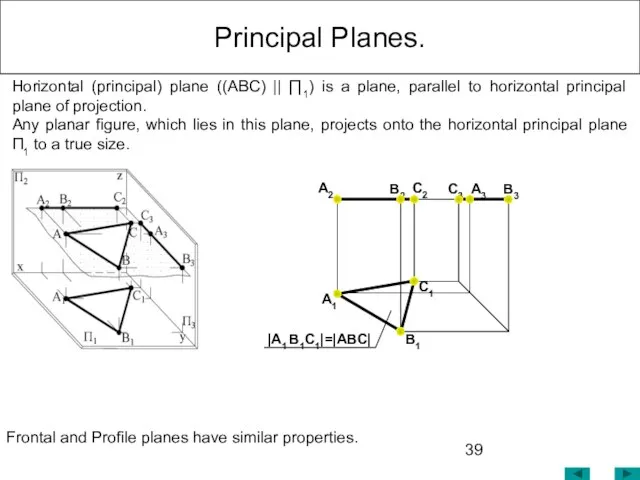
- 39. Principal Planes. Horizontal (principal) plane ((ABC) || ∏1) is a plane, parallel to horizontal principal plane
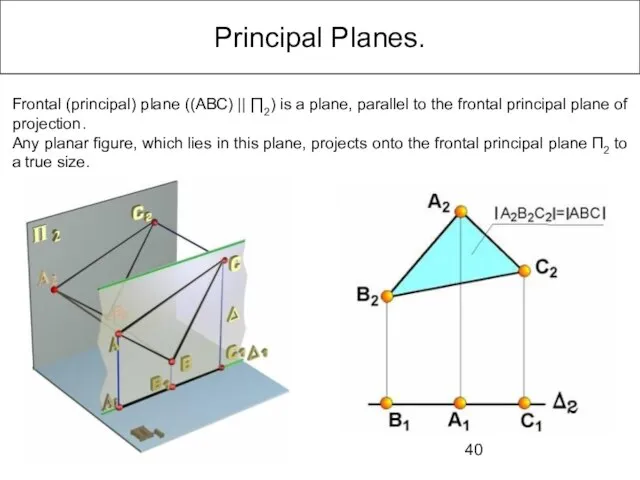
- 40. Principal Planes. Frontal (principal) plane ((ABC) || ∏2) is a plane, parallel to the frontal principal
- 41. Principal Planes. Profile (principal) plane ((ABC) || ∏3) is a plane, parallel to the profile principal
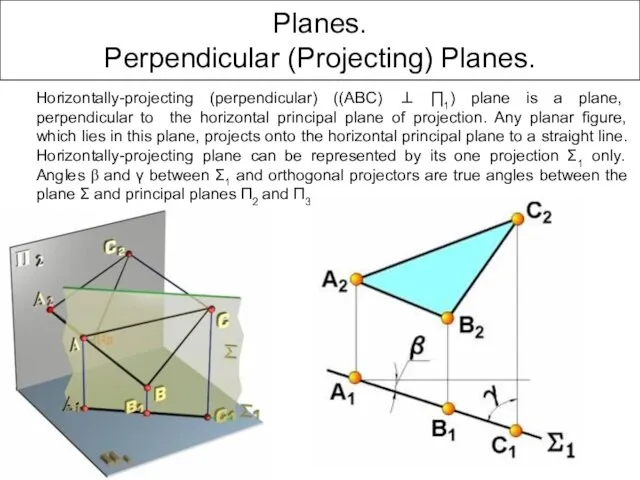
- 42. Planes. Perpendicular (Projecting) Planes. Horizontally-projecting (perpendicular) ((ABC) ⊥ ∏1) plane is a plane, perpendicular to the
- 43. Planes. Perpendicular (Projecting) Planes. Frontally-projecting (perpendicular) ((ABC) ⊥ ∏2) plane is a plane, perpendicular to the
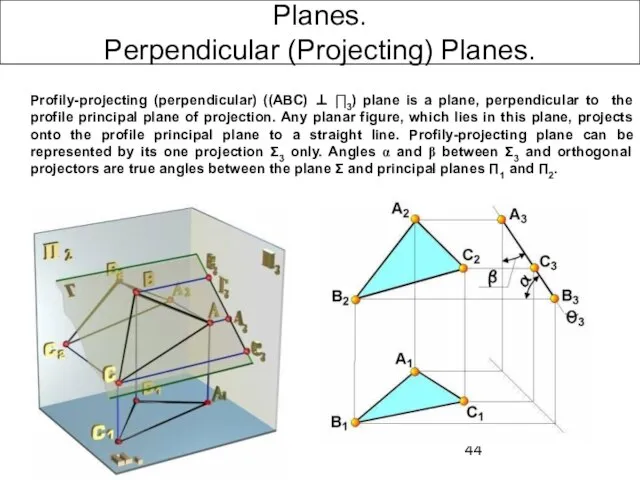
- 44. Planes. Perpendicular (Projecting) Planes. Profily-projecting (perpendicular) ((ABC) ⊥ ∏3) plane is a plane, perpendicular to the
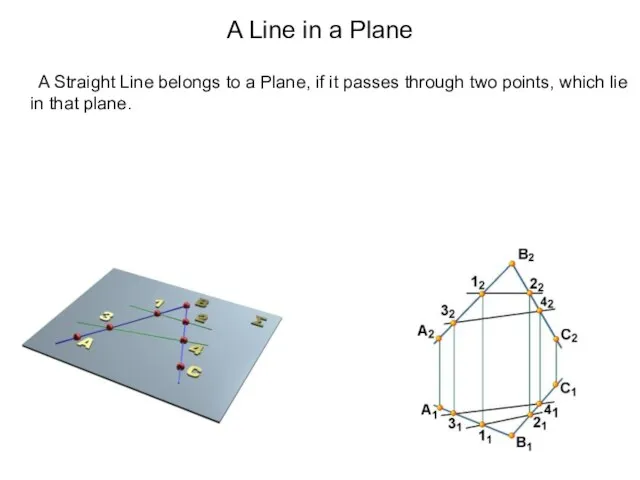
- 45. A Line in a Plane A Straight Line belongs to a Plane, if it passes through
- 46. A Point in a Plane. Algorithm: 1.Through the frontal projection of the point М2(N2) draw any
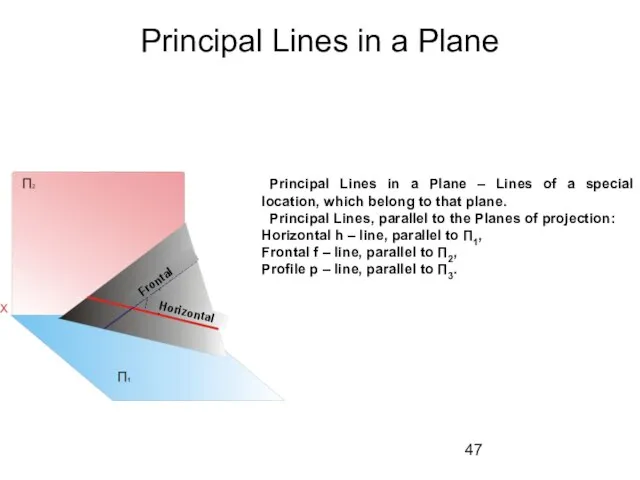
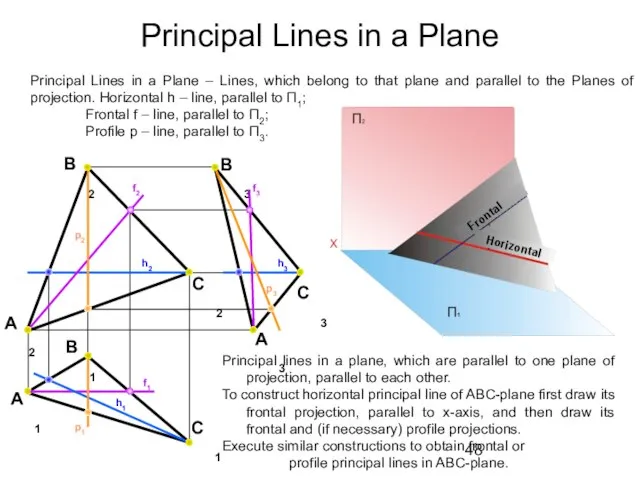
- 47. Principal Lines in a Plane Principal Lines in a Plane – Lines of a special location,
- 48. Principal Lines in a Plane B2 A2 C2 B3 A3 C1 B1 A1 Principal lines in
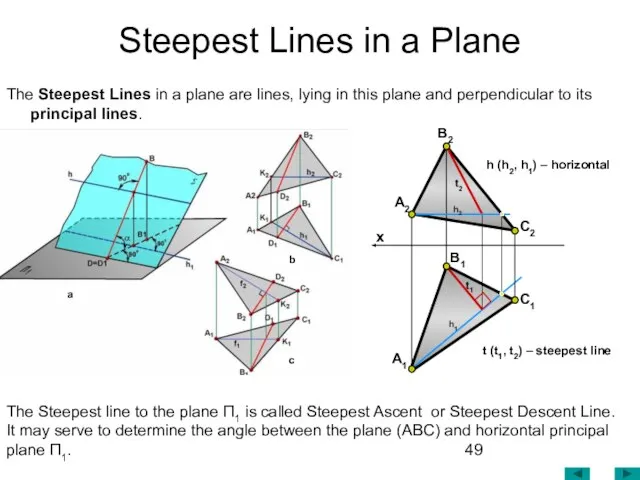
- 49. Steepest Lines in a Plane The Steepest Lines in a plane are lines, lying in this
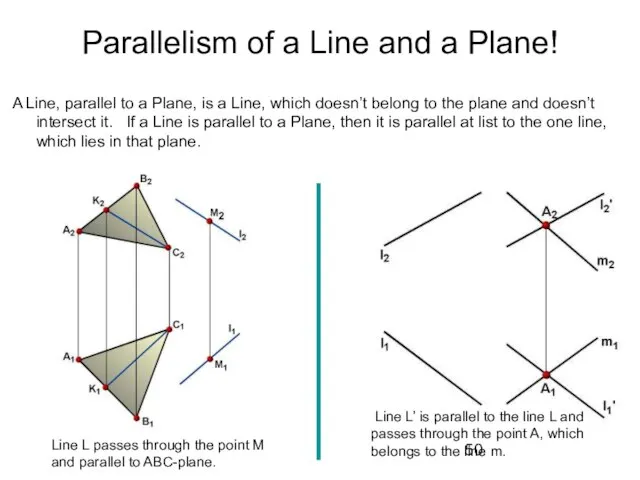
- 50. Parallelism of a Line and a Plane! A Line, parallel to a Plane, is a Line,
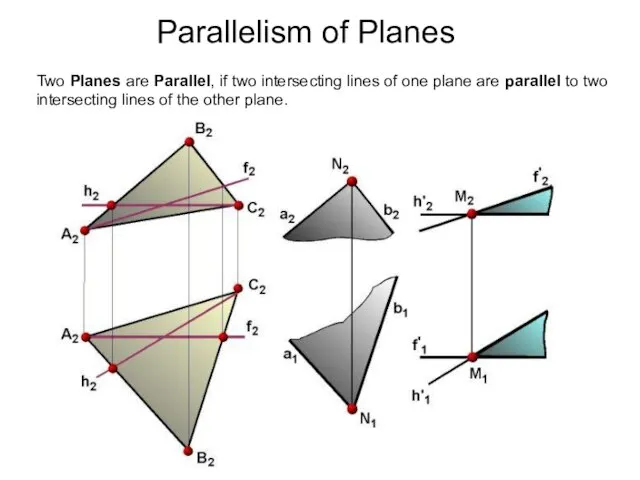
- 51. Two Planes are Parallel, if two intersecting lines of one plane are parallel to two intersecting
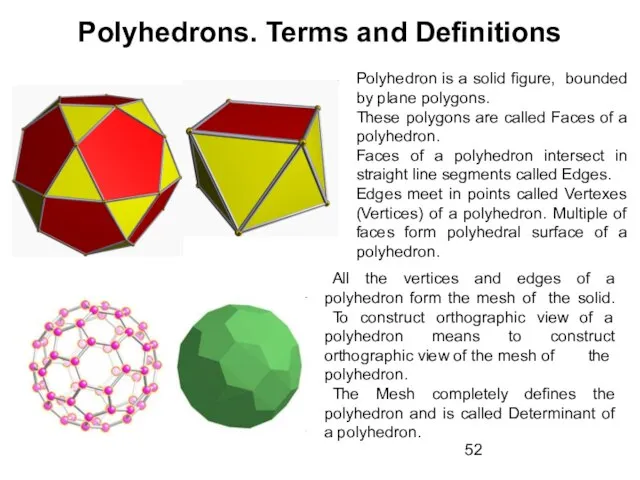
- 52. Polyhedrons. Terms and Definitions Polyhedron is a solid figure, bounded by plane polygons. These polygons are
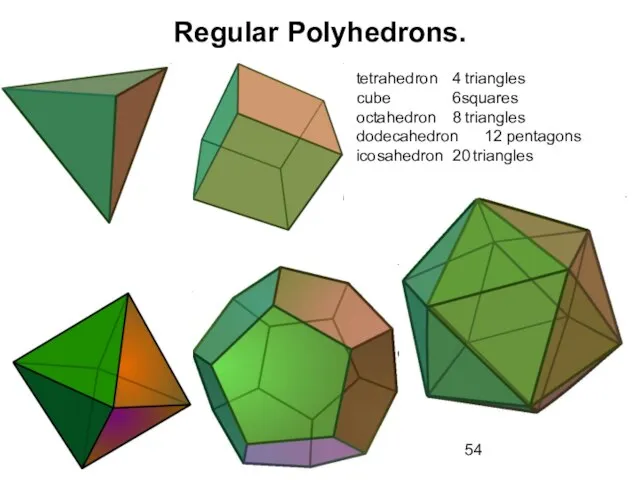
- 53. Regular Polyhedrons. A Regular polyhedron is a polyhedron whose faces are all regular polygons which are
- 54. Regular Polyhedrons. tetrahedron 4 triangles cube 6squares octahedron 8 triangles dodecahedron 12 pentagons icosahedron 20 triangles
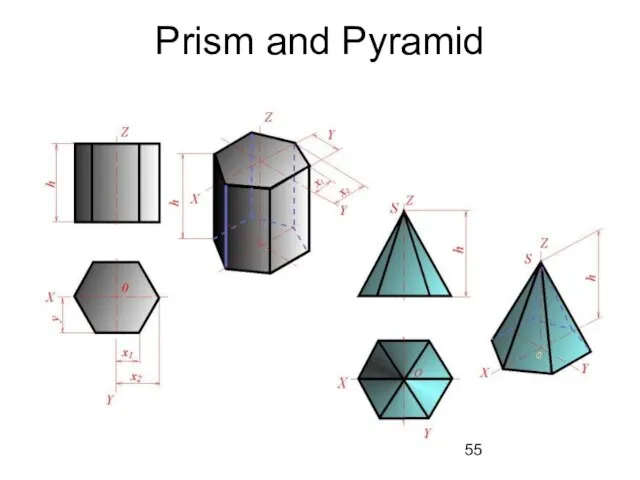
- 55. Prism and Pyramid
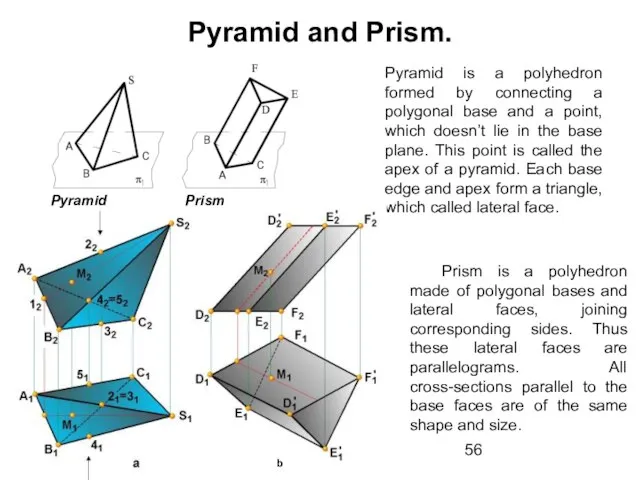
- 56. Pyramid and Prism. Pyramid is a polyhedron formed by connecting a polygonal base and a point,
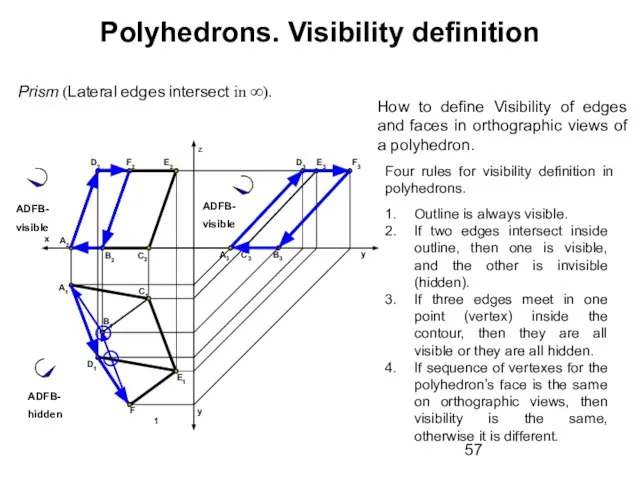
- 57. Polyhedrons. Visibility definition Prism (Lateral edges intersect in ∞). A1 D1 C2 C1 y y z
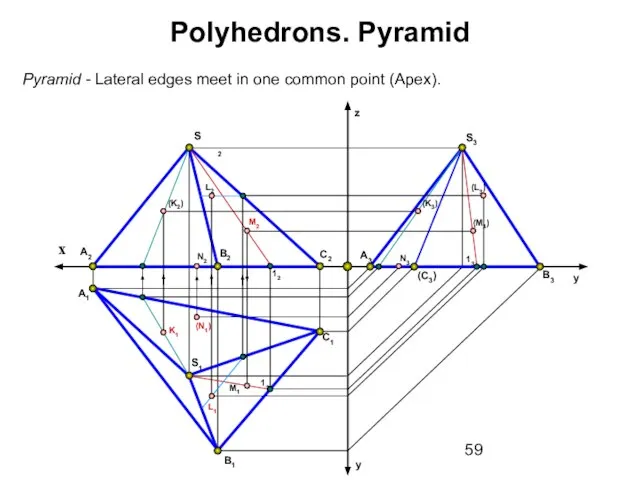
- 58. Polyhedrons. Pyramid Pyramid - Lateral edges meet in one common point (Apex). B1 y A2 A1
- 59. B1 y A2 A1 x B2 C1 C2 y z S2 S1 Pyramid - Lateral edges
- 61. Скачать презентацию












 От структуры к содержанию исследовательской работы
От структуры к содержанию исследовательской работы Право в системе социальных норм (5)
Право в системе социальных норм (5) РАЗДЕЛ №3МЕТОДЫ И СРЕДСТВА РЕШЕНИЯ ЗАДАЧ УПРАВЛЕНИЯ КОММЕРЧЕСКОЙ ДЕЯТЕЛЬНОСТЬЮ
РАЗДЕЛ №3МЕТОДЫ И СРЕДСТВА РЕШЕНИЯ ЗАДАЧ УПРАВЛЕНИЯ КОММЕРЧЕСКОЙ ДЕЯТЕЛЬНОСТЬЮ Роль дополнительных образовательных программ в современной системе качественного образования
Роль дополнительных образовательных программ в современной системе качественного образования Управление клипом
Управление клипом Как снимается кинофильм
Как снимается кинофильм Московский концептуализм
Московский концептуализм LEKTsIYa_POSOBIE_PO_NETRUDOSPOSOBNOSTI__kopia
LEKTsIYa_POSOBIE_PO_NETRUDOSPOSOBNOSTI__kopia Поп-Арт
Поп-Арт www.yaware.com.ua
www.yaware.com.ua «Современные формы и содержание работы образовательного учреждения по повышению педагогической культуры родителей, совместная
«Современные формы и содержание работы образовательного учреждения по повышению педагогической культуры родителей, совместная  У настоящего мужчины всегда есть что сказать!!!
У настоящего мужчины всегда есть что сказать!!! Північна Америка
Північна Америка Подготовил: Анищенко Д.В. ДС -04
Подготовил: Анищенко Д.В. ДС -04 Презентация на тему Первые киевские князья (6 класс)
Презентация на тему Первые киевские князья (6 класс) 16-цветок
16-цветок Выделяя основные элементы общества, их взаимосвязь и взаимодействие, учёные характеризуют общество как
Выделяя основные элементы общества, их взаимосвязь и взаимодействие, учёные характеризуют общество как Основные концептуальные идеи технологии личностно-ориентированного воспитания
Основные концептуальные идеи технологии личностно-ориентированного воспитания Сотрудник как адвокат бренда работодателя. Вовлеченность сотрудника
Сотрудник как адвокат бренда работодателя. Вовлеченность сотрудника Изготовление кормушки для птиц
Изготовление кормушки для птиц Особенности изделий HSME
Особенности изделий HSME Hierarchy of genres
Hierarchy of genres 8-хімія Орбіталі
8-хімія Орбіталі Прикамский социальный институт
Прикамский социальный институт Лоис Лоури Дающий
Лоис Лоури Дающий Карьера ИТ-менеджера точка зрения рекрутера Ерина Марина
Карьера ИТ-менеджера точка зрения рекрутера Ерина Марина Автоматизация звука р
Автоматизация звука р Выбор методологических подходов педагогического исследования
Выбор методологических подходов педагогического исследования