Integration as a Process of Summation (using integration

Consider the area under a curve
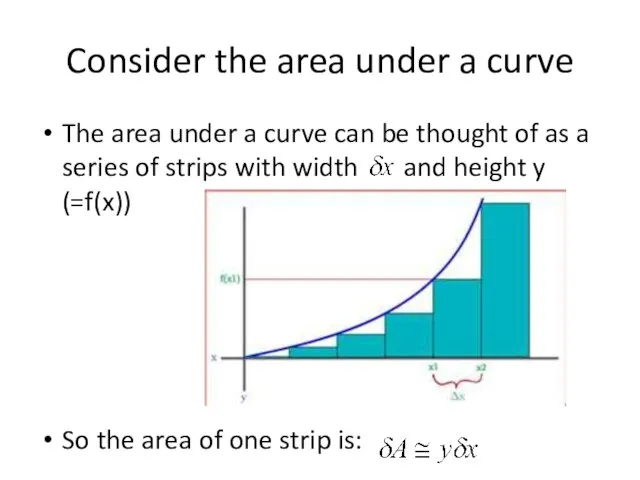
The area under a curve can be thought of as a series of strips with width and height y (=f(x))
So the area of one strip is:

Consider the area under a curve
Now consider the graph:
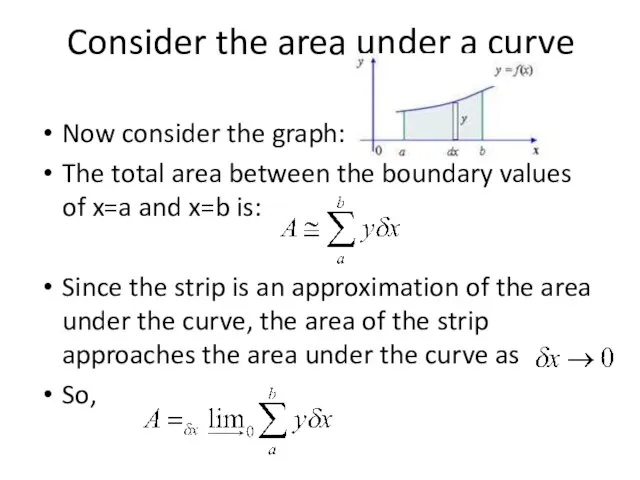
The total area between the boundary values of x=a and x=b is:
Since the strip is an approximation of the area under the curve, the area of the strip approaches the area under the curve as
So,

Consider the area under a curve
Now consider an alternative expression for A:
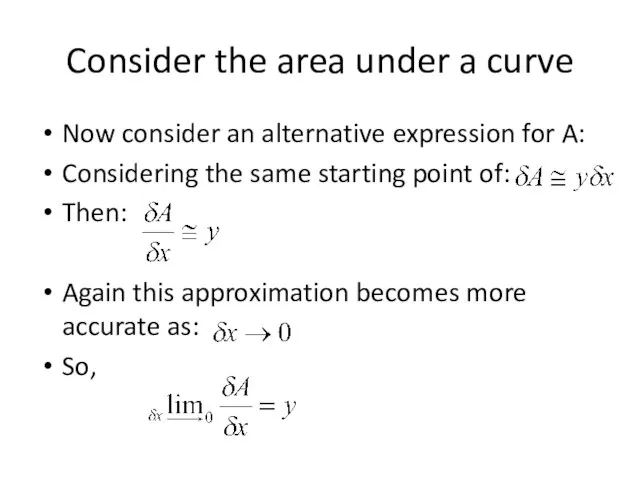
Considering the same starting point of:
Then:
Again this approximation becomes more accurate as:
So,

Consider the area under a curve
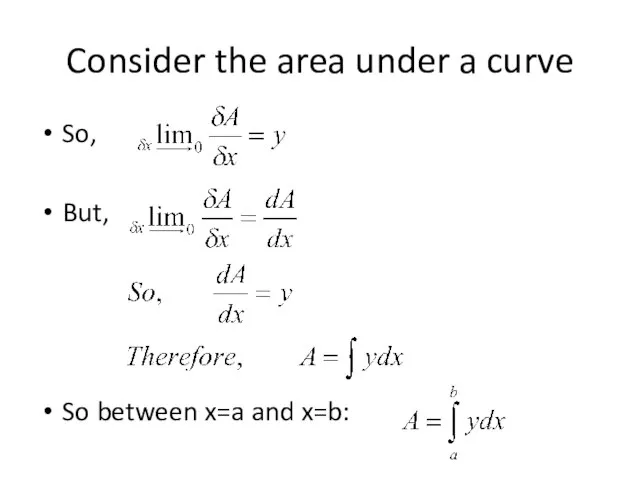
So,
But,
So between x=a and x=b: