Optimization models

Demand function
What shall we do with our selling Price, if:
P1 = $1,000, then Q1 = 400 units, and R1 = $400,000
P2 = $1,750, then Q2 = 250 units, and R2 = $437,500
To do:
(a) increase the price, or
(b) decrease the price, or
(c) keep the price at $1,750?
SOLUTION: The price that MAX the revenue shall be: $2,250,
$2,000,
$1,750,
$1,500,
$1,250?

Demand function
Correct answer:
The “best” price to MAX the revenue would be: $1,500
Popt = $1,500, then Qopt = 300 units, and RMAX = $450,000
To do:
(a) increase the price
(b) decrease the price
(c) keep the price at $1,750
This can be solved through (1) finding the demand function equation, and (2) solving a revenue maximization problem.

Demand function
Can be found using the approaches:
Sales tests:
P1, Q1
P2, Q2
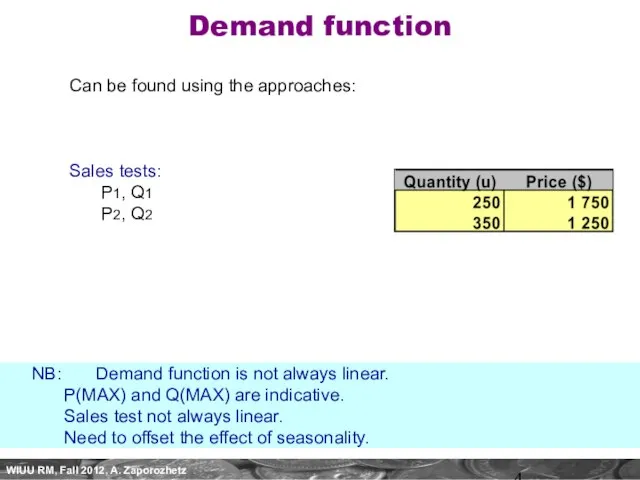
NB: Demand function is not always linear.
P(MAX) and Q(MAX) are indicative.
Sales test not always linear.
Need to offset the effect of seasonality.

Demand Function Equation
Y = a + b*X, basic linear equation
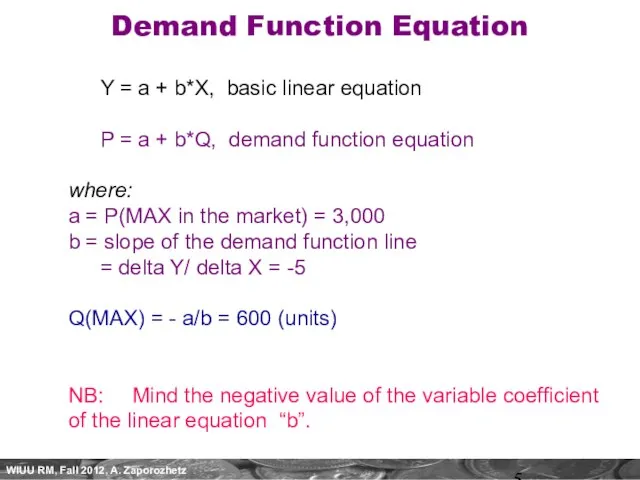
P = a + b*Q, demand function equation
where:
a = P(MAX in the market) = 3,000
b = slope of the demand function line
= delta Y/ delta X = -5
Q(MAX) = - a/b = 600 (units)
NB: Mind the negative value of the variable coefficient of the linear equation “b”.

Task: Revenue maximization
Q*(Revenue MAX) = - a/2b = 300 (u)
Substitute Q* into the Demand function equation,
will find P* (= the price at Q* point)
P*= 3,000 +(-5)*300 = $1,500
R* = P* x Q* = 450,000
NB: R* is highest revenue possible at the current demand.

Profit maximization
Q** (Profit MAX) = - (a – VC(u)) / 2b
P** shall correspond to the value of Q**
Data needed:
fixed and variable costs
FC = $100,000
VC(u) = $500
Q** = 250(u), then
P** = 1,750, then
R** = 437,500, and
Pr** = R** - FC – VC(u)Q** = $212,500
Pr** is highest operating profit possible at the current demand and total costs