Capital badgeting

What is
Capital Budgeting (CB)?
The process of identifying, analyzing, and selecting investment projects whose cash flows are expected to extend beyond one year.
CB is NOT the same as Budgeting (=preparing annual financial plans and proforma statements for a business company)

The Capital Budgeting Process
Generate investment proposals consistent with the firm’s strategic objectives.
Estimate after-tax cash flows for the investment projects.
Evaluate project cash flows.
NB: CF, FCF – no unified methodology

The Capital Budgeting Process
Select projects based on a value-maximizing acceptance criterion.
Reevaluate implemented investment projects continually and perform post-audits for completed projects.

Investment Project Proposals
1. New products or product modifications
Replacement of existing equipment or buildings
Real estate: hotels, etc
R&D
Exploration
Other (e.g., safety- or pollution-related)

Estimating After-Tax Incremental Cash Flows
Cash (not accounting income) flows
Excluding financing costs
After-tax flows
Incremental flows
Basic characteristics of relevant project flows

Estimating After-Tax Incremental Cash Flows
Ignore sunk costs
Include project-driven changes in working capital
Include effects of inflation
Principles that must be adhered to in the estimation

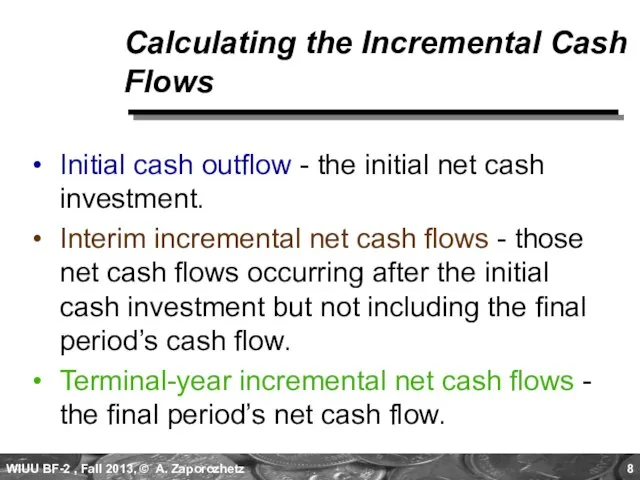
Calculating the Incremental Cash Flows
Initial cash outflow - the initial net cash investment.
Interim incremental net cash flows - those net cash flows occurring after the initial cash investment but not including the final period’s cash flow.
Terminal-year incremental net cash flows - the final period’s net cash flow.

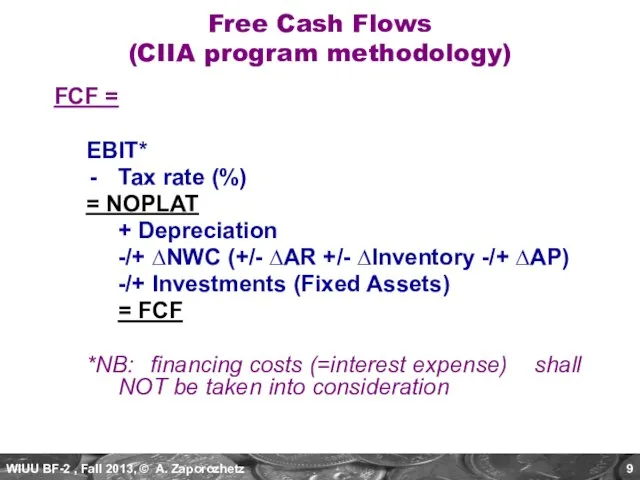
FCF =
EBIT*
Tax rate (%)
= NOPLAT
+ Depreciation
-/+ ∆NWC (+/- ∆AR +/- ∆Inventory -/+ ∆AP)
-/+ Investments (Fixed Assets)
= FCF
*NB: financing costs (=interest expense) shall NOT be taken into consideration
Free Cash Flows
(CIIA program methodology)

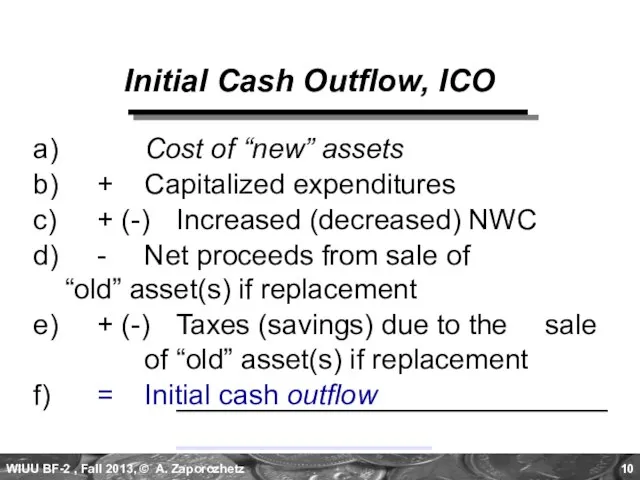
Initial Cash Outflow, ICO
a) Cost of “new” assets
b) + Capitalized expenditures
c) + (-) Increased (decreased) NWC
d) - Net proceeds from sale of “old” asset(s) if replacement
e) + (-) Taxes (savings) due to the sale of “old” asset(s) if replacement
f) = Initial cash outflow

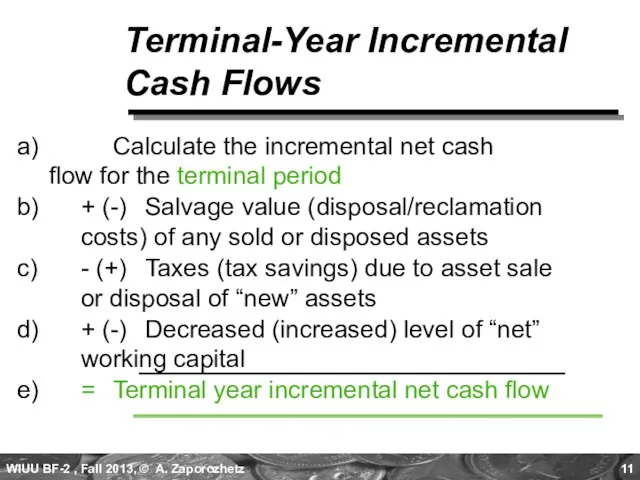
Terminal-Year Incremental Cash Flows
a) Calculate the incremental net cash flow for the terminal period
b) + (-) Salvage value (disposal/reclamation costs) of any sold or disposed assets
c) - (+) Taxes (tax savings) due to asset sale or disposal of “new” assets
d) + (-) Decreased (increased) level of “net” working capital
e) = Terminal year incremental net cash flow

Project Evaluation: Alternative Methods
Payback Period (PBP)
Discounted PBP (DPBP)
Internal Rate of Return (IRR)
Net Present Value (NPV) – most popular ($)
Profitability Index (PI)
Other methods: ARR, MIRR, EAC…

Proposed Project Data
Julie is evaluating a new project for her firm, Basket Wonders (BW). She has determined that the after-tax cash flows for the project will be $10,000; $12,000; $15,000; $10,000; and $7,000, respectively, for each of the Years 1 through 5.
The ICO is $40,000.

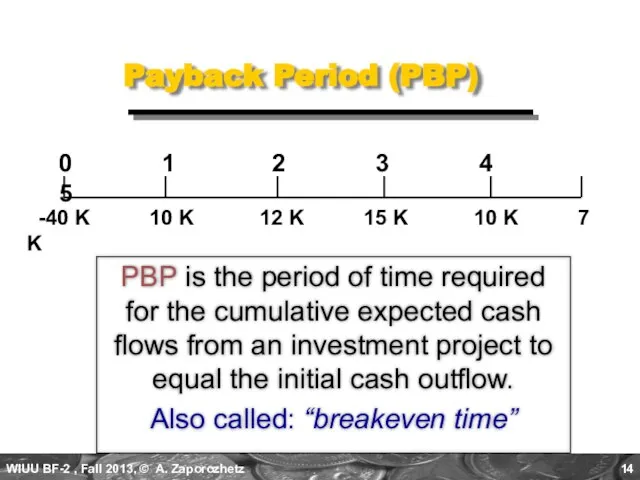
Payback Period (PBP)
PBP is the period of time required for the cumulative expected cash flows from an investment project to equal the initial cash outflow.
Also called: “breakeven time”
0 1 2 3 4 5
-40 K 10 K 12 K 15 K 10 K 7 K

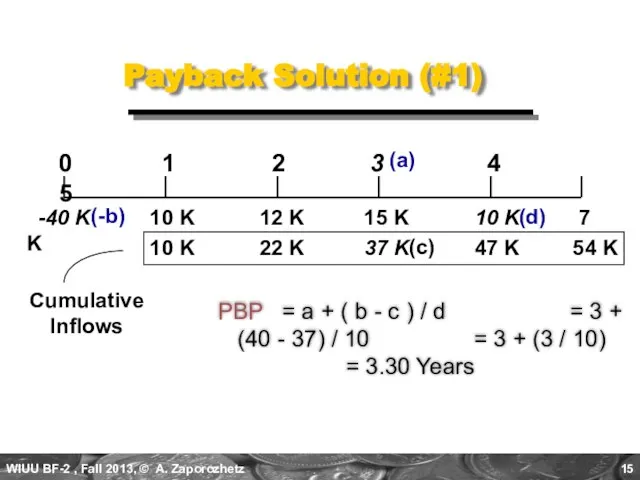
(c)
10 K 22 K 37 K 47 K 54 K
Payback Solution (#1)
PBP = a + ( b - c ) / d = 3 + (40 - 37) / 10 = 3 + (3 / 10) = 3.30 Years
0 1 2 3 4 5
-40 K 10 K 12 K 15 K 10 K 7 K
Cumulative
Inflows
(a)
(-b)
(d)

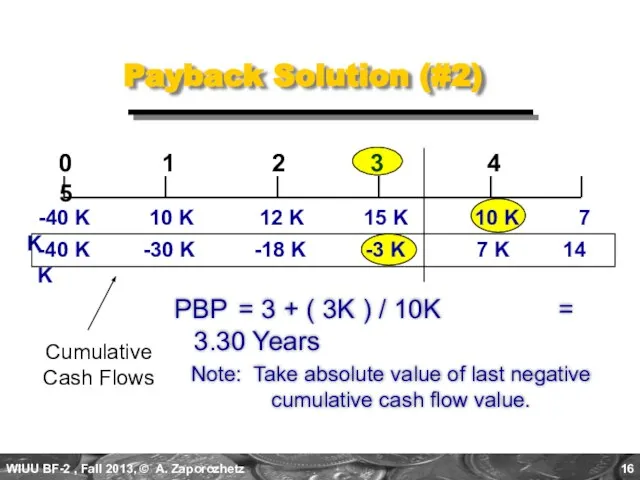
Payback Solution (#2)
PBP = 3 + ( 3K ) / 10K = 3.30 Years
Note: Take absolute value of last negative cumulative cash flow value.
Cumulative
Cash Flows
-40 K 10 K 12 K 15 K 10 K 7 K
0 1 2 3 4 5
-40 K -30 K -18 K -3 K 7 K 14 K

PBP Acceptance Criterion
Yes! The firm will receive back the initial cash outlay in less than 3.5 years.
[3.3 Years < 3.5 Year MAX]
The management of Basket Wonders has set a maximum PBP of 3.5 years for projects of this type.
Should this project be accepted?

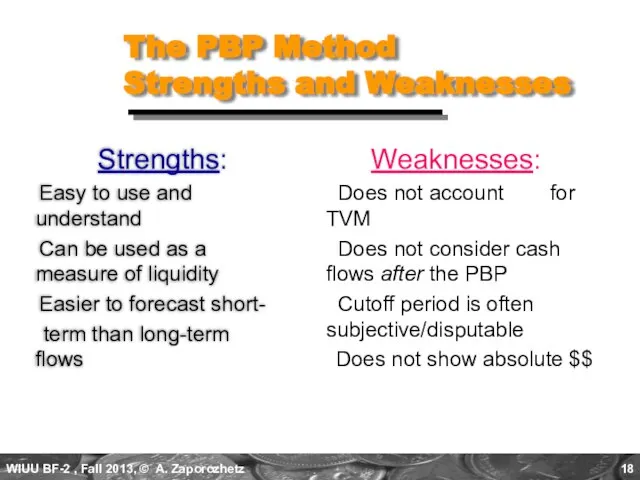
The PBP Method
Strengths and Weaknesses
Strengths:
Easy to use and understand
Can be used as a measure of liquidity
Easier to forecast short-
term than long-term flows
Weaknesses:
Does not account for TVM
Does not consider cash flows after the PBP
Cutoff period is often subjective/disputable
Does not show absolute $$

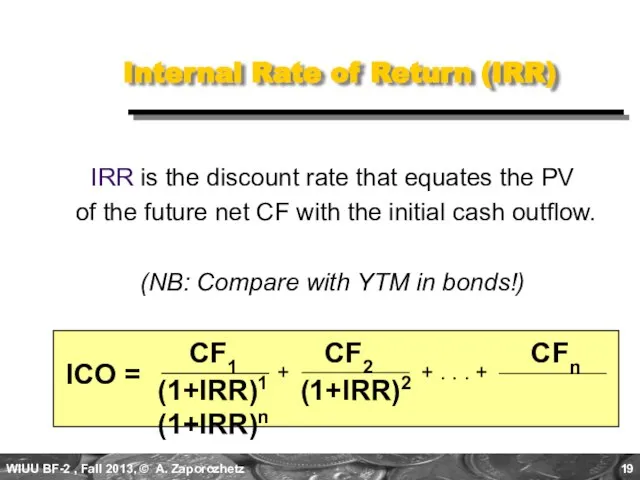
Internal Rate of Return (IRR)
IRR is the discount rate that equates the PV
of the future net CF with the initial cash outflow.
(NB: Compare with YTM in bonds!)
CF1 CF2 CFn
(1+IRR)1 (1+IRR)2 (1+IRR)n
+ . . . +
+
ICO =

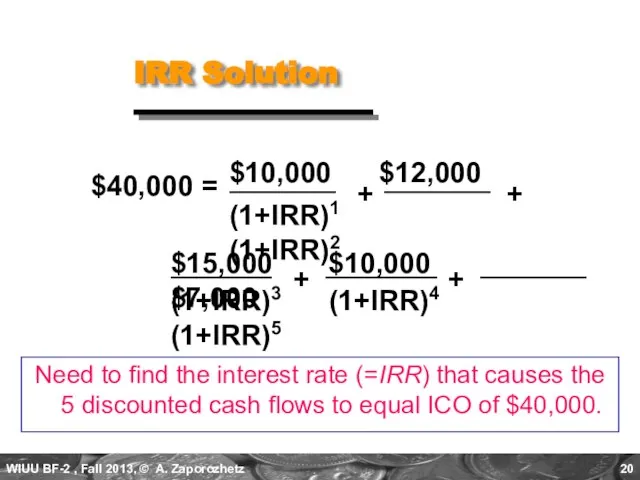
$15,000 $10,000 $7,000
IRR Solution
$10,000 $12,000
(1+IRR)1 (1+IRR)2
Need to find the interest rate (=IRR) that causes the 5 discounted cash flows to equal ICO of $40,000.
+
+
+
+
$40,000 =
(1+IRR)3 (1+IRR)4 (1+IRR)5

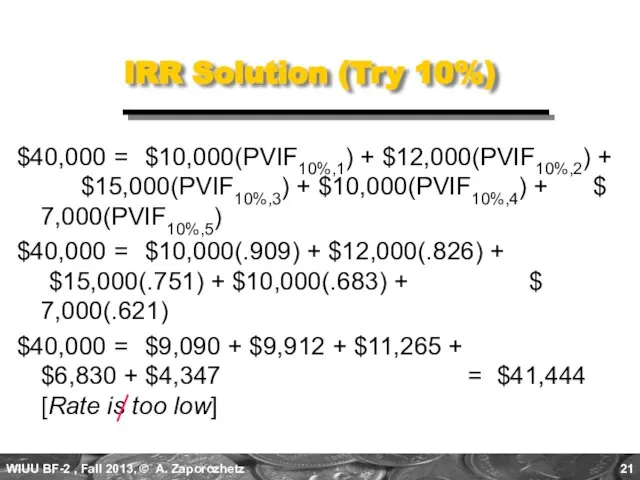
IRR Solution (Try 10%)
$40,000 = $10,000(PVIF10%,1) + $12,000(PVIF10%,2) + $15,000(PVIF10%,3) + $10,000(PVIF10%,4) + $ 7,000(PVIF10%,5)
$40,000 = $10,000(.909) + $12,000(.826) + $15,000(.751) + $10,000(.683) + $ 7,000(.621)
$40,000 = $9,090 + $9,912 + $11,265 + $6,830 + $4,347 = $41,444 [Rate is too low]

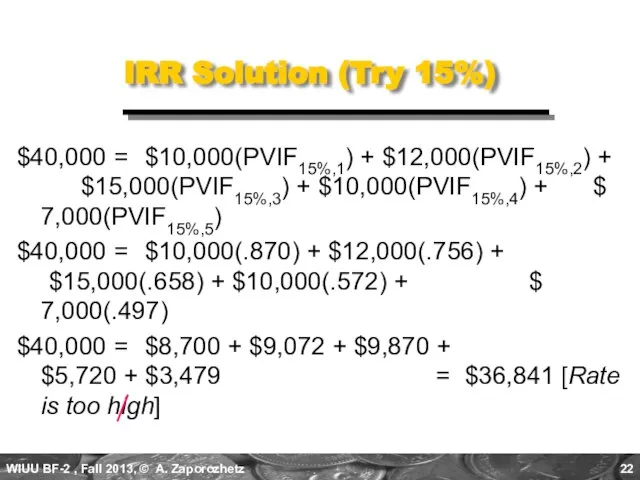
IRR Solution (Try 15%)
$40,000 = $10,000(PVIF15%,1) + $12,000(PVIF15%,2) + $15,000(PVIF15%,3) + $10,000(PVIF15%,4) + $ 7,000(PVIF15%,5)
$40,000 = $10,000(.870) + $12,000(.756) + $15,000(.658) + $10,000(.572) + $ 7,000(.497)
$40,000 = $8,700 + $9,072 + $9,870 + $5,720 + $3,479 = $36,841 [Rate is too high]

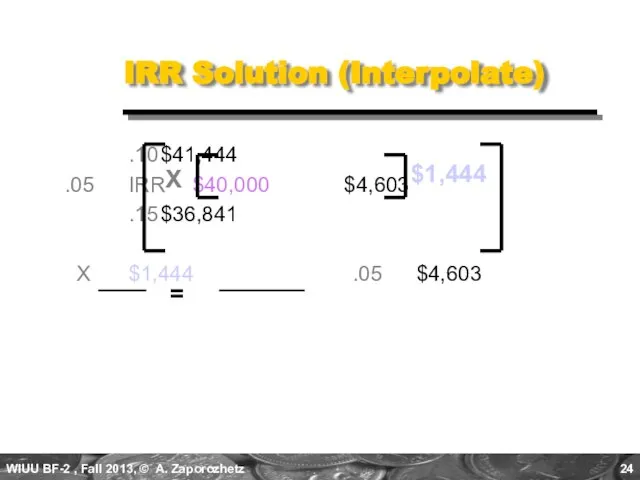
0.10 $41,444
0.05 IRR $40,000 $4,603
0.15 $36,841
X $1,444 0.05 $4,603
IRR Solution (Interpolate)
$1,444
X
=

.10 $41,444
.05 IRR $40,000 $4,603
.15 $36,841
X $1,444 .05 $4,603
IRR Solution (Interpolate)
$1,444
X
=

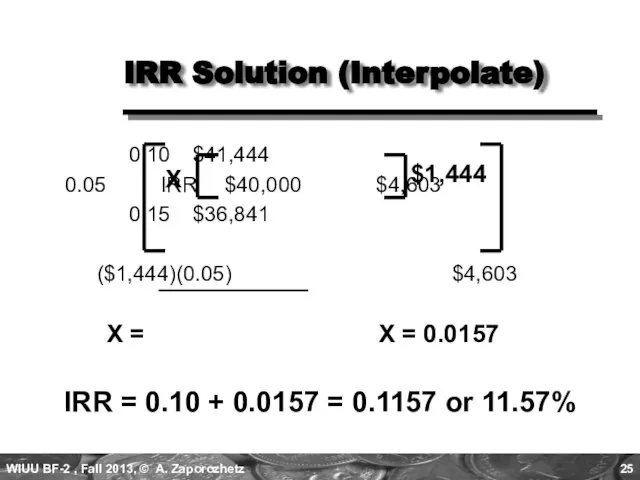
0.10 $41,444
0.05 IRR $40,000 $4,603
0.15 $36,841
($1,444)(0.05) $4,603
IRR Solution (Interpolate)
$1,444
X
X =
X = 0.0157
IRR = 0.10 + 0.0157 = 0.1157 or 11.57%

IRR Acceptance Criterion
No! The firm will “receive” 11.57% for each dollar “required” for this project at a cost of 13%. [ IRR < RRR, “Hurdle Rate” ]
The management of Basket Wonders has determined that the hurdle rate is 13% for projects of this type.
Should this project be accepted?

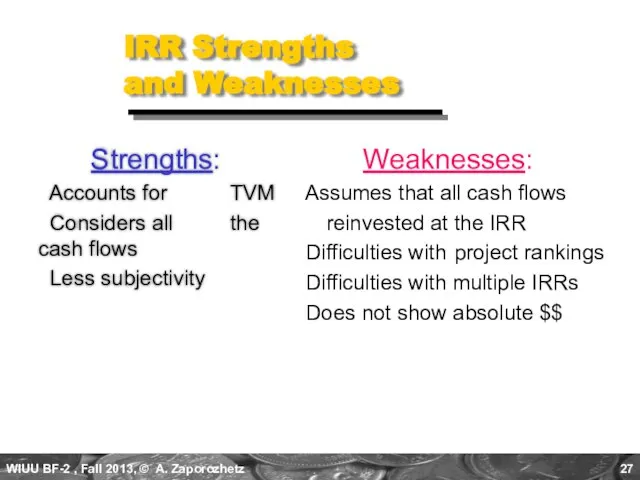
IRR Strengths
and Weaknesses
Strengths:
Accounts for TVM
Considers all the cash flows
Less subjectivity
Weaknesses:
Assumes that all cash flows
reinvested at the IRR
Difficulties with project rankings
Difficulties with multiple IRRs
Does not show absolute $$

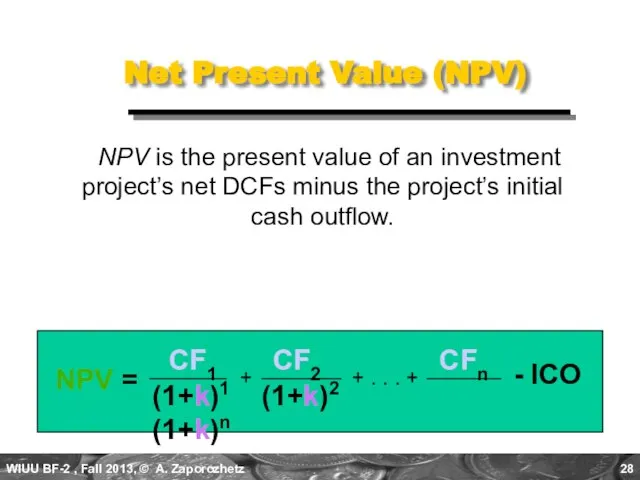
Net Present Value (NPV)
NPV is the present value of an investment project’s net DCFs minus the project’s initial cash outflow.
CF1 CF2 CFn
(1+k)1 (1+k)2 (1+k)n
+ . . . +
+
- ICO
NPV =

Basket Wonders has determined that the appropriate discount rate (k) for this project is 13%.
$10,000 $7,000
NPV Solution
$10,000 $12,000 $15,000
(1.13)1 (1.13)2 (1.13)3
+
+
+
- $40,000
(1.13)4 (1.13)5
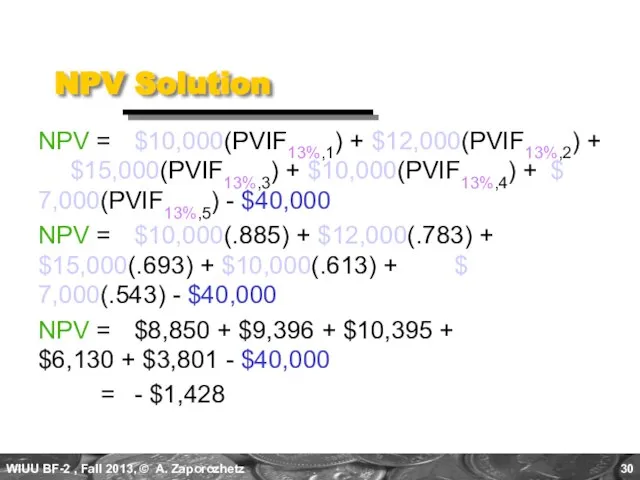
NPV =
+

NPV Solution
NPV = $10,000(PVIF13%,1) + $12,000(PVIF13%,2) + $15,000(PVIF13%,3) + $10,000(PVIF13%,4) + $ 7,000(PVIF13%,5) - $40,000
NPV = $10,000(.885) + $12,000(.783) + $15,000(.693) + $10,000(.613) + $ 7,000(.543) - $40,000
NPV = $8,850 + $9,396 + $10,395 + $6,130 + $3,801 - $40,000
= - $1,428

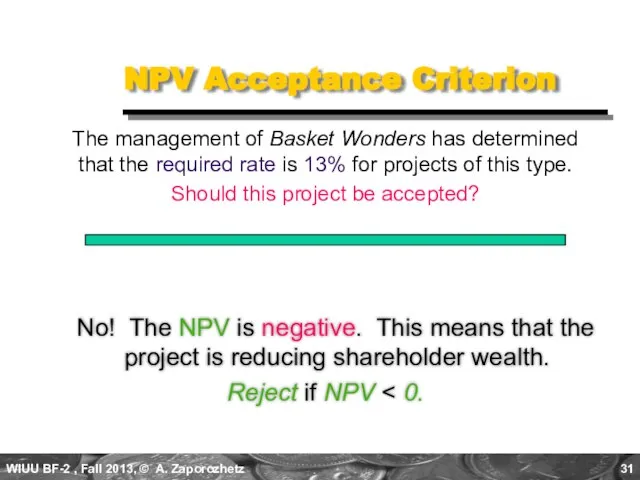
NPV Acceptance Criterion
No! The NPV is negative. This means that the project is reducing shareholder wealth.
Reject if NPV < 0.
The management of Basket Wonders has determined that the required rate is 13% for projects of this type.
Should this project be accepted?

NPV Strengths
and Weaknesses
Strengths:
(Cash flows assumed to be reinvested at the hurdle rate.)
Accounts for TVM.
Considers all cash flows.
Shows absolute $ value
in PRESENT $.
Weaknesses:
Problems with projects’ sizes
“Correct” RRR (?)

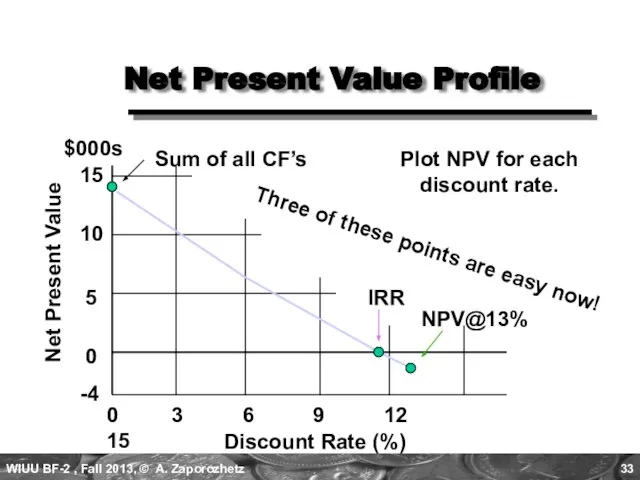
Net Present Value Profile
Discount Rate (%)
0 3 6 9 12 15
IRR
NPV@13%
Sum of all CF’s
Plot NPV for each
discount rate.
Three of these points are easy now!
Net Present Value
$000s
15
10
5
0
-4

Profitability Index (PI)
PI is the ratio of the present value of a project’s future net cash flows to the project’s initial cash outflow.
CF1 CF2 CFn
(1+k)1 (1+k)2 (1+k)n
+ . . . +
+
ICO
PI =
PI = 1 + [ NPV / |ICO| ]
<< OR >>

PI Acceptance Criterion
No! The PI is less than 1.00.
This means that the project is not profitable.
Reject if PI < 1.00 times.
PI = $38,572 / $40,000
= 0.9643
Should this project be accepted?

PI Strengths
and Weaknesses
Strengths:
Same as NPV
Allows for comparison of different scale and lifetime projects
The correct solution in capital rationing
Weaknesses:
Provides only relative profitability (in times)
Potential ranking problems

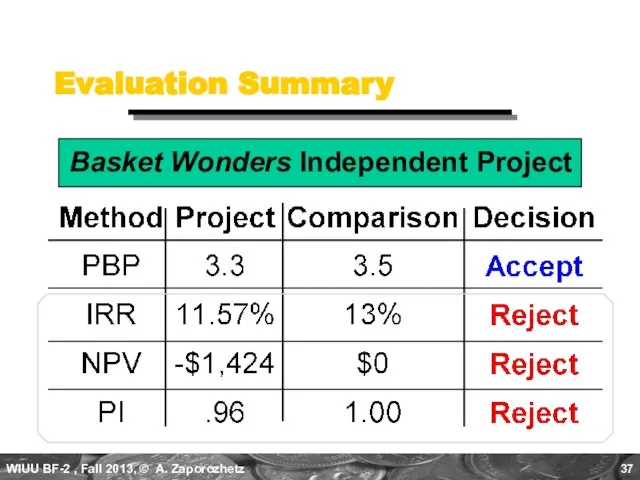
Evaluation Summary
Basket Wonders Independent Project

Other Project Relationships
Mutually Exclusive - A project whose acceptance precludes the acceptance of one or more alternative projects.
Dependent - A project whose acceptance depends on the acceptance of one or more other projects.

Potential Problems
Under Mutual Exclusivity
A. Scale of Investment
B. Cash-flow Pattern
C. Project Life Ranking of project proposals may create contradictory results.

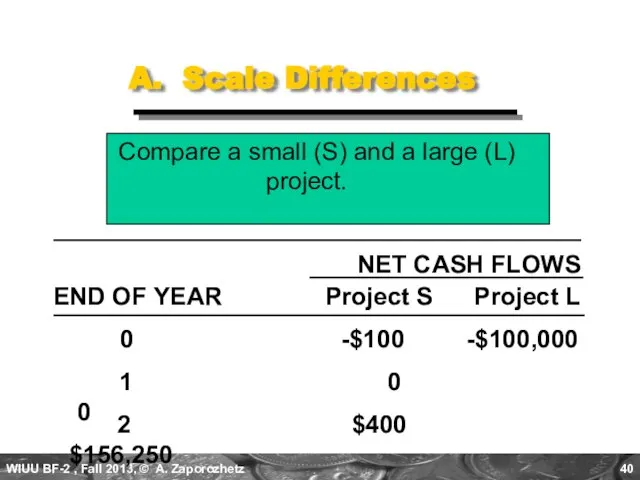
A. Scale Differences
Compare a small (S) and a large (L) project.
NET CASH FLOWS
Project S Project L
END OF YEAR
0 -$100 -$100,000
1 0 0
2 $400 $156,250

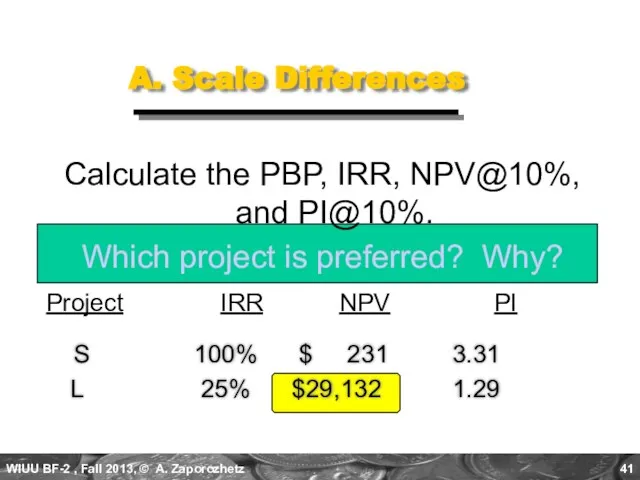
A. Scale Differences
Calculate the PBP, IRR, NPV@10%, and PI@10%.
Which project is preferred? Why?
Project IRR NPV PI
S 100% $ 231 3.31
L 25% $29,132 1.29

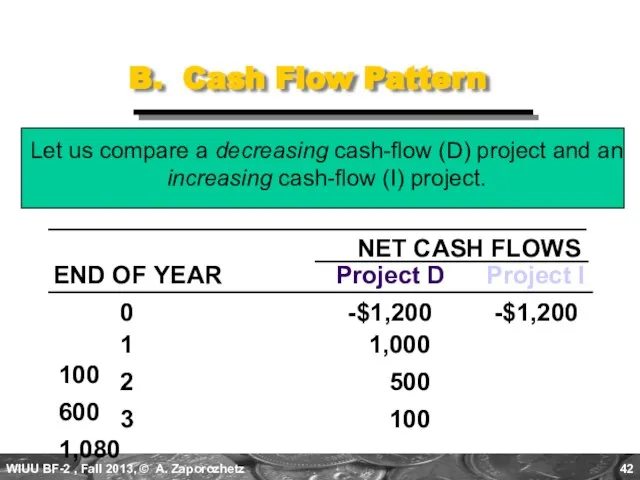
B. Cash Flow Pattern
Let us compare a decreasing cash-flow (D) project and an increasing cash-flow (I) project.
NET CASH FLOWS
Project D Project I
END OF YEAR
0 -$1,200 -$1,200
1 1,000 100
2 500 600
3 100 1,080

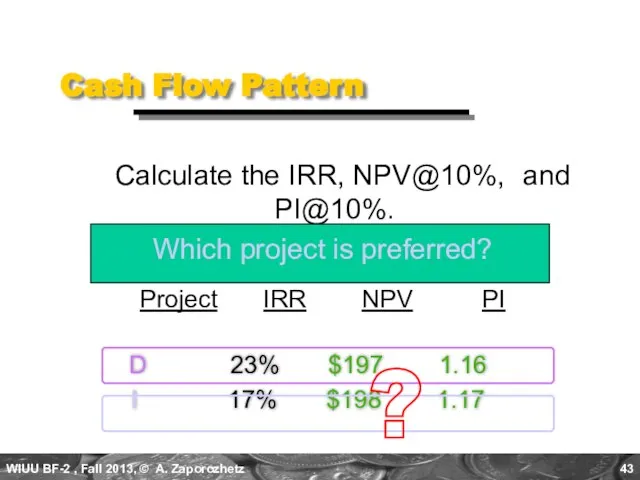
D 23% $197 1.16
I 17% $198 1.17
Cash Flow Pattern
Calculate the IRR, NPV@10%, and PI@10%.
Which project is preferred?
Project IRR NPV PI
?

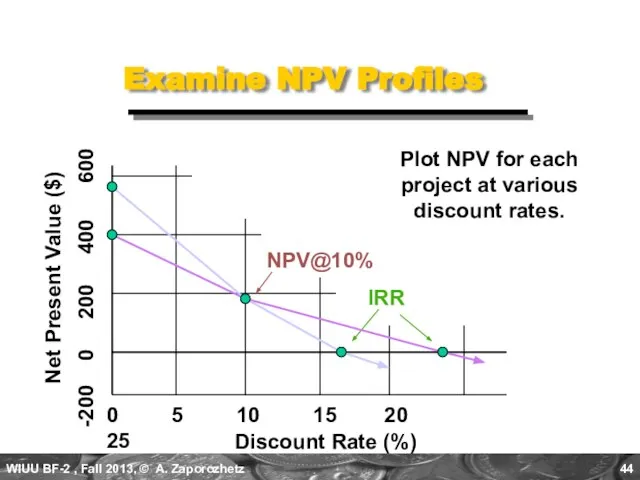
Examine NPV Profiles
Discount Rate (%)
0 5 10 15 20 25
-200 0 200 400 600
IRR
NPV@10%
Plot NPV for each
project at various
discount rates.
Net Present Value ($)

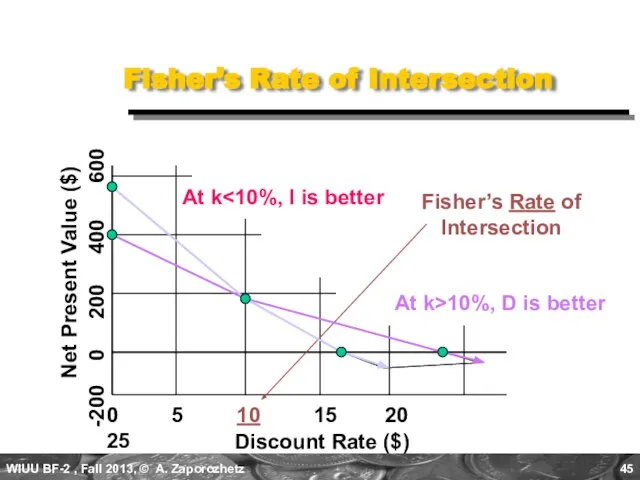
Fisher’s Rate of Intersection
Discount Rate ($)
0 5 10 15 20 25
-200 0 200 400 600
Net Present Value ($)
At k<10%, I is better
Fisher’s Rate of
Intersection
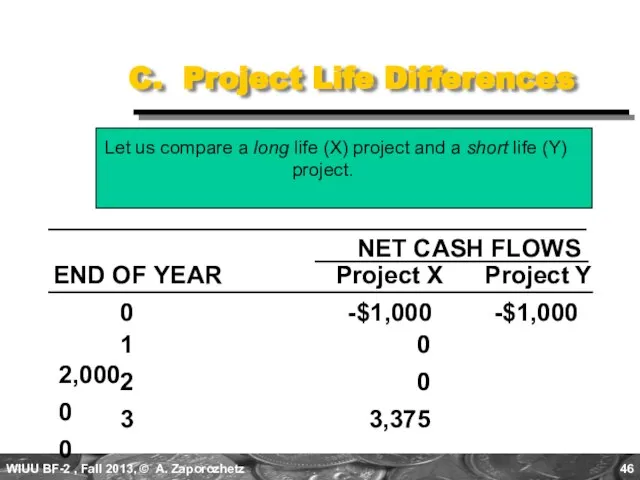
At k>10%, D is better

C. Project Life Differences
Let us compare a long life (X) project and a short life (Y) project.
NET CASH FLOWS
Project X Project Y
END OF YEAR
0 -$1,000 -$1,000
1 0 2,000
2 0 0
3 3,375 0

X 50% $1,536 2.54
Y 100% $ 818 1.82
Project Life Differences
Calculate the PBP, IRR, NPV@10%, and PI@10%.
Which project is preferred? Why?
Project IRR NPV PI
?

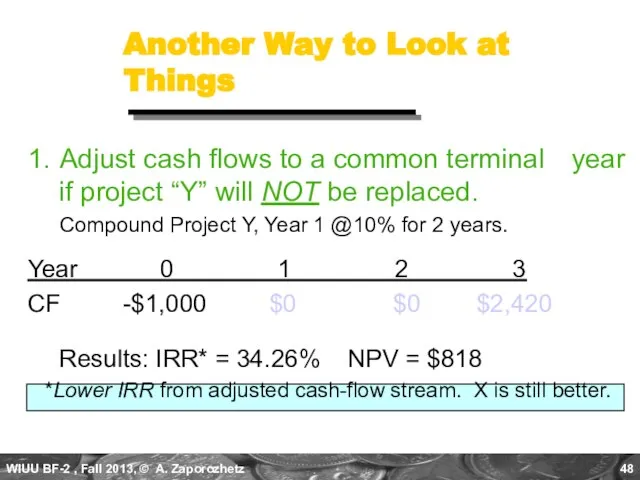
Another Way to Look at Things
1. Adjust cash flows to a common terminal year if project “Y” will NOT be replaced.
Compound Project Y, Year 1 @10% for 2 years.
Year 0 1 2 3
CF -$1,000 $0 $0 $2,420
Results: IRR* = 34.26% NPV = $818
*Lower IRR from adjusted cash-flow stream. X is still better.

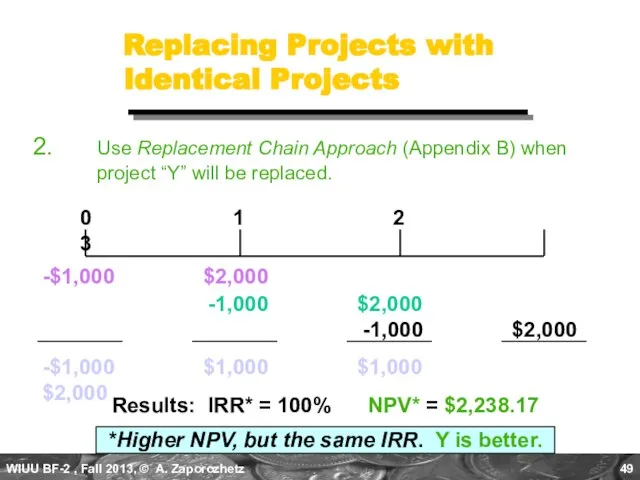
Replacing Projects with Identical Projects
2. Use Replacement Chain Approach (Appendix B) when project “Y” will be replaced.
0 1 2 3
-$1,000 $2,000
-1,000 $2,000
-1,000 $2,000
-$1,000 $1,000 $1,000 $2,000
Results: IRR* = 100% NPV* = $2,238.17
*Higher NPV, but the same IRR. Y is better.

Capital Rationing
Capital Rationing occurs when a constraint (or budget ceiling) is placed on the total size of capital expenditures during a particular period.
Example: Julie Miller must determine what investment opportunities to undertake for Basket Wonders (BW). She is limited to a maximum expenditure of $32,500 only for this capital budgeting period.

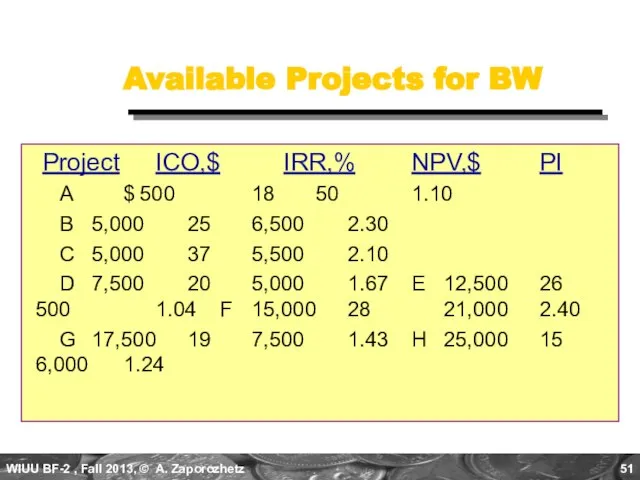
Available Projects for BW
Project ICO,$ IRR,% NPV,$ PI
A $ 500 18 50 1.10
B 5,000 25 6,500 2.30
C 5,000 37 5,500 2.10
D 7,500 20 5,000 1.67 E 12,500 26 500 1.04 F 15,000 28 21,000 2.40
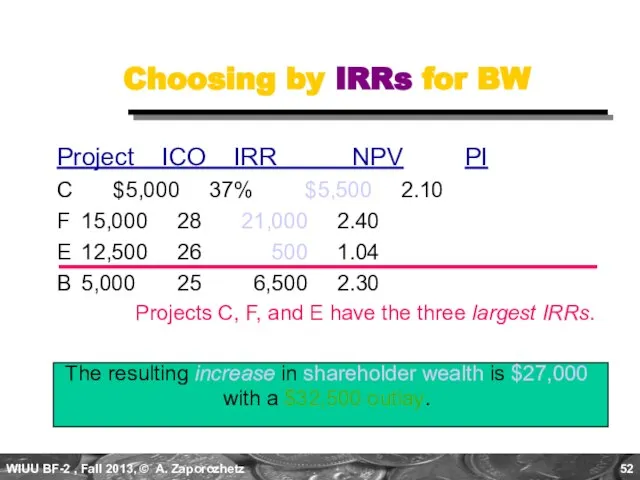
G 17,500 19 7,500 1.43 H 25,000 15 6,000 1.24

Choosing by IRRs for BW
Project ICO IRR NPV PI
C $5,000 37% $5,500 2.10
F 15,000 28 21,000 2.40
E 12,500 26 500 1.04
B 5,000 25 6,500 2.30
Projects C, F, and E have the three largest IRRs.
The resulting increase in shareholder wealth is $27,000 with a $32,500 outlay.

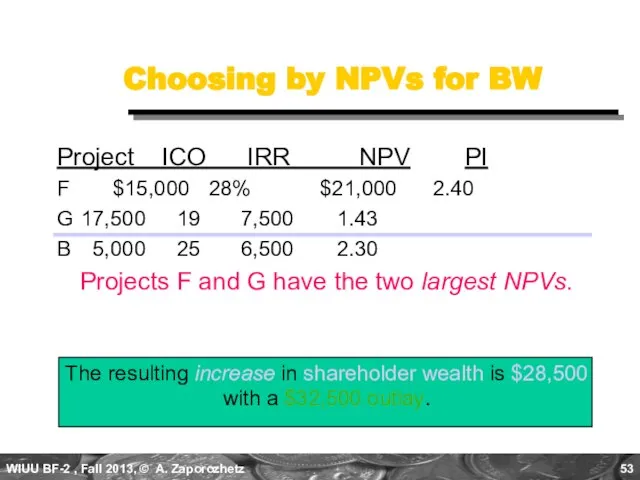
Choosing by NPVs for BW
Project ICO IRR NPV PI
F $15,000 28% $21,000 2.40
G 17,500 19 7,500 1.43
B 5,000 25 6,500 2.30
Projects F and G have the two largest NPVs.
The resulting increase in shareholder wealth is $28,500 with a $32,500 outlay.

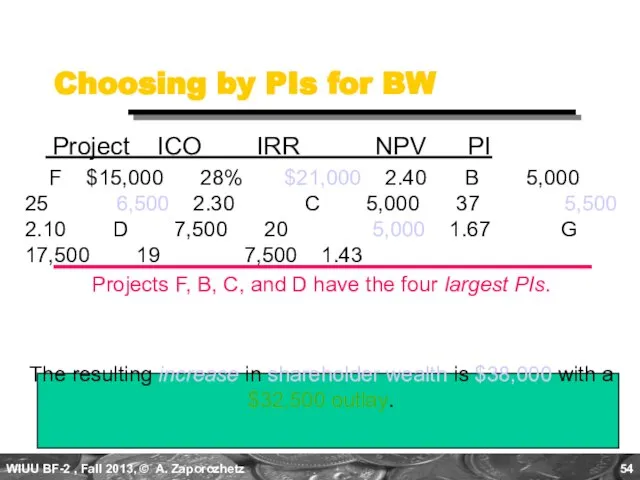
Choosing by PIs for BW
Project ICO IRR NPV PI
F $15,000 28% $21,000 2.40 B 5,000 25 6,500 2.30 C 5,000 37 5,500 2.10 D 7,500 20 5,000 1.67 G 17,500 19 7,500 1.43
Projects F, B, C, and D have the four largest PIs.
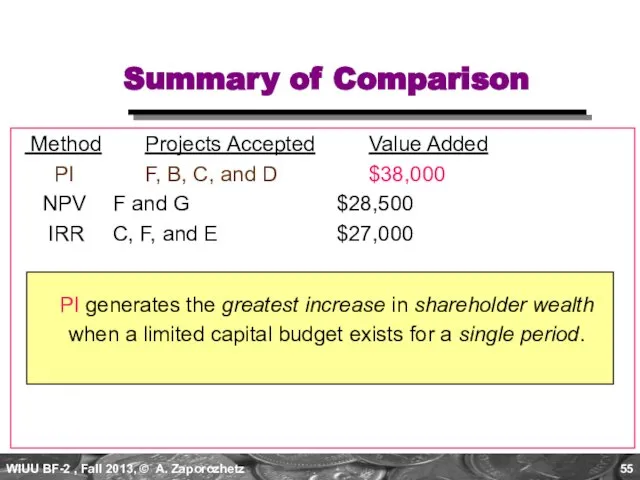
The resulting increase in shareholder wealth is $38,000 with a $32,500 outlay.

Summary of Comparison
Method Projects Accepted Value Added
PI F, B, C, and D $38,000
NPV F and G $28,500
IRR C, F, and E $27,000
PI generates the greatest increase in shareholder wealth
when a limited capital budget exists for a single period.

Post-Completion Audit:
Usus Magister Est Optimus
Post-completion Audit
A formal comparison of the actual costs and benefits of a project with original estimates.
Identify any project weaknesses
Develop a possible set of corrective actions
Provide appropriate feedback
Result: Making better future decisions!

Multiple IRR Problem
Two!! There are as many potential IRRs as there are sign changes.
Let us assume the following cash flow pattern for a project for Years 0 to 4:
-$100 +$100 +$900 -$1,000
How many potential IRRs could this project have?

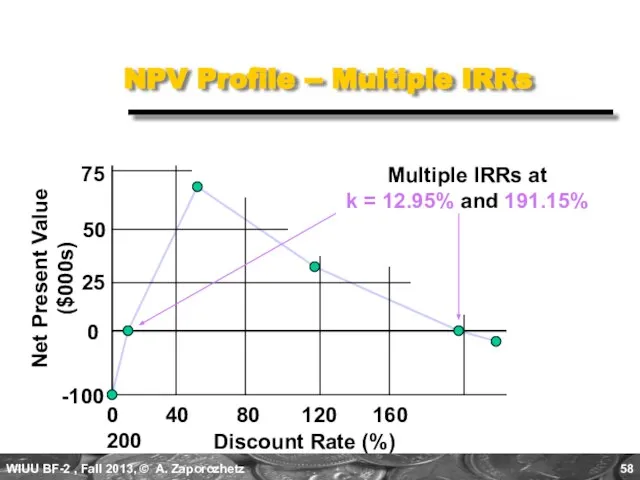
NPV Profile -- Multiple IRRs
Discount Rate (%)
0 40 80 120 160 200
Net Present Value
($000s)
Multiple IRRs at
k = 12.95% and 191.15%
75
50
25
0
-100