Слайд 2Angular momentum. Moment of inertia and more properties of angular motion.
Слайд 3Moment of inertia, I
For linear momentum, p = mv
Similarly, for angular momentum,
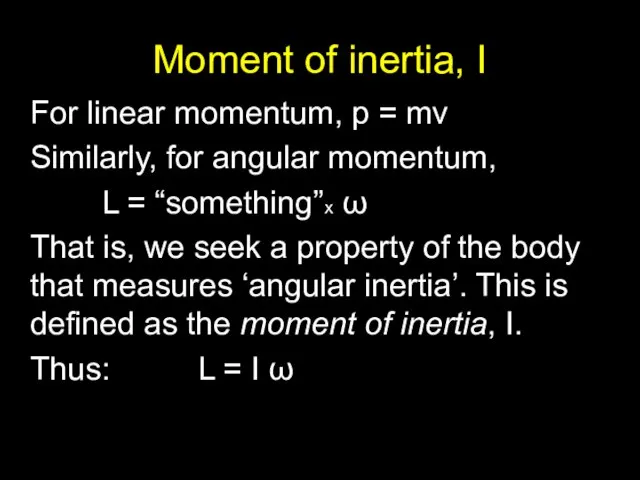
L = “something”x ω
That is, we seek a property of the body that measures ‘angular inertia’. This is defined as the moment of inertia, I.
Thus: L = I ω
Слайд 4One way to get a formula for I
Consider a body rotating with
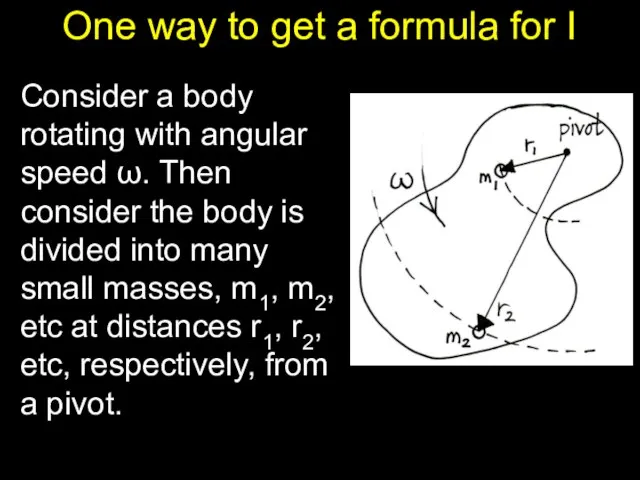
angular speed ω. Then consider the body is divided into many small masses, m1, m2, etc at distances r1, r2, etc, respectively, from a pivot.
Слайд 5Moment of inertia
is the angular speed

Слайд 7More on I
Note: we know that 1) if no external forces act

on the system, linear momentum is conserved.
We now know that 2) if no external torques act on the system, angular momentum is conserved. This explains why, e.g., an ice skater rotates faster when she pulls in her arms.
Слайд 8Conservation of angular momentum
Since L = I ω, if no external torques

act, L is constant.
So, when the skater moves her arms in, closer to her body,
I decreases and therefore ω increases.
Слайд 9Conservation of angular momentum
In (1) I = m1a2 +m2a2
In (2) I =
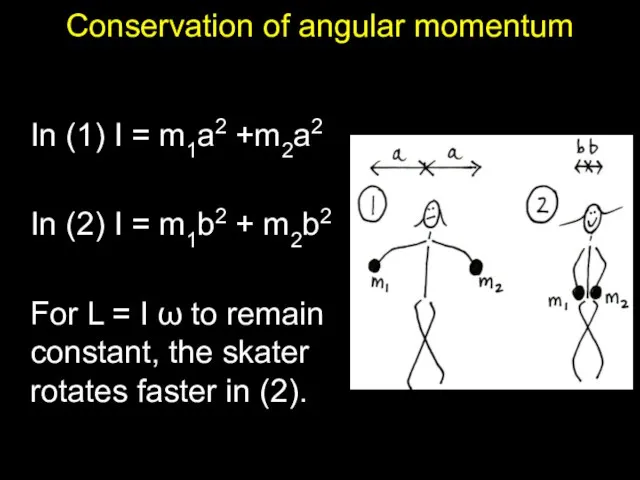
m1b2 + m2b2
For L = I ω to remain constant, the skater rotates faster in (2).
Слайд 10Please don’t try this!
A crazy physics professor stands on a swivel chair

with weights in his outstretched arms. A student causes him to rotate.
The professor brings in his arms and…
Слайд 11Comparison of equations for linear and angular motion
Слайд 12Example 1. Conservation of angular momentum
A horizontal disc rotates around a vertical
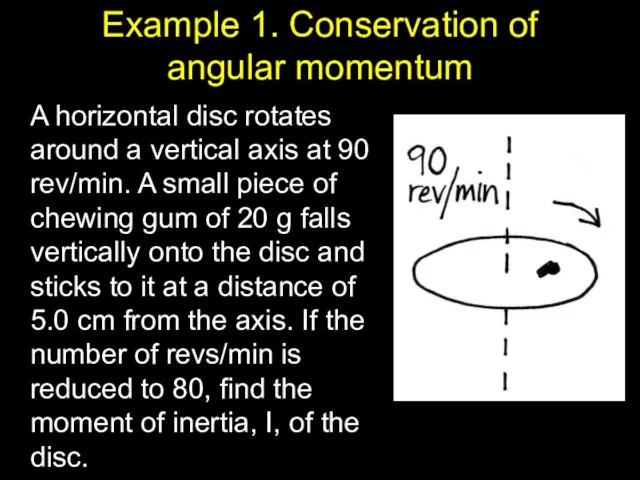
axis at 90 rev/min. A small piece of chewing gum of 20 g falls vertically onto the disc and sticks to it at a distance of 5.0 cm from the axis. If the number of revs/min is reduced to 80, find the moment of inertia, I, of the disc.
Слайд 13Work done by torque
Note: We can also define work done by a

constant torque in moving through an angle θ as: W = τθ
The power
Слайд 14Example 2
An electric motor supplies a power of 500 W to drive
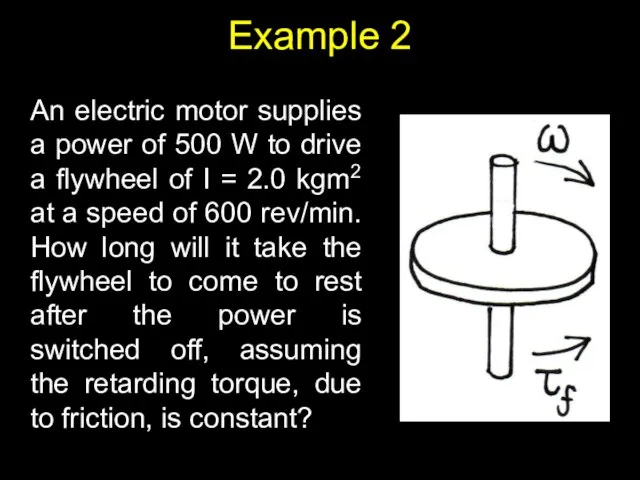
a flywheel of I = 2.0 kgm2 at a speed of 600 rev/min. How long will it take the flywheel to come to rest after the power is switched off, assuming the retarding torque, due to friction, is constant?














 Украина. Донецкая область. Коммунальное предприятие Коммунальник. Функции юридического отдела
Украина. Донецкая область. Коммунальное предприятие Коммунальник. Функции юридического отдела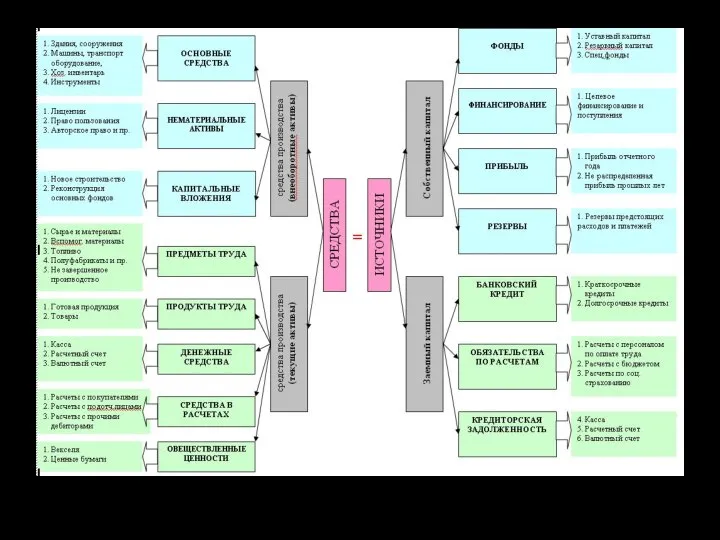 БУ торгово-материальные ценности
БУ торгово-материальные ценности Танха ыйа
Танха ыйа Армейский экшен-кликер Full Metal Clicker (описание игры)
Армейский экшен-кликер Full Metal Clicker (описание игры) Вода в природе и её значение
Вода в природе и её значение Сестринское дело: Потребностно-информационная теория
Сестринское дело: Потребностно-информационная теория !!!
!!! День Защитника Отечества 23 февраля
День Защитника Отечества 23 февраля Мотивация ученика - основное условие успешного обучения
Мотивация ученика - основное условие успешного обучения Вес воздуха. Атмосферное давление
Вес воздуха. Атмосферное давление Руководство по концепции и применению процессного подхода к системам менеджмента
Руководство по концепции и применению процессного подхода к системам менеджмента Управление качеством
Управление качеством Тема исследования: «Любовь в жизни и творчестве М.Цветаевой» Автор: Иванов Николай Руководитель: Ко
Тема исследования: «Любовь в жизни и творчестве М.Цветаевой» Автор: Иванов Николай Руководитель: Ко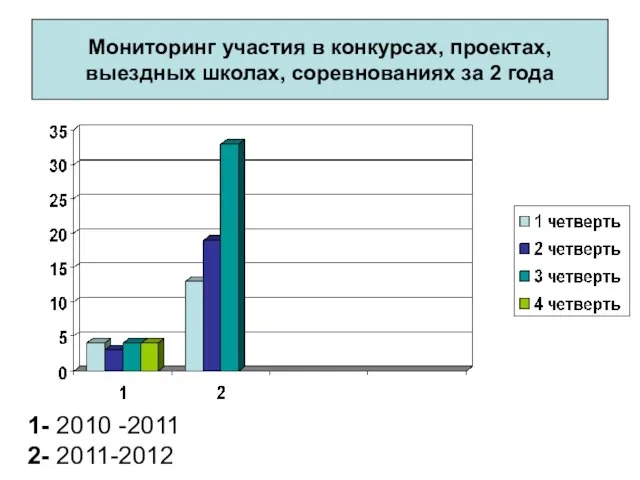 1- 2010 -2011 2- 2011-2012
1- 2010 -2011 2- 2011-2012 Роль бабы яги в сюжетах русских народных сказок
Роль бабы яги в сюжетах русских народных сказок Изобрели топливо XXI века
Изобрели топливо XXI века ГМО
ГМО Средства автоматизации сметных расчетов
Средства автоматизации сметных расчетов Венецианское биенале 2016: ахитектура социальной ответственности
Венецианское биенале 2016: ахитектура социальной ответственности Складки одежды
Складки одежды Презентация на тему НАСЕЛЕНИЕ ЗЕМЛИ
Презентация на тему НАСЕЛЕНИЕ ЗЕМЛИ  Гуляй по улицам с умом
Гуляй по улицам с умом ОБЩИЙ ОБЗОР ПОСОБИЙ ИЗДАТЕЛЬСТВА ДЛЯ ДОШКОЛЬНИКОВ
ОБЩИЙ ОБЗОР ПОСОБИЙ ИЗДАТЕЛЬСТВА ДЛЯ ДОШКОЛЬНИКОВ Государственные и муниципальные финансы. Решение задач
Государственные и муниципальные финансы. Решение задач Электронная почта
Электронная почта Методы креативных технологий
Методы креативных технологий Судебная система Российской Федерации: Мировые судьи
Судебная система Российской Федерации: Мировые судьи Районный конкурс «Юные лидеры образования»
Районный конкурс «Юные лидеры образования»