Содержание
- 2. What is important in the representation of a crystal ?
- 3. What is important in the representation of a crystal ? It is not its shape It
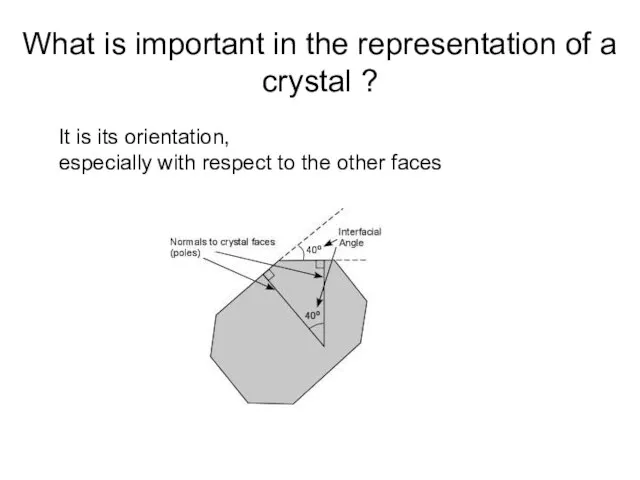
- 4. What is important in the representation of a crystal ? It is its orientation, especially with
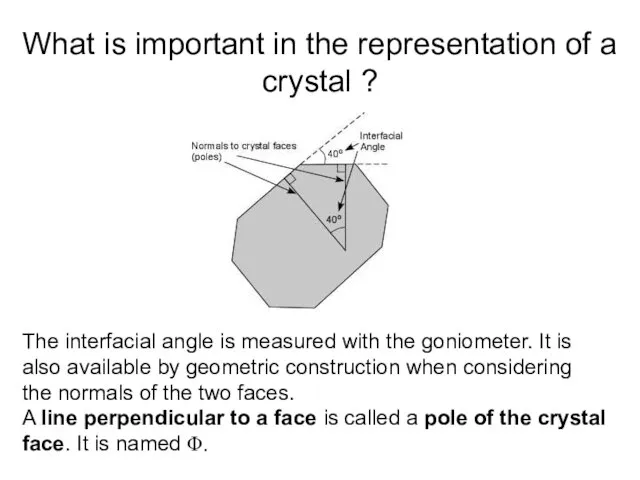
- 5. What is important in the representation of a crystal ? The interfacial angle is measured with
- 6. How to flatten the Earth ? Illustration by Rubens for "Opticorum libri sex philosophis juxta ac
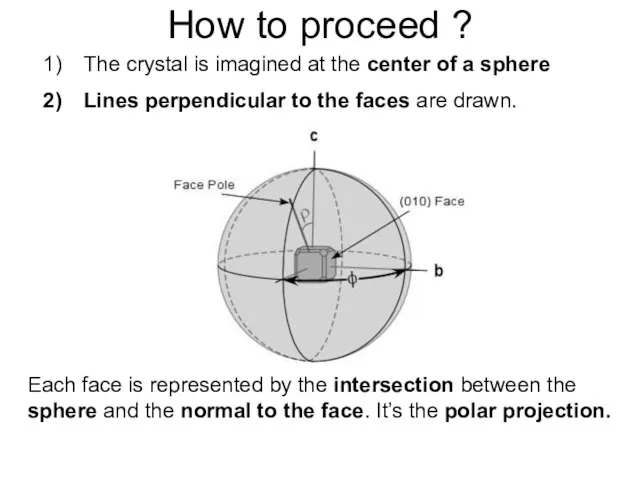
- 7. How to proceed ? The crystal is imagined at the center of a sphere Lines perpendicular
- 8. Cyclographic projection The angle between the normals of two faces are found on the sphere
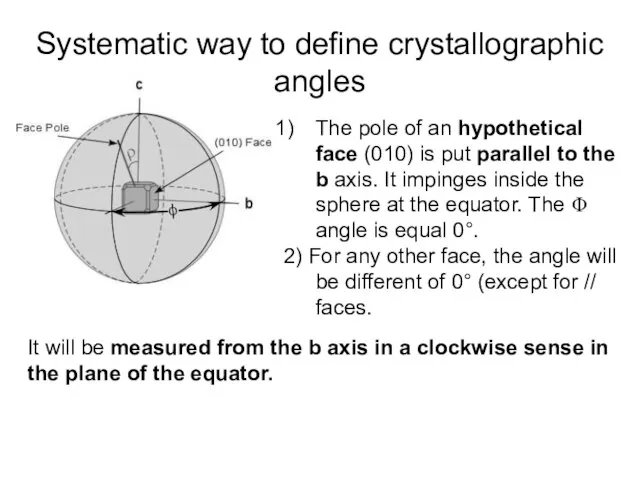
- 9. Systematic way to define crystallographic angles The pole of an hypothetical face (010) is put parallel
- 10. Systematic way to define crystallographic angles A ρ angle is defined between the c axis and
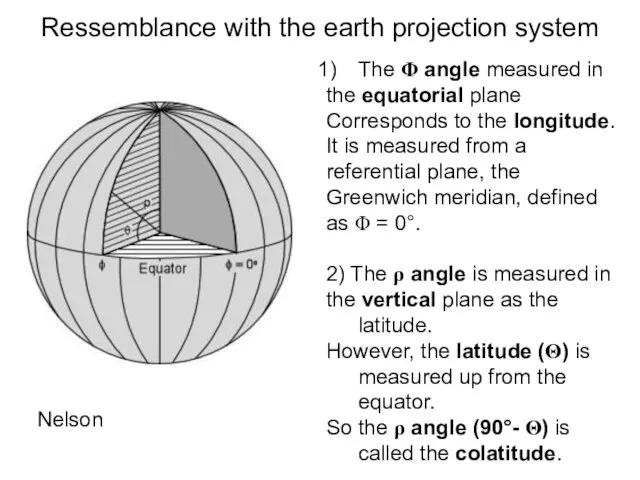
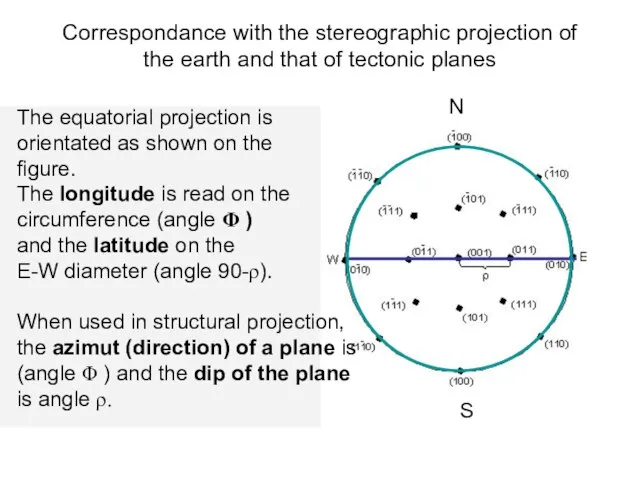
- 11. Ressemblance with the earth projection system Nelson The Φ angle measured in the equatorial plane Corresponds
- 12. Example for a crystal - face (111) The Φ angle measured in the equatorial plane, clockwise
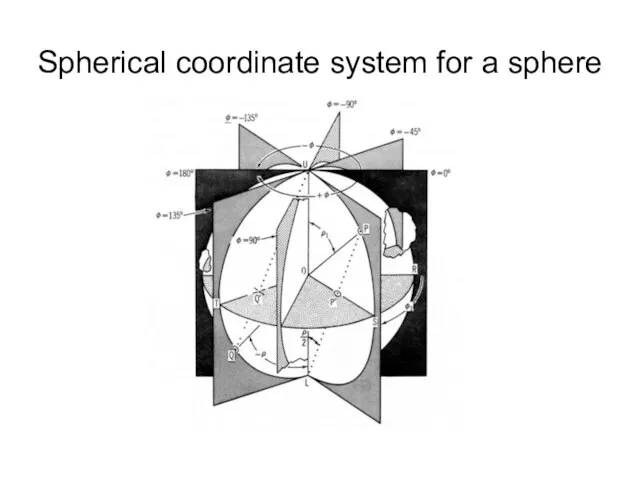
- 13. Spherical coordinate system for a sphere
- 14. Projection on a plane - Stereographic projection 2) The second step is to imagine a system
- 15. Why to use a stereographic projection in crystallography?
- 16. Why to use a stereographic projection in crystallography? The projection of a circle is maintained as
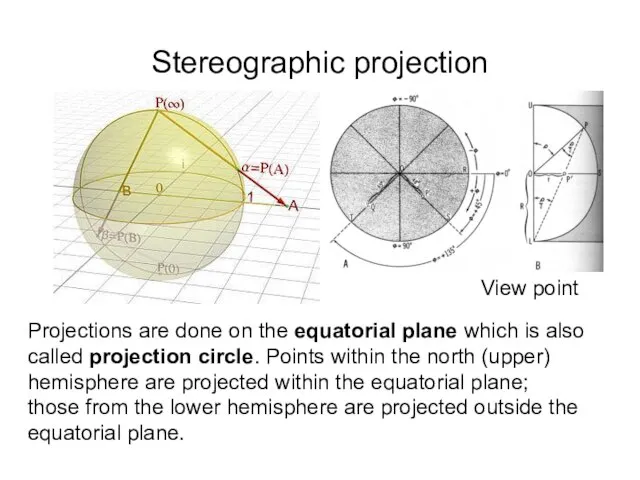
- 17. Stereographic projection Projections are done on the equatorial plane which is also called projection circle. Points
- 18. Direct and reciprocal projections N S
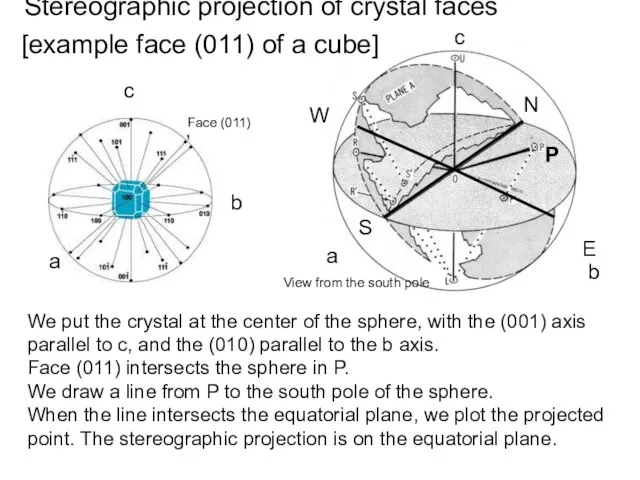
- 19. Stereographic projection of crystal faces c b a View from the south pole [example face (011)
- 20. Stereographic projection of crystal faces [example face (011) of a cube]
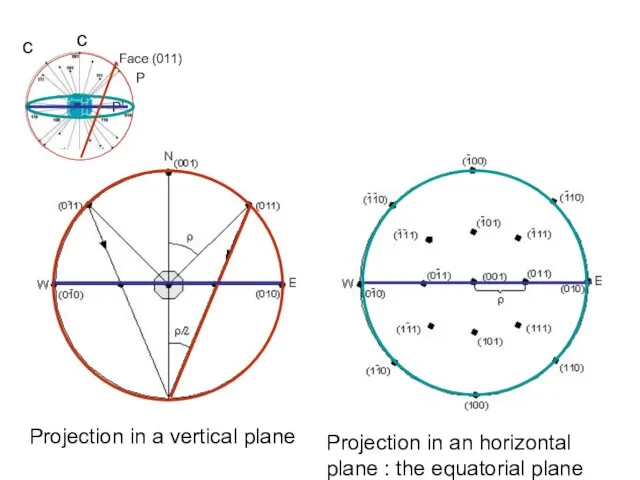
- 21. c Projection in a vertical plane Projection in an horizontal plane : the equatorial plane
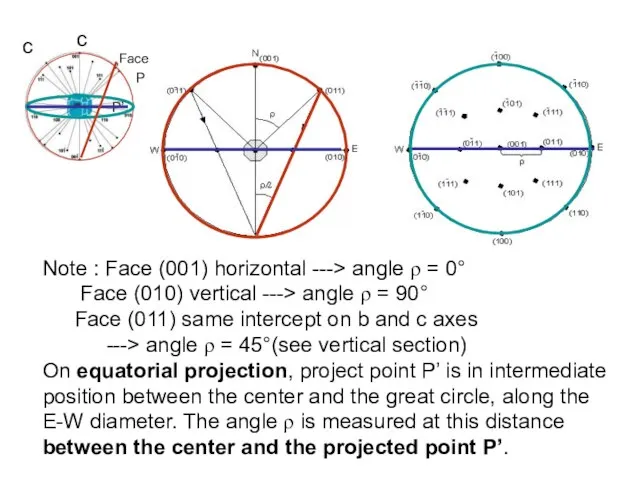
- 22. c Note : Face (001) horizontal ---> angle ρ = 0° Face (010) vertical ---> angle
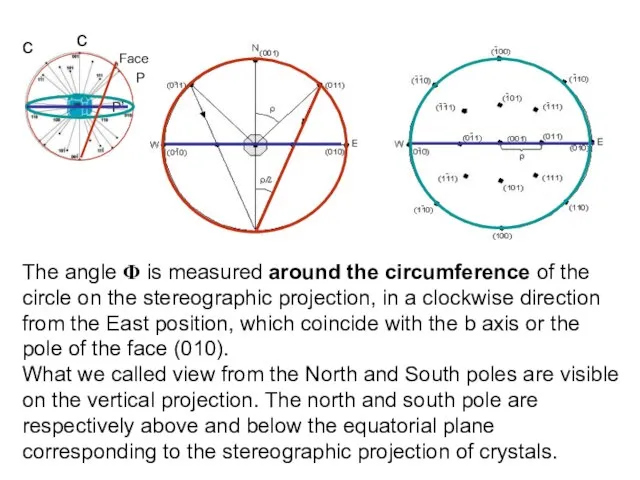
- 23. c The angle Φ is measured around the circumference of the circle on the stereographic projection,
- 24. Correspondance with the stereographic projection of the earth and that of tectonic planes
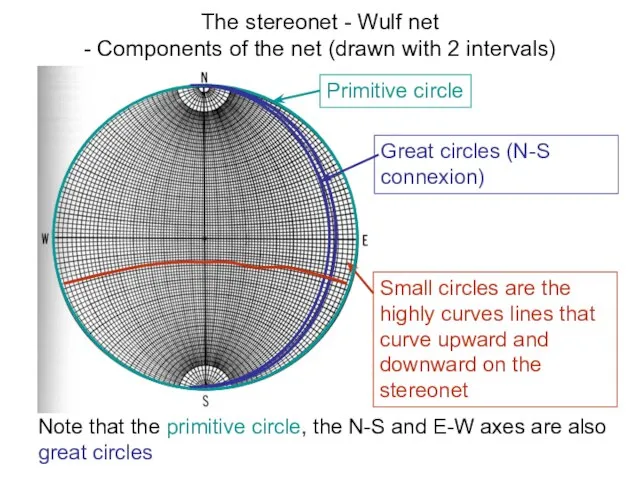
- 25. The stereonet - Wulf net - Components of the net (drawn with 2 intervals) Primitive circle
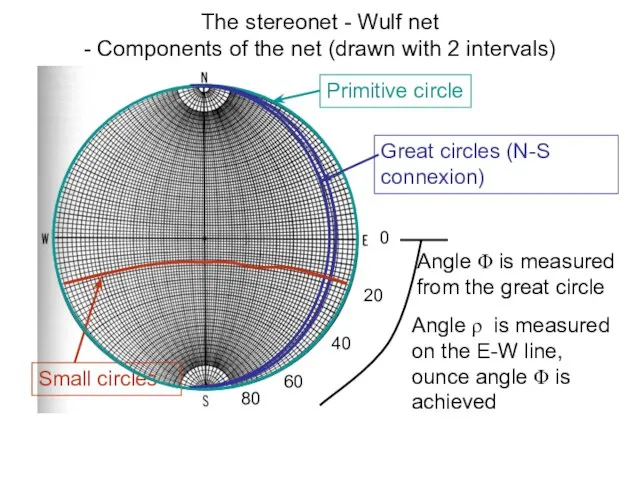
- 26. The stereonet - Wulf net - Components of the net (drawn with 2 intervals) Primitive circle
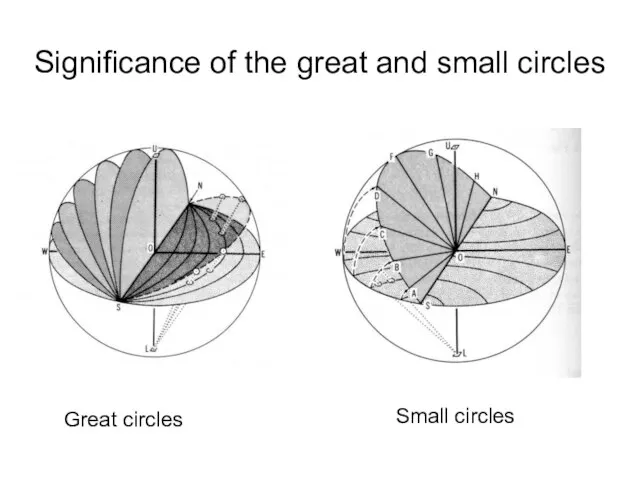
- 27. Significance of the great and small circles Great circles Small circles
- 28. Use of the stereonet - (1) All crystal faces are plotted as poles (lines perpendicular to
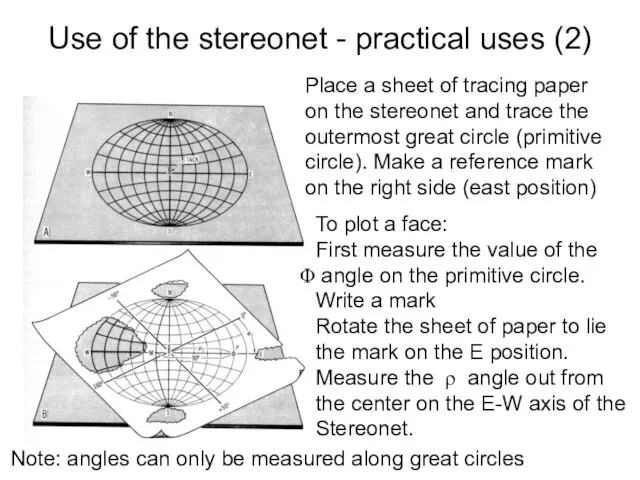
- 29. Use of the stereonet - practical uses (2) To plot a face: First measure the value
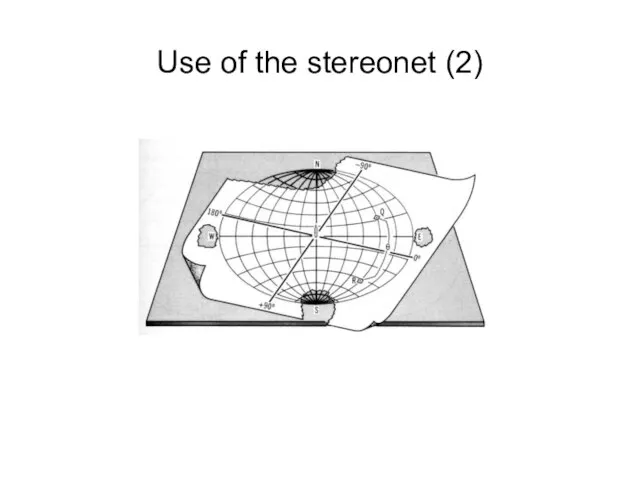
- 30. Use of the stereonet (2)
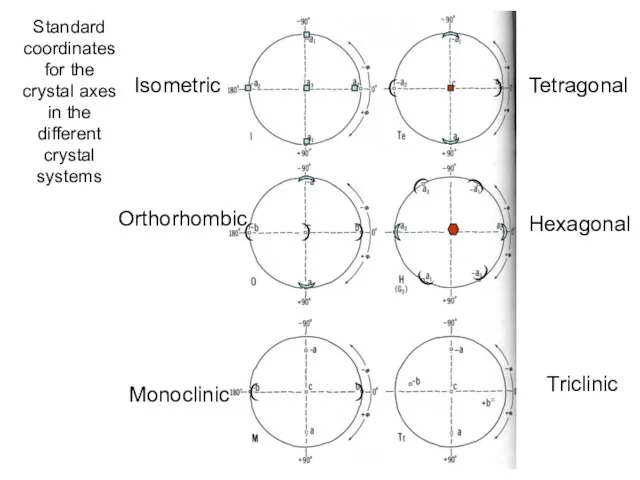
- 31. Standard coordinates for the crystal axes in the different crystal systems Isometric Tetragonal Triclinic Hexagonal Orthorhombic
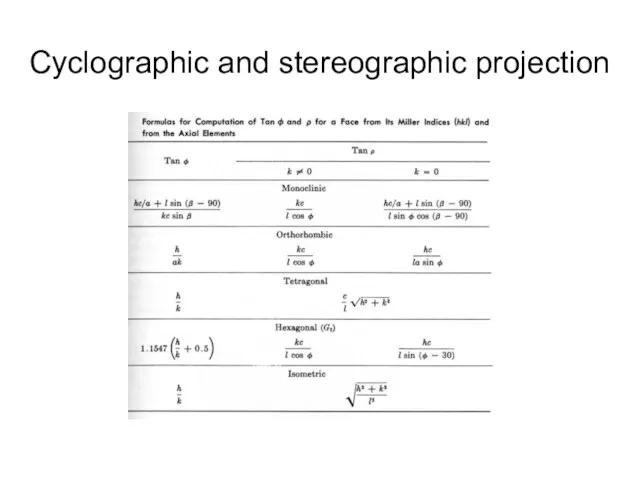
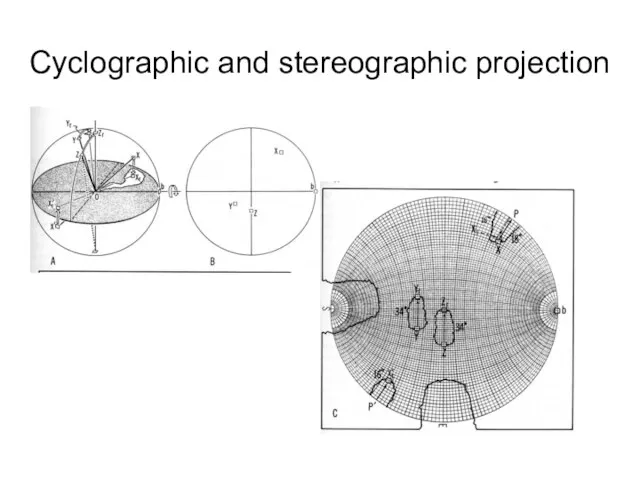
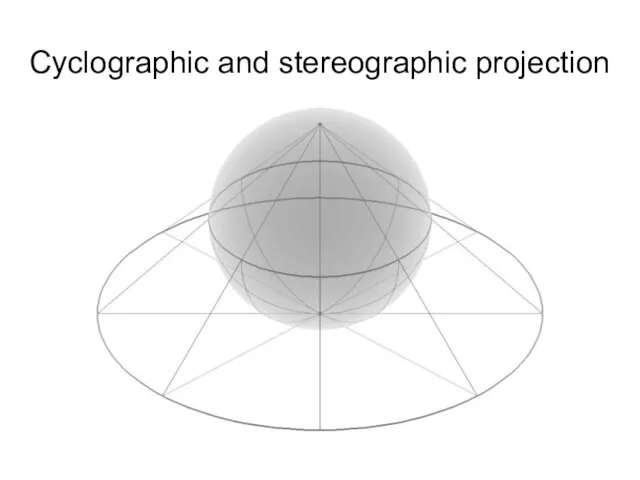
- 32. Cyclographic and stereographic projection
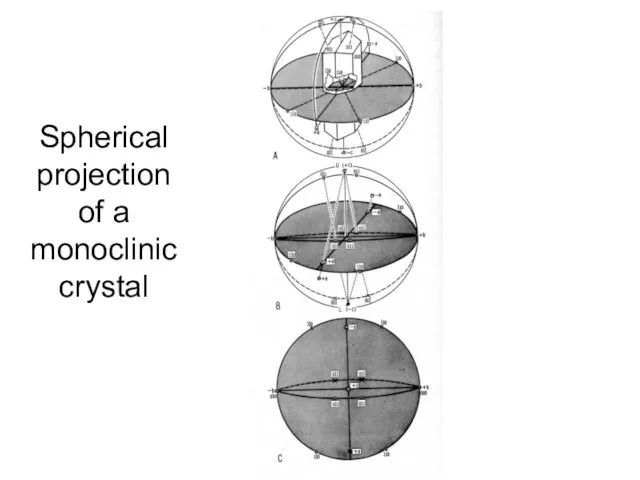
- 33. Spherical projection of a monoclinic crystal
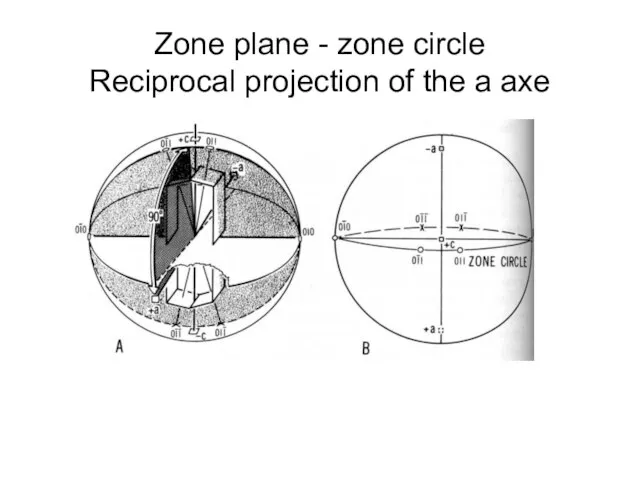
- 34. Zone plane - zone circle Reciprocal projection of the a axe
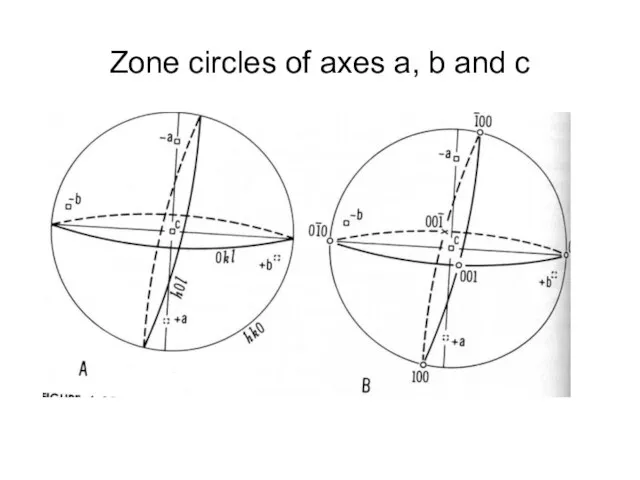
- 35. Zone circles of axes a, b and c
- 36. Cyclographic and stereographic projection
- 37. Cyclographic and stereographic projection
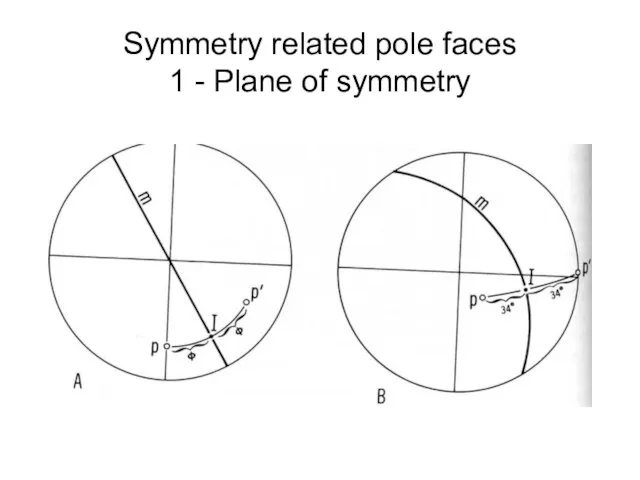
- 38. Symmetry related pole faces 1 - Plane of symmetry
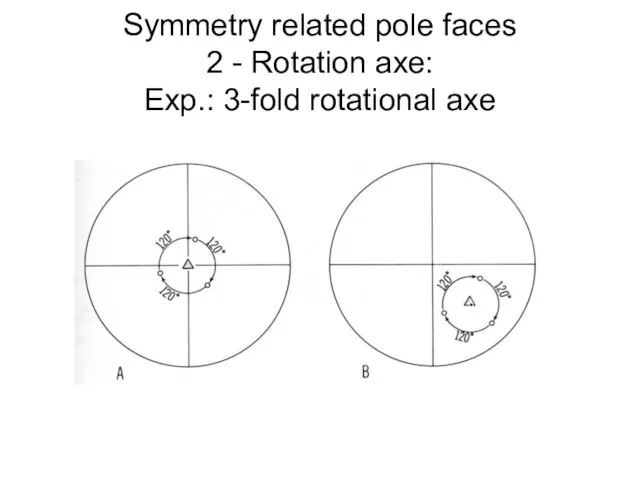
- 39. Symmetry related pole faces 2 - Rotation axe: Exp.: 3-fold rotational axe
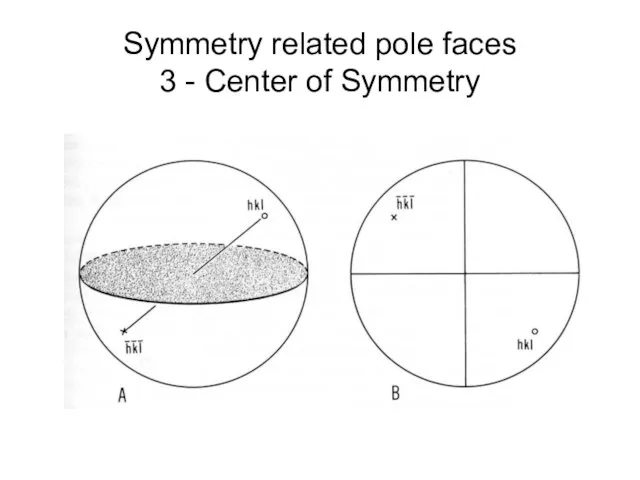
- 40. Symmetry related pole faces 3 - Center of Symmetry
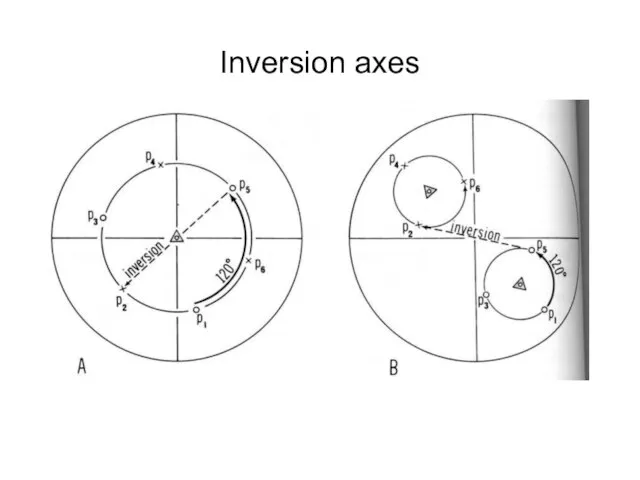
- 41. Inversion axes
- 42. Gnomonic projection
- 44. Скачать презентацию









![Stereographic projection of crystal faces [example face (011) of a cube]](/_ipx/f_webp&q_80&fit_contain&s_1440x1080/imagesDir/jpg/374064/slide-19.jpg)


 Георгии Карлович Вагнер
Георгии Карлович Вагнер Урок презентация Словарные слова в загадках
Урок презентация Словарные слова в загадках  Презентация на тему Портрет профессии (10 класс)
Презентация на тему Портрет профессии (10 класс) Каштановые и засоленные почвы
Каштановые и засоленные почвы Повышение эффективности процессов управления учетными записями в ОАО “МТС”
Повышение эффективности процессов управления учетными записями в ОАО “МТС” Презентация на тему Рождество Христово.
Презентация на тему Рождество Христово. Красив, богат и оригинален марийский народный костюм Каждый народ имеет свои особенности в материальной и духовной культуре
Красив, богат и оригинален марийский народный костюм Каждый народ имеет свои особенности в материальной и духовной культуре Презентация на тему Вильям Шекспир
Презентация на тему Вильям Шекспир  Зелёная Тара, Тибет, первая половина XX века
Зелёная Тара, Тибет, первая половина XX века Календарные праздники древних славян
Календарные праздники древних славян Я нацелен на успех
Я нацелен на успех ПРЕДВАРИТЕЛЬНАЯ ПРОГРАММА ПАРИЖСКОГО МОДУЛЯ «МОСКВА – ПАРИЖ» EXECUTIVE MBA LWB ГУУ – ESCP-EAP
ПРЕДВАРИТЕЛЬНАЯ ПРОГРАММА ПАРИЖСКОГО МОДУЛЯ «МОСКВА – ПАРИЖ» EXECUTIVE MBA LWB ГУУ – ESCP-EAP Классо Особенности композиции рассказа Л.Н.Толстого «После бала»
Классо Особенности композиции рассказа Л.Н.Толстого «После бала» Наши птицы - москвичи
Наши птицы - москвичи Управление системой здоровьесбережения в Златоустовском городском округе Начальник отдела С(К)О и здоровьесбережения МКУ Управл
Управление системой здоровьесбережения в Златоустовском городском округе Начальник отдела С(К)О и здоровьесбережения МКУ Управл НПП «Цифровые решения»
НПП «Цифровые решения» Элементы инфраструктуры рынка
Элементы инфраструктуры рынка Динамика объема рынка PR-услуг в России в 2009 году
Динамика объема рынка PR-услуг в России в 2009 году МБОУ методический центр в системе дополнительного педагогического образования (повышения квалификации)
МБОУ методический центр в системе дополнительного педагогического образования (повышения квалификации) История 1994 – Была создана и занималась продажей стройматериалов 1998 – Начали производство пены в Эстонии 2003 – Новые производствен
История 1994 – Была создана и занималась продажей стройматериалов 1998 – Начали производство пены в Эстонии 2003 – Новые производствен Соболева Татьяна Владимировна
Соболева Татьяна Владимировна Презентация на тему Деньги и их функции
Презентация на тему Деньги и их функции Индекс потребительских цен на продукты питания по Тюменской области (без автономных округов) в сравнении с РФ по данным статисти
Индекс потребительских цен на продукты питания по Тюменской области (без автономных округов) в сравнении с РФ по данным статисти Школа здоровья – повышение качества образования
Школа здоровья – повышение качества образования Хотите быть успешными?Говорите правильно!
Хотите быть успешными?Говорите правильно! Организационно-управленческое проектирование введения курса ОРКСЭ
Организационно-управленческое проектирование введения курса ОРКСЭ Последствия насилия
Последствия насилия International Market Research
International Market Research