Содержание
- 2. Independent and parallel visual processing of ensemble statistics: Evidence from dual tasks Vladislav Khvostov and Igor
- 3. An example
- 4. Greater or smaller than average?
- 5. Ensemble summary statistics The visual system can compute mean (Alvarez & Oliva, 2009), numerosity (Halberda, Sires,
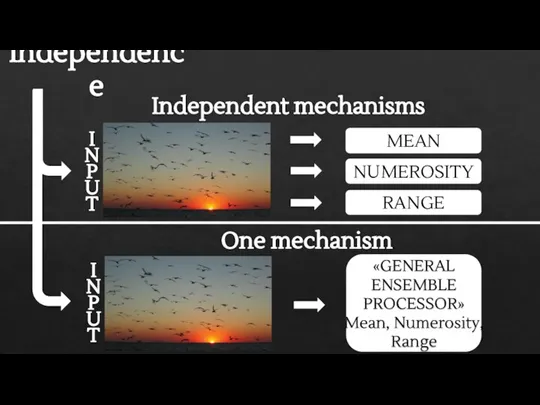
- 6. Independence
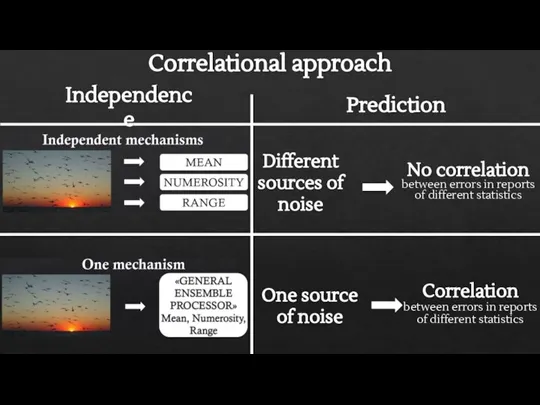
- 7. Correlational approach Prediction Independence
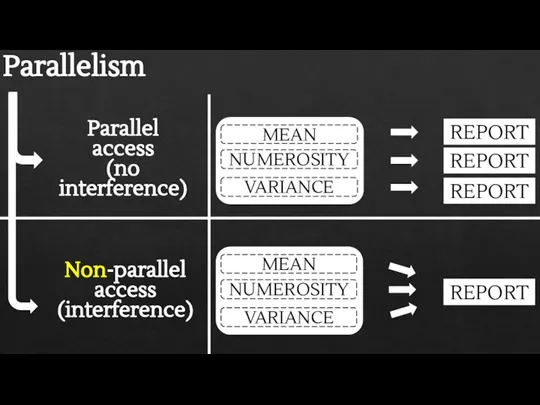
- 8. Parallelism Non-parallel access (interference)
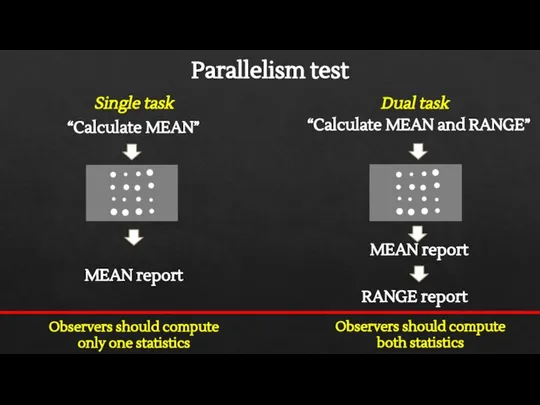
- 9. Parallelism test Single task “Calculate MEAN” MEAN report Dual task Observers should compute only one statistics
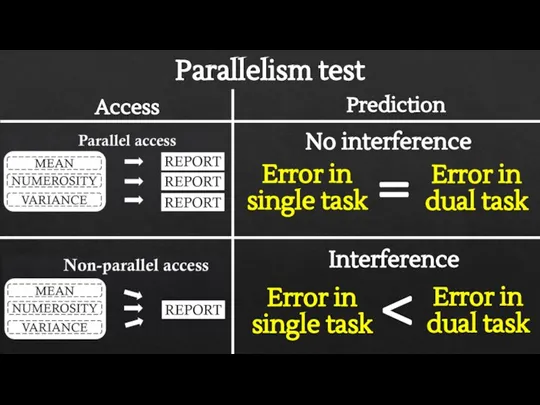
- 10. Parallelism test Prediction Access
- 11. Experiment 1 Whether mean and numerosity can be calculated independently and in parallel? N=23
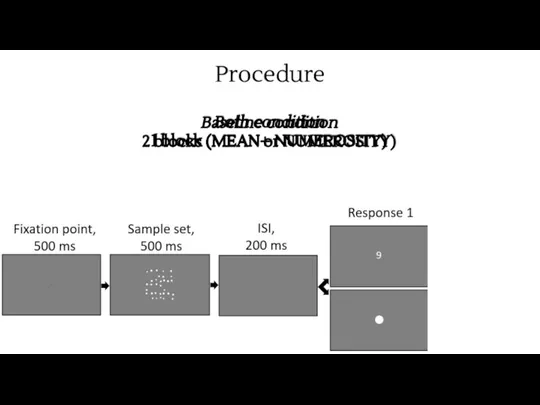
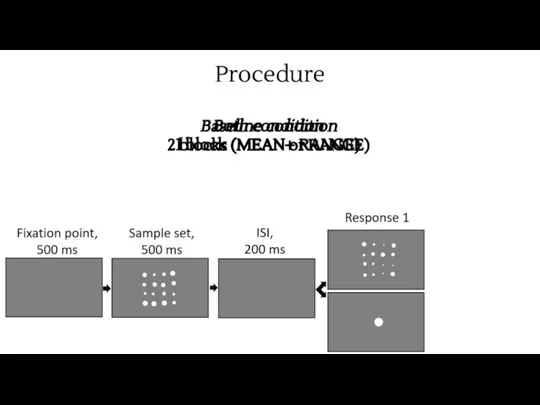
- 12. Procedure Baseline condition 2 blocks (MEAN or NUMEROSITY) Both condition 1 block (MEAN+ NUMEROSITY)
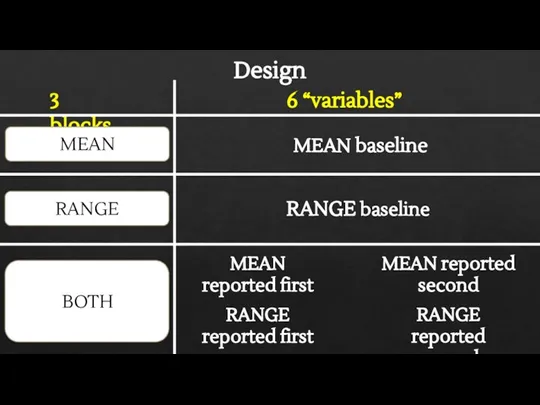
- 13. Design MEAN baseline 3 blocks MEAN NIMEROSITY BOTH 6 “variables” NIMEROSITY baseline MEAN reported first NIMEROSITY
- 14. Data analysis (1) Correlation between mean errors of 6 variables (across observers) (2) Trial-by-trial correlation between
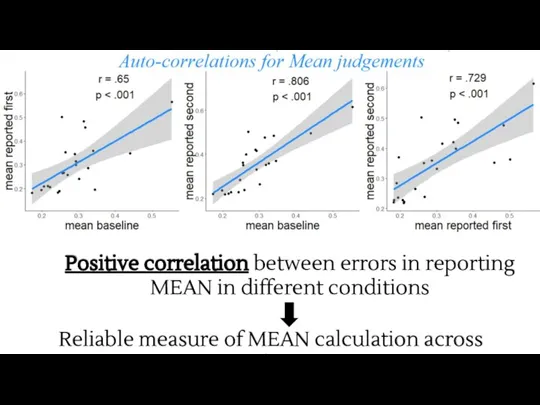
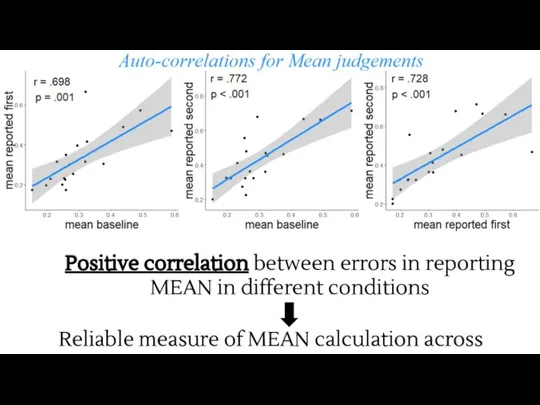
- 15. Positive correlation between errors in reporting MEAN in different conditions Reliable measure of MEAN calculation across
- 16. Positive correlation between errors in reporting NUMEROSITY in different conditions Reliable measure of NUMEROSITY calculation across
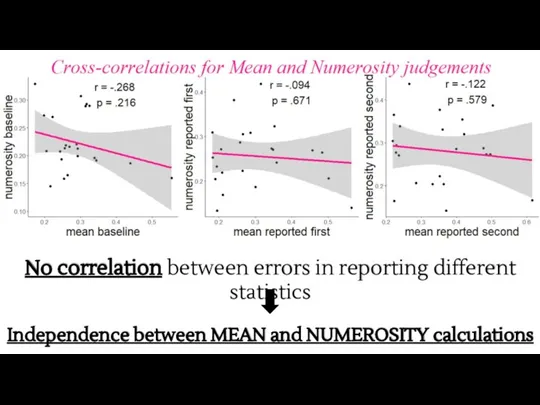
- 17. No correlation between errors in reporting different statistics Independence between MEAN and NUMEROSITY calculations
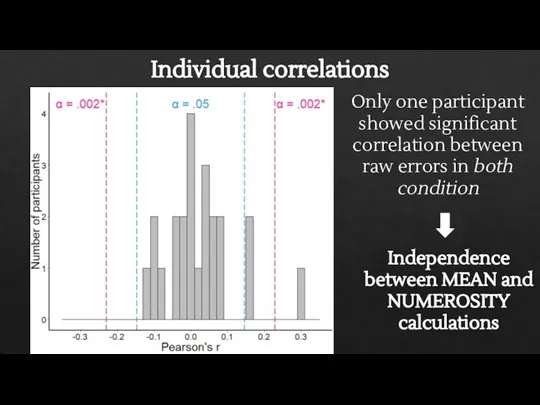
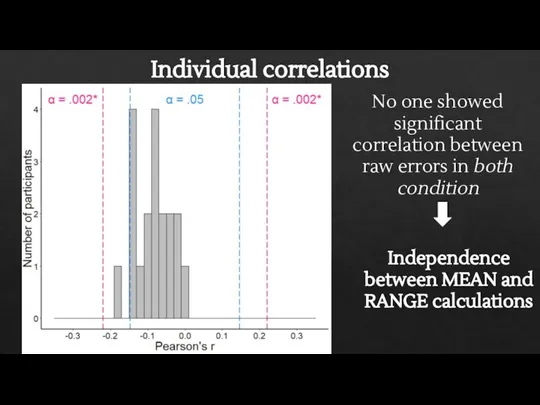
- 18. Individual correlations Only one participant showed significant correlation between raw errors in both condition Independence between
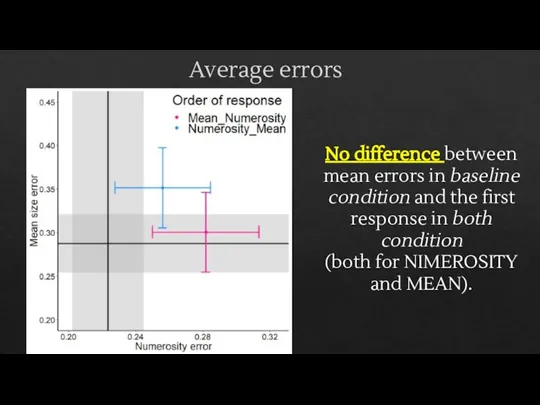
- 19. Average errors No difference between mean errors in baseline condition and the first response in both
- 20. Conclusion Mean and numerosity are calculated independently and in parallel
- 21. Experiment 2 Whether mean and range can be calculated independently and in parallel? N=20
- 22. Procedure Baseline condition 2 blocks (MEAN or RANGE) Both condition 1 block (MEAN+ RANGE)
- 23. Design MEAN baseline 3 blocks MEAN RANGE BOTH 6 “variables” RANGE baseline MEAN reported first RANGE
- 24. Positive correlation between errors in reporting MEAN in different conditions Reliable measure of MEAN calculation across
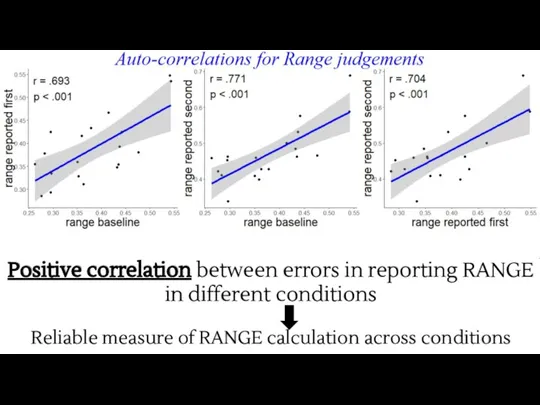
- 25. Positive correlation between errors in reporting RANGE in different conditions Reliable measure of RANGE calculation across
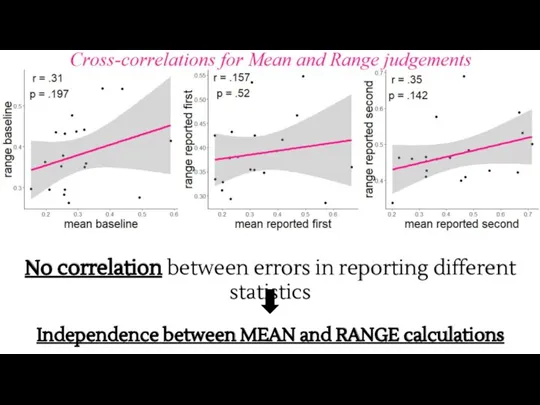
- 26. No correlation between errors in reporting different statistics Independence between MEAN and RANGE calculations
- 27. Individual correlations No one showed significant correlation between raw errors in both condition Independence between MEAN
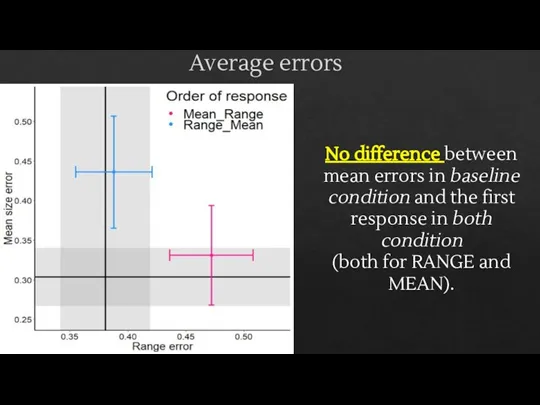
- 28. Average errors No difference between mean errors in baseline condition and the first response in both
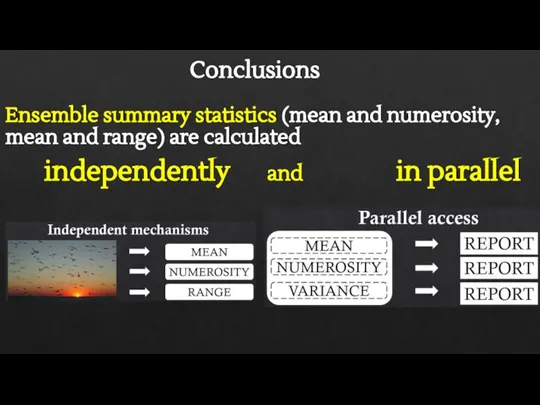
- 29. Conclusions Ensemble summary statistics (mean and numerosity, mean and range) are calculated independently and in parallel
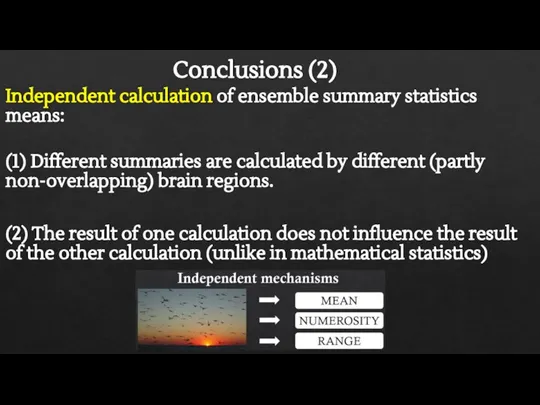
- 30. Conclusions (2) Independent calculation of ensemble summary statistics means: (1) Different summaries are calculated by different
- 31. For details and even one more experiment please read: Khvostov V.A., Utochkin I. S. Independent and
- 32. Thank you for being with me till the end of the first part
- 33. Confidence intervals in within-subject designs *Based on Cousineau, 2005 Part #2
- 34. It is all from this 4-pages paper
- 35. The problem Different subjects can perform very differently which increases a size of error bars Inconsistency
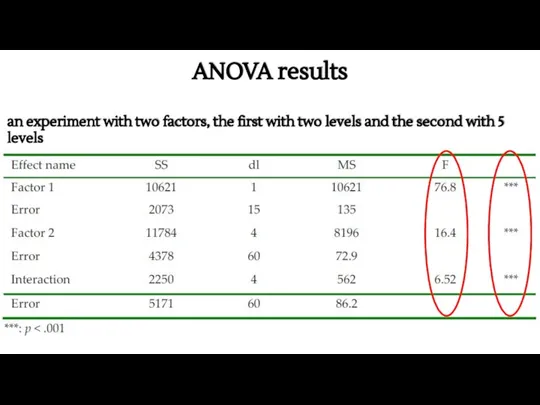
- 36. ANOVA results an experiment with two factors, the first with two levels and the second with
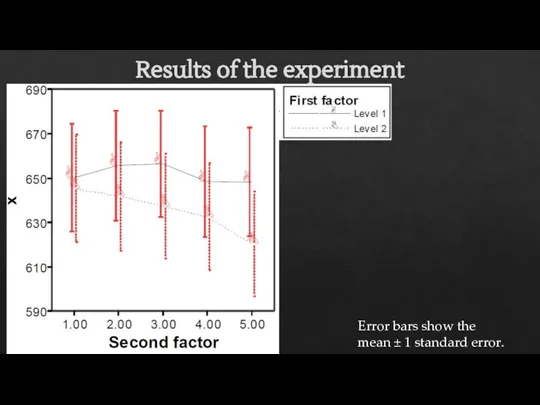
- 37. Results of the experiment Error bars show the mean ± 1 standard error.
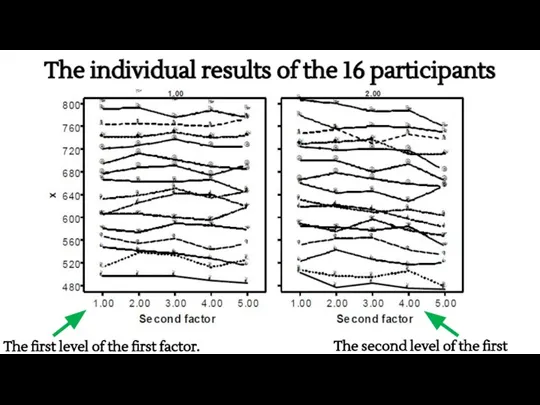
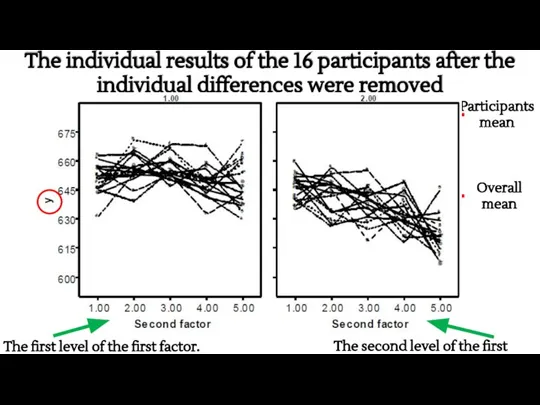
- 38. The individual results of the 16 participants The first level of the first factor. The second
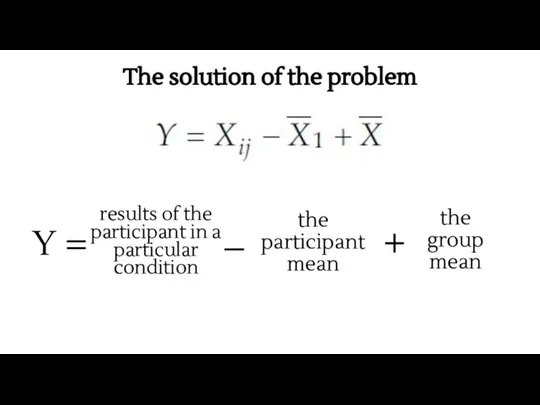
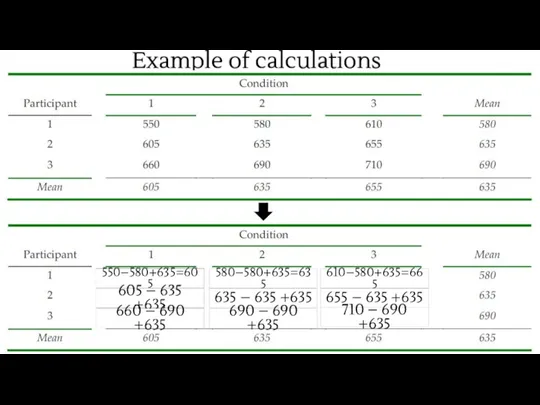
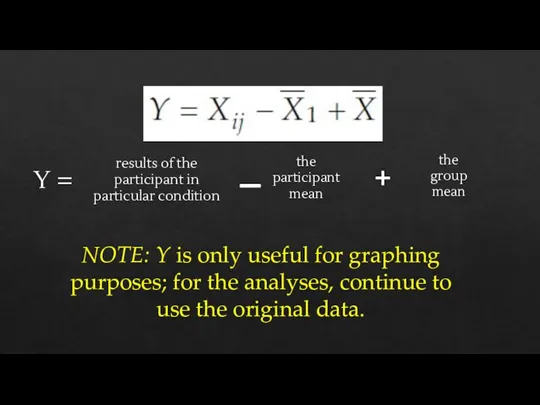
- 39. The solution of the problem the participant mean Y = _ + the group mean results
- 40. Example of calculations 550–580+635=605 580–580+635=635 610–580+635=665 605 – 635 +635 635 – 635 +635 655 –
- 41. The individual results of the 16 participants after the individual differences were removed The first level
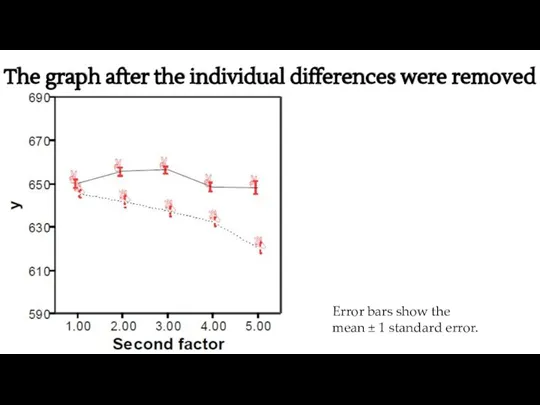
- 42. The graph after the individual differences were removed Error bars show the mean ± 1 standard
- 43. NOTE: Y is only useful for graphing purposes; for the analyses, continue to use the original
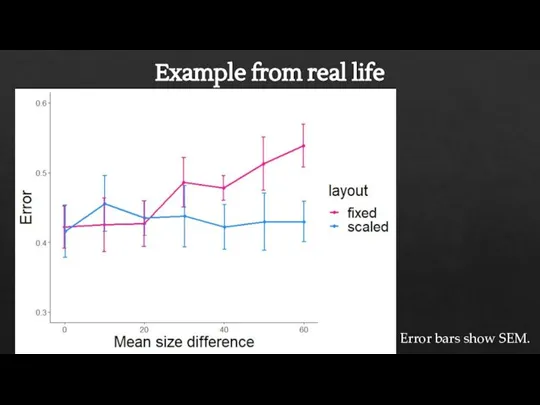
- 44. Example from real life Error bars show SEM.
- 45. Example from real life Error bars show SEM.
- 47. Скачать презентацию















 Порты ввода-вывода в компьютере
Порты ввода-вывода в компьютере Презентация на тему Графический редактор Paint
Презентация на тему Графический редактор Paint  Входной пакет заданий
Входной пакет заданий Формирование архива данных аэрологического зондирования
Формирование архива данных аэрологического зондирования Инструменты распознования текстов и компьютерного перевода
Инструменты распознования текстов и компьютерного перевода NVIDIA RSP (Real Sense Platform). Игровые технологии
NVIDIA RSP (Real Sense Platform). Игровые технологии Система СМИ в Португалии
Система СМИ в Португалии Макрос DragAndDrop
Макрос DragAndDrop Растровая и векторная графика
Растровая и векторная графика Базовые структуры алгоритмов (продолжение)
Базовые структуры алгоритмов (продолжение) Freelance. Звіт з курсового проекту
Freelance. Звіт з курсового проекту Роль материаловедения в графическом дизайне
Роль материаловедения в графическом дизайне Установка сносок
Установка сносок Initial SET-UP
Initial SET-UP Язык программирования Python. Операторы ветвления в языке Python
Язык программирования Python. Операторы ветвления в языке Python Знакомство с нейросетями и немного магии на JavaScript
Знакомство с нейросетями и немного магии на JavaScript Презентация на тему Виды моделей баз данных
Презентация на тему Виды моделей баз данных  Рисуем супер-узоры
Рисуем супер-узоры Системы счисления. Способы представления чисел
Системы счисления. Способы представления чисел КУРС Базовые приемы программирования. 2022. Онлайн - Лекция 2. Выполнение ЛР № 1. Списки.(2 поток)
КУРС Базовые приемы программирования. 2022. Онлайн - Лекция 2. Выполнение ЛР № 1. Списки.(2 поток) Системные объекты. Системотехника
Системные объекты. Системотехника Сетка / пропорции
Сетка / пропорции Начало. Конец
Начало. Конец Мир 3Д. Моделирование брелка
Мир 3Д. Моделирование брелка Громкоговоритель в школе
Громкоговоритель в школе Черепаха-графический учебный исполнитель
Черепаха-графический учебный исполнитель Если у вас мало времени и много вычислений… … поможет Excel
Если у вас мало времени и много вычислений… … поможет Excel Дорогое завтра! Литературный конкурс, посвященный технологиям и развитию искусственного интеллекта
Дорогое завтра! Литературный конкурс, посвященный технологиям и развитию искусственного интеллекта