Содержание
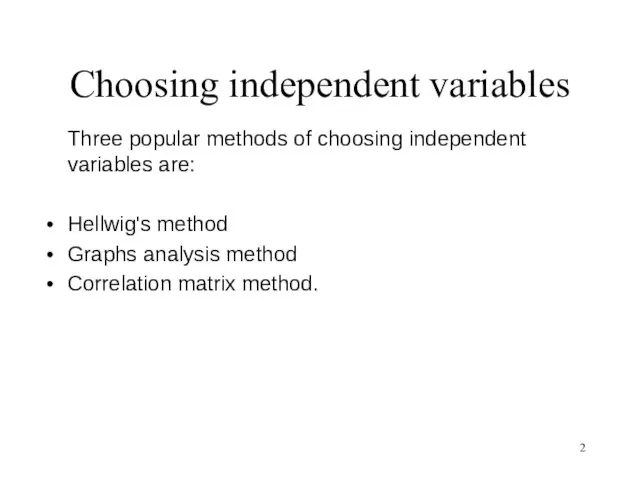
- 2. Choosing independent variables Three popular methods of choosing independent variables are: Hellwig's method Graphs analysis method
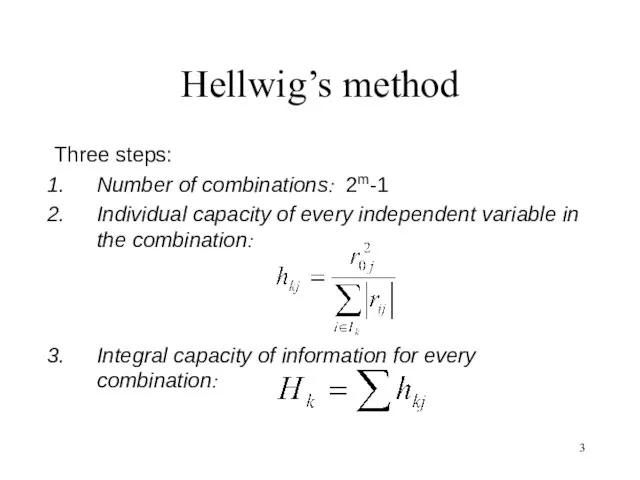
- 3. Hellwig’s method Three steps: Number of combinations: 2m-1 Individual capacity of every independent variable in the
- 4. Hellwig’s method 1. Number of combinations In Hellwig’s method the number of combinations is provided by
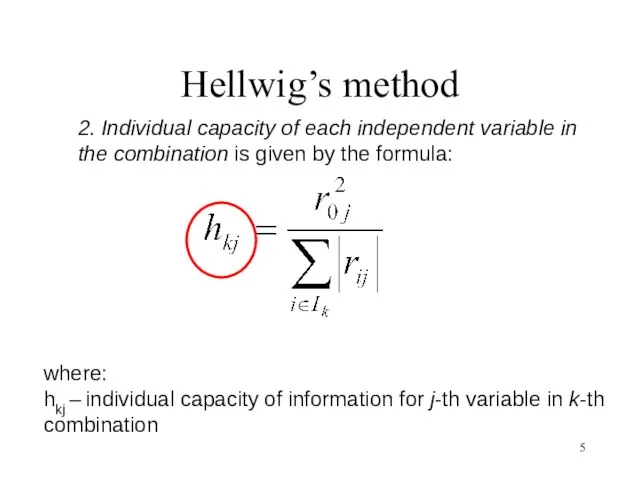
- 5. Hellwig’s method 2. Individual capacity of each independent variable in the combination is given by the
- 6. Hellwig’s method 2. Individual capacity of each independent variable in the combination is given by the
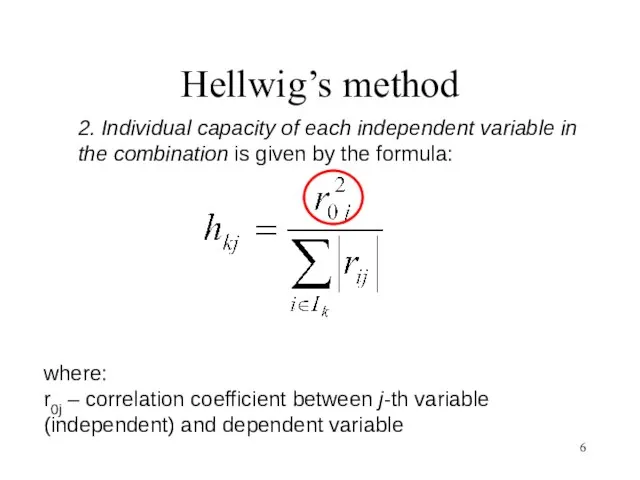
- 7. Hellwig’s method 2. Individual capacity of each independent variable in the combination is given by the
- 8. Hellwig’s method 2. Individual capacity of each independent variable in the combination is given by the
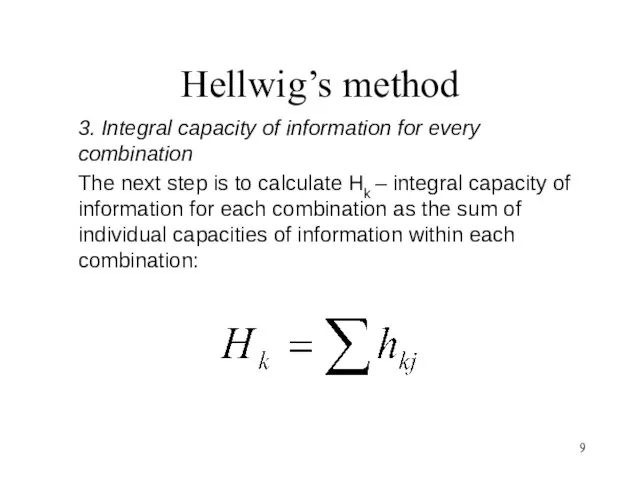
- 9. Hellwig’s method 3. Integral capacity of information for every combination The next step is to calculate
- 10. Hellwig’s method Q: HOW TO CHOOSE INDEPENDENT VARIABLES? A: LOOK AT INTEGRAL CAPACITIES OF INFORMATION. THE
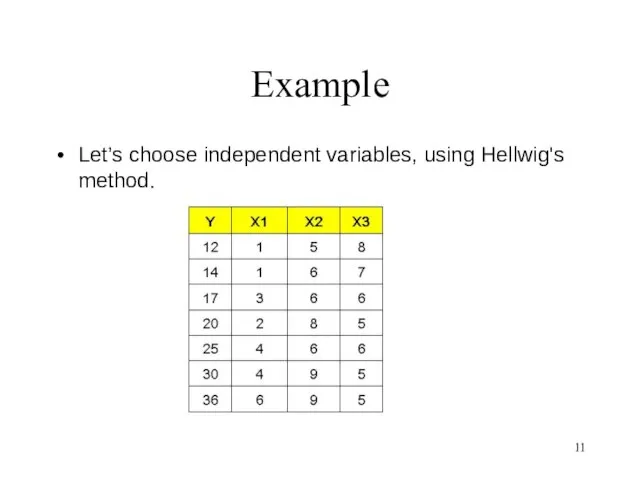
- 11. Example Let’s choose independent variables, using Hellwig's method.
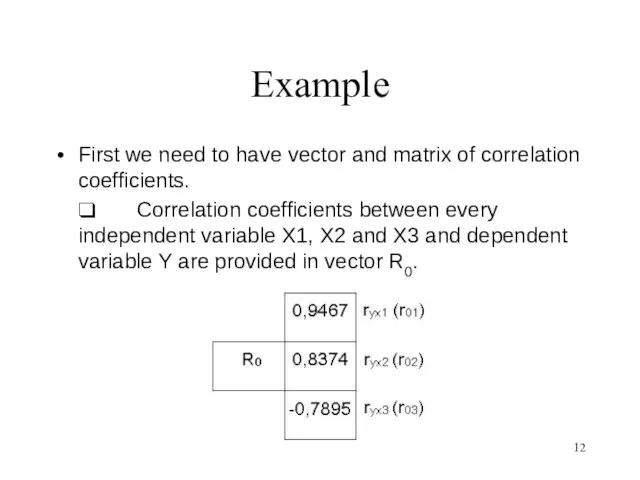
- 12. Example First we need to have vector and matrix of correlation coefficients. ❑ Correlation coefficients between
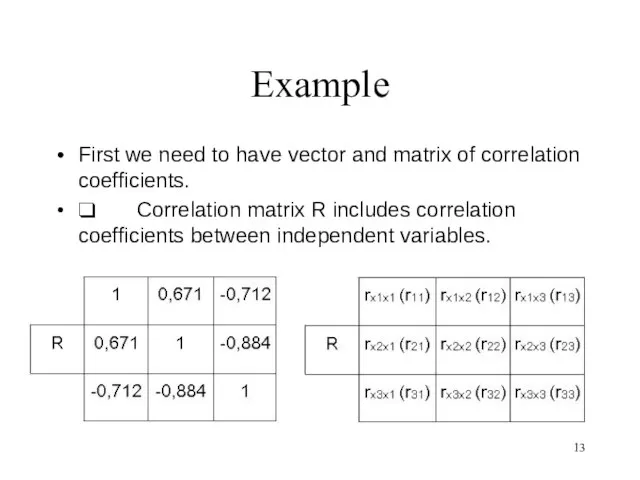
- 13. Example First we need to have vector and matrix of correlation coefficients. ❑ Correlation matrix R
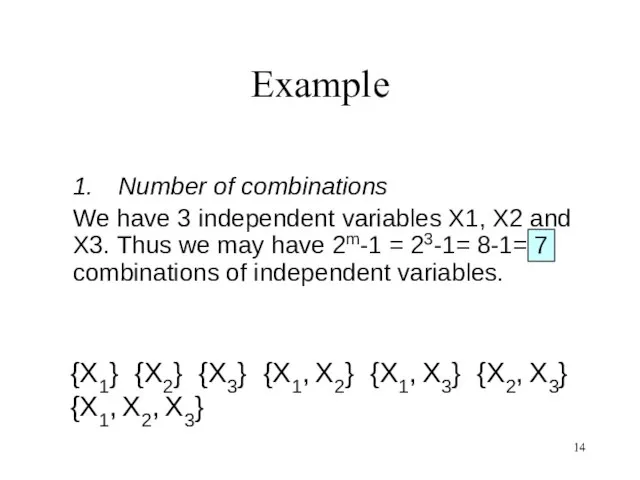
- 14. Example 1. Number of combinations We have 3 independent variables X1, X2 and X3. Thus we
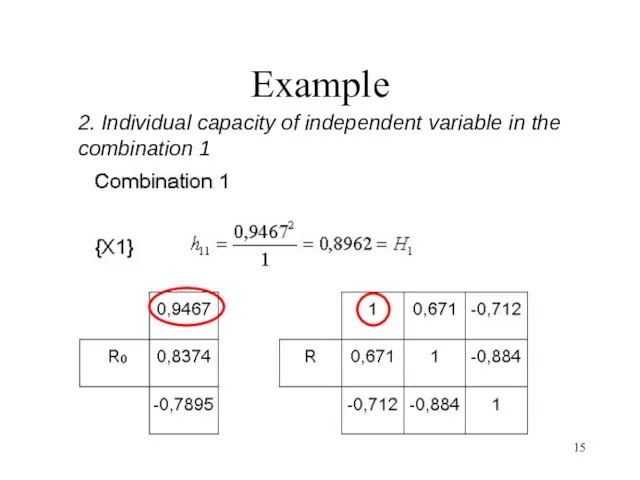
- 15. Example 2. Individual capacity of independent variable in the combination 1
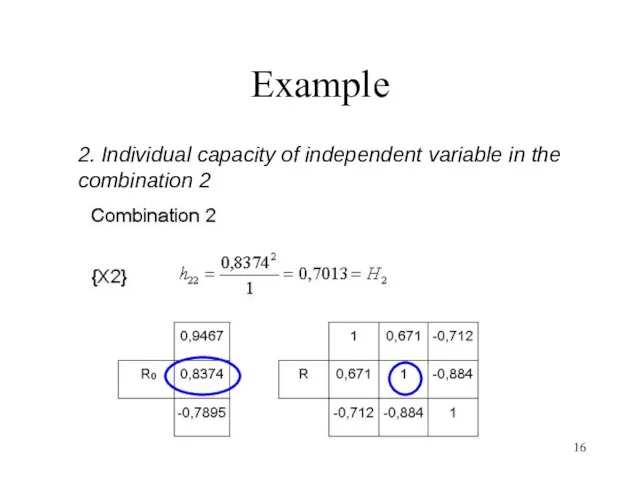
- 16. Example 2. Individual capacity of independent variable in the combination 2
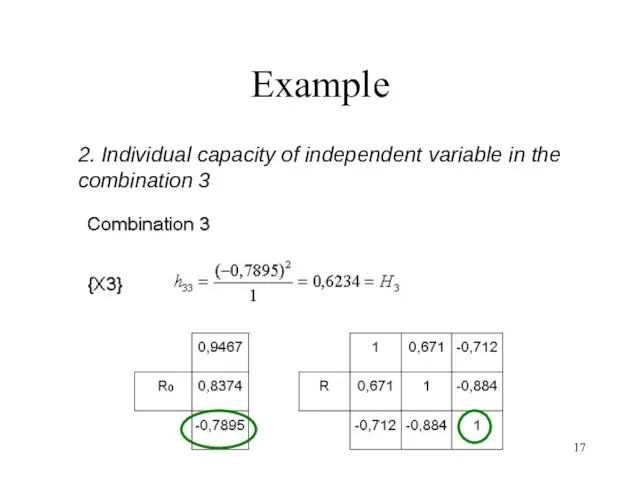
- 17. Example 2. Individual capacity of independent variable in the combination 3
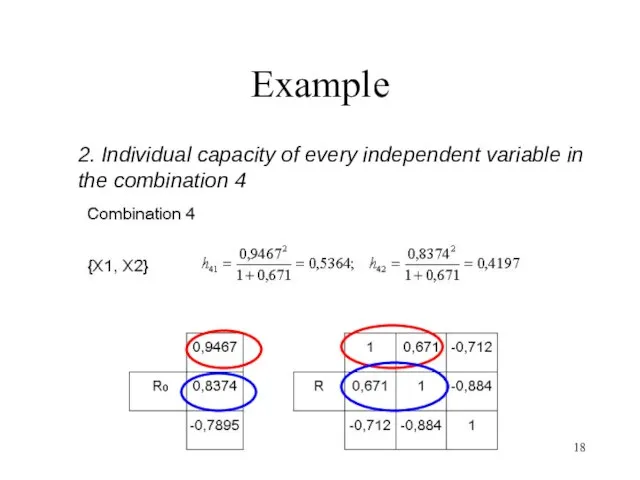
- 18. Example 2. Individual capacity of every independent variable in the combination 4
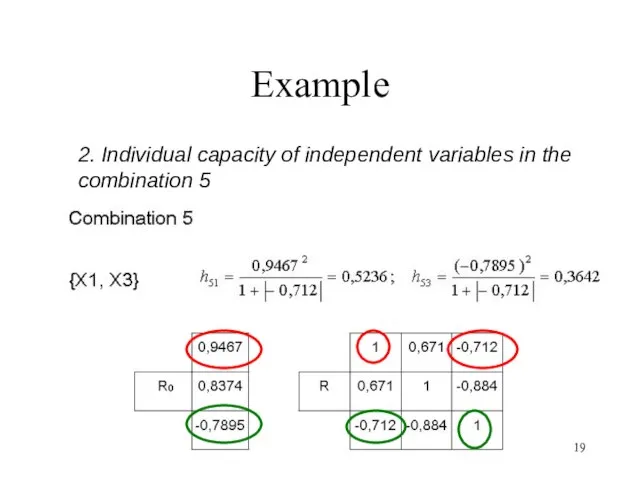
- 19. Example 2. Individual capacity of independent variables in the combination 5
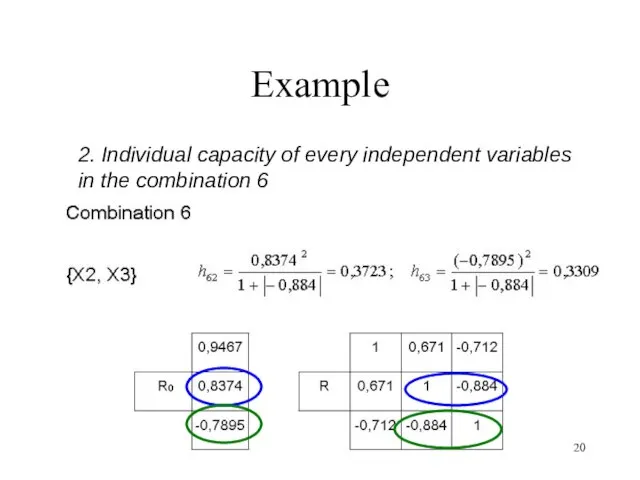
- 20. Example 2. Individual capacity of every independent variables in the combination 6
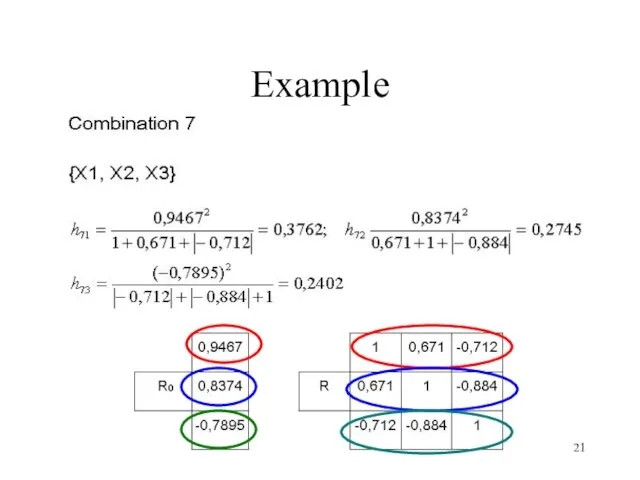
- 21. Example
- 22. Example 3. Integral capacity of information for each combination The greatest integral capacity is for combination
- 23. Graph analysis method Three steps Calculating r* Modification of correlation matrix Drawing the graph
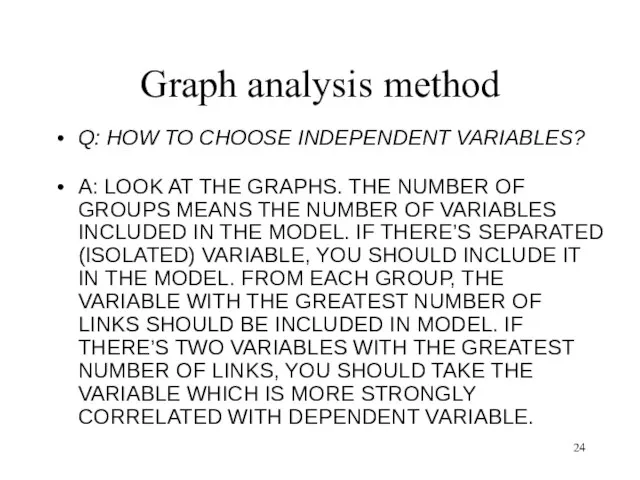
- 24. Graph analysis method Q: HOW TO CHOOSE INDEPENDENT VARIABLES? A: LOOK AT THE GRAPHS. THE NUMBER
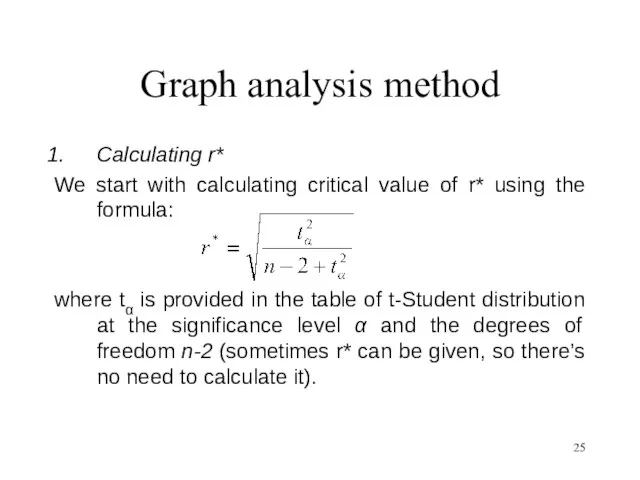
- 25. Graph analysis method Calculating r* We start with calculating critical value of r* using the formula:
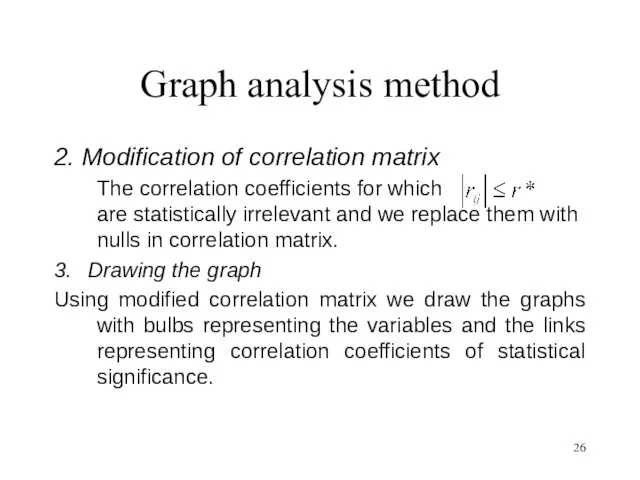
- 26. Graph analysis method 2. Modification of correlation matrix The correlation coefficients for which are statistically irrelevant
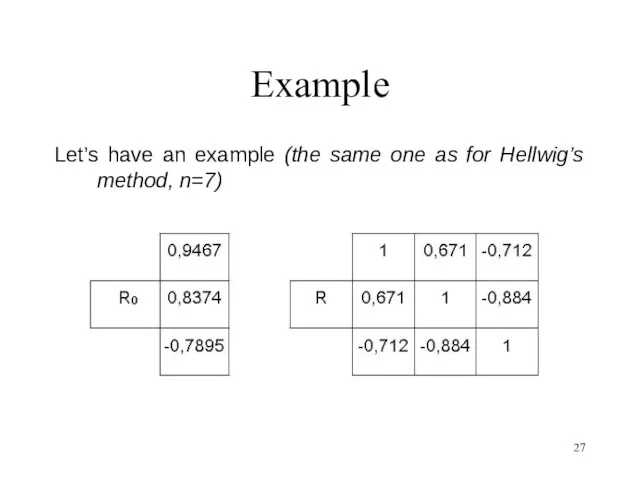
- 27. Example Let’s have an example (the same one as for Hellwig’s method, n=7)
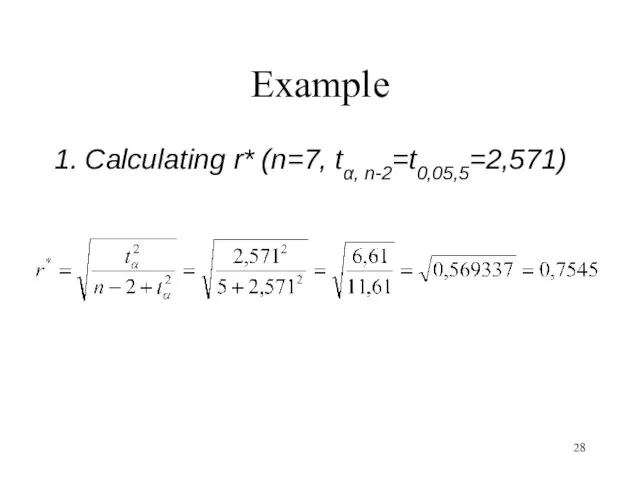
- 28. Example 1. Calculating r* (n=7, tα, n-2=t0,05,5=2,571)
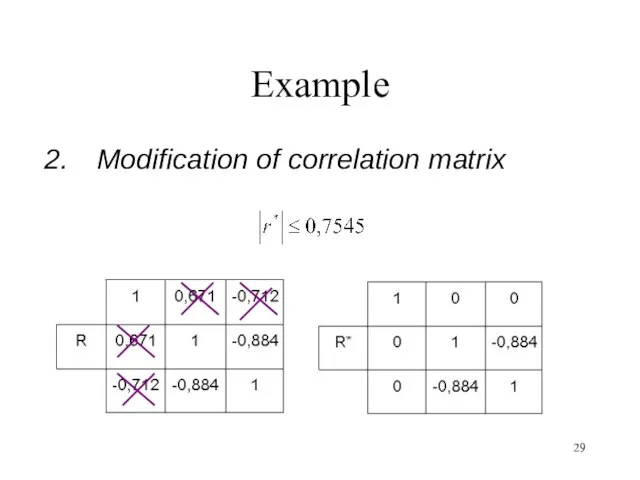
- 29. Example Modification of correlation matrix
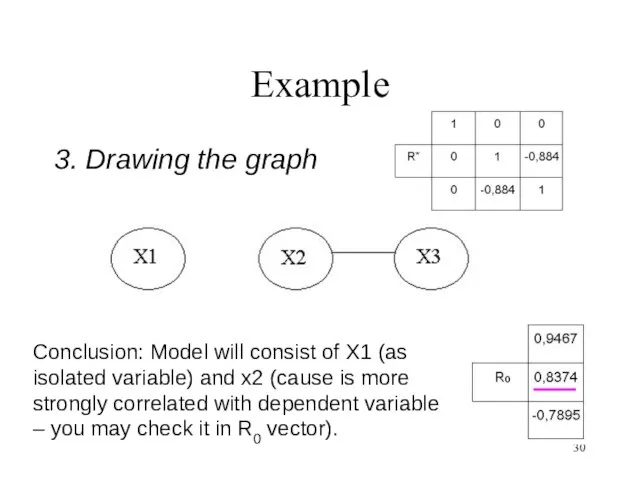
- 30. Example 3. Drawing the graph Conclusion: Model will consist of X1 (as isolated variable) and x2
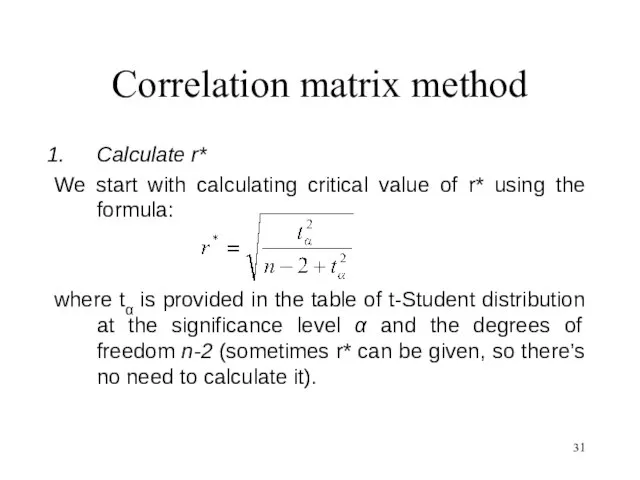
- 31. Correlation matrix method Calculate r* We start with calculating critical value of r* using the formula:
- 32. 2. To eliminate Xi variables weakly correlated withY 3. To choose Xs where [Xs is the
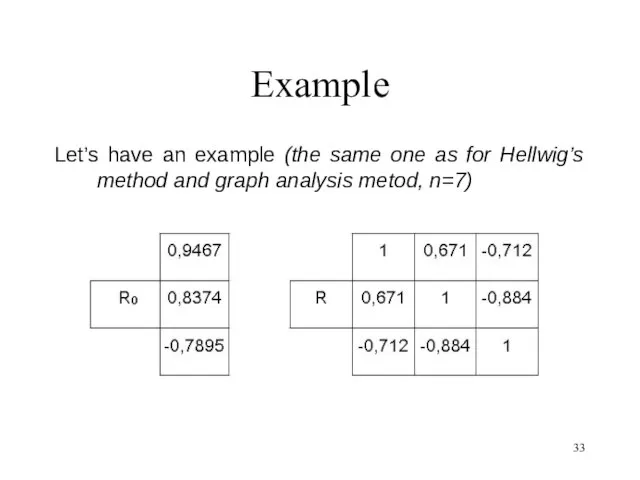
- 33. Example Let’s have an example (the same one as for Hellwig’s method and graph analysis metod,
- 34. Example 1. Calculating r* (n=7, tα, n-2=t0,05,5=2,571)
- 35. 2. To eliminate Xi variables weakly correlated withY None of the variables will be eliminated
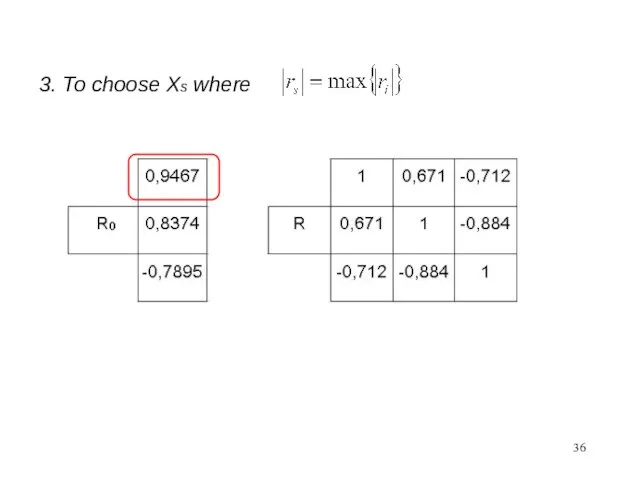
- 36. 3. To choose Xs where
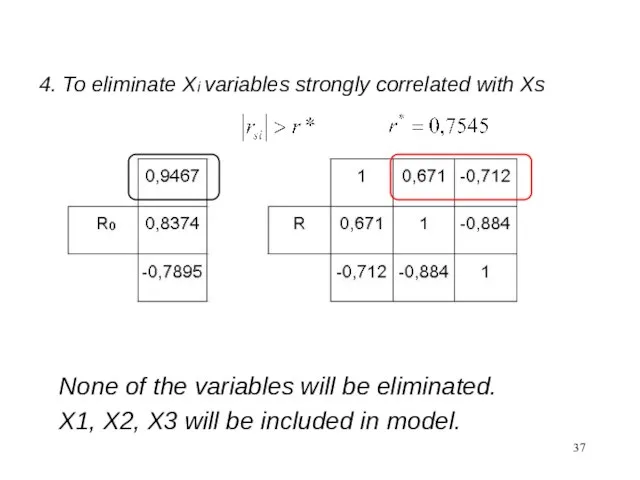
- 37. 4. To eliminate Xi variables strongly correlated with Xs None of the variables will be eliminated.
- 39. Скачать презентацию






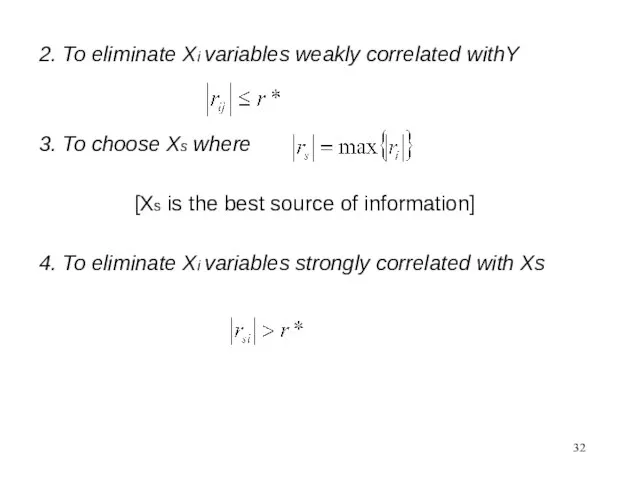


 ДЕНЬ НАРОДНОГО ЕДИНСТВА
ДЕНЬ НАРОДНОГО ЕДИНСТВА Медико-гуманитарный факультет
Медико-гуманитарный факультет Монополия в рыночной экономике
Монополия в рыночной экономике МОУ Ясашно-Ташлинская СОШКонкурс Номинация:«Социальная реклама»«Русский язык-язык межнационального общения в России». Подгот
МОУ Ясашно-Ташлинская СОШКонкурс Номинация:«Социальная реклама»«Русский язык-язык межнационального общения в России». Подгот Приключения, детективы, фэнтази для девочек
Приключения, детективы, фэнтази для девочек Премьера Вдохновляя на жизнь
Премьера Вдохновляя на жизнь Занимательные задачи (5 класс)
Занимательные задачи (5 класс) Юридические аспекты города-курорта Сочи как исторического поселения
Юридические аспекты города-курорта Сочи как исторического поселения Методические рекомендации по работе с автоматизированной информационно-библиотечной системой «Mark-SQL. Версия для школьных библиот
Методические рекомендации по работе с автоматизированной информационно-библиотечной системой «Mark-SQL. Версия для школьных библиот Общая структура персонального Общая структура персонального компьютера компьютера Системная магистраль (шина) Основная память М
Общая структура персонального Общая структура персонального компьютера компьютера Системная магистраль (шина) Основная память М Примечания и оговорки в нормативно-правовых актах
Примечания и оговорки в нормативно-правовых актах Политическое участие и политические технологии. Лекция 4
Политическое участие и политические технологии. Лекция 4 Что же делать ?
Что же делать ? Кольори. Послідовність кольорів
Кольори. Послідовність кольорів График квадратичной функции Занятие в 9 классе Продолжительность 40 минут Учитель математики Лещенко Любовь Владимировна в
График квадратичной функции Занятие в 9 классе Продолжительность 40 минут Учитель математики Лещенко Любовь Владимировна в Отчет о прохождении практики в компании Nord Haus
Отчет о прохождении практики в компании Nord Haus Healthy Food
Healthy Food Urok_27_03_Maria
Urok_27_03_Maria Электромагнитные волны
Электромагнитные волны  Технологии компьютерного моделированияЛекционная часть курса (все теор. темы кроме ООМ)
Технологии компьютерного моделированияЛекционная часть курса (все теор. темы кроме ООМ) Художественная фотография как вид искусства
Художественная фотография как вид искусства Система образованияв Японии и Кыргызстане
Система образованияв Японии и Кыргызстане Немой кинематограф. Вклад Александра Петровича Довженко
Немой кинематограф. Вклад Александра Петровича Довженко Решение задач финансового содержания
Решение задач финансового содержания Презентация на тему Производство и передача электроэнергии
Презентация на тему Производство и передача электроэнергии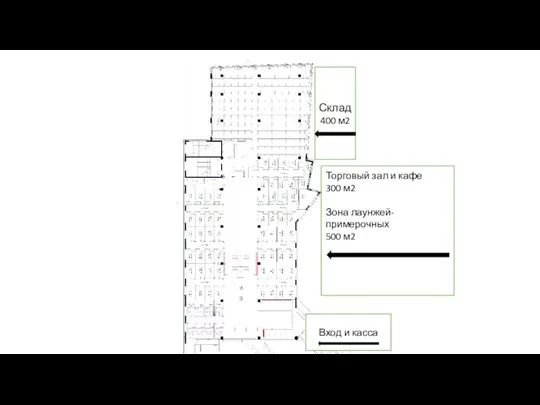 Схема супермаркета
Схема супермаркета DP maintenance course
DP maintenance course TNS Media Research Marketing Index Project
TNS Media Research Marketing Index Project