Содержание
- 2. Key Points Understanding The Timing of Returns Cash Flows Risk and Return Time Value of Money
- 3. Introduction You may have learned from your studies of economics that the goal of the business
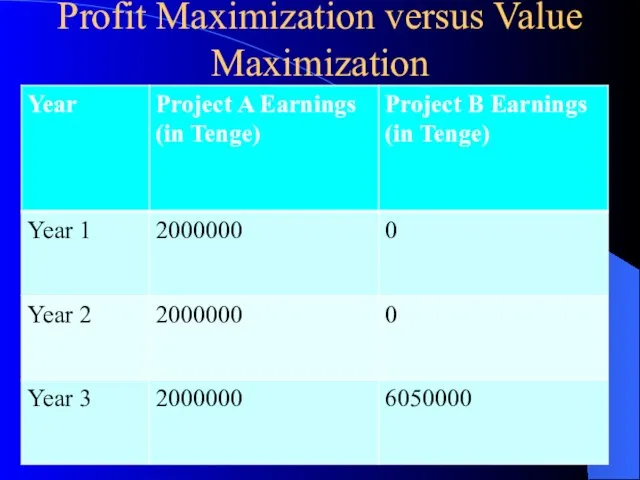
- 4. Profit Maximization versus Value Maximization
- 5. Profit Maximizing Versus Value Maximizing Profit maximization would dictate the choice of Project B, as it
- 6. Cash Flows Profits do not represent cash flows. Although profits may be used as the traditional
- 7. Risk The objective of profit maximization also ignores the concept of risk, which, for our purposes
- 8. Risk Clearly Project B, in this scenario, would be the higher risk investment and therefore its
- 9. Core Concepts of Financial Management In making financial decisions, the financial manager will be guided and
- 10. Core Concepts of FM We can now proceed to examine the core concepts of financial management,
- 11. Cash Flow ‘Cash generation is the foundation of creative value for shareholders’ (J Sainsbury Plc, Annual
- 12. Profit versus Cash Flow Profit is an accounting concept whereas cash is a financial management concept,
- 13. Profit versus Cash Flow Wild Rover Joinery is a small company which designs and manufactures interior
- 14. Profit versus Cash Flow Accounting perspective-If the costs figure includes the costs of manufacture (wages, materials,
- 15. Measuring Cash Flow A firm’s net cash flow is USUALLY calculated by deducting cash payments from
- 16. Measuring Cash Flow However, the important point is that depreciation is not a cash expense. For
- 17. Measuring Cash Flow This measurement of cash flow shows the discretionary cash remaining after making the
- 18. Measuring Cash Flow However it is measured or defined, cash flow generation is the true test
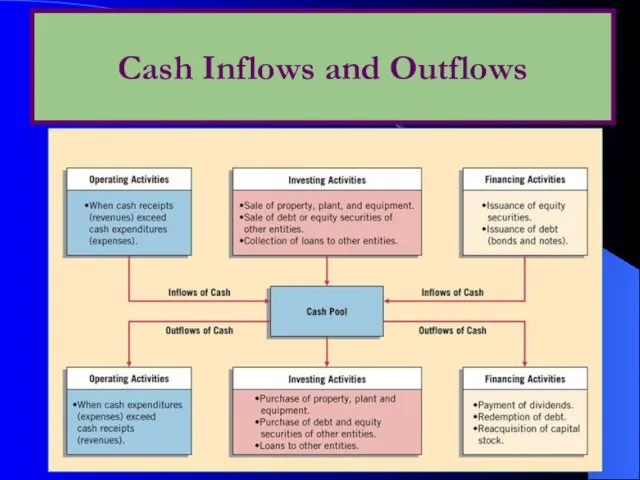
- 19. Cash Inflows and Outflows
- 20. Risk and Return The motivation for undertaking an investment decision is the expectation of gaining a
- 21. Risk and Return In the context of financial management by risk we mean the chance that
- 22. Risk and Return This allows the decision-maker to create probability weightings for a range of possible
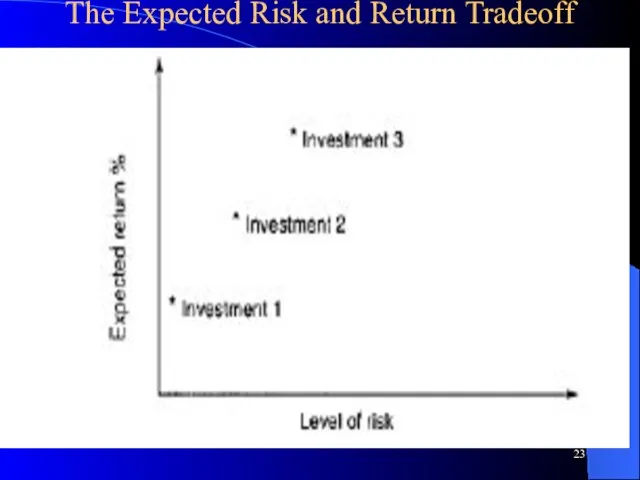
- 23. The Expected Risk and Return Tradeoff
- 24. Risk and Return The risk of investing in a bank or building society savings account (Investment
- 25. The investor’s propensity for risk-taking Figure shows, other things being equal, the positive relationship (correlation) between
- 26. The investor’s propensity for risk-taking Individuals and corporations are generally considered to be risk-averse, in the
- 27. Time Value of Money Cash flows which are to be received at some time in the
- 28. Time Value of Money Not only is the concept of measuring returns in terms of cash
- 29. The Time Value of Money Obviously, $1,000 today. Money received sooner rather than later allows one
- 30. INFLATION You may have wondered about the role inflation plays in the time value of money.
- 31. Why TIME? Observe that the concepts of future value and present value are inversely related. Over
- 32. How can one compare amounts in different time periods? One can adjust values from different time
- 33. Compound Interest When interest is paid on not only the principal amount invested, but also on
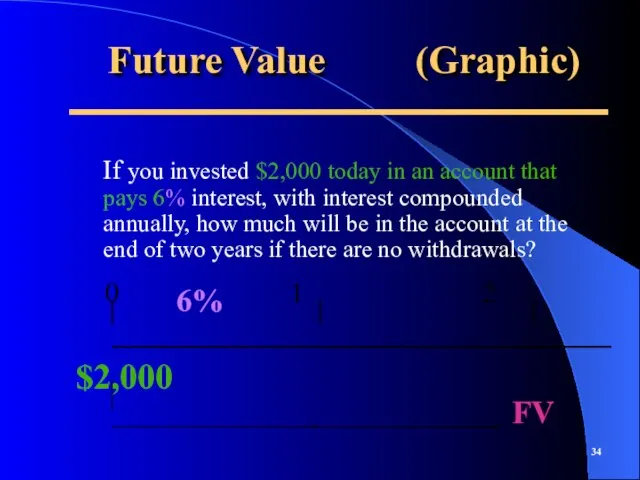
- 34. Future Value (Graphic) If you invested $2,000 today in an account that pays 6% interest, with
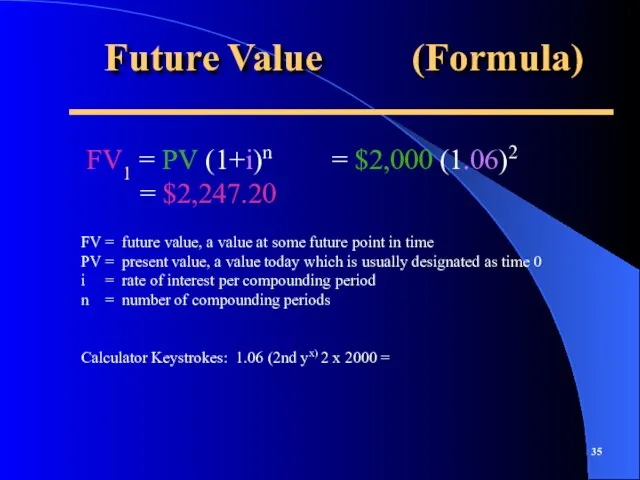
- 35. Future Value (Formula) FV1 = PV (1+i)n = $2,000 (1.06)2 = $2,247.20 FV = future value,
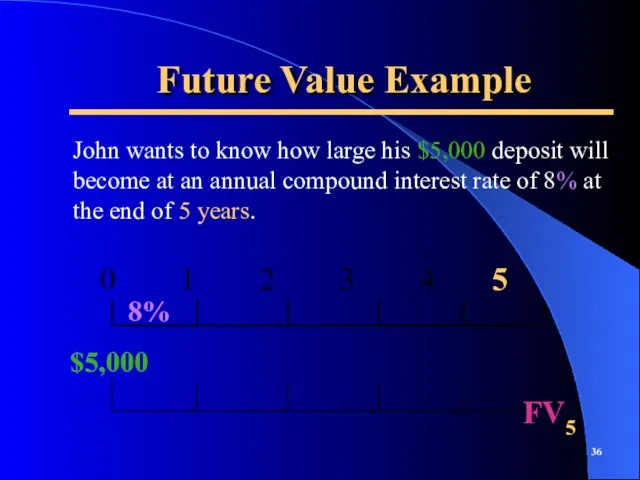
- 36. Future Value Example John wants to know how large his $5,000 deposit will become at an
- 37. Future Value Solution Calculator keystrokes: 1.08 2nd yx x 5000 = Calculation based on general formula:
- 38. Double Your Money!!! Quick! How long does it take to double $2,000 at a compound rate
- 39. The “Rule-of-72” Quick! How long does it take to double $2,000 at a compound rate of
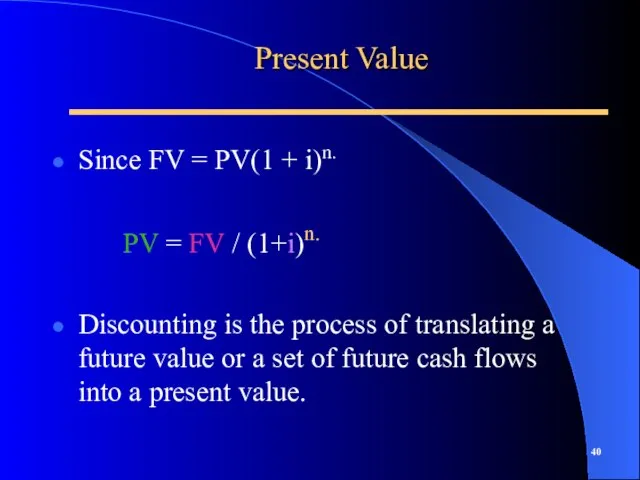
- 40. Present Value Since FV = PV(1 + i)n. PV = FV / (1+i)n. Discounting is the
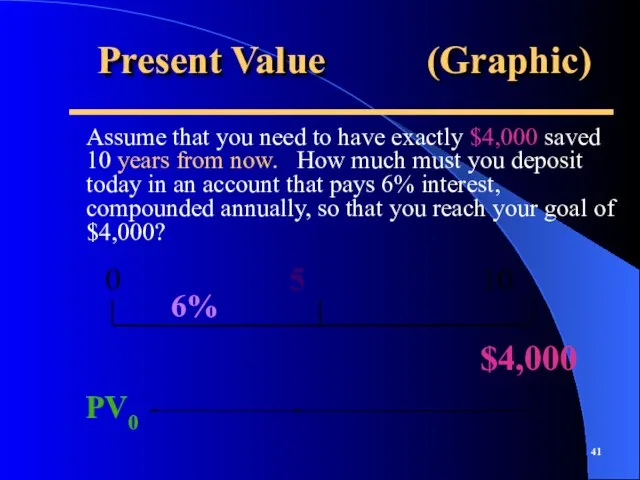
- 41. Present Value (Graphic) Assume that you need to have exactly $4,000 saved 10 years from now.
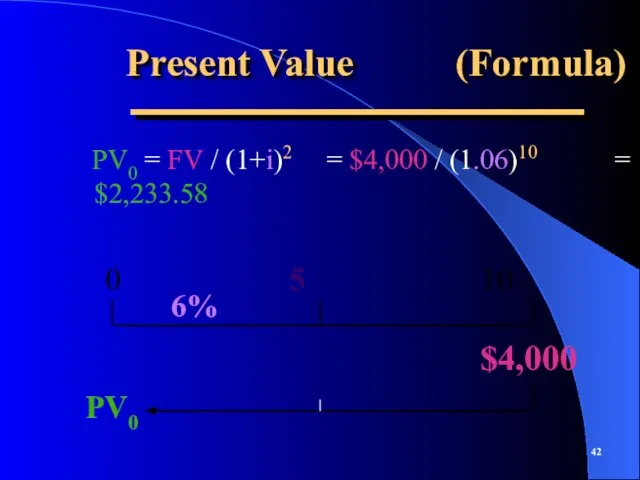
- 42. Present Value (Formula) PV0 = FV / (1+i)2 = $4,000 / (1.06)10 = $2,233.58 0 5
- 43. Present Value Example Joann needs to know how large of a deposit to make today so
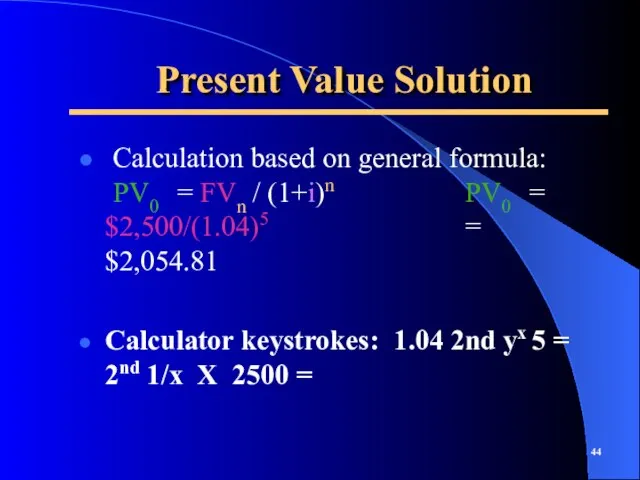
- 44. Present Value Solution Calculation based on general formula: PV0 = FVn / (1+i)n PV0 = $2,500/(1.04)5
- 45. Finding “n” or “i” when one knows PV and FV(6/8.5) If one invests $2,000 today and
- 46. Frequency of Compounding General Formula: FVn = PV0(1 + [i/m])mn n: Number of Years m: Compounding
- 47. Frequency of Compounding Example Suppose you deposit $1,000 in an account that pays 12% interest, compounded
- 48. Solution based on formula: FV= PV (1 + i)n = 1,000(1.03)32 = 2,575.10 Calculator Keystrokes: 1.03
- 49. Annuities An annuity is a special cash flow pattern in which, as its name implies, a
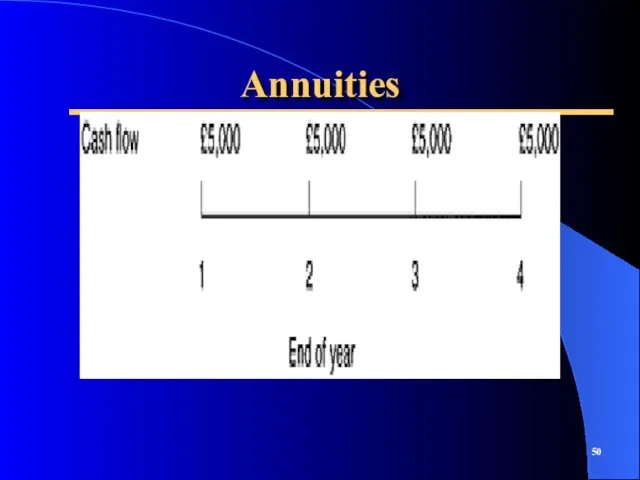
- 50. Annuities
- 51. Annuities-Examples Example 8 —The future value of an annuity Anna would like to know how much
- 52. FV of an Annuities-Examples
- 53. FV of an Annuities-Examples Notice that since the first £1,000 is invested at the end of
- 54. FV of an Annuities-Examples For example, if you know that you will need £10,000 in eight
- 56. Скачать презентацию


































![Frequency of Compounding General Formula: FVn = PV0(1 + [i/m])mn n: Number](/_ipx/f_webp&q_80&fit_contain&s_1440x1080/imagesDir/jpg/376780/slide-45.jpg)







 Академия ИТС по продажам сервисов 1С:ИТС. Этапы отработки входящего звонка
Академия ИТС по продажам сервисов 1С:ИТС. Этапы отработки входящего звонка 29-30 марта 2012 года в государственном университете им. М. Ауэзова состоялась IV Республиканская предметная олимпиада среди студентов
29-30 марта 2012 года в государственном университете им. М. Ауэзова состоялась IV Республиканская предметная олимпиада среди студентов  Оценка и отбор перспективных идей нового товара, разработка концепции нового товара
Оценка и отбор перспективных идей нового товара, разработка концепции нового товара Учет и внутренний контроль производственных запасов
Учет и внутренний контроль производственных запасов Новые государственные стандарты. Документы
Новые государственные стандарты. Документы Почему протекают химические реакции
Почему протекают химические реакции Наполним музыкой сердца
Наполним музыкой сердца ВКР: Оптимизация работы участка дистанции погрузочноразгрузочных работ с крупнотоннажными контейнерами
ВКР: Оптимизация работы участка дистанции погрузочноразгрузочных работ с крупнотоннажными контейнерами A.П.Чexoв - вpaч. Oтpaжeниe этoй тeмы в твopчecтвe пиcaтeля
A.П.Чexoв - вpaч. Oтpaжeниe этoй тeмы в твopчecтвe пиcaтeля Организация привалов и ночлегов в походе. Виды привалов. Требования к месту выбора бивака
Организация привалов и ночлегов в походе. Виды привалов. Требования к месту выбора бивака Что такое «нанотехнологии»???
Что такое «нанотехнологии»??? Цех по производству мобильных пусковых установок для межконтинентальных баллистических ракет Муравейник 3М
Цех по производству мобильных пусковых установок для межконтинентальных баллистических ракет Муравейник 3М Справочные материалы к Концепции социально – экономического развития Российской Федерации до 2020 года
Справочные материалы к Концепции социально – экономического развития Российской Федерации до 2020 года Синдром эмоционального выгорания педагогов Автор : педагог-психолог высшей квал
Синдром эмоционального выгорания педагогов Автор : педагог-психолог высшей квал Корпоративная Система управления документамина платформах Documentum и SAPООО «Газпром трансгаз Уфа»
Корпоративная Система управления документамина платформах Documentum и SAPООО «Газпром трансгаз Уфа» "Я ЛЮБЛЮ ТЕБЯ,РОССИЯ!" Игра "Звездный час" (для учащихся 3-4классов)
"Я ЛЮБЛЮ ТЕБЯ,РОССИЯ!" Игра "Звездный час" (для учащихся 3-4классов) Кто хочет стать отличником?
Кто хочет стать отличником? Правда в пьесе М. Горького «На дне»
Правда в пьесе М. Горького «На дне» BTAATAAB В ведение Тезис Аргументы Аргументы Тезис Аргументы Аргументы ывод.
BTAATAAB В ведение Тезис Аргументы Аргументы Тезис Аргументы Аргументы ывод. Специалист по документальному обеспечению управления
Специалист по документальному обеспечению управления Трехмерный индикатор:Светодиодный куб
Трехмерный индикатор:Светодиодный куб Bosu – что это
Bosu – что это ТЗ для фотосъемки
ТЗ для фотосъемки 6 шиповое соединение
6 шиповое соединение Презентация на тему Фитонцидные растения
Презентация на тему Фитонцидные растения  Проекты компании "ТЕРМИКА" по обучению и повышению квалификации персонала, работающего в сфере документационного обеспечения уп
Проекты компании "ТЕРМИКА" по обучению и повышению квалификации персонала, работающего в сфере документационного обеспечения уп Design
Design Информация для промоутеров о бренде Pulpy (Палпи) Свежая мякоть
Информация для промоутеров о бренде Pulpy (Палпи) Свежая мякоть