Содержание
- 2. Tunnel effect is one of the most important manifestations of quantum mechanics
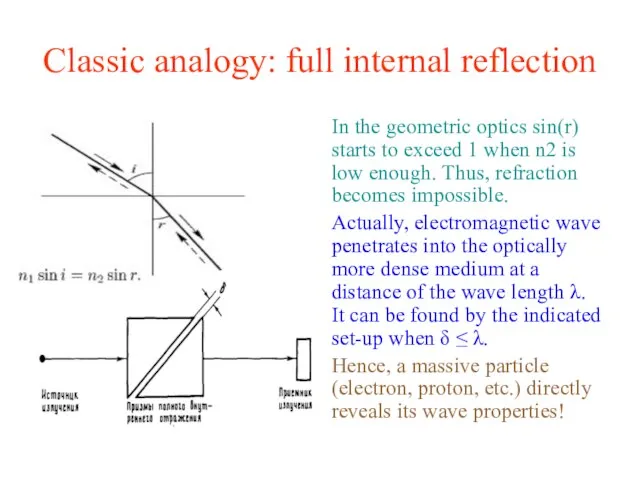
- 3. Classic analogy: full internal reflection In the geometric optics sin(r) starts to exceed 1 when n2
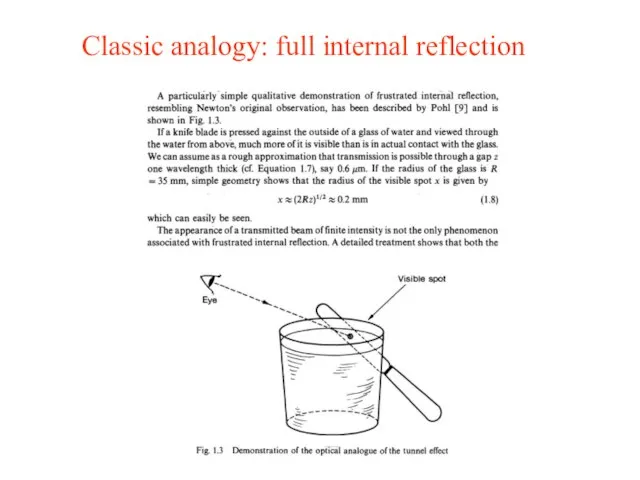
- 4. Classic analogy: full internal reflection
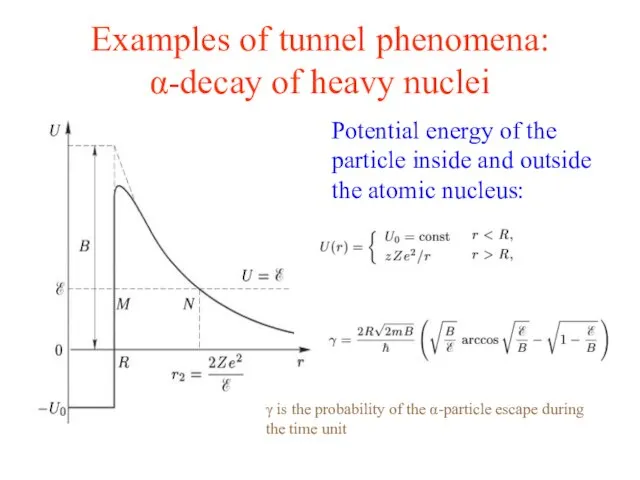
- 5. Examples of tunnel phenomena: α-decay of heavy nuclei Potential energy of the particle inside and outside
- 6. Scientists, who discovered the tunnel effect
- 7. Scientists, who discovered the tunnel effect
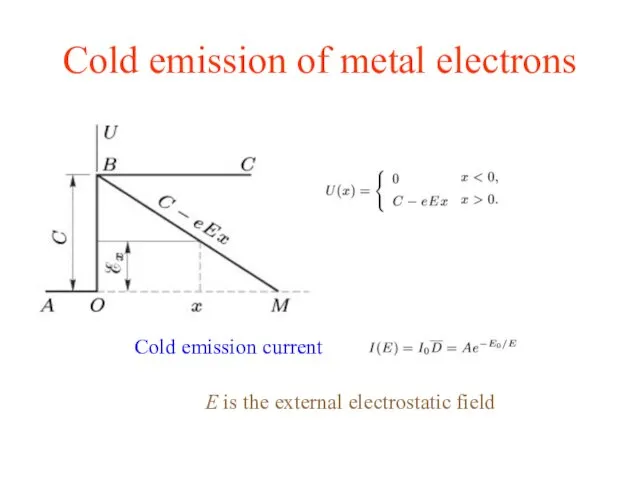
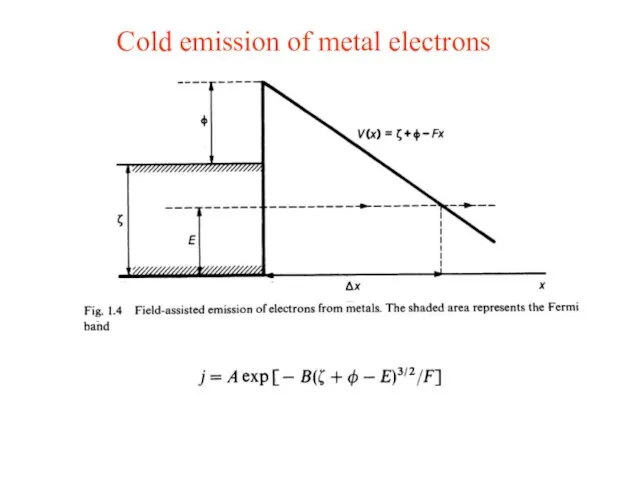
- 8. Cold emission of metal electrons Cold emission current E is the external electrostatic field
- 9. Cold emission of metal electrons
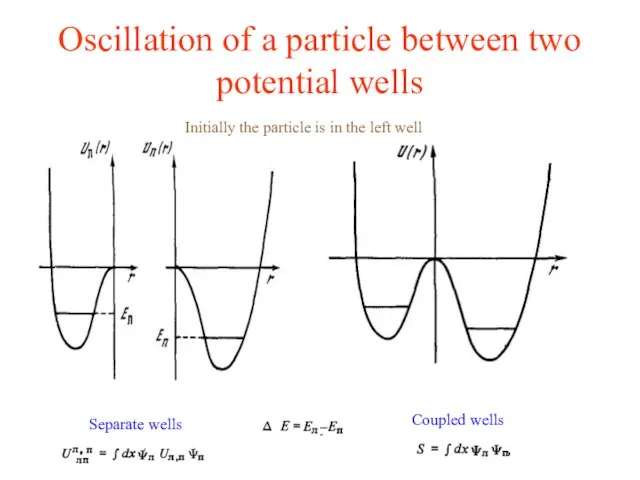
- 10. Oscillation of a particle between two potential wells Separate wells Coupled wells Initially the particle is
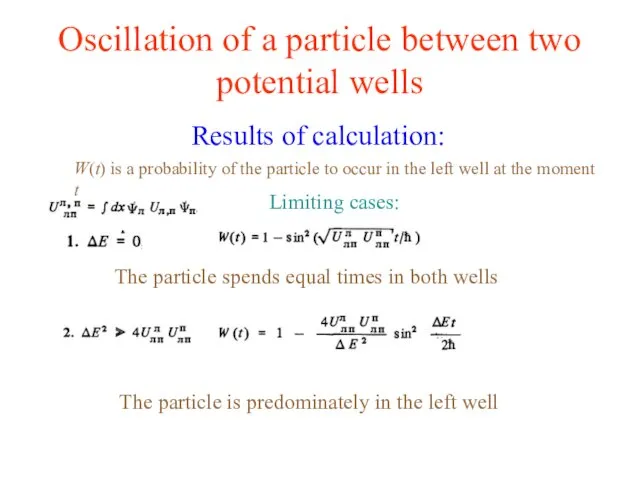
- 11. Oscillation of a particle between two potential wells Results of calculation: W(t) is a probability of
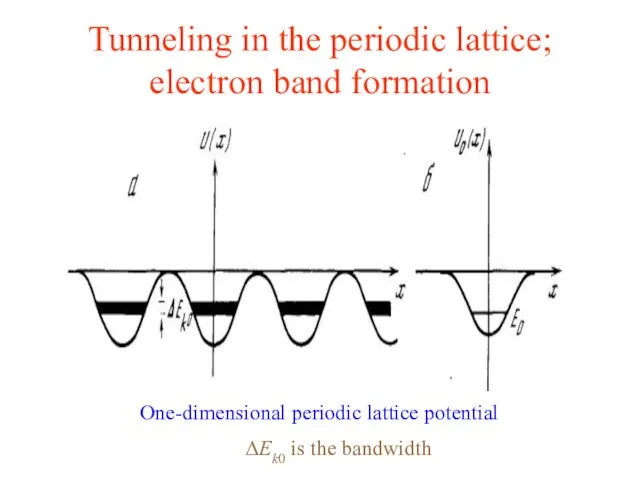
- 12. Tunneling in the periodic lattice; electron band formation One-dimensional periodic lattice potential ΔEk0 is the bandwidth
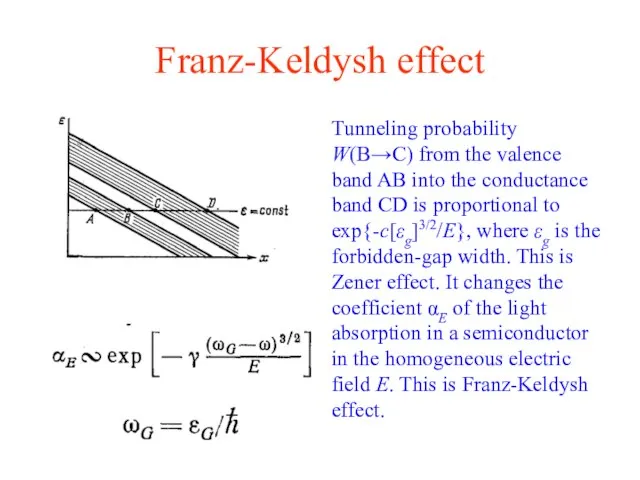
- 13. Franz-Keldysh effect Tunneling probability W(B→C) from the valence band AB into the conductance band CD is
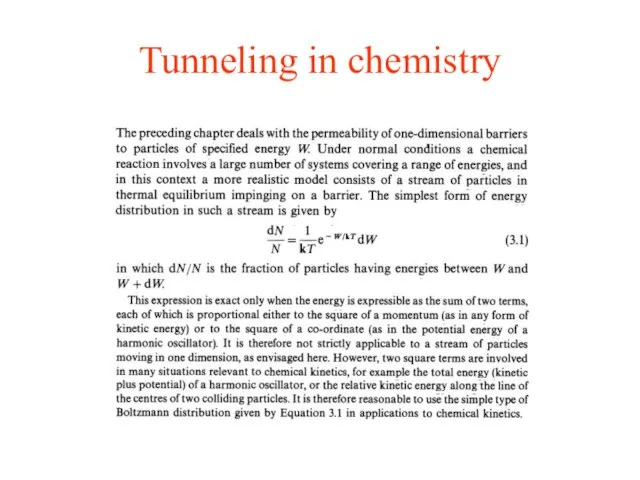
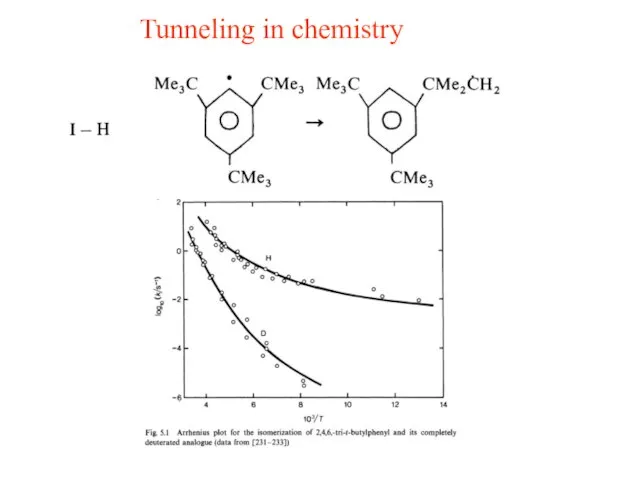
- 14. Tunneling in chemistry
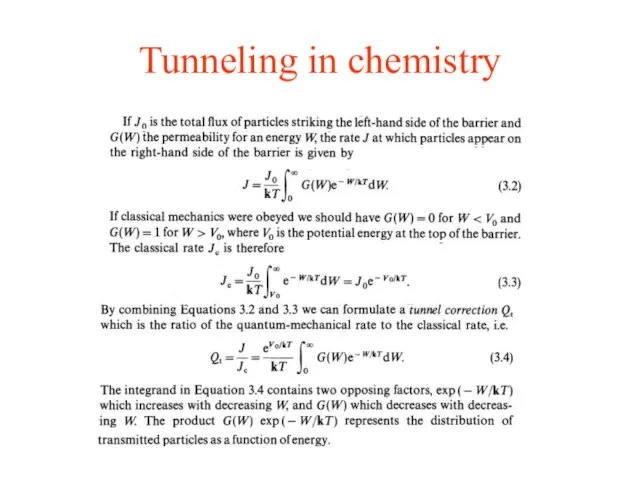
- 15. Tunneling in chemistry
- 16. Tunneling in chemistry
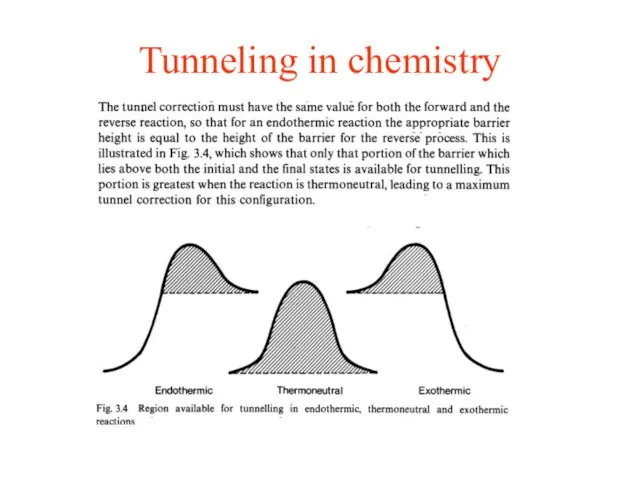
- 17. Tunneling in chemistry
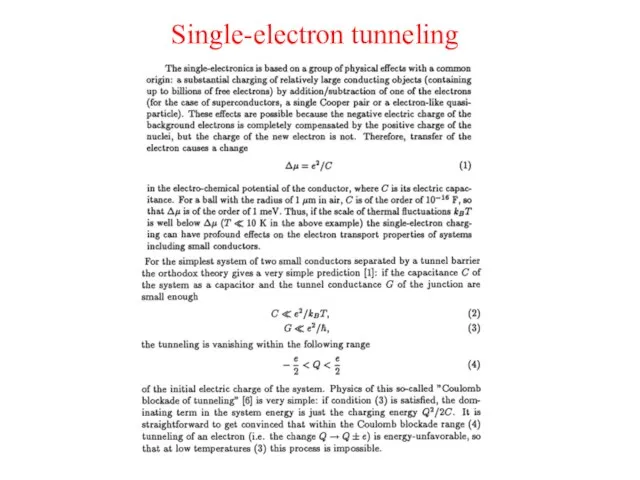
- 18. Single-electron tunneling
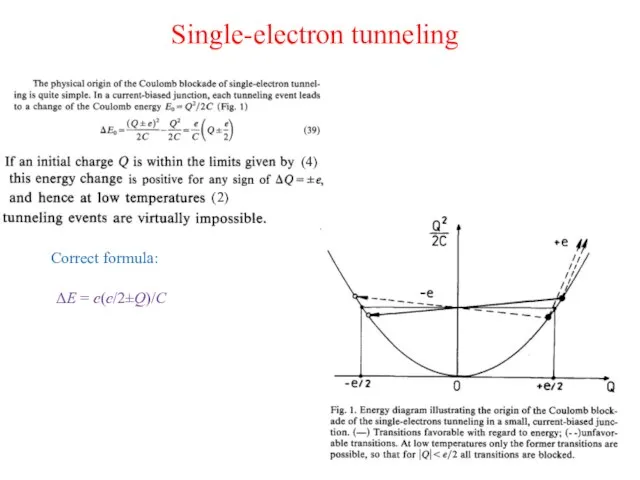
- 19. Single-electron tunneling (4) (2) ΔE = e(e/2±Q)/C Correct formula:
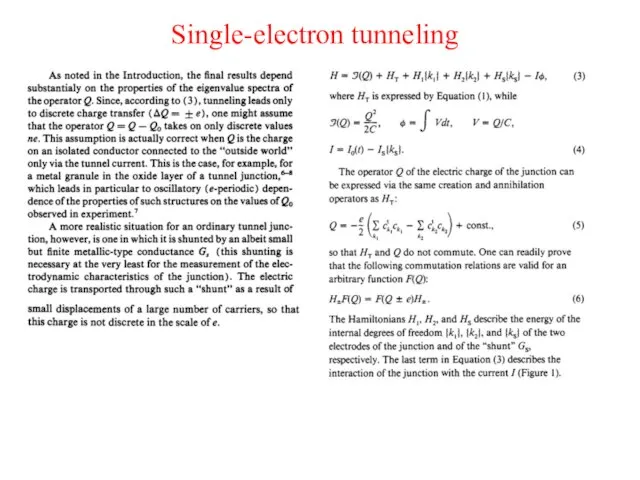
- 20. Single-electron tunneling
- 22. Скачать презентацию



 Тверские зодчие
Тверские зодчие «Вологжане в Отечественной войне 1812 года»
«Вологжане в Отечественной войне 1812 года» Презентация на тему Образ Бабы Яги в русских народных сказках
Презентация на тему Образ Бабы Яги в русских народных сказках Кулинария- творческий проект
Кулинария- творческий проект  Требования и виды КИМ (для разработки РП)
Требования и виды КИМ (для разработки РП) совместно с Театром праздника «Солнечный зайчик»
совместно с Театром праздника «Солнечный зайчик»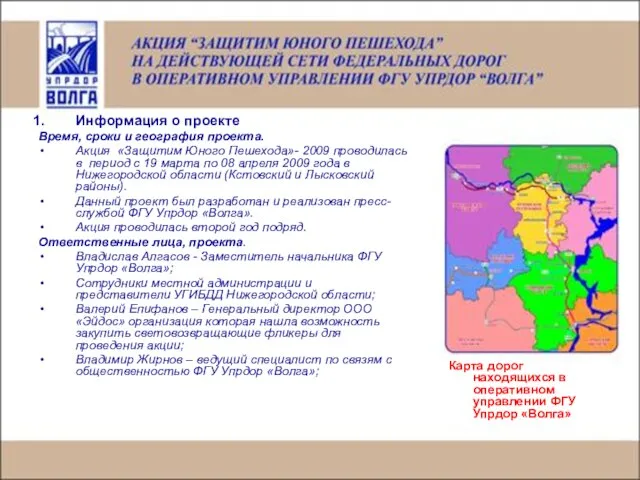 Информация о проекте Время, сроки и география проекта. Акция «Защитим Юного Пешехода»- 2009 проводилась в период с 19 марта по 08 апре
Информация о проекте Время, сроки и география проекта. Акция «Защитим Юного Пешехода»- 2009 проводилась в период с 19 марта по 08 апре М.М. Денисов, Б.Е.Левицкий Кубанский государственный университет г. Краснодар
М.М. Денисов, Б.Е.Левицкий Кубанский государственный университет г. Краснодар Монетизация/продажа патентов - конкретный пример сделки одной русской софтверной фирмы
Монетизация/продажа патентов - конкретный пример сделки одной русской софтверной фирмы Матрёшка
Матрёшка Решение задач на составление уравнений
Решение задач на составление уравнений Закрытая распродажа мебели
Закрытая распродажа мебели Жарқын болашаққа жол
Жарқын болашаққа жол Социальная реклама и её влияние на социализацию учащихся.
Социальная реклама и её влияние на социализацию учащихся. Поведение
Поведение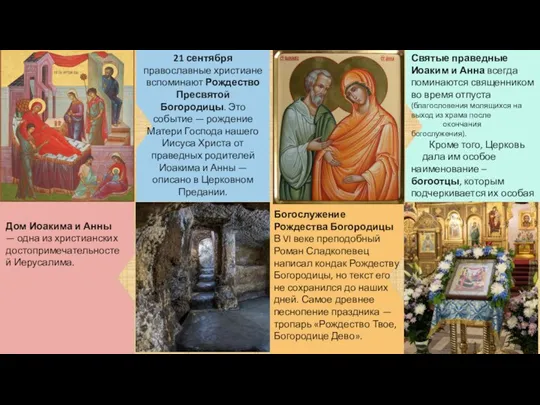 21 сентября - православные христиане вспоминают Рождество
21 сентября - православные христиане вспоминают Рождество Здоровый образ жизни
Здоровый образ жизни Александр Невский
Александр Невский Страхи в дошкольном возрасте
Страхи в дошкольном возрасте Управление поведением потребителей на примере торговой сети Верный
Управление поведением потребителей на примере торговой сети Верный Актуальные вопросы преподавания новейшей истории и обществознания в общеобразовательных учреждениях и разработки государственн
Актуальные вопросы преподавания новейшей истории и обществознания в общеобразовательных учреждениях и разработки государственн school
school Фирма "1С" в электроэнергетике: анализ крупнейших внедрений
Фирма "1С" в электроэнергетике: анализ крупнейших внедрений Философия_как_способ_духовного_познания
Философия_как_способ_духовного_познания Это мои первые слайды про семью
Это мои первые слайды про семью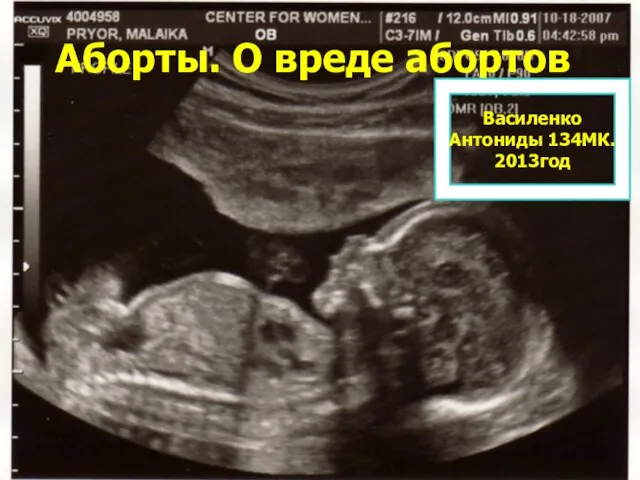 Аборты. О вреде абортов
Аборты. О вреде абортов Жилин Олег Иванович заместитель директора по учебной и научной работекандидат технических наук, доцент
Жилин Олег Иванович заместитель директора по учебной и научной работекандидат технических наук, доцент Способы обработки низа юбки
Способы обработки низа юбки