Содержание
- 2. Goal The goal of this tutorial is to provide a background in cryogenics suitable for workers
- 3. Outline Part 1: Catching Cold Introduction To Cryogenics Basic refrigeration processes Isenthalpic (Joule-Thomson) Isentropic expansion Carnot
- 4. Outline Part 2: Keeping Cold Cryogenic Safety Oxygen Deficiency Hazards Pressure safety High Level Guidelines Cryostats
- 5. What is Cryogenics ? Cryogenics is the science & engineering of phenomena that occur at temperatures
- 6. Some Examples
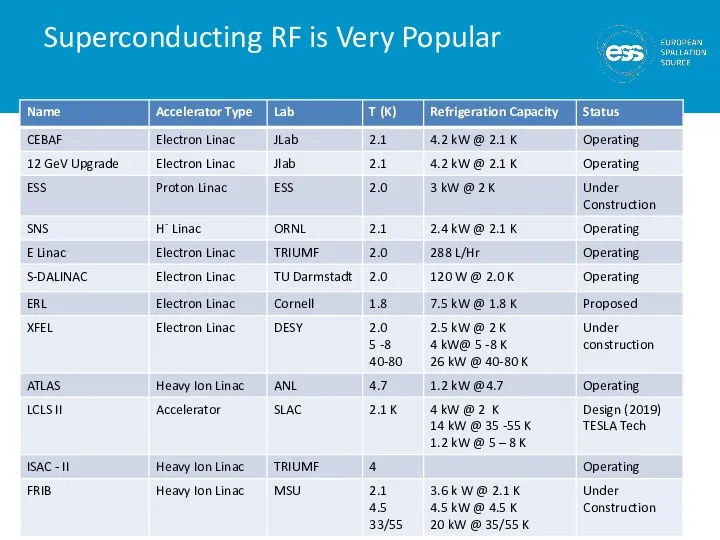
- 7. Superconducting RF is Very Popular
- 8. Catching Cold Before we get involved in thermodynamic cycles, let’s go over the basics There are
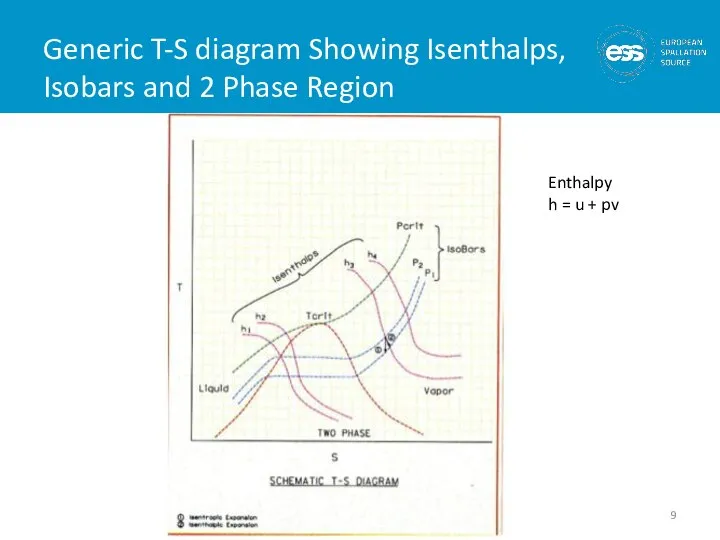
- 9. Generic T-S diagram Showing Isenthalps, Isobars and 2 Phase Region Enthalpy h = u + pv
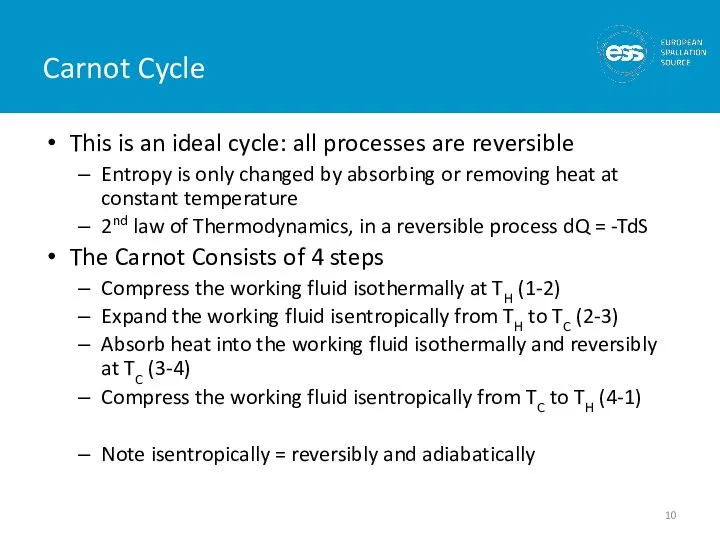
- 10. Carnot Cycle This is an ideal cycle: all processes are reversible Entropy is only changed by
- 11. Carnot Cycle How do we describe the performance of such a cycle?
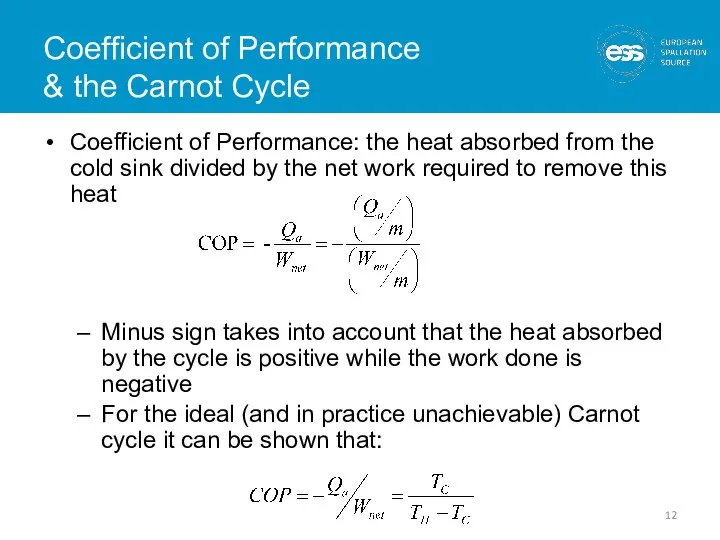
- 12. Coefficient of Performance & the Carnot Cycle Coefficient of Performance: the heat absorbed from the cold
- 13. Coefficient of Performance & the Carnot Cycle For a plant operating between room 300 K and
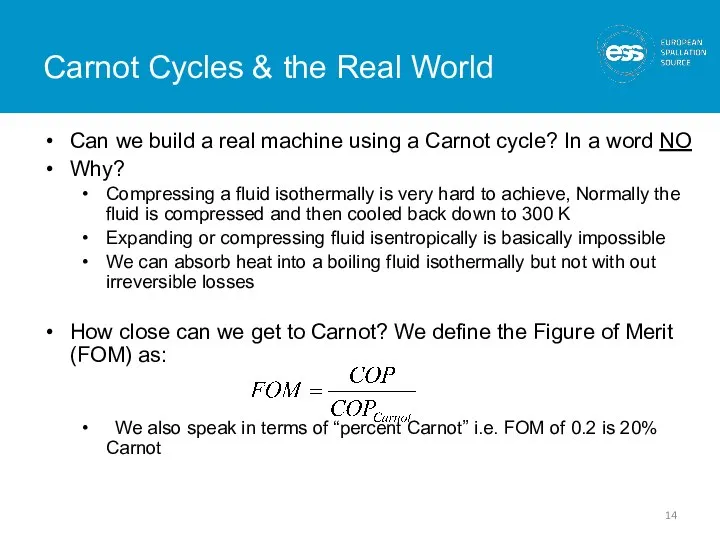
- 14. Carnot Cycles & the Real World Can we build a real machine using a Carnot cycle?
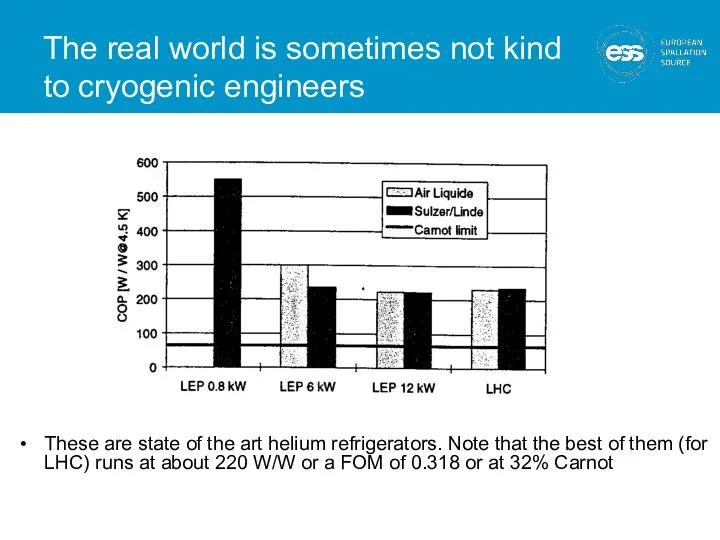
- 15. The real world is sometimes not kind to cryogenic engineers These are state of the art
- 16. Practical Impact of Plant Performance How much power does it take to operate a large cryogenic
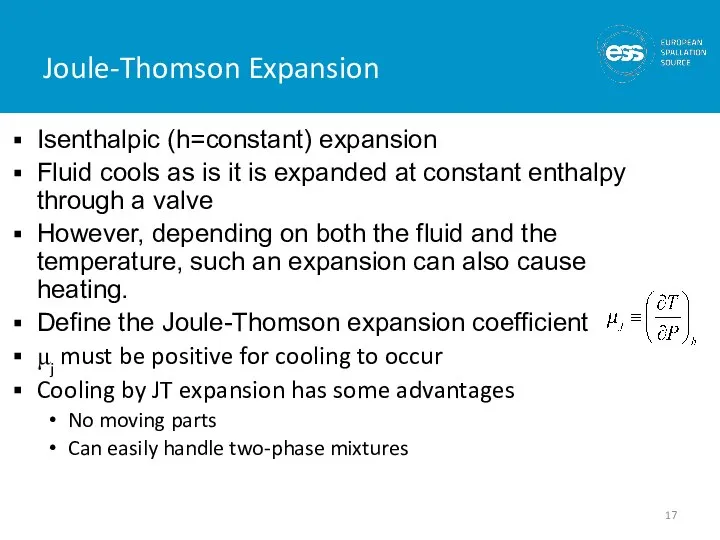
- 17. Joule-Thomson Expansion Isenthalpic (h=constant) expansion Fluid cools as is it is expanded at constant enthalpy through
- 18. JT Inversion Curve & Maximum Inversion Temperatures Maximum inversion temperature for helium is 43 K Note
- 19. Practical Large Scale Helium Refrigerators Modern large scale Helium refrigerators/liquefiers use a variation of the Claude
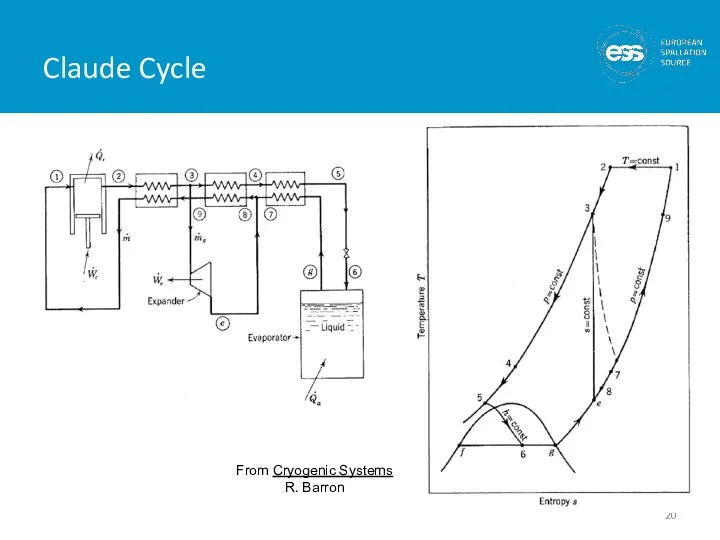
- 20. Claude Cycle From Cryogenic Systems R. Barron
- 21. Cycle consists of : 1) Compression to ~ 16 Bar with cooling back to 300 K
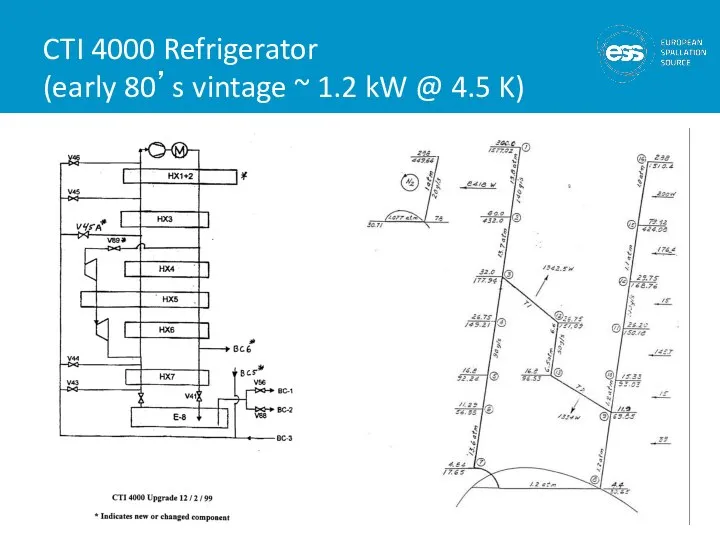
- 22. CTI 4000 Refrigerator (early 80’s vintage ~ 1.2 kW @ 4.5 K)
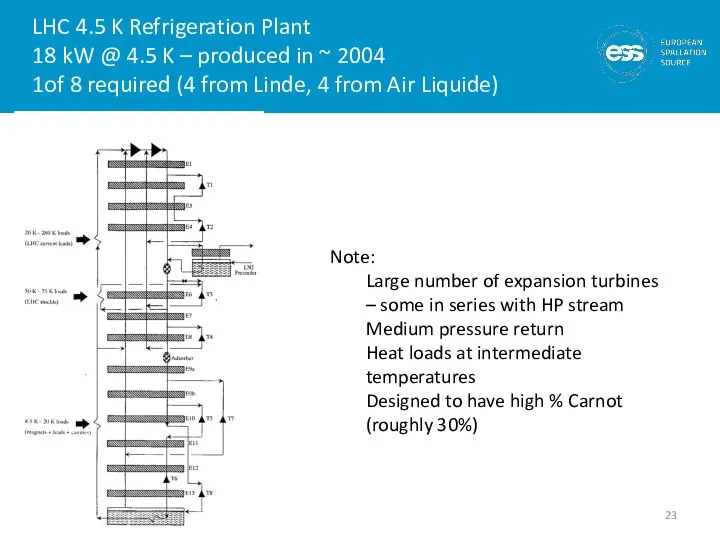
- 23. LHC 4.5 K Refrigeration Plant 18 kW @ 4.5 K – produced in ~ 2004 1of
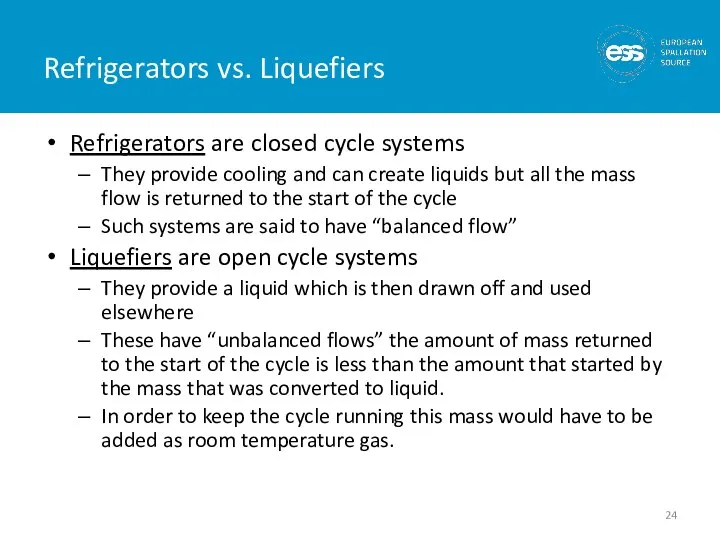
- 24. Refrigerators vs. Liquefiers Refrigerators are closed cycle systems They provide cooling and can create liquids but
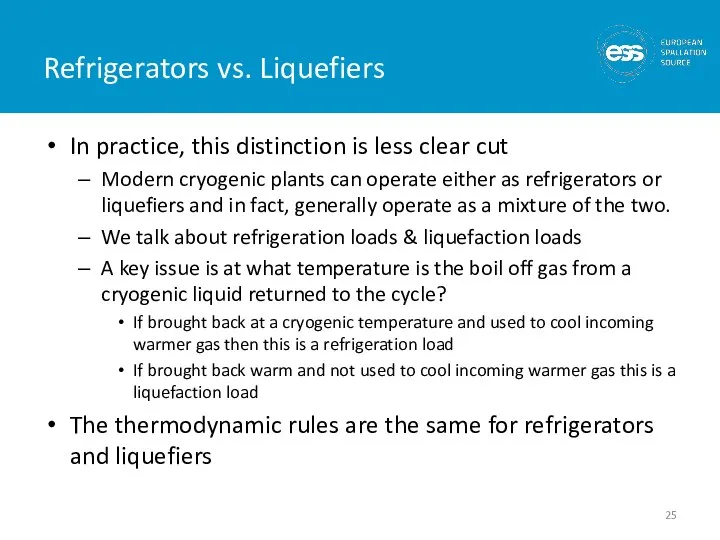
- 25. Refrigerators vs. Liquefiers In practice, this distinction is less clear cut Modern cryogenic plants can operate
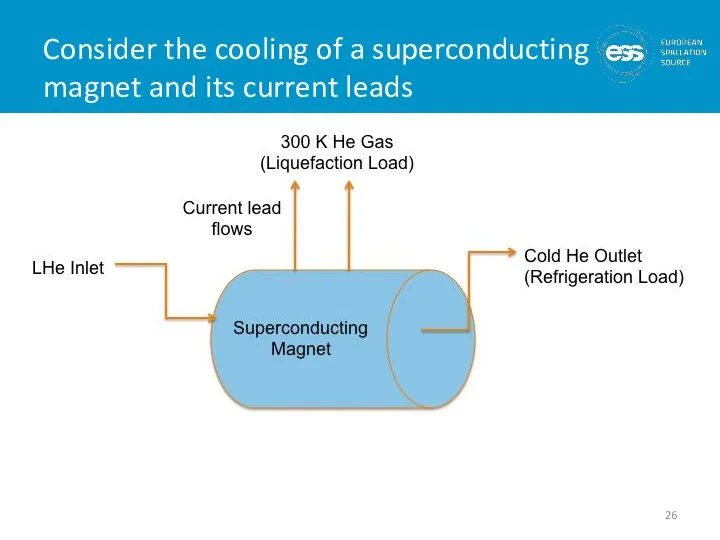
- 26. Consider the cooling of a superconducting magnet and its current leads
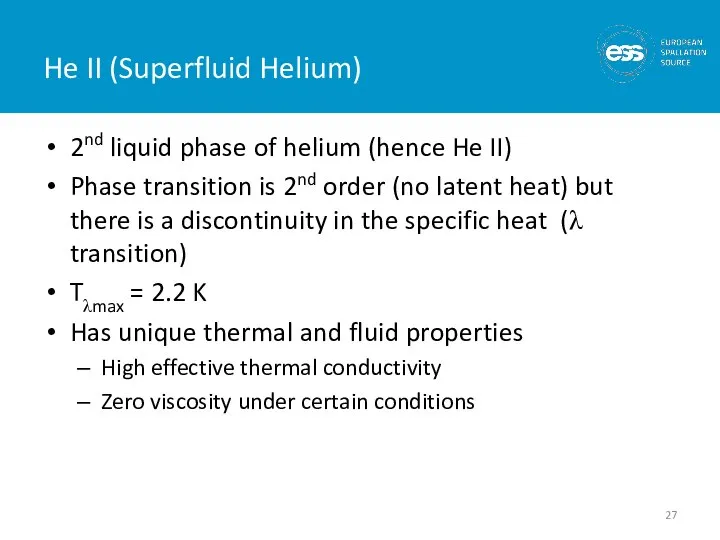
- 27. He II (Superfluid Helium) 2nd liquid phase of helium (hence He II) Phase transition is 2nd
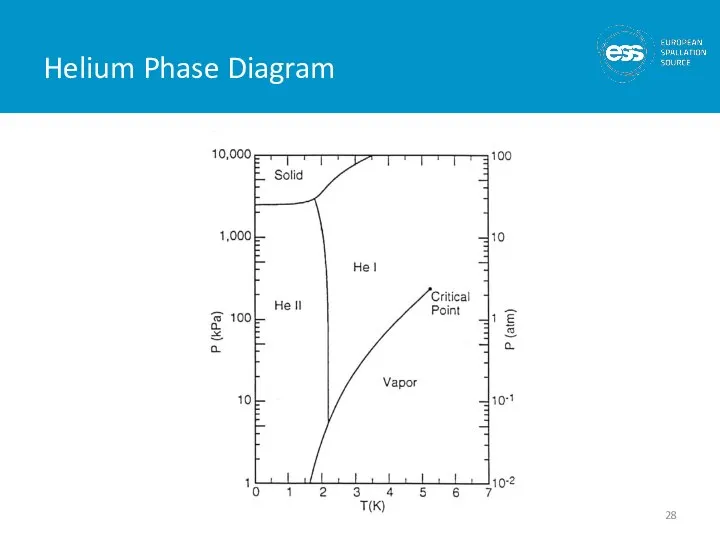
- 28. Helium Phase Diagram
- 29. Why Use He II in SRF Systems? The biggest single advantage is the lower temperature (
- 30. What is He II ? A “Bose – Einstein like” Condensate A fraction of atoms in
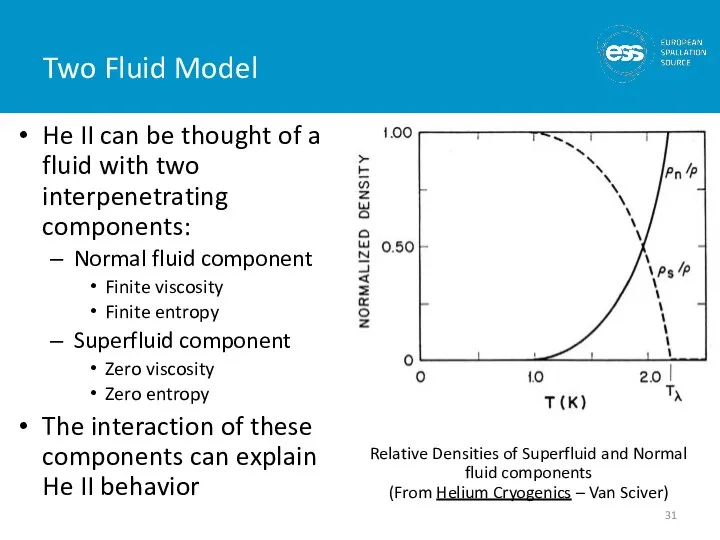
- 31. Two Fluid Model He II can be thought of a fluid with two interpenetrating components: Normal
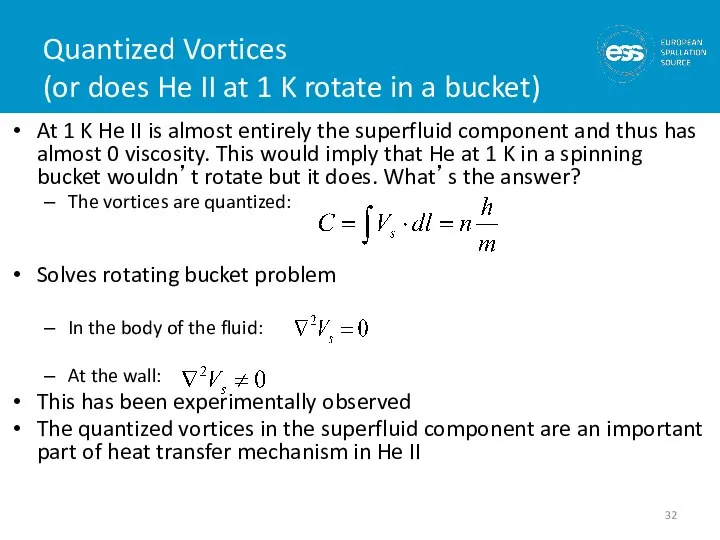
- 32. Quantized Vortices (or does He II at 1 K rotate in a bucket) At 1 K
- 33. Direct Observation of Quantized Vortices via Electron Trapping
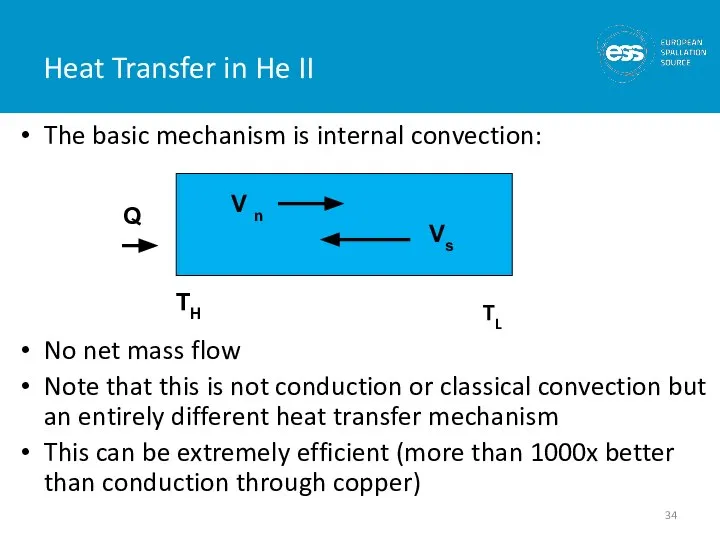
- 34. Heat Transfer in He II The basic mechanism is internal convection: No net mass flow Note
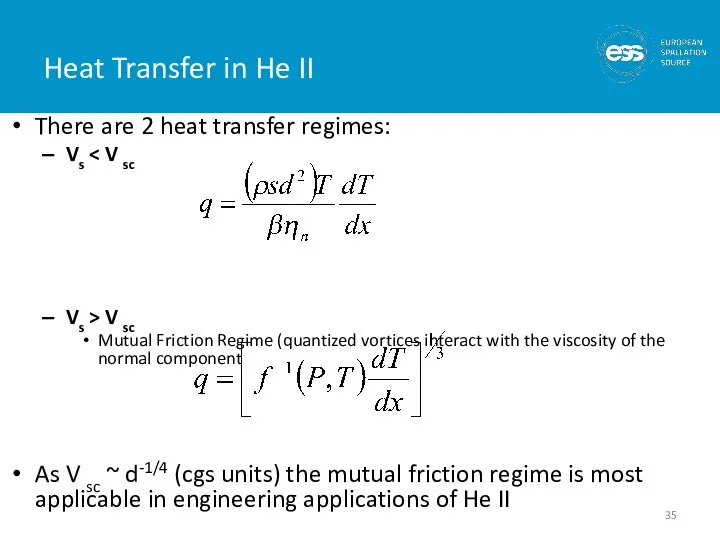
- 35. Heat Transfer in He II There are 2 heat transfer regimes: Vs Vs > V sc
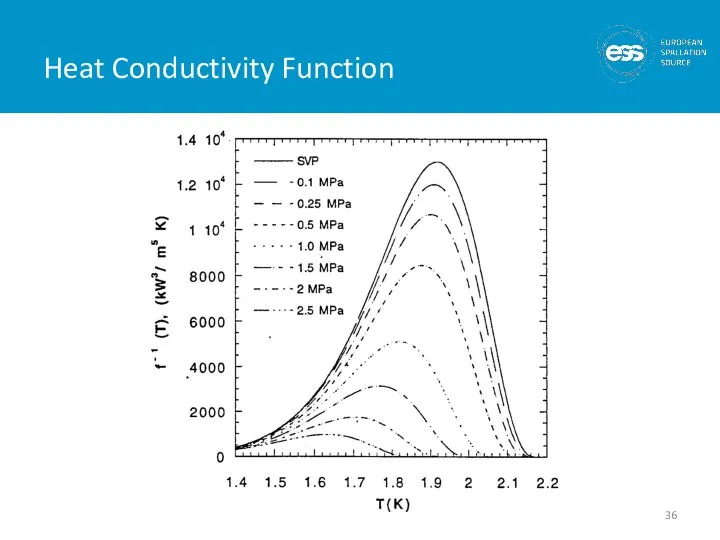
- 36. Heat Conductivity Function
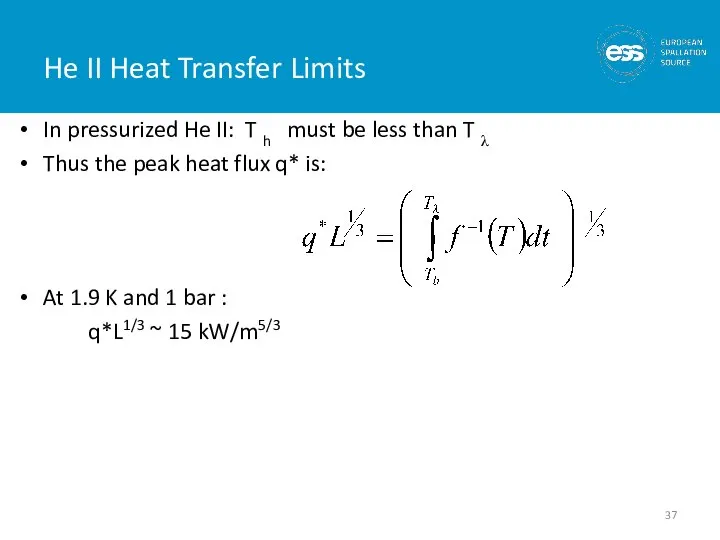
- 37. He II Heat Transfer Limits In pressurized He II: T h must be less than T
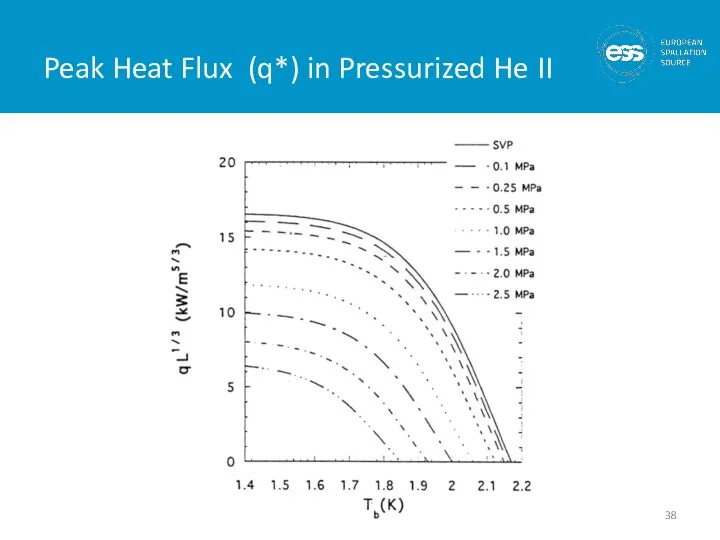
- 38. Peak Heat Flux (q*) in Pressurized He II
- 39. Limits on He II Heat Transfer In saturated He II, the limit is given by the
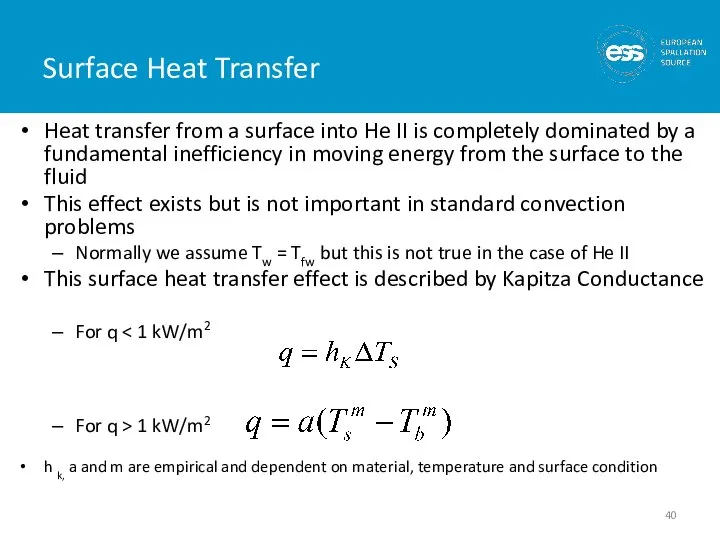
- 40. Surface Heat Transfer Heat transfer from a surface into He II is completely dominated by a
- 41. Surface Heat Transfer m ~ 3 Kapitza conductance is not dependent on helium flow rate
- 42. Forced Convection and He II If Kapitza Conductance is independent of flow rate does forced convection
- 43. He II Fluid Dynamics Despite the presence of the superfluid component, in almost all engineering applications
- 44. He II Fluid Dynamics He II does behave differently in cases of: Film flow Porous plugs
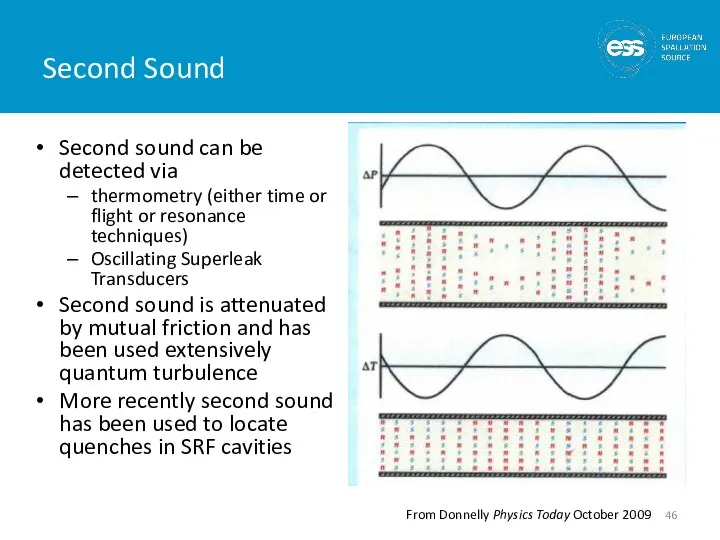
- 45. Second Sound The two-fluid model predicts and experiments show that temperature waves may be established in
- 46. Second Sound Second sound can be detected via thermometry (either time or flight or resonance techniques)
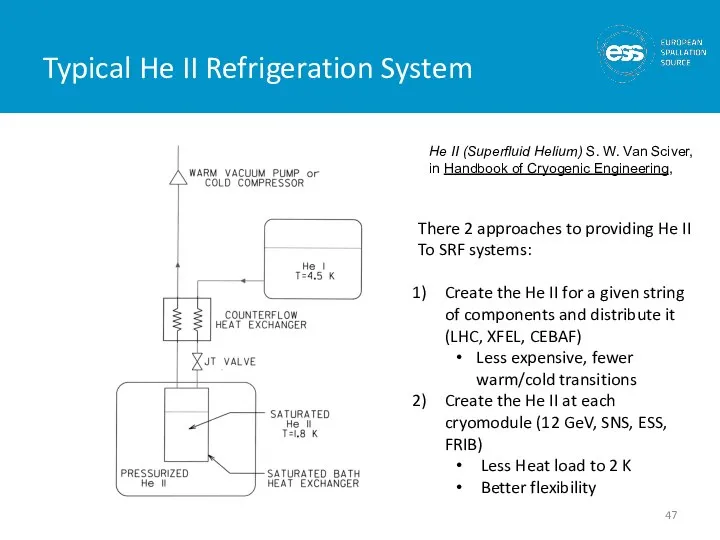
- 47. Typical He II Refrigeration System He II (Superfluid Helium) S. W. Van Sciver, in Handbook of
- 49. Скачать презентацию















 Distributed Version Control Systems
Distributed Version Control Systems L2_Semiotika
L2_Semiotika Педагогическая система ноосферной ориентацииМОУ лицей №3 г. Екатеринбурга
Педагогическая система ноосферной ориентацииМОУ лицей №3 г. Екатеринбурга Cairngorm Microarchitecture
Cairngorm Microarchitecture Трудовое право
Трудовое право Грусть. Как помочь тому кто грустит. Занятие с дошкольниками
Грусть. Как помочь тому кто грустит. Занятие с дошкольниками Маркетинг в 20 веке: Найди потребность клиента и удовлетвори её. Маркетинг в 21 веке:Создай потребность и заставь оплатить
Маркетинг в 20 веке: Найди потребность клиента и удовлетвори её. Маркетинг в 21 веке:Создай потребность и заставь оплатить Презентация Лоскутное шитьё "Мозаика цвета неба"
Презентация Лоскутное шитьё "Мозаика цвета неба" Чыгарылыш-79
Чыгарылыш-79 Инвестирование и кредитование предприятий энергетической отрасли в России – финансовые стратегии
Инвестирование и кредитование предприятий энергетической отрасли в России – финансовые стратегии Дошкольное образование в Швейцарии
Дошкольное образование в Швейцарии Знатоки природы
Знатоки природы Концепция пропорциональности в международном гуманитарном праве
Концепция пропорциональности в международном гуманитарном праве Шаблон. Научно-исследовательская работа
Шаблон. Научно-исследовательская работа NOVAE – НОВЫЕ ЗВЕЗДЫ
NOVAE – НОВЫЕ ЗВЕЗДЫ Презентация на тему "Абстрактное искусство"
Презентация на тему "Абстрактное искусство" Romanticism
Romanticism Белорусский государственный технологический университет. Кафедра экономики и управления на предприятиях
Белорусский государственный технологический университет. Кафедра экономики и управления на предприятиях Уважаемые Коллеги! Примите самые искренние поздравления с профессиональным праздником - Днем медицинского работника! Традиционно
Уважаемые Коллеги! Примите самые искренние поздравления с профессиональным праздником - Днем медицинского работника! Традиционно Визитная карточка компании ЗАО СШС
Визитная карточка компании ЗАО СШС Что значит быть патриотом сегодня?
Что значит быть патриотом сегодня? Винтовой насос
Винтовой насос ПОТЕРЯННЫЕ ДУШИ
ПОТЕРЯННЫЕ ДУШИ История Северной войны
История Северной войны ИНФОРМАЦИОННЫЕ МОДЕЛИ
ИНФОРМАЦИОННЫЕ МОДЕЛИ Внутренняя среда организма. Плазма крови
Внутренняя среда организма. Плазма крови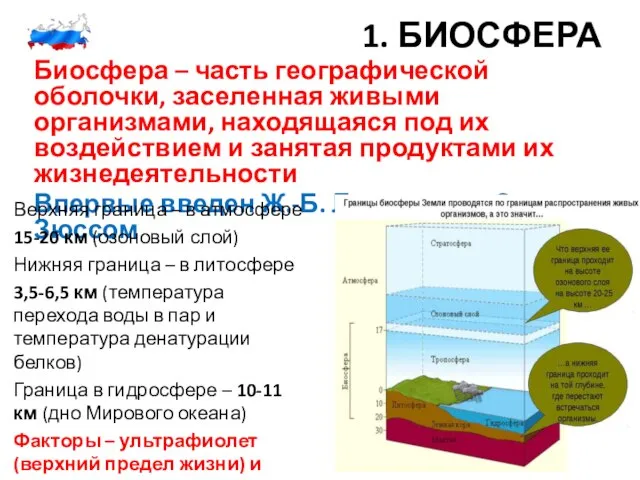 БИОСФЕРА
БИОСФЕРА РоссельхозБанк. Вакансии
РоссельхозБанк. Вакансии