Слайд 2Quantum Physics – introduction
Getting ‘into’ the atom
Late in the 19th century, physicists

explained properties of matter with particle model, light with wave model. Classical physics, which was continuous, had no theoretical limits on size of matter or radiation. Mechanics and electromagnetism together seemed to be enough.
However…
Слайд 3Black-body radiation remained unexplained
All hot bodies radiate.
The known spectrum did not coincide
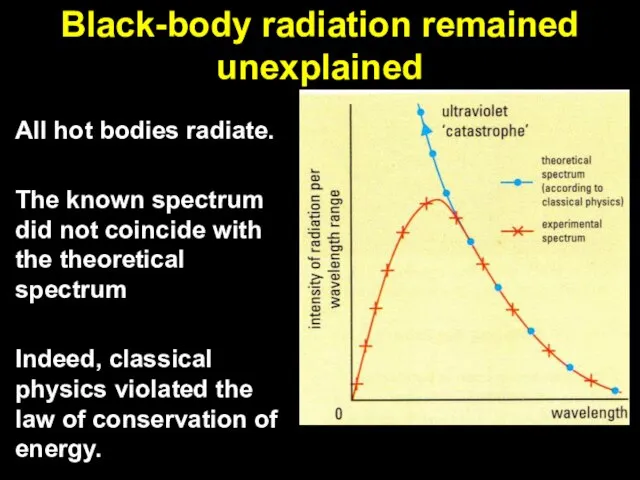
with the theoretical spectrum
Indeed, classical physics violated the law of conservation of energy.
Слайд 4In 1900, Planck postulated that
All oscillators that emit radiation can only emit

discrete energies. That is, radiation comes in packets or quanta (plural of quantum). These quanta are proportional to frequency:
E = hf
where h = 6.63 x 10-34 Js = 4.14 x 10-15 eV-s
h is known as “Planck’s constant”
Слайд 5Definition
What is one electronvolt?

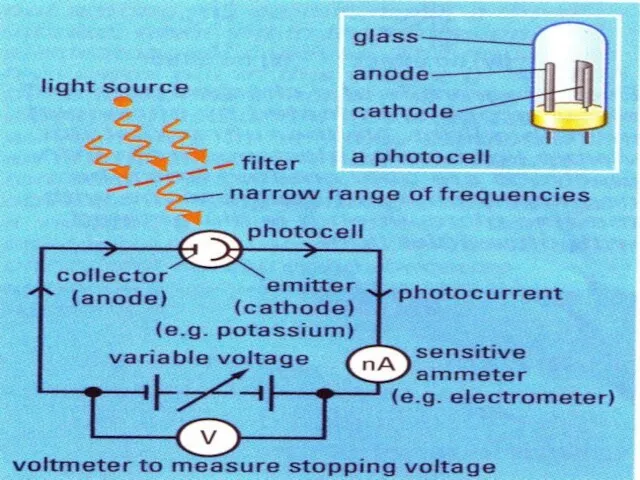
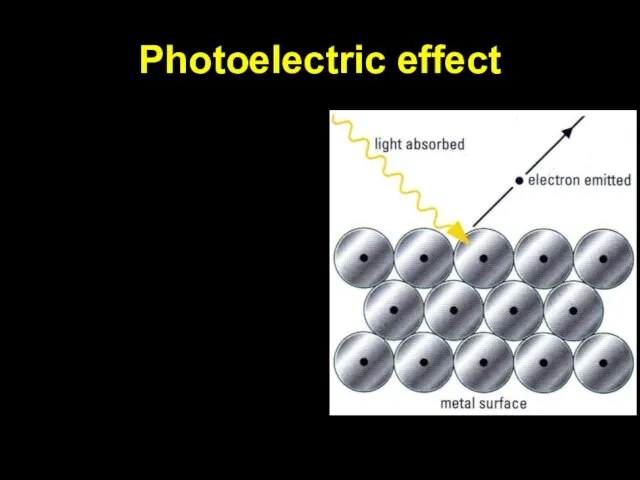
Слайд 6Along comes Albert…
Photoelectric effect

Слайд 9This equation explained
1) For each metal, there is a threshold frequency below

which no electrons are emitted.
2) The number of electrons emitted is proportional to intensity of radiation.
3) Emitted electrons have KE up to a maximum value which depends on frequency of radiation*.
(* unexplained by classical physics)
Слайд 10
For his work on
the photoelectric
Effect, Einstein
was awarded
the Nobel
Prize in 1921

Слайд 11Example 1
a) Find the longest wavelength beyond which no electrons are emitted

from a caesium surface if Φcaesium = 1.80 eV.
b) If the surface is illuminated with monochromatic light of wavelength λ = 450 nm, calculate KEmax of emitted photoelectrons.
c) What is the stopping potential which just prevents photoemission in this situation?
Слайд 12De Broglie equation
During his PhD work, de Broglie proposed that electrons might

behave as waves.
Слайд 13Electron diffraction
Using de Broglie equation for electrons accelerated through 1kV gives a

wavelength of about _0.0388 nm_.
This is comparable to the wavelength of X-Rays.
Why are electrons easier to use than X-Rays when studying crystalline structures?
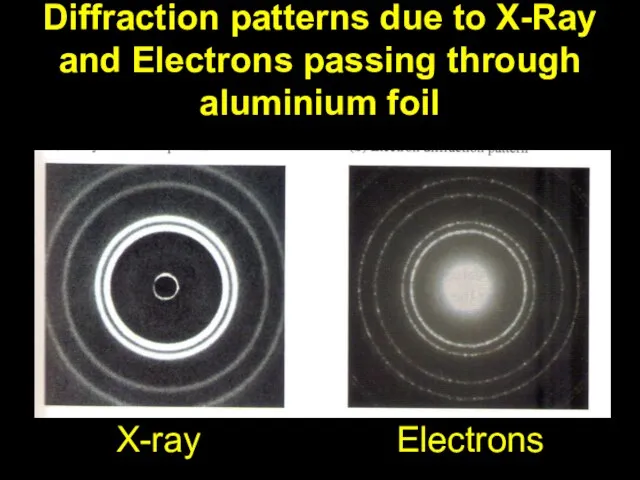
Слайд 14Diffraction patterns due to X-Ray and Electrons passing through aluminium foil
X-ray
Electrons
Слайд 15If all moving objects have an associated wave, why can’t we see

the waves associated with buses?
Слайд 16Wave-particle duality
Sometimes light behaves like a wave, sometimes light behaves like a

particle.
Wave-particle duality exists in the universe!
How accurately physical properties can be measured is limited.
Слайд 17Heisenberg’s uncertainty principle















 Vneshnyaya_politika_veduschikh_stran_mira
Vneshnyaya_politika_veduschikh_stran_mira Практика предоставления государственного имущества субъектам МСП и самозанятым гражданам в Республике Коми
Практика предоставления государственного имущества субъектам МСП и самозанятым гражданам в Республике Коми Презентация на тему Теорема Виета
Презентация на тему Теорема Виета  Презентация на тему Квест-технология
Презентация на тему Квест-технология  Ударение. Ударный слог
Ударение. Ударный слог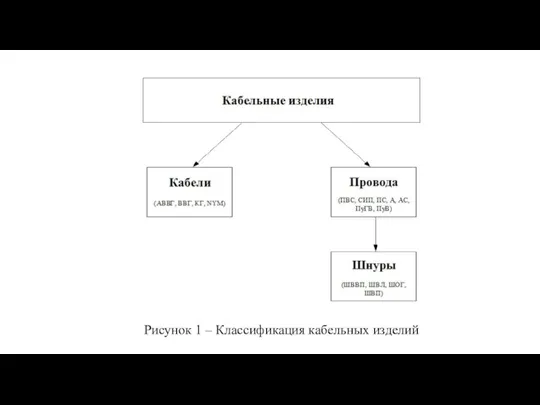 Кабельная продукция
Кабельная продукция Основы измерений на ВОЛС
Основы измерений на ВОЛС Прогулки по Великобритании
Прогулки по Великобритании Грим. Правила нанесения грима
Грим. Правила нанесения грима Композиция - сочетание принтов
Композиция - сочетание принтов Гепатит С
Гепатит С Экологические группы растений
Экологические группы растений  Energy Camp
Energy Camp ИВАНОВСКОЕ РЕГИОНАЛЬНОЕ НЕМЕЦКОЕ ОБЩЕСТВО (по поддержке деятельности, направленной на содействие развитию дружеских контактов и
ИВАНОВСКОЕ РЕГИОНАЛЬНОЕ НЕМЕЦКОЕ ОБЩЕСТВО (по поддержке деятельности, направленной на содействие развитию дружеских контактов и  Слово Победителю
Слово Победителю Электромагнитные колебания
Электромагнитные колебания Понятие и структура основного капитала корпорации
Понятие и структура основного капитала корпорации Брендинг террриторий
Брендинг террриторий Camping car !!!
Camping car !!! Оказание медицинской помощи при несчастных случаях
Оказание медицинской помощи при несчастных случаях Посвящение в первоклассники
Посвящение в первоклассники Компания НПО Криста. Бюджетный процесс и его участники
Компания НПО Криста. Бюджетный процесс и его участники Программа официальных открытых соревнований по триатлону
Программа официальных открытых соревнований по триатлону Комплексная автоматизация органов местного самоуправления для решения задач повышения налоговых доходов местных бюджетов, орган
Комплексная автоматизация органов местного самоуправления для решения задач повышения налоговых доходов местных бюджетов, орган ДП «Укрметртестстандарт» Науково-технічний семінар-нарада «Проблеми підвищення рівня метрологічного забезпечення вимірювань
ДП «Укрметртестстандарт» Науково-технічний семінар-нарада «Проблеми підвищення рівня метрологічного забезпечення вимірювань СТАНЦИЯ «ГЕОМЕТРИЧЕСКАЯ»
СТАНЦИЯ «ГЕОМЕТРИЧЕСКАЯ» Стимулирование свободным временем
Стимулирование свободным временем Социальный приют Маша. Фотоальбом
Социальный приют Маша. Фотоальбом