Содержание
- 2. 11-Aug-23 Lecture 9 Uniform Distribution. Normal (Gaussian Distribution) Distributions.
- 3. 11-Aug-23 Contents Uniform distribution – the general view The Normal Distribution
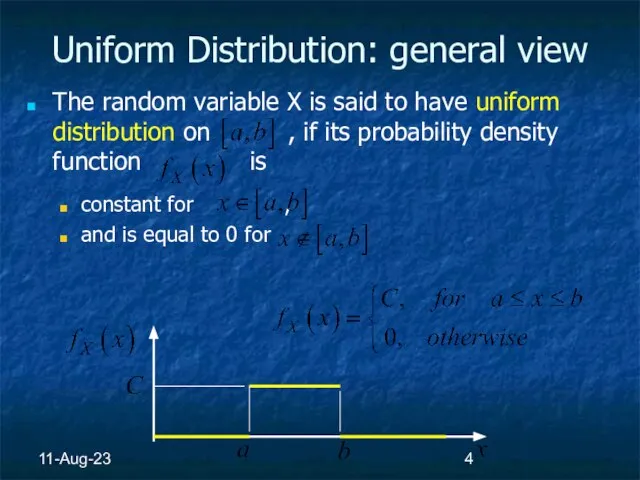
- 4. 11-Aug-23 Uniform Distribution: general view The random variable X is said to have uniform distribution on
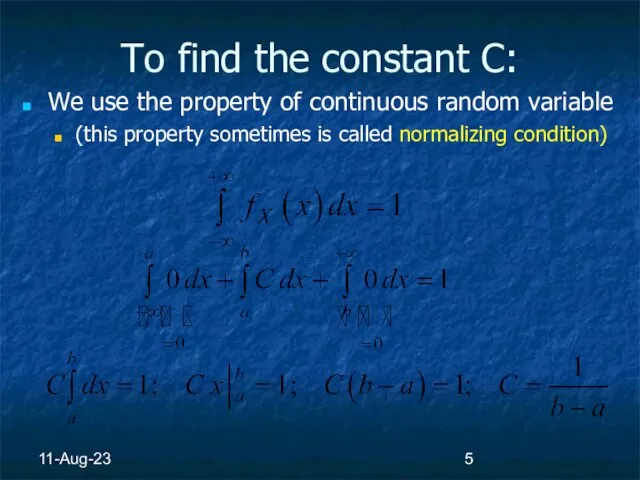
- 5. 11-Aug-23 To find the constant C: We use the property of continuous random variable (this property
- 6. 11-Aug-23 So we have in the general terms: For example, if we are interesting in probability
- 7. 11-Aug-23 To find the expectation of the uniformly distributed random variable: We remember the definition of
- 8. 11-Aug-23 To find the expectation of the uniformly distributed random variable: For the uniform distribution
- 9. 11-Aug-23 To find the variance of the uniformly distributed random variable: We remember the definition of
- 10. 11-Aug-23 To find the variance of the uniformly distributed random variable: Substituting the value of and
- 11. 11-Aug-23 To find the variance of the uniformly distributed random variable:
- 12. 11-Aug-23 So the variance of the uniformly distributed random variable: It could be calculated as
- 13. 11-Aug-23 To find the cumulative distribution function for uniform distribution We use the definition of cumulative
- 14. 11-Aug-23 To find the cumulative distribution function for uniform distribution 2. For 3. For
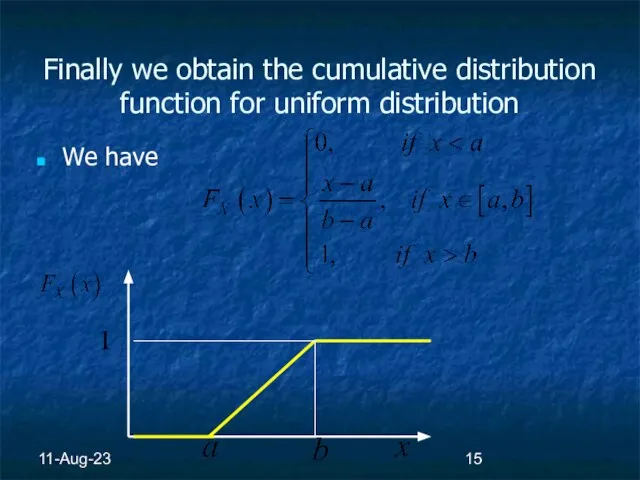
- 15. 11-Aug-23 Finally we obtain the cumulative distribution function for uniform distribution We have
- 16. 11-Aug-23 The Normal Distribution We introduce now a continuous distribution that plays a central role in
- 17. 11-Aug-23 The Normal Distribution If the average score on the test is 60, we would expect
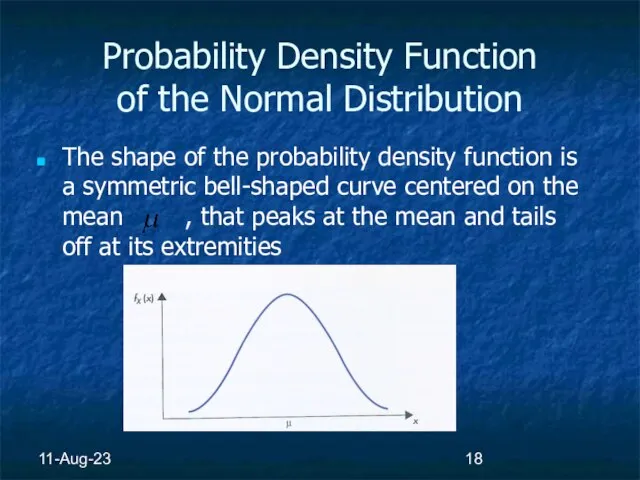
- 18. 11-Aug-23 Probability Density Function of the Normal Distribution The shape of the probability density function is
- 19. 11-Aug-23 Probability Density Function of the Normal Distribution If the random variable X has probability density
- 20. 11-Aug-23 Comments It can be seen from the definition that there is not a single normal
- 21. 11-Aug-23 Some Properties of the Normal Distribution Suppose that the random variable X follows a normal
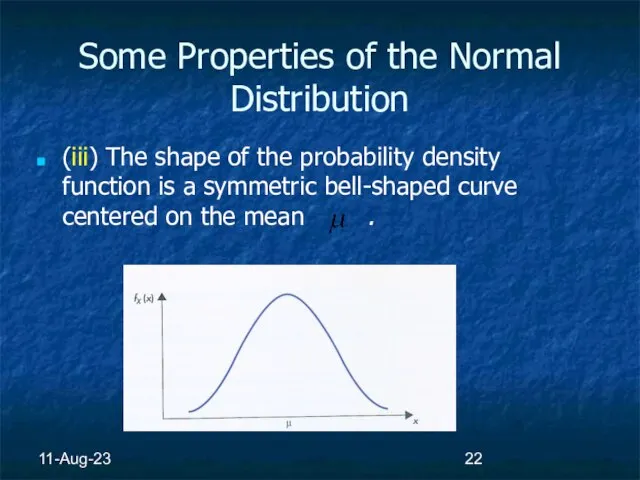
- 22. 11-Aug-23 Some Properties of the Normal Distribution (iii) The shape of the probability density function is
- 23. 11-Aug-23 Comments & Notation It follows from these properties that given the mean and variance of
- 24. 11-Aug-23 Comments Now, the mean of any distribution provides a measure of central location, while the
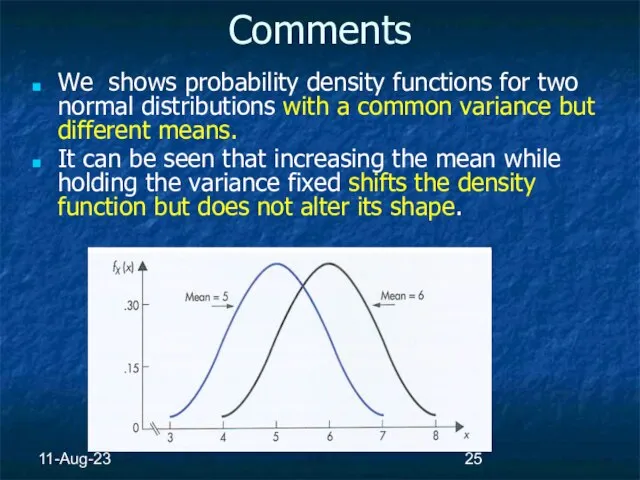
- 25. 11-Aug-23 Comments We shows probability density functions for two normal distributions with a common variance but
- 26. 11-Aug-23 Comments The two density functions are of normal random variables with a common mean but
- 27. 11-Aug-23 Cumulative Distribution Function of the Normal Distribution An extremely important practical question concerns the determination
- 28. 11-Aug-23 Cumulative Distribution Function of the Normal Distribution Suppose that X is a normal random variable
- 29. 11-Aug-23 Cumulative Distribution Function of the Normal Distribution The shaded area is the probability that X
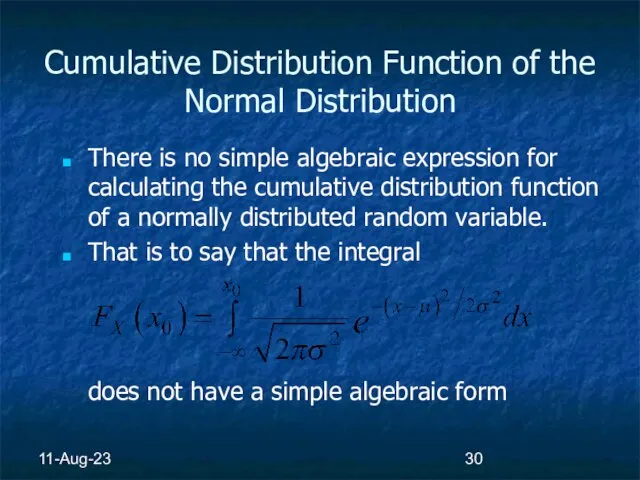
- 30. 11-Aug-23 Cumulative Distribution Function of the Normal Distribution There is no simple algebraic expression for calculating
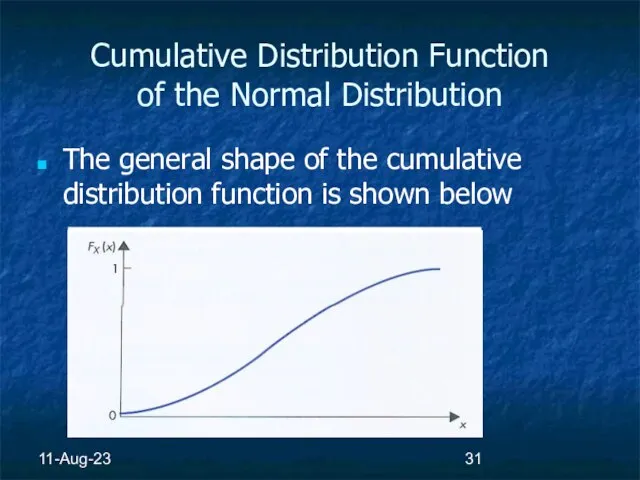
- 31. 11-Aug-23 Cumulative Distribution Function of the Normal Distribution The general shape of the cumulative distribution function
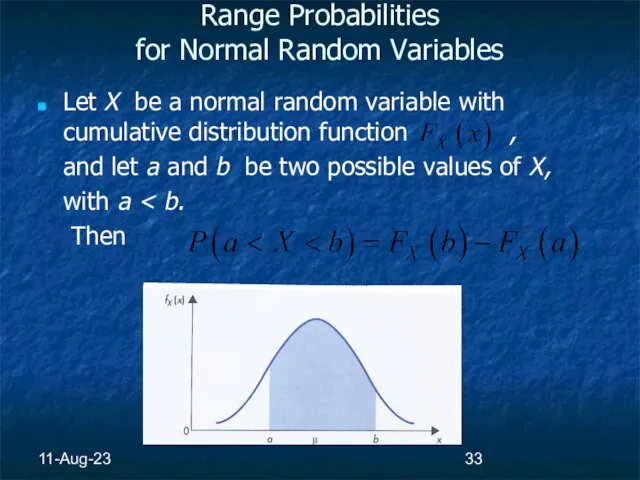
- 32. 11-Aug-23 Range Probabilities for Normal Random Variables We have already seen that for any continuous random
- 33. 11-Aug-23 Range Probabilities for Normal Random Variables Let X be a normal random variable with cumulative
- 34. 11-Aug-23 Range Probabilities for Normal Random Variables Any required probability can be obtained from the cumulative
- 35. 11-Aug-23 Range Probabilities for Normal Random Variables However, it would be enormously tedious if we had
- 36. 11-Aug-23 The Standard Normal Distribution We now introduce the particular distribution that is used for this
- 37. 11-Aug-23 The Standard Normal Distribution If the cumulative distribution function of this random variable is denoted
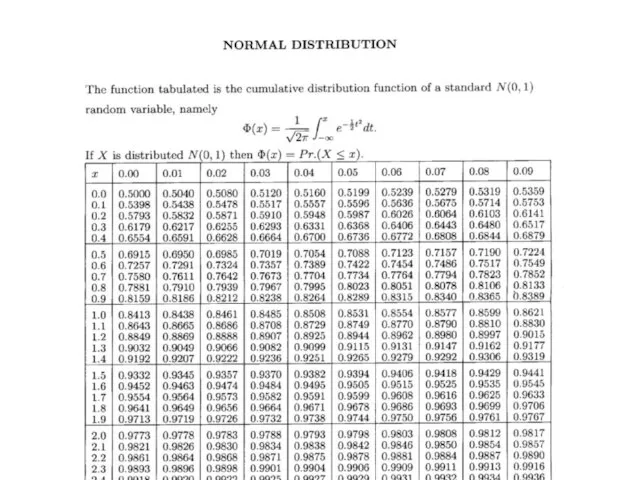
- 38. 11-Aug-23 The table of Normal Distribution This table gives values of for nonnegative values of z.
- 39. 11-Aug-23
- 40. 11-Aug-23 Values of the cumulative distribution function for negative values of z can be inferred from
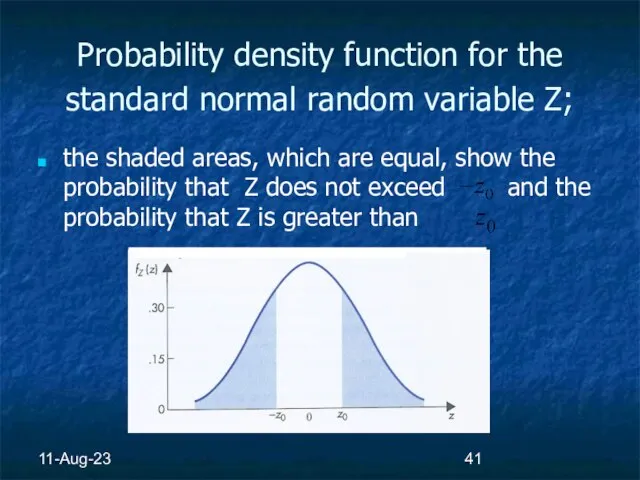
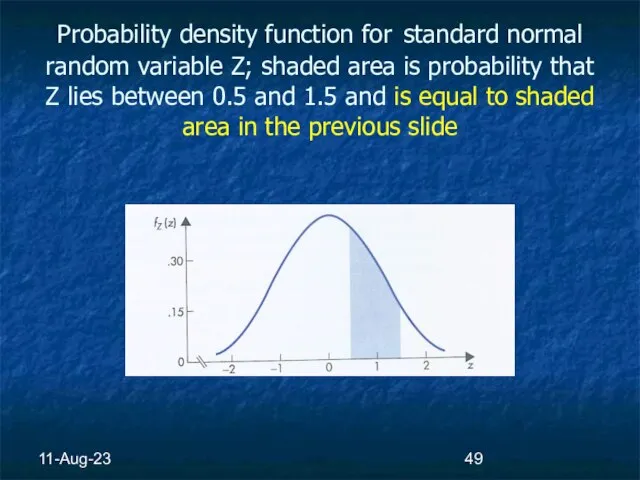
- 41. 11-Aug-23 Probability density function for the standard normal random variable Z; the shaded areas, which are
- 42. 11-Aug-23 Moreover, since the total area under the curve is 1: Hence, it follows that For
- 43. 11-Aug-23 Example If Z is a standard normal random variable, find The required probability is Then,
- 44. 11-Aug-23 How can probabilities for any normal random variable be expressed in terms of those for
- 45. 11-Aug-23 How can probabilities for any normal random variable be expressed in terms of those for
- 46. 11-Aug-23 Finding Range Probabilities for Normal Random Variables Let X be a normal random variable with
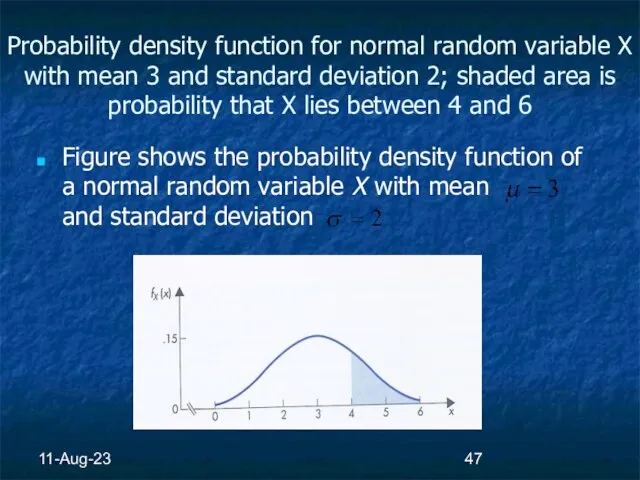
- 47. 11-Aug-23 Probability density function for normal random variable X with mean 3 and standard deviation 2;
- 48. 11-Aug-23 Probability density function for normal random variable X with mean 3 and standard deviation 2;
- 49. 11-Aug-23 Probability density function for standard normal random variable Z; shaded area is probability that Z
- 50. 11-Aug-23 Example If X ~ N(15, 16), find the probability that X is larger than 18.
- 51. 11-Aug-23 Example If X is normally distributed with mean 3 and standard deviation 2, find P(4
- 52. 11-Aug-23 Example A company produces lightbulbs whose lifetimes follow a normal distribution with mean 1,200 hours
- 53. 11-Aug-23 Example. Then Hence, the probability is approximately 0.54 that a lightbulb will last between 900
- 54. 11-Aug-23 Example A very large group of students obtains test scores that are normally distributed with
- 55. 11-Aug-23 Example Then we have That is, 3.76% of the students obtained scores in the range
- 56. 11-Aug-23 Example For the test scores of the previous Example, find the cutoff point for the
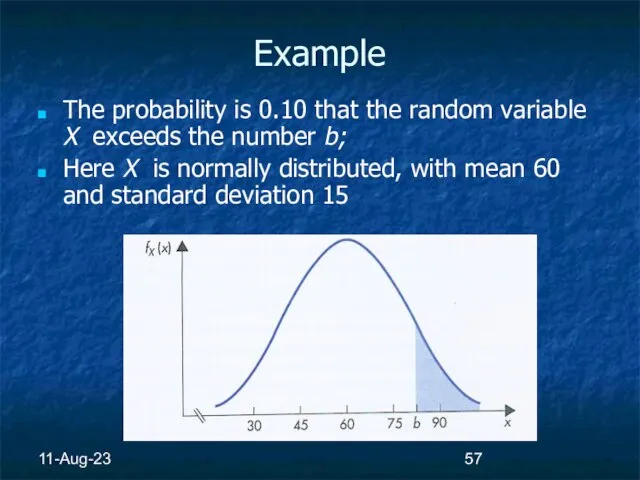
- 57. 11-Aug-23 Example The probability is 0.10 that the random variable X exceeds the number b; Here
- 58. 11-Aug-23 Example Let the number b denote the minimum score needed to be in the highest
- 59. 11-Aug-23 Example So Hence, it follows that Now, from Table, if then z = 1.28.
- 60. 11-Aug-23 Example Therefore, we have So The conclusion is that 10% of the students obtain scores
- 61. 11-Aug-23 Comments In Examples, if the scores awarded on the test were integers, the distribution of
- 63. Скачать презентацию














































 Русские народные загадки
Русские народные загадки Презентация на тему School subjects (Школьные предметы)
Презентация на тему School subjects (Школьные предметы)  Сложение чисел с помощью координатной прямой
Сложение чисел с помощью координатной прямой Горловский колледж промышленных технологий и экономики
Горловский колледж промышленных технологий и экономики МОУ Чурачикская СОШ Цивильского района ЧР
МОУ Чурачикская СОШ Цивильского района ЧР Аналоговые телефонные шлюзы компании AudioCodes Ltd. Серия MediaPack Версия 4.4
Аналоговые телефонные шлюзы компании AudioCodes Ltd. Серия MediaPack Версия 4.4 Владимиров В.Н., Дёмкин А.В., Киселева Е.А., Колдаков Д.В., Перебоев Р.Н., Силина И.Г.Алтайский государственный университет, г.Барнаул,
Владимиров В.Н., Дёмкин А.В., Киселева Е.А., Колдаков Д.В., Перебоев Р.Н., Силина И.Г.Алтайский государственный университет, г.Барнаул,  Проектное основание
Проектное основание Информационная карта как технология описания инновационного опыта
Информационная карта как технология описания инновационного опыта Анализ стартовой ситуации. Маркетинг и сбыт
Анализ стартовой ситуации. Маркетинг и сбыт Современное состояние и охрана атмосферы
Современное состояние и охрана атмосферы Материально-техническое обеспечение и оснащенность образовательного процесса БОУ СОШ №31 МО Динской район
Материально-техническое обеспечение и оснащенность образовательного процесса БОУ СОШ №31 МО Динской район ОБ ЭЛЕКТРОЭНЕРГИИ ОСНОВАННОЙ НА НЕЗАМКНУТЫХ ТОКАХ
ОБ ЭЛЕКТРОЭНЕРГИИ ОСНОВАННОЙ НА НЕЗАМКНУТЫХ ТОКАХ Презентация на тему Коррекционная работа по развитию связной письменной речи
Презентация на тему Коррекционная работа по развитию связной письменной речи Стили фотографий и обработка
Стили фотографий и обработка Взгляд с другой стороны баррикад, или чего же на самом деле хочет Клиент
Взгляд с другой стороны баррикад, или чего же на самом деле хочет Клиент Christopher Columbus
Christopher Columbus Правозащитная экспертиза
Правозащитная экспертиза Повесть о Шемякином суде. ΧVІІ век
Повесть о Шемякином суде. ΧVІІ век Морская стихия. 2 класс
Морская стихия. 2 класс Дорожные знаки 5 класс
Дорожные знаки 5 класс Презентация на тему Конвекция о правах ребенка
Презентация на тему Конвекция о правах ребенка Орыс революциясыны азастана сері
Орыс революциясыны азастана сері 185 пример расчета и применение теплозащиты Мищенко
185 пример расчета и применение теплозащиты Мищенко Обряды и обычаи русского народа
Обряды и обычаи русского народа Полуфабрикаты из мяса
Полуфабрикаты из мяса Модель методического сопровождения педагогов, проучившихся в ММЦ
Модель методического сопровождения педагогов, проучившихся в ММЦ Планета Венера
Планета Венера