Содержание
- 2. Test of population mean vs. hypothesized value, population standard deviation unknown
- 5. Exercise (13)
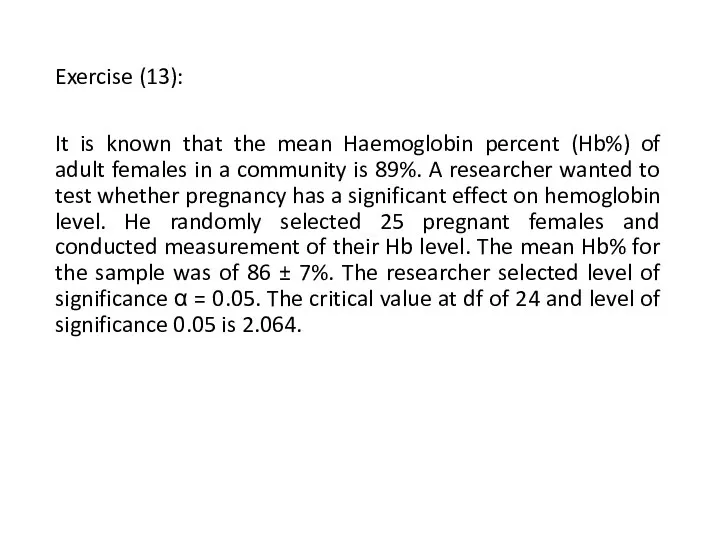
- 6. Exercise (13): It is known that the mean Haemoglobin percent (Hb%) of adult females in a
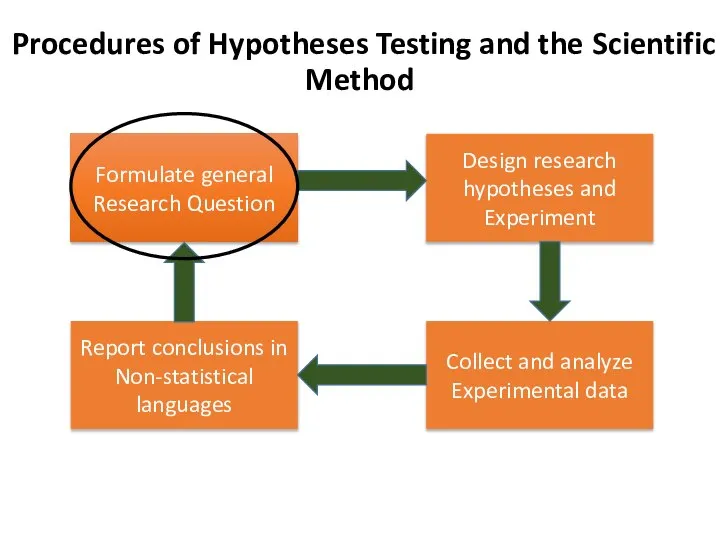
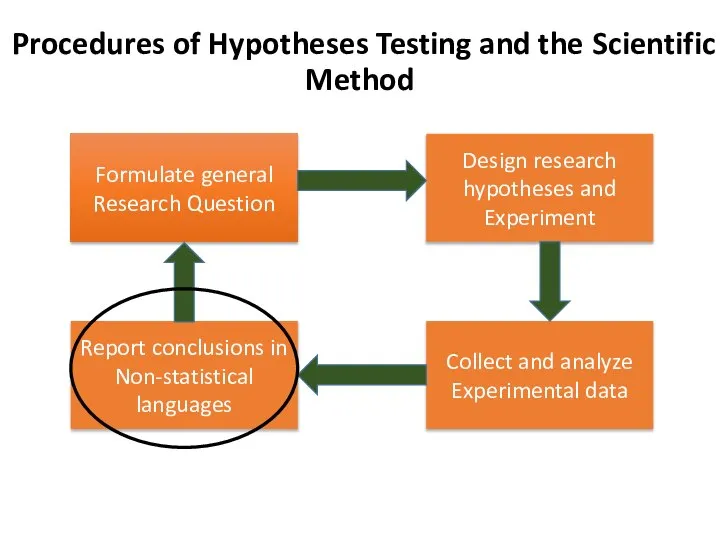
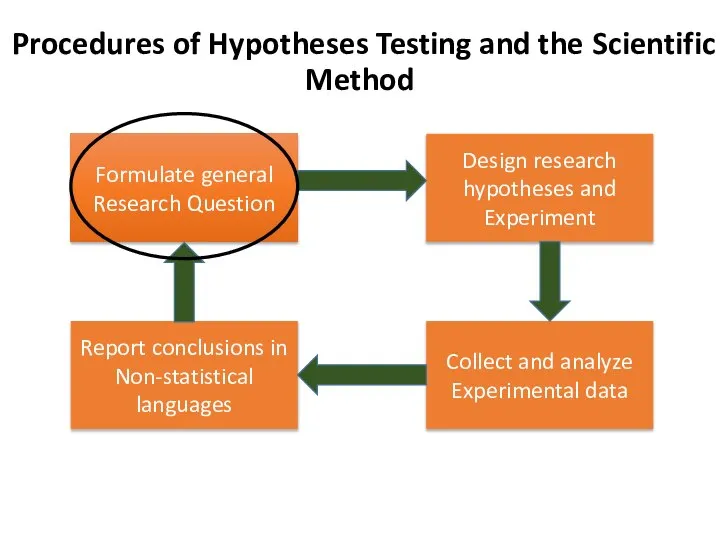
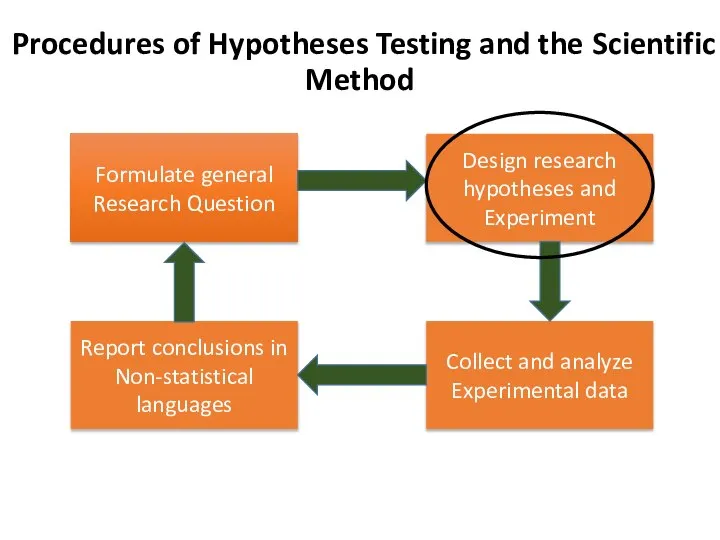
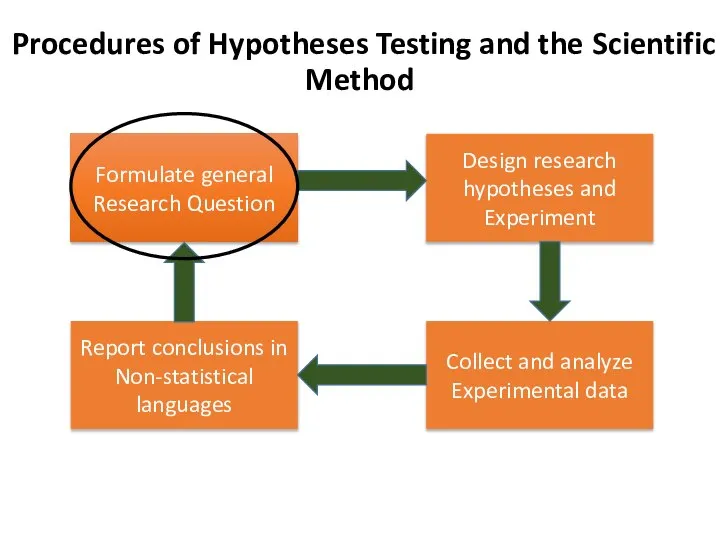
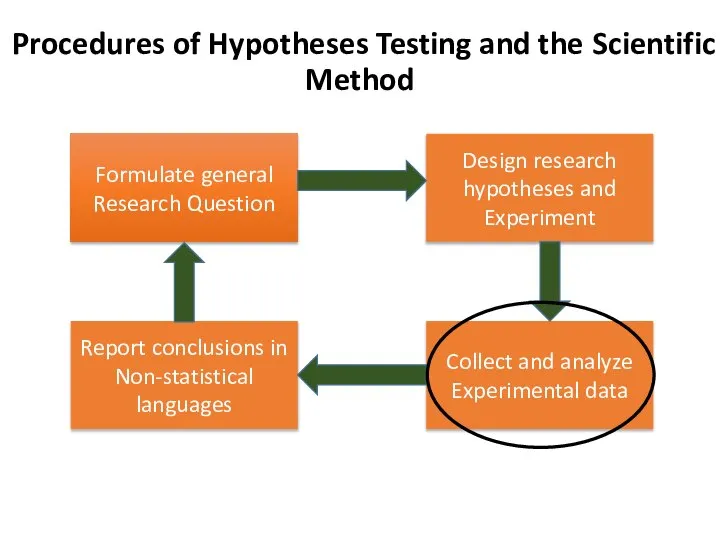
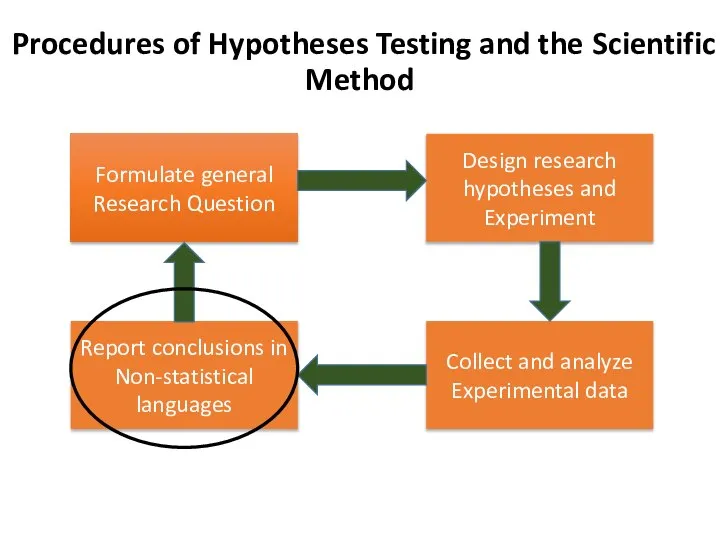
- 7. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 8. I. Formulate general Research Question Does pregnancy have significant effect on mean Hb%?
- 9. I. Formulate general Research Question Does pregnancy have significant effect on mean Hb%?
- 10. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
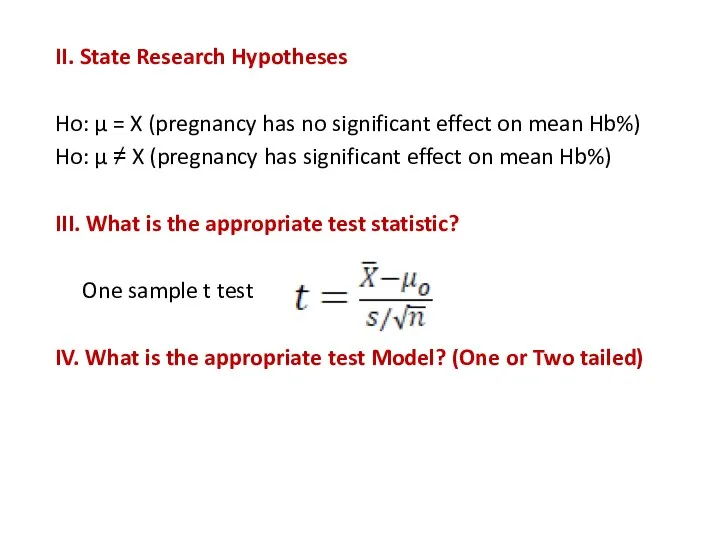
- 11. II. State Research Hypotheses Ho: µ = X (pregnancy has no significant effect on mean Hb%)
- 12. II. State Research Hypotheses Ho: µ = X (pregnancy has no significant effect on mean Hb%)
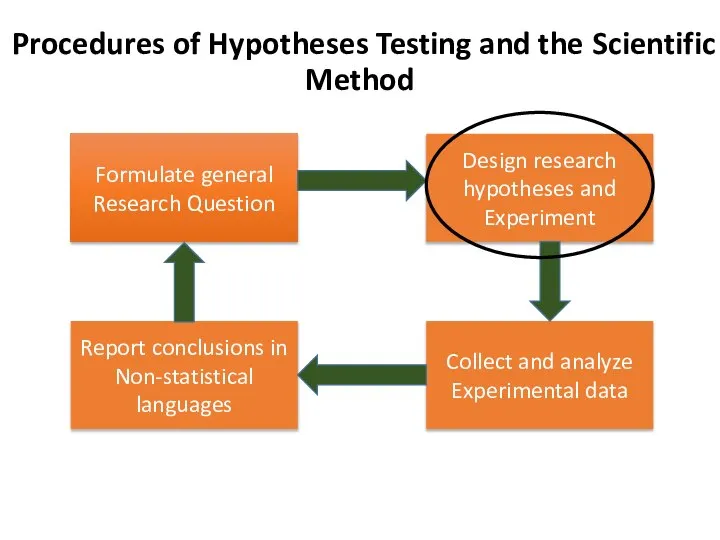
- 13. II. State Research Hypotheses Ho: µ = X (pregnancy has no significant effect on mean Hb%)
- 14. II. State Research Hypotheses Ho: µ = X (pregnancy has no significant effect on mean Hb%)
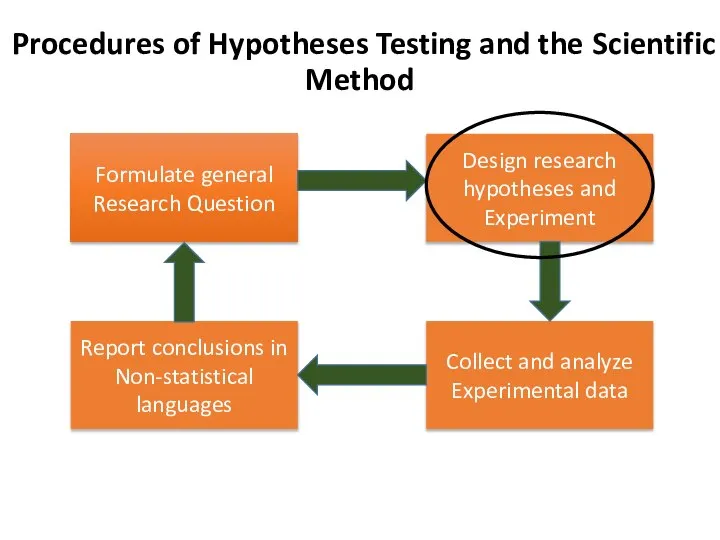
- 15. Design Research Hypotheses and Experiment
- 16. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
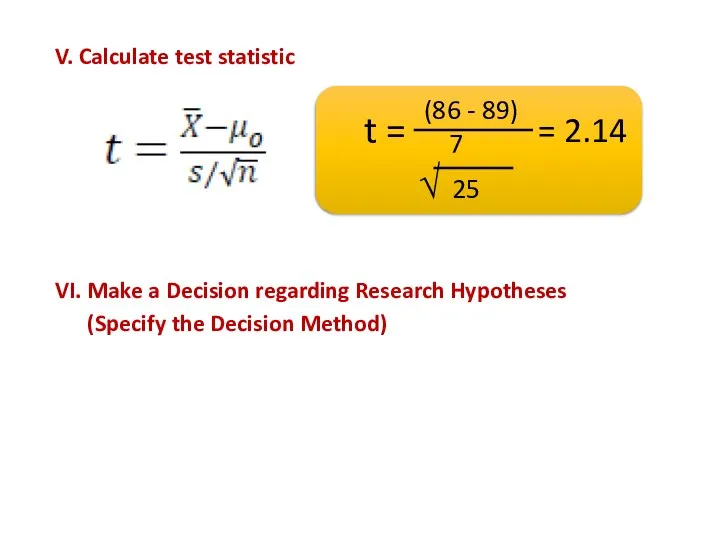
- 17. V. Calculate test statistic VI. Make a Decision regarding Research Hypotheses (Specify the Decision Method) Reject
- 18. V. Calculate test statistic VI. Make a Decision regarding Research Hypotheses (Specify the Decision Method) Reject
- 19. V. Calculate test statistic VI. Make a Decision regarding Research Hypotheses (Specify the Decision Method) Reject
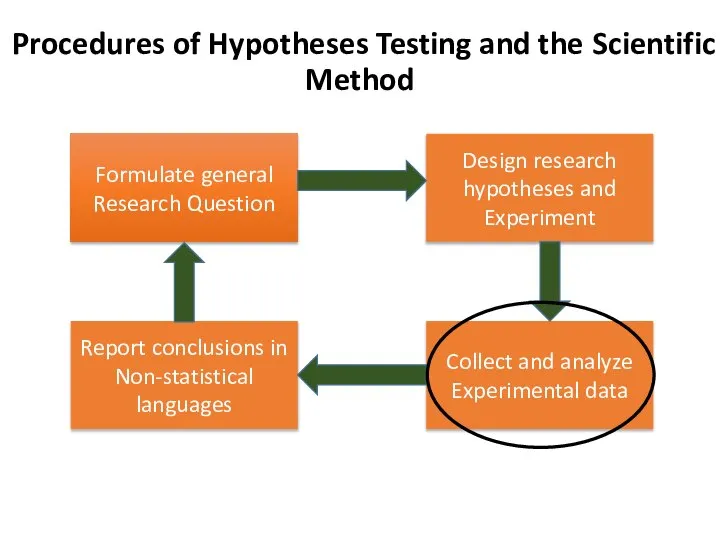
- 20. Collect and Analyze Experimental Data
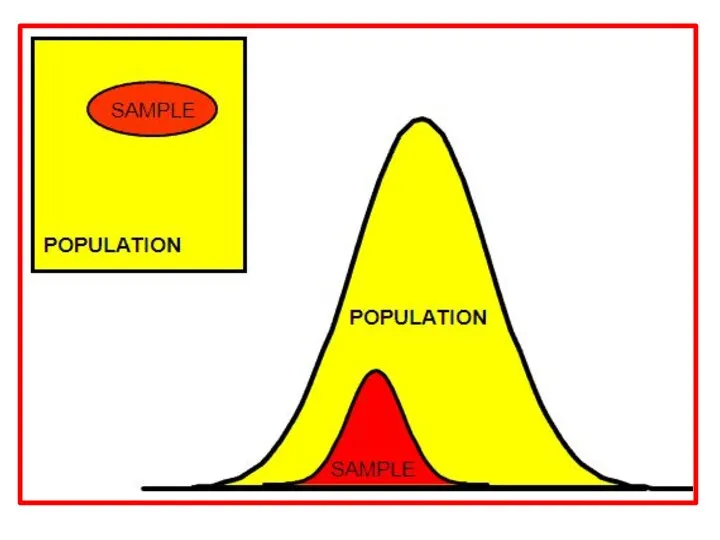
- 22. Reject Ho Fail to Reject Ho Fail to Reject Ho Reject Ho +1.96 -1.96 µ 89%
- 23. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 24. VII. Report a conclusion Pregnancy has a significant effect on mean Hb%. The mean Hb% of
- 25. VII. Report a conclusion Pregnancy has a significant effect on mean Hb%. The mean Hb% of
- 26. Comparing Means (Parametric tests) One Population Inference Two Population Inference Independent Sampling Model Dependent Sampling Model
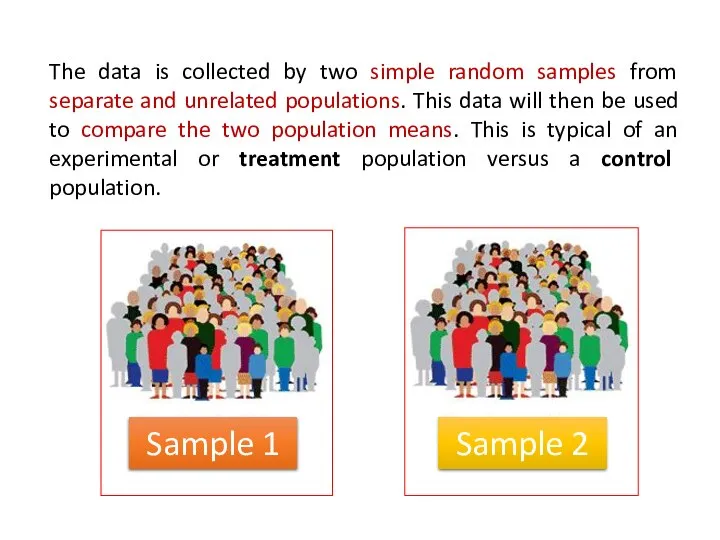
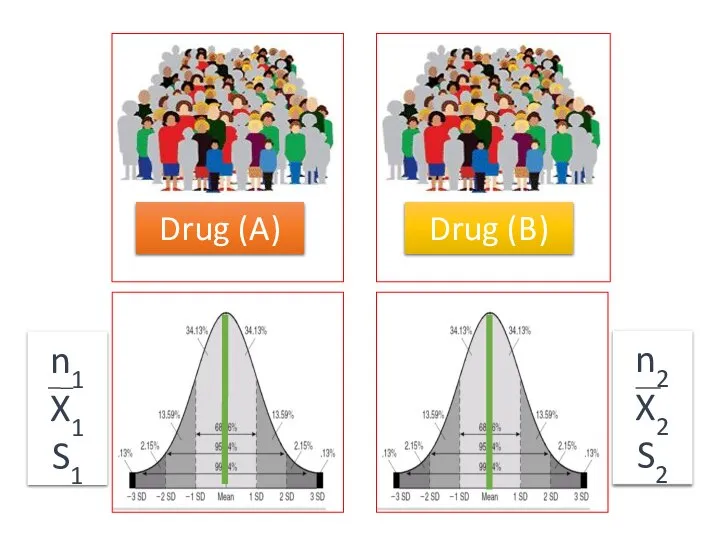
- 27. Sample 1 Sample 2 The data is collected by two simple random samples from separate and
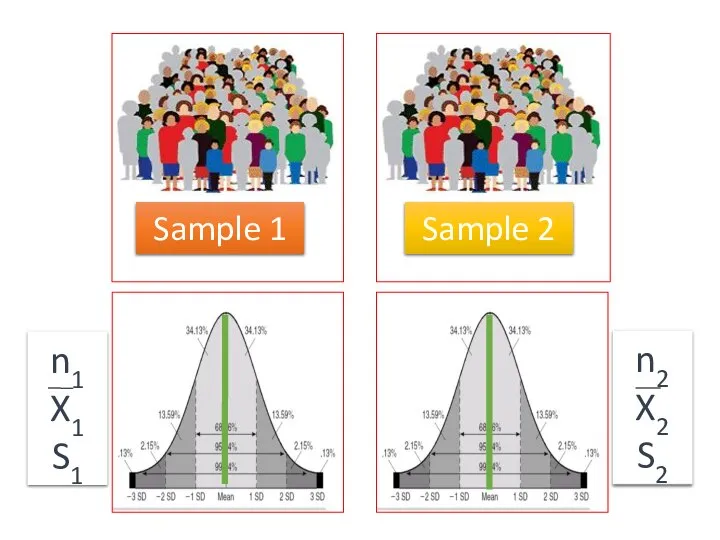
- 28. Sample 1 Sample 2 n1 X1 S1 n2 X2 S2
- 29. Exercise (14)
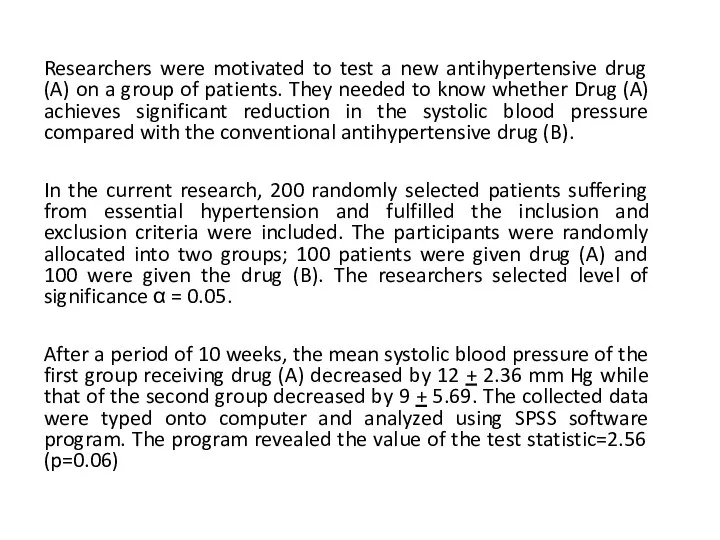
- 30. Researchers were motivated to test a new antihypertensive drug (A) on a group of patients. They
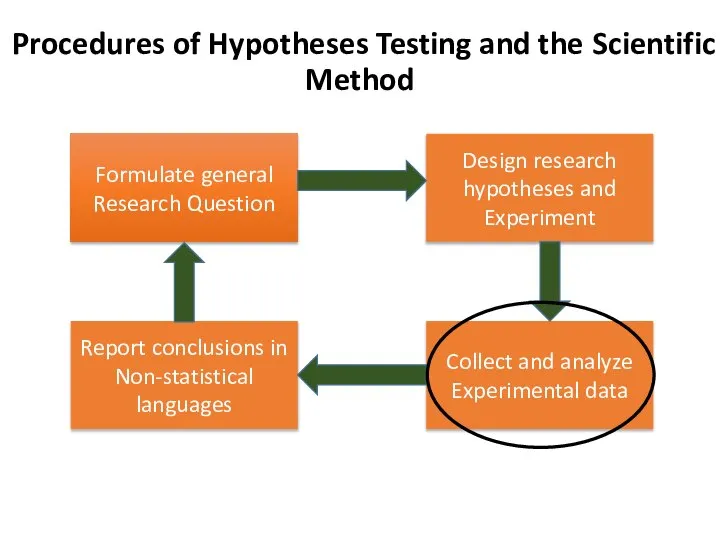
- 31. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 32. I. Formulate general Research Question Does pregnancy have significant effect on mean Hb%?
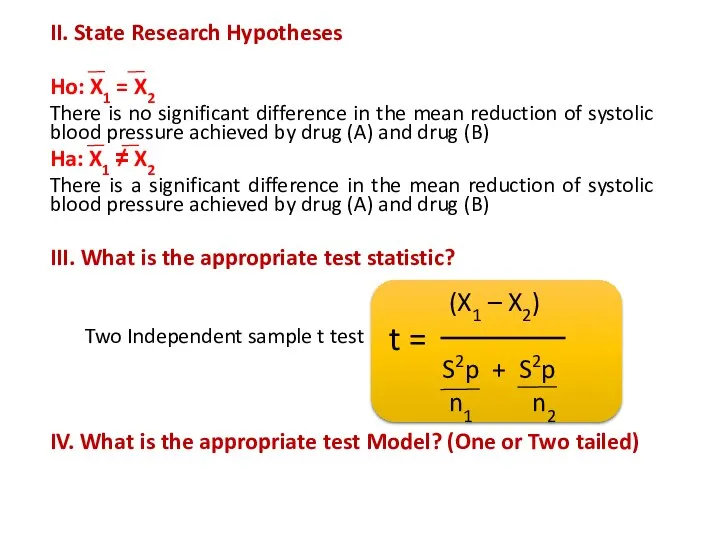
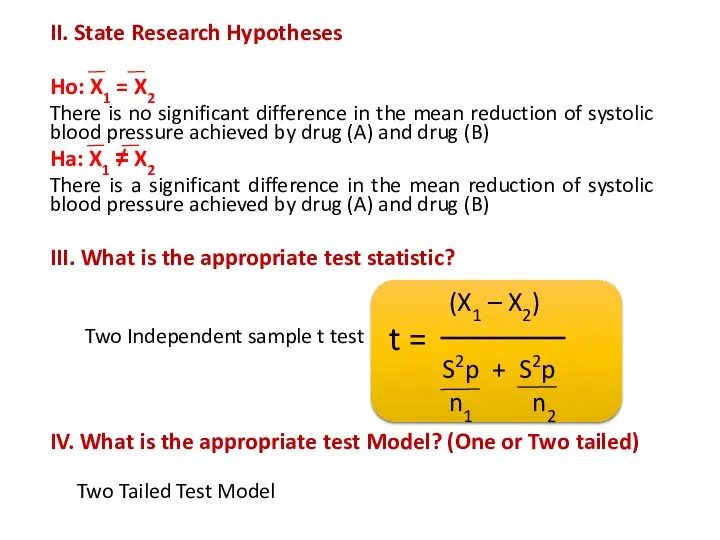
- 33. I. Formulate general Research Question Is there any significant difference in the mean reduction of systolic
- 34. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 35. II. State Research Hypotheses Ho: X1 = X2 There is no significant difference in the mean
- 36. II. State Research Hypotheses Ho: X1 = X2 There is no significant difference in the mean
- 37. II. State Research Hypotheses Ho: X1 = X2 There is no significant difference in the mean
- 38. II. State Research Hypotheses Ho: X1 = X2 There is no significant difference in the mean
- 39. Design Research Hypotheses and Experiment
- 40. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 41. V. Make a Decision regarding Research Hypotheses (Specify the Decision Method) Fail to Reject Null Hypothesis
- 42. Collect and Analyze Experimental Data
- 43. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 44. VI. Report a conclusion Pregnancy has a significant effect on mean Hb%. The mean Hb% of
- 45. VI. Report a conclusion There is insufficient evidence to support the claim that there is a
- 46. Drug (A) Drug (B) n1 X1 S1 n2 X2 S2
- 47. +1.96 -1.96 X1 9 X2 12 Fail to Reject Ho Reject Ho Reject Ho Fail to
- 48. Comparing Means (Parametric tests) One Population Inference Two Population Inference Independent Sampling Model Dependent Sampling Model
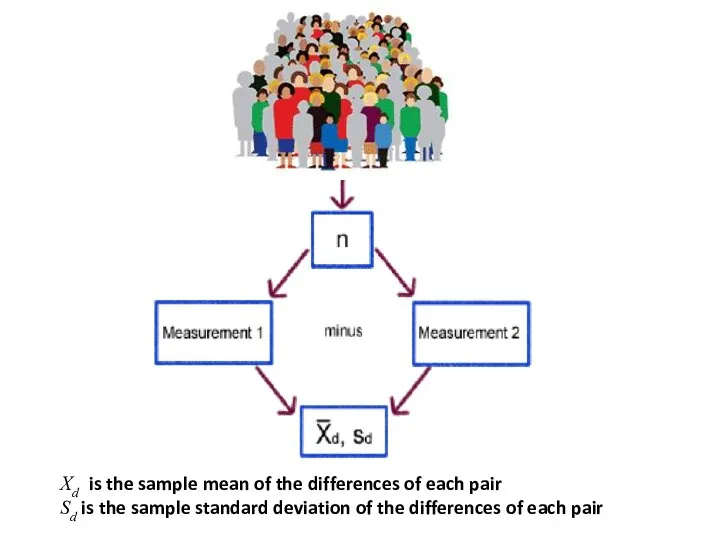
- 49. Sample The data consists of a single population and two measurements. A simple random sample is
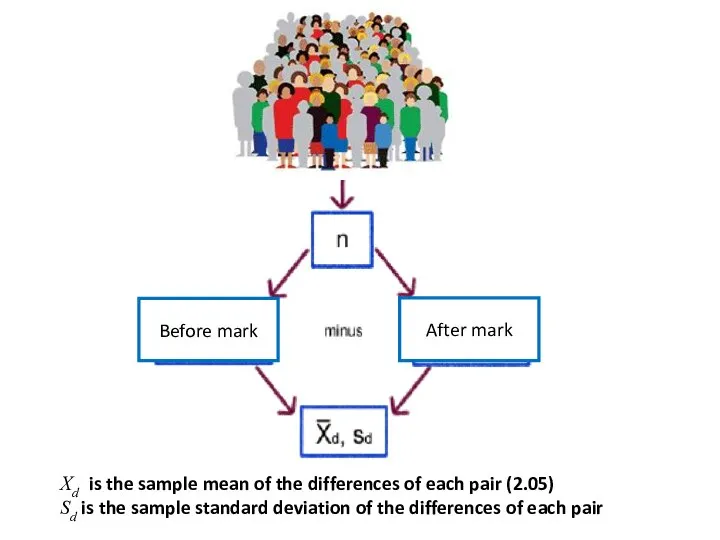
- 50. Xd is the sample mean of the differences of each pair Sd is the sample standard
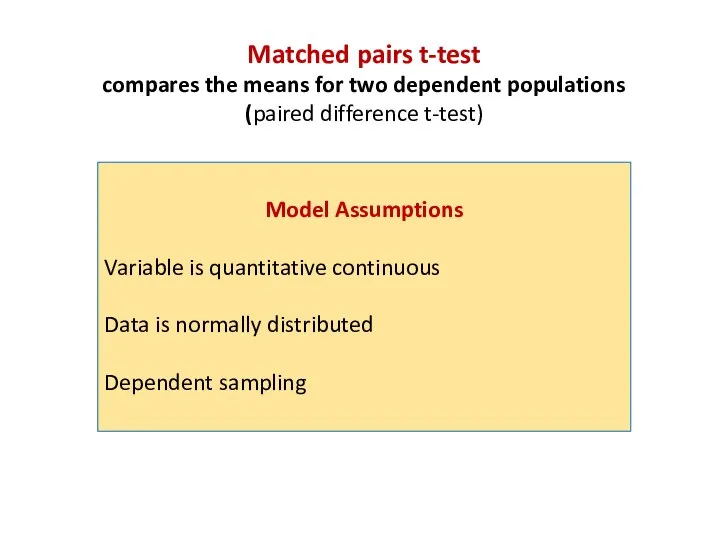
- 51. Matched pairs t-test compares the means for two dependent populations (paired difference t-test) Model Assumptions Variable
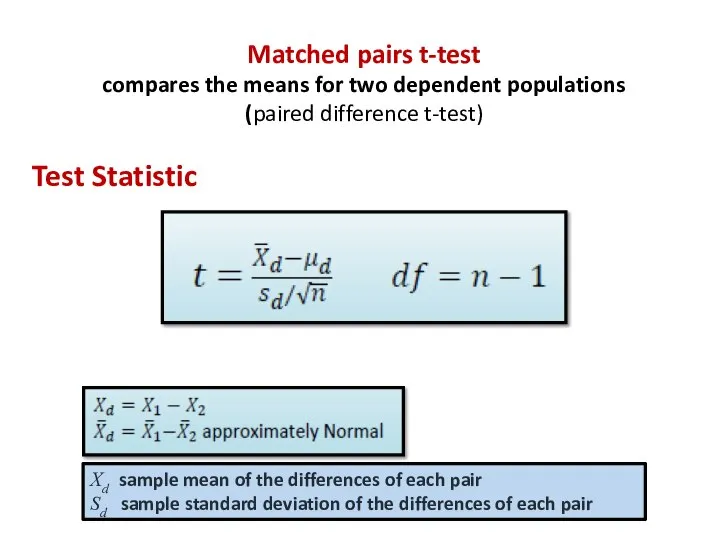
- 52. Matched pairs t-test compares the means for two dependent populations (paired difference t-test) Test Statistic Xd
- 53. Exercise (15)
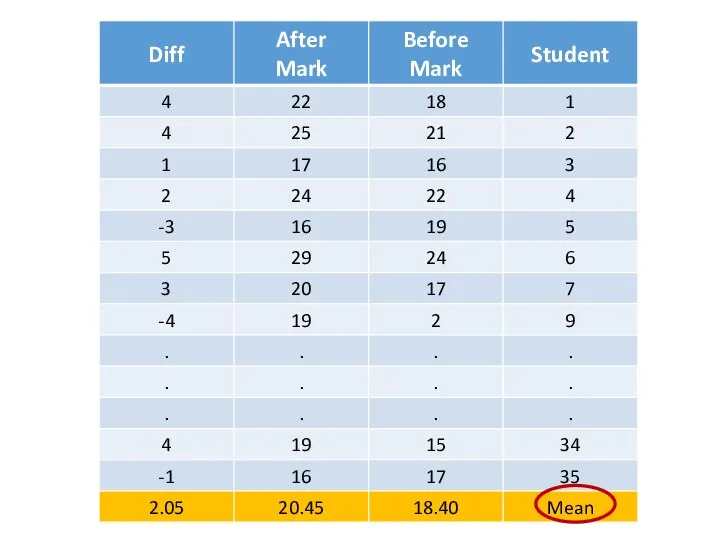
- 54. Exercise (15): An instructor of Anatomy course wants to know if student marks are different on
- 55. Xd is the sample mean of the differences of each pair (2.05) Sd is the sample
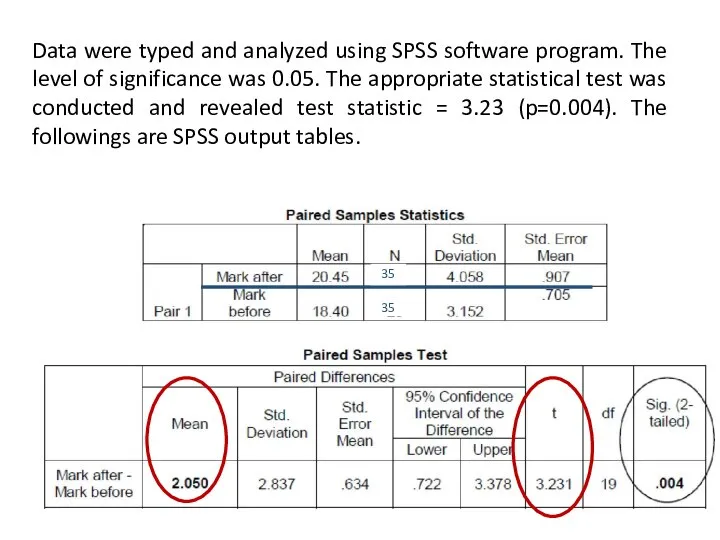
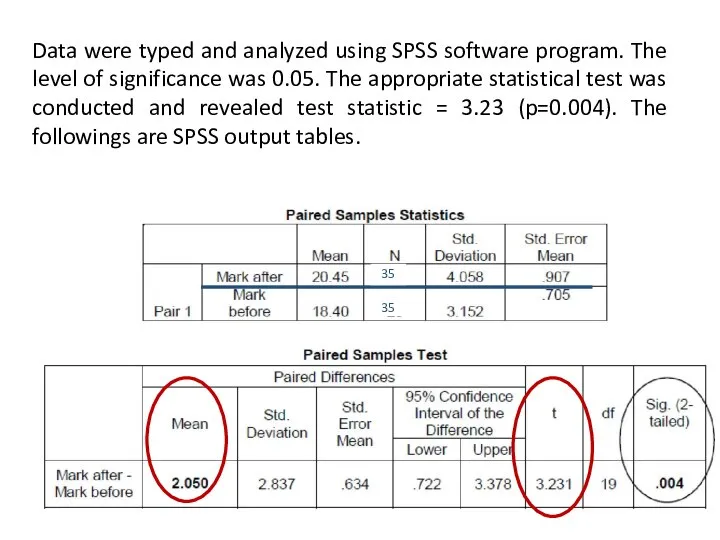
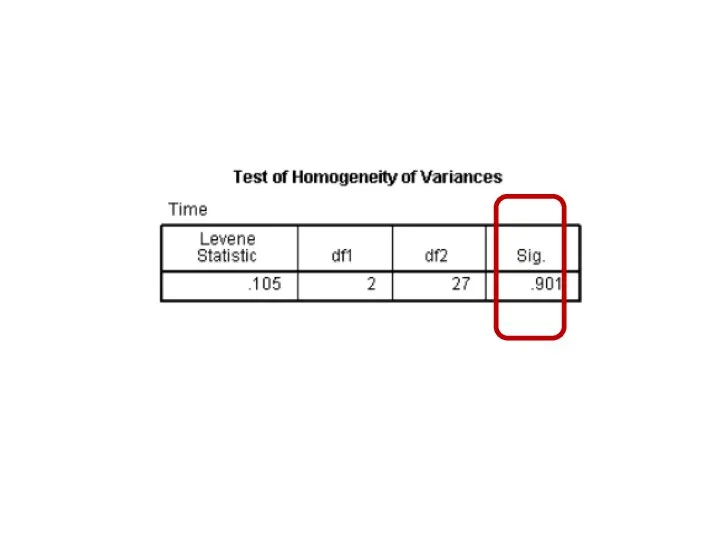
- 57. Data were typed and analyzed using SPSS software program. The level of significance was 0.05. The
- 58. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 59. I. Formulate general Research Question Does pregnancy have significant effect on mean Hb%?
- 60. I. Formulate general Research Question Is there a difference in students’ marks following implementation of TBL
- 61. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
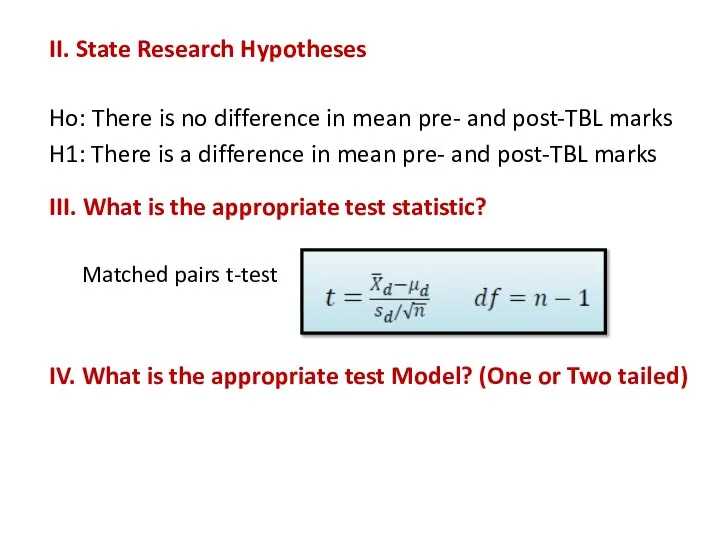
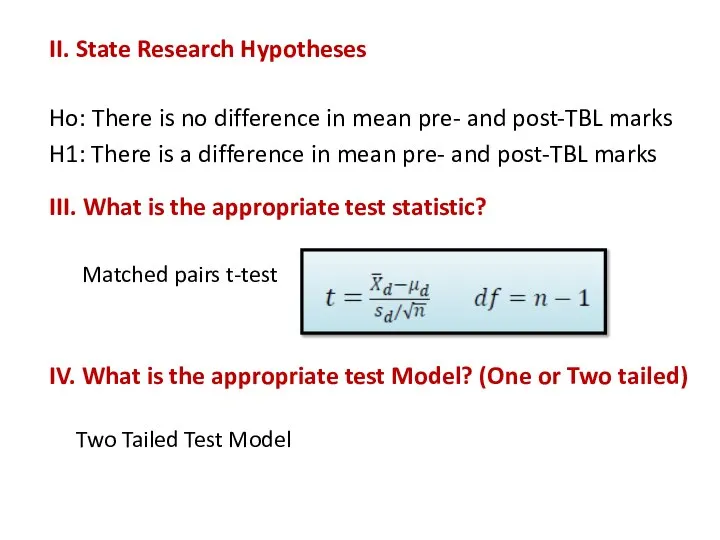
- 62. II. State Research Hypotheses Ho: There is no difference in mean pre- and post-TBL marks H1:
- 63. II. State Research Hypotheses Ho: There is no difference in mean pre- and post-TBL marks H1:
- 64. II. State Research Hypotheses Ho: There is no difference in mean pre- and post-TBL marks H1:
- 65. II. State Research Hypotheses Ho: There is no difference in mean pre- and post-TBL marks H1:
- 66. Design Research Hypotheses and Experiment
- 67. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 68. Collect and Analyze Experimental Data
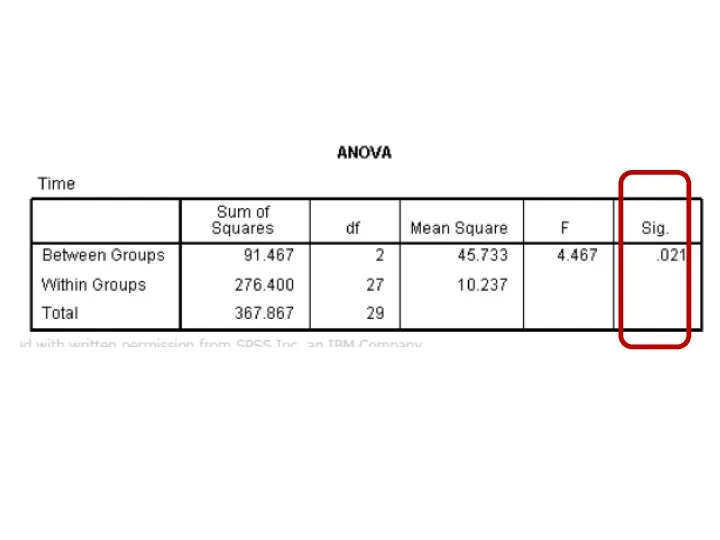
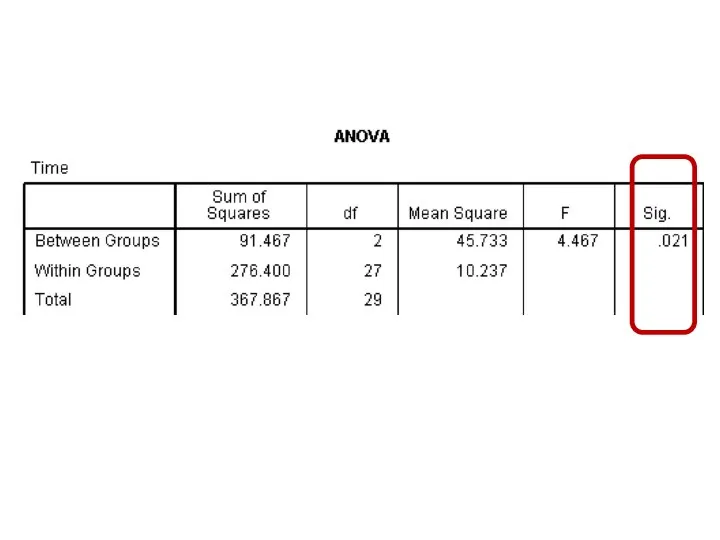
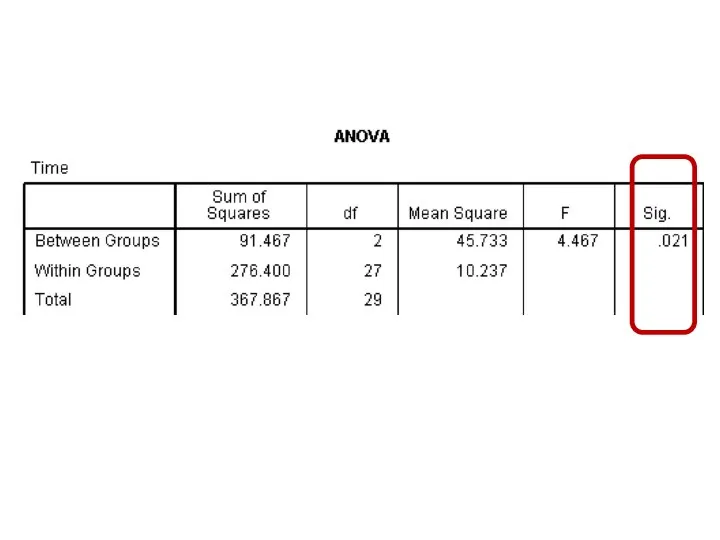
- 69. V. Make a Decision regarding Research Hypotheses (Specify the Decision Method) Reject Null Hypothesis Ho P-value
- 70. Data were typed and analyzed using SPSS software program. The level of significance was 0.05. The
- 71. V. Make a Decision regarding Research Hypotheses (Specify the Decision Method) Reject Null Hypothesis Ho P-value
- 72. Formulate general Research Question Procedures of Hypotheses Testing and the Scientific Method Design research hypotheses and
- 73. VI. Report a conclusion Pregnancy has a significant effect on mean Hb%. The mean Hb% of
- 74. VI. Report a conclusion There is a strong evidence (t = 3.23, p = 0.004) that
- 75. Xd is the sample mean of the differences of each pair (2.05) Sd is the sample
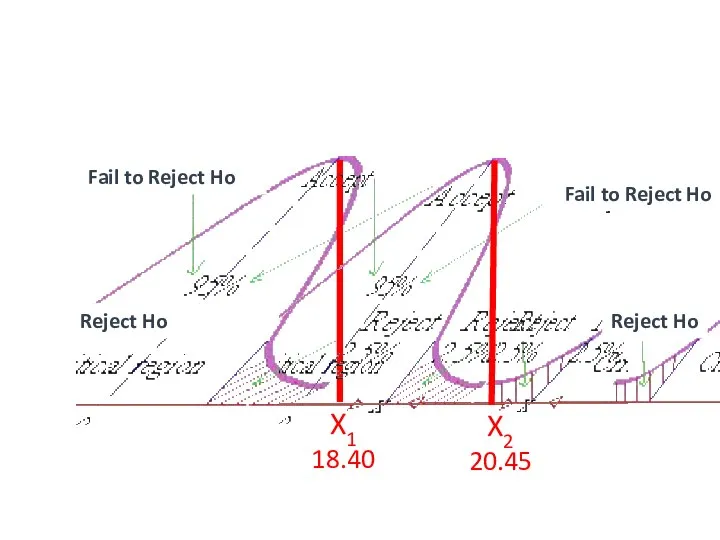
- 76. +1.96 -1.96 X1 18.40 X2 20.45 Fail to Reject Ho Reject Ho Reject Ho Fail to
- 77. Comparing Means (Parametric tests) One Population Inference Two Population Inference Independent Sampling Model Dependent Sampling Model
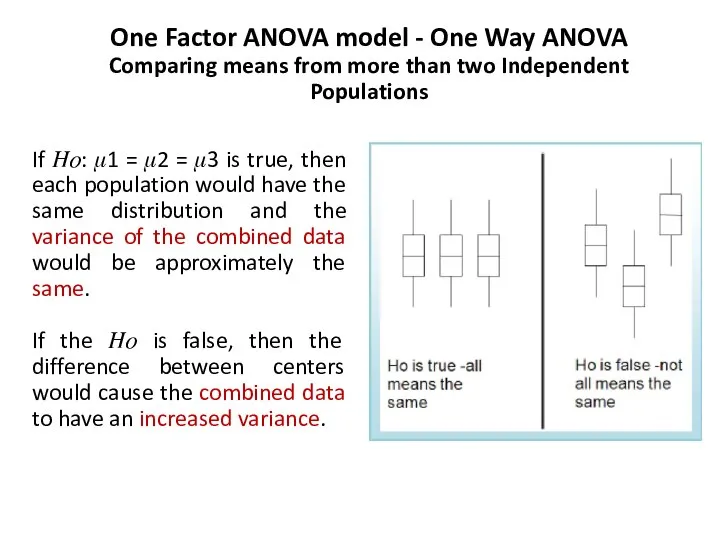
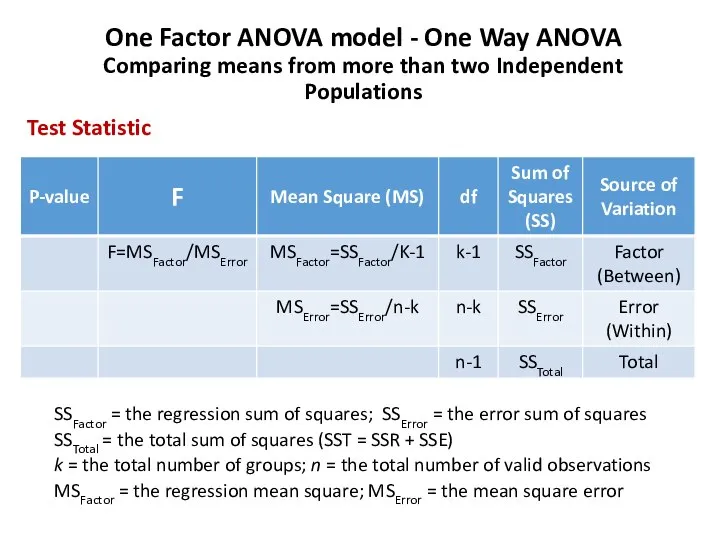
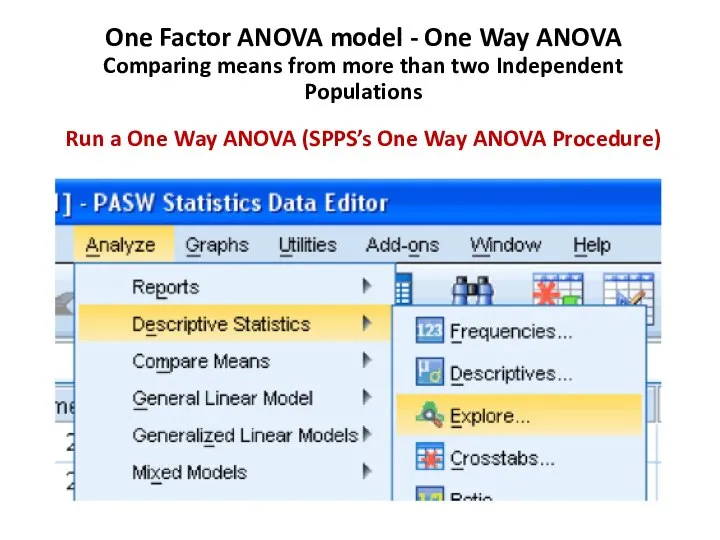
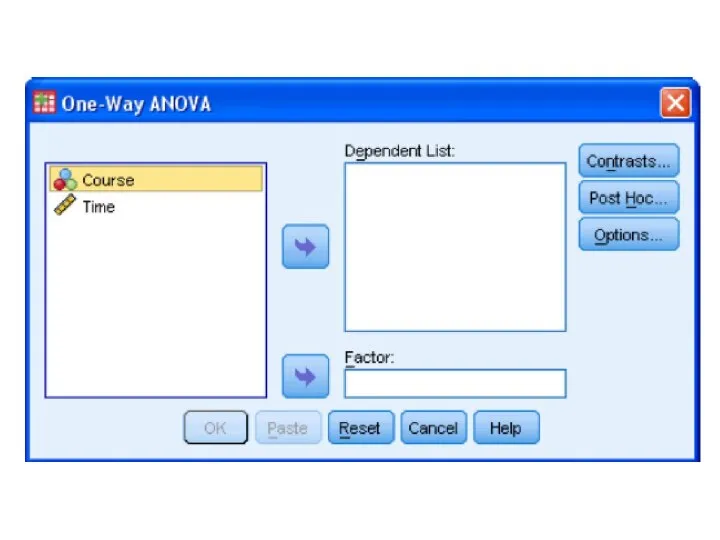
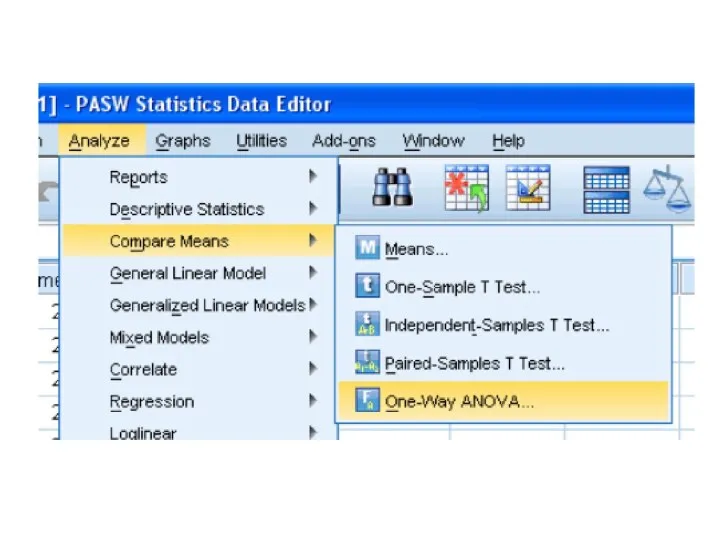
- 78. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 79. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
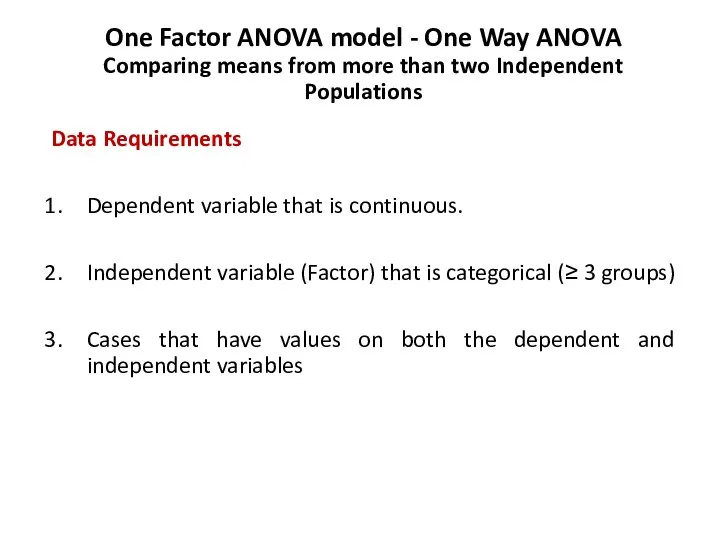
- 80. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 81. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 82. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
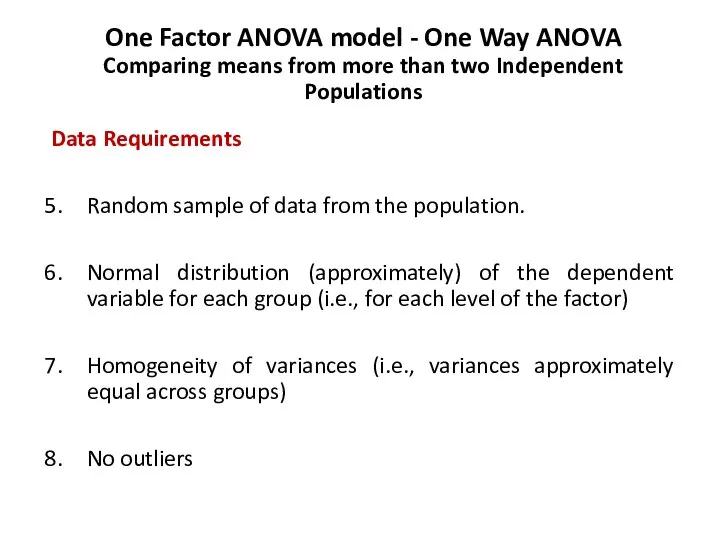
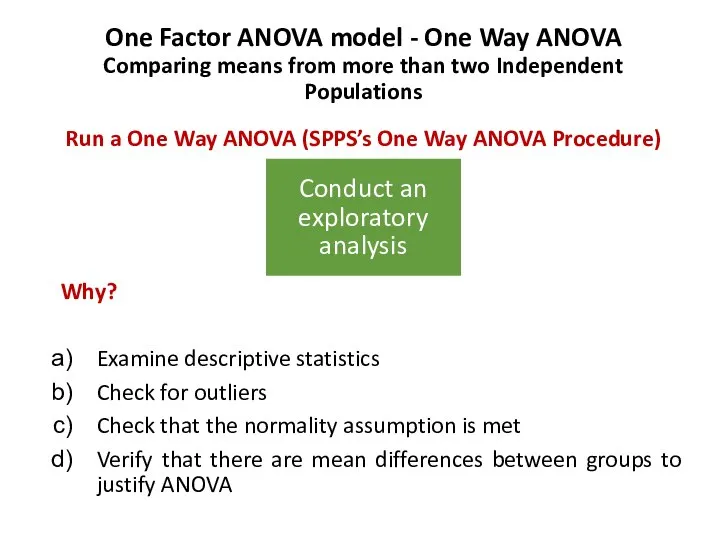
- 83. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 84. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 85. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
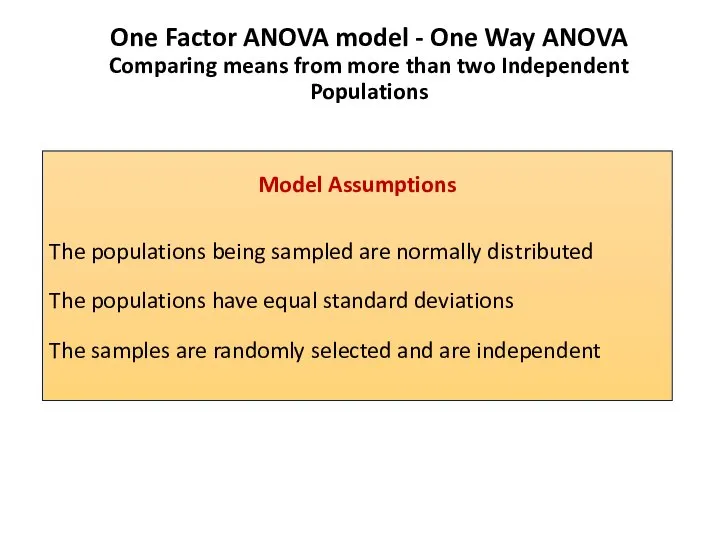
- 86. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
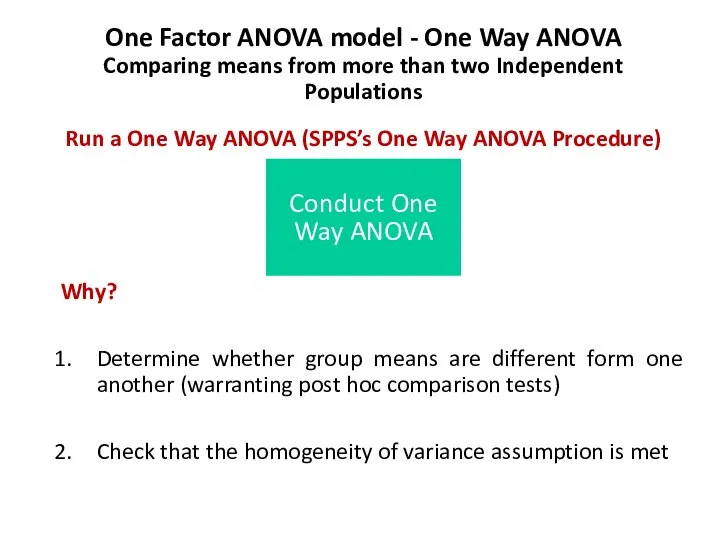
- 87. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 88. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 89. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 90. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
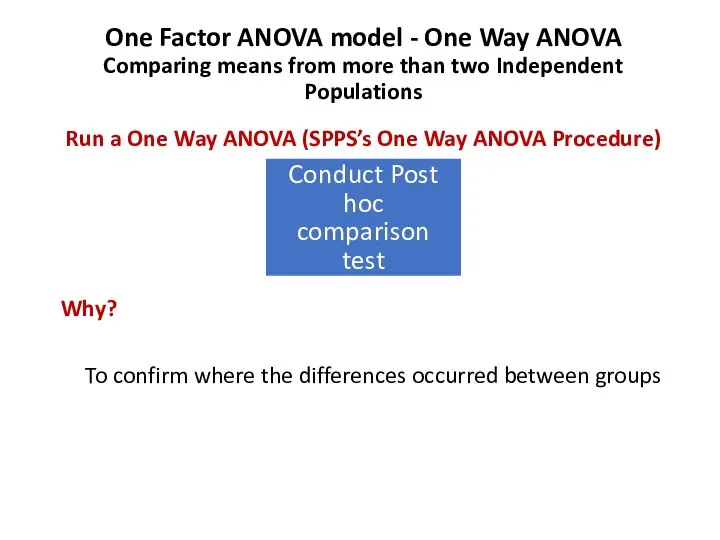
- 91. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 92. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
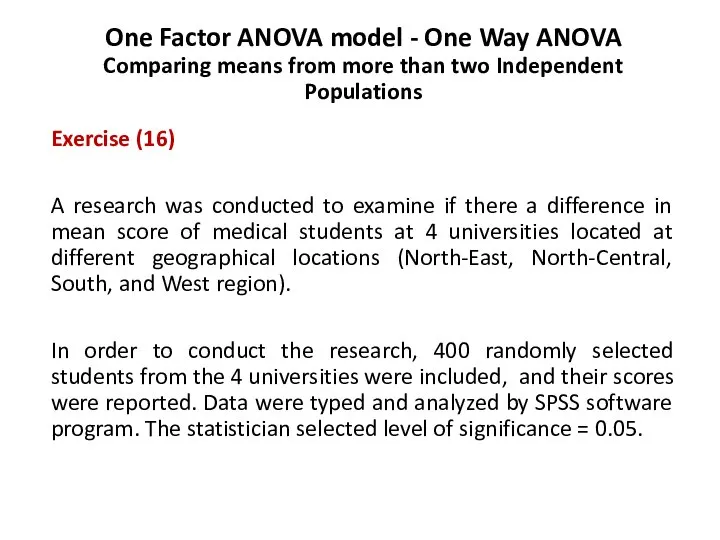
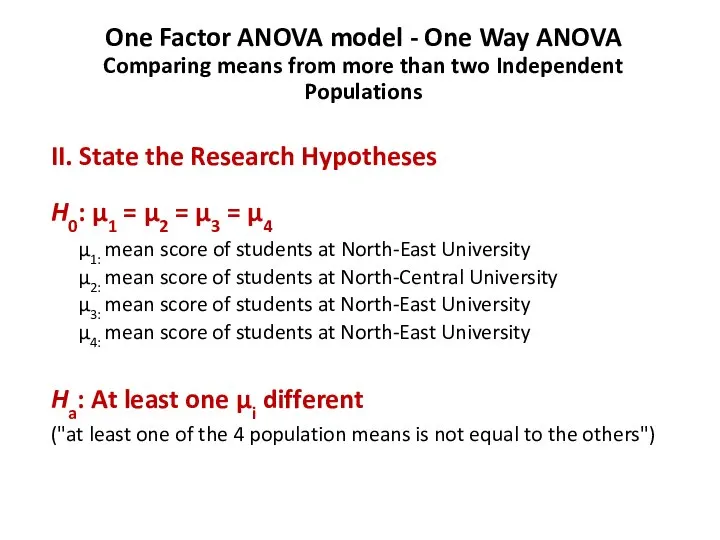
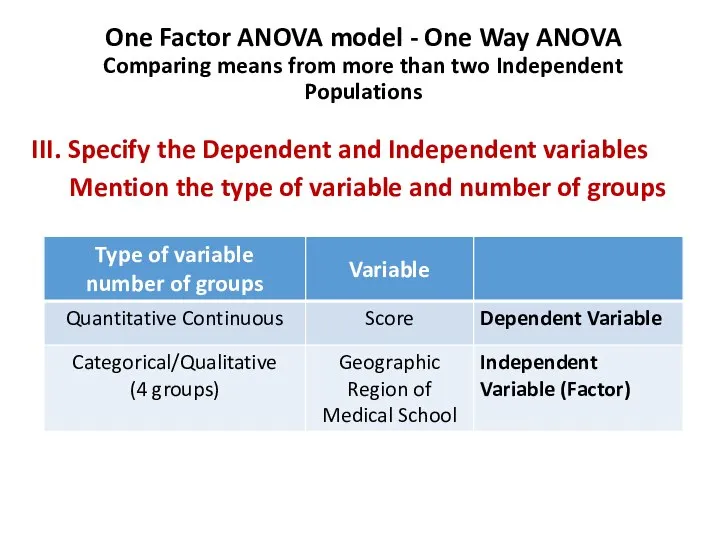
- 93. Exercise (16)
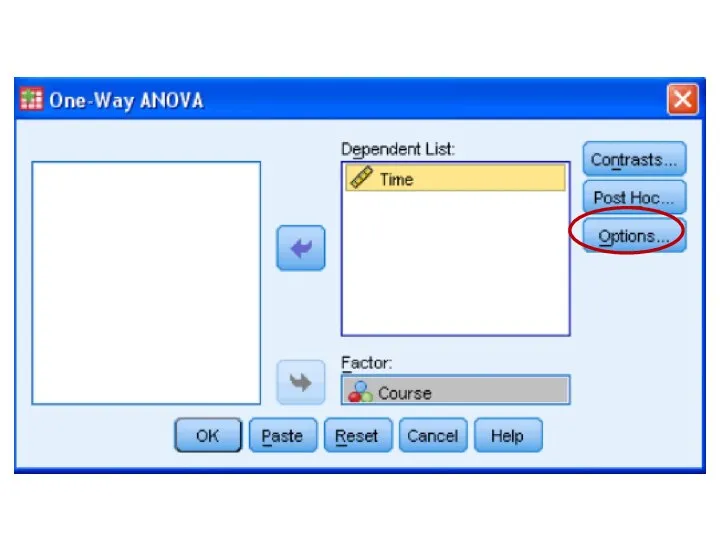
- 94. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 95. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 96. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 97. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 98. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 99. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 100. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
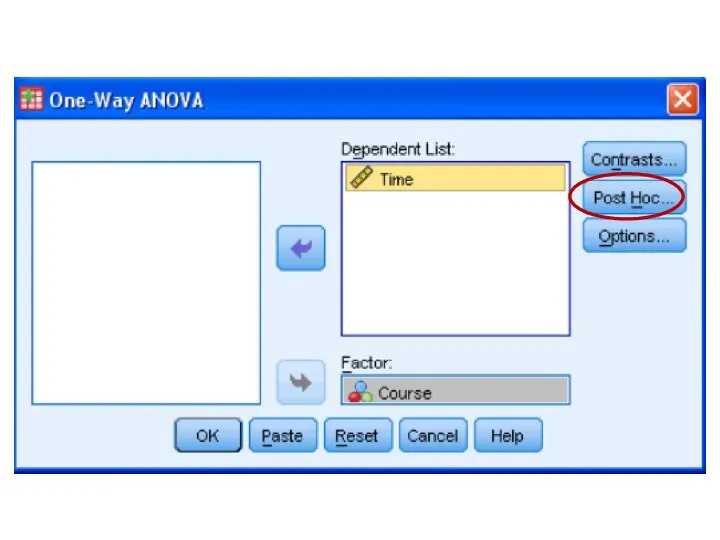
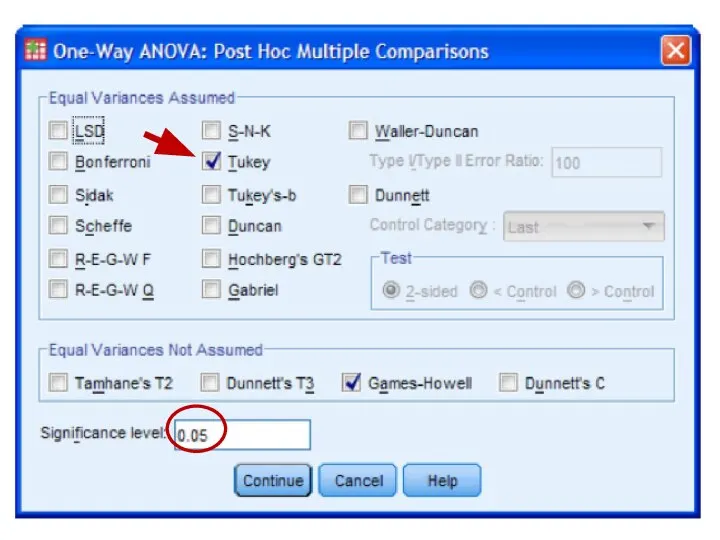
- 101. Exercise (17)
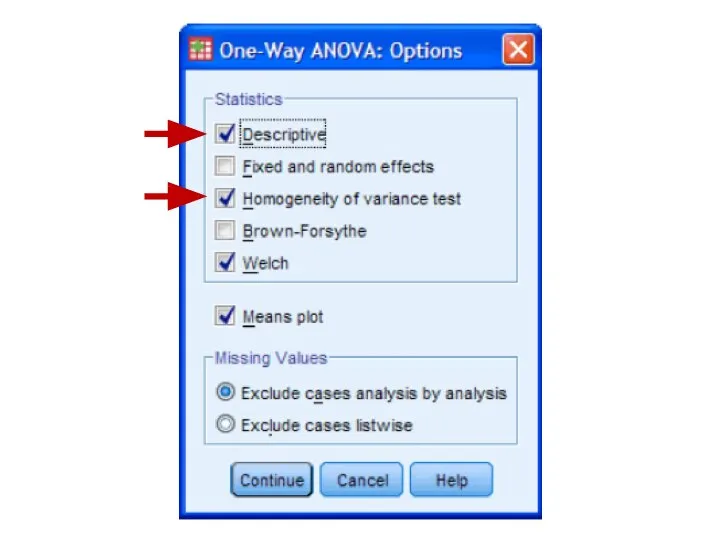
- 102. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 103. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 104. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 105. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 106. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 107. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 108. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 109. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 110. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 111. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 112. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 113. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
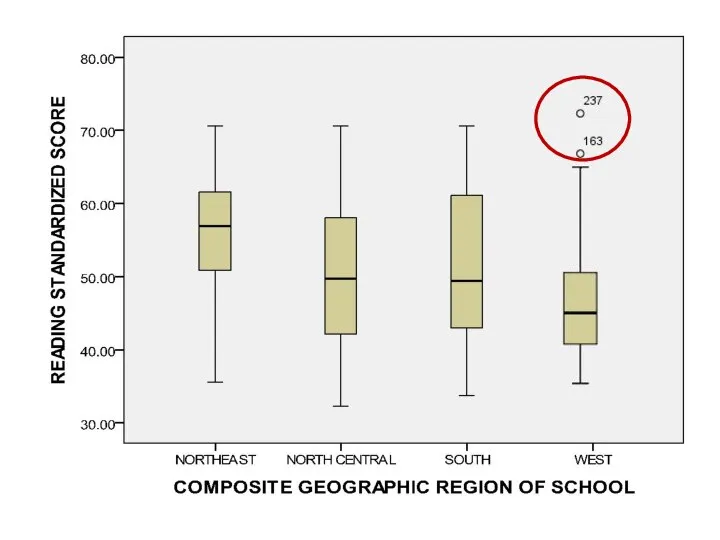
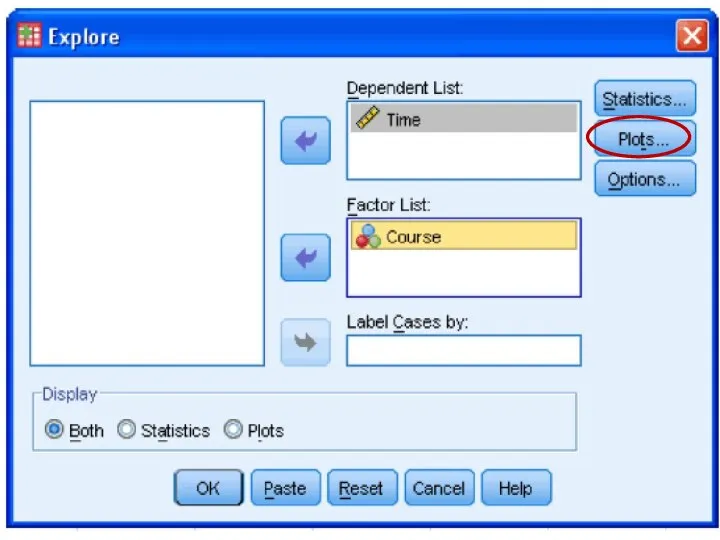
- 114. Box plot graph
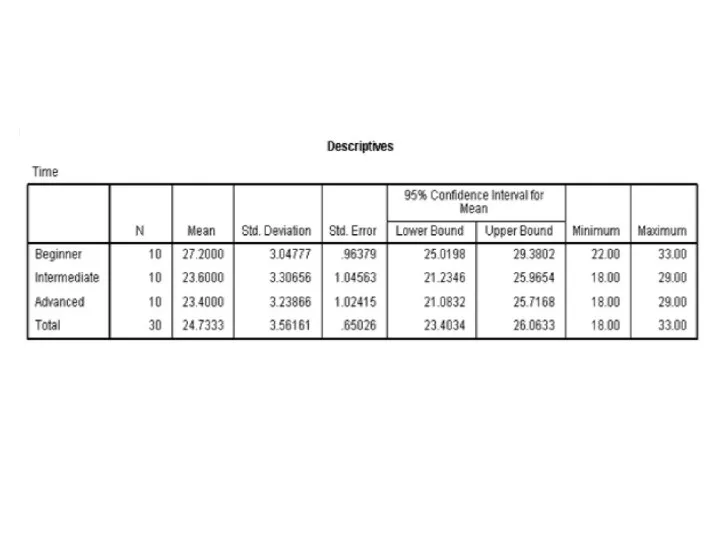
- 116. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
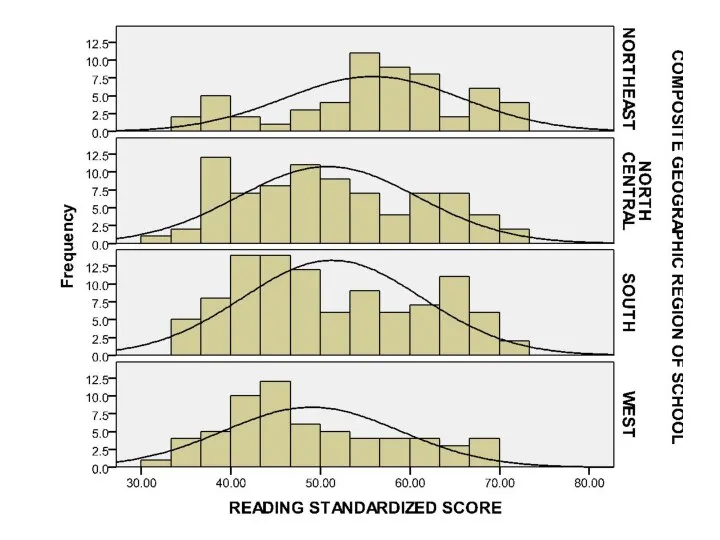
- 117. Histogram
- 119. Tests of Normality
- 120. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 122. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 123. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 124. Error Bar graph
- 126. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 127. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 128. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 136. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 137. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 138. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 144. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 145. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
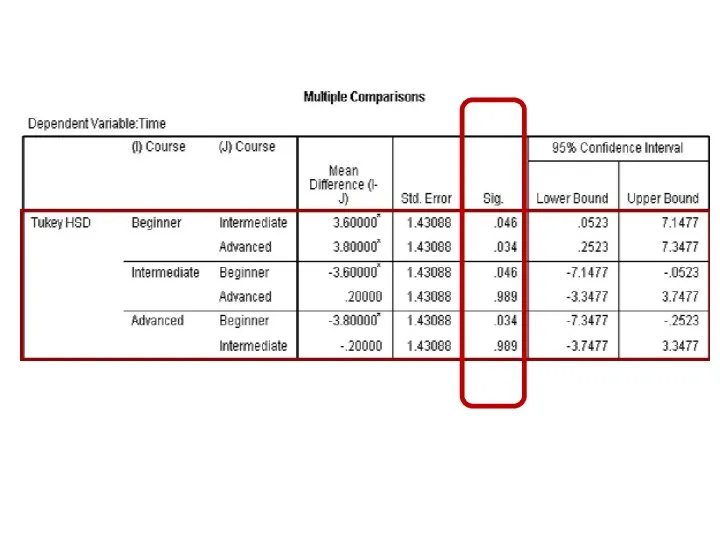
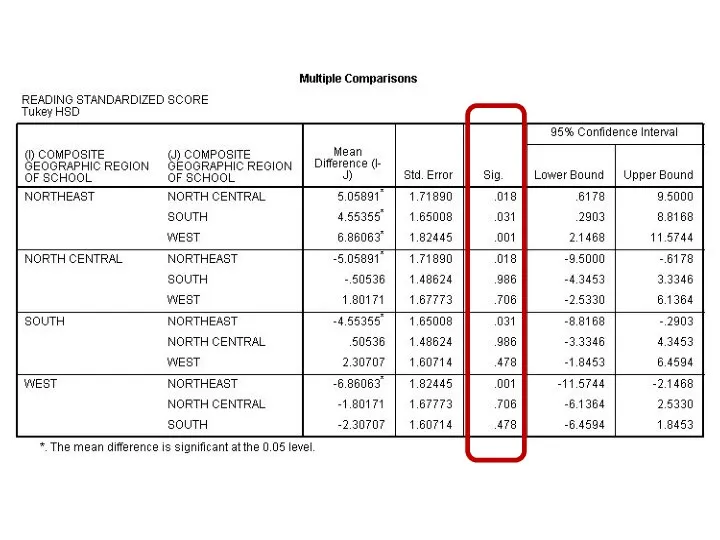
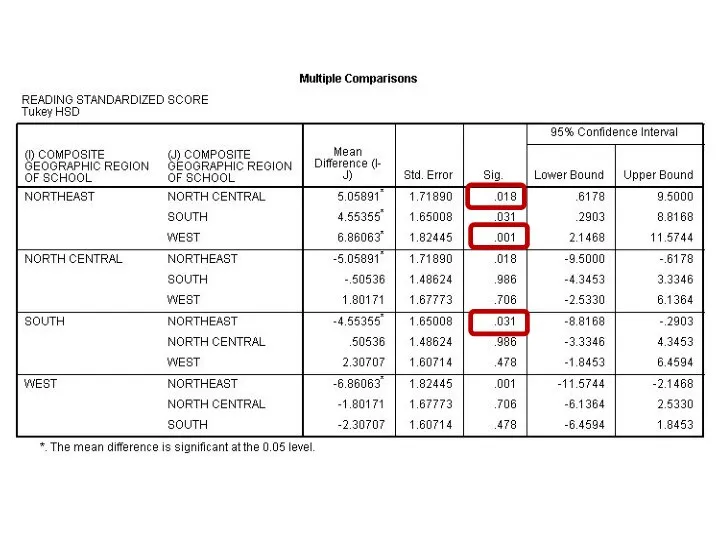
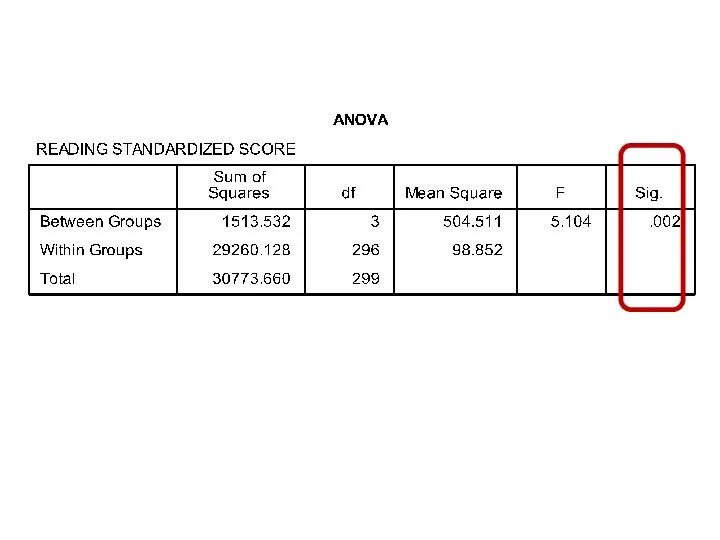
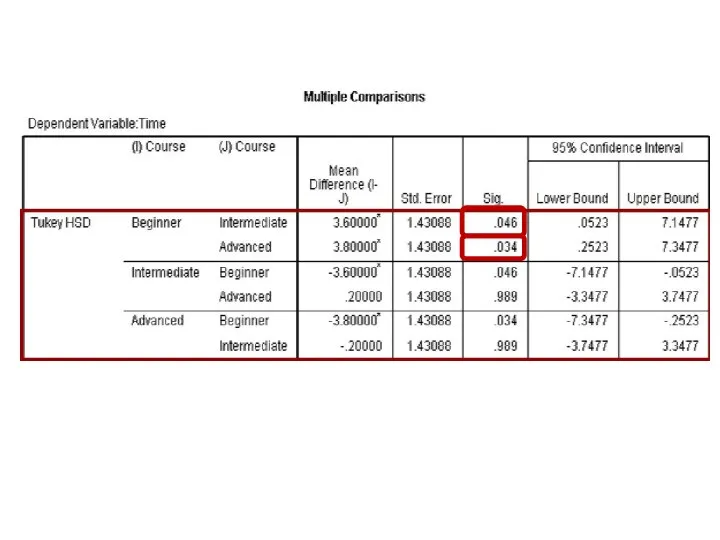
- 146. Return to Exercise (16)
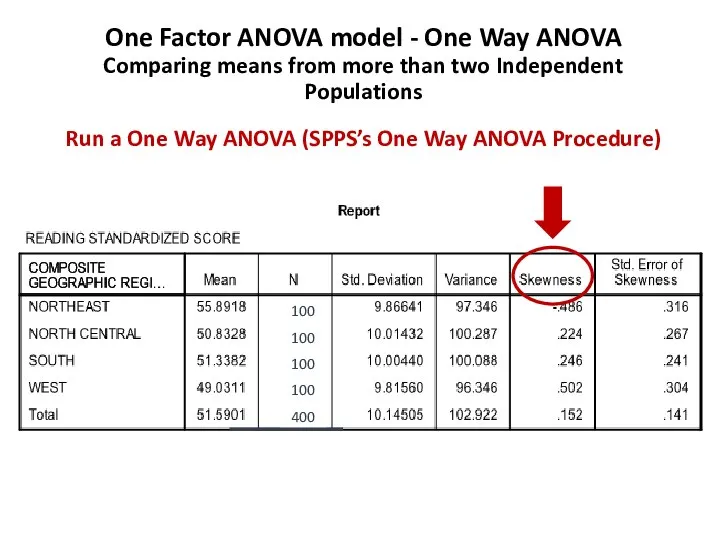
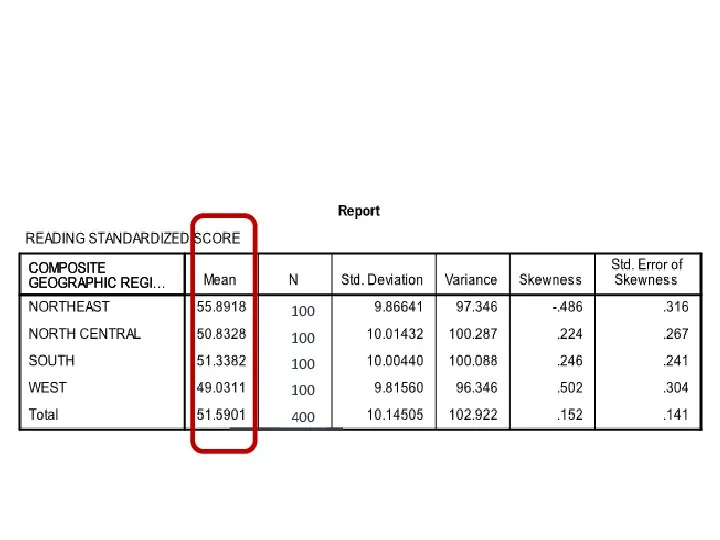
- 149. 100 100 100 100 400
- 150. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 152. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 153. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 155. 100 100 100 100 400
- 156. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 157. Return to Exercise (17)
- 161. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 163. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 164. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 167. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 168. One Factor ANOVA model - One Way ANOVA Comparing means from more than two Independent Populations
- 169. Comparing Means (Parametric tests) One Population Inference Two Population Inference Independent Sampling Model Dependent Sampling Model
- 172. Скачать презентацию





















































































 Вахта трехлистная
Вахта трехлистная Шизофрения_лечебники
Шизофрения_лечебники Пациенты с коронавирусом
Пациенты с коронавирусом Действие наркотиков и алкоголя на психику человека
Действие наркотиков и алкоголя на психику человека Медицинский осмотр
Медицинский осмотр Влияние курения на внутриутробное развитие плода
Влияние курения на внутриутробное развитие плода Сердечно-легочная реанимация
Сердечно-легочная реанимация Нервно-гуморальная регуляция функций организма
Нервно-гуморальная регуляция функций организма Синхронизация колебаний в связанных осцилляторах ФитцХью-Нагумо в ходе эпилептического приступа
Синхронизация колебаний в связанных осцилляторах ФитцХью-Нагумо в ходе эпилептического приступа Дистрофия (dis- бузилиш, trophe- озиқланиш)
Дистрофия (dis- бузилиш, trophe- озиқланиш) Острые ларингиты
Острые ларингиты Лазеромагнитная терапия
Лазеромагнитная терапия Методи візуалізації в променевій діагностиці
Методи візуалізації в променевій діагностиці Технология CEREC
Технология CEREC Ұрықтануға қарсы таблеткалар. Горманальды контрацептивтердің егілетін түрі
Ұрықтануға қарсы таблеткалар. Горманальды контрацептивтердің егілетін түрі Гипофиздің аденомасы
Гипофиздің аденомасы Систематика психических расстройств. Психопатологические симптомы
Систематика психических расстройств. Психопатологические симптомы Детская инфекционная болезнь корь
Детская инфекционная болезнь корь Переломы нижней челюсти у детей. Способы их устранения
Переломы нижней челюсти у детей. Способы их устранения Полисемия клинических терминоэлементов
Полисемия клинических терминоэлементов Патография Фридриха Ницше (1844-1900)
Патография Фридриха Ницше (1844-1900) Экстракция плода за тазовый конец, приём Морисо-Левре-Ляшапель
Экстракция плода за тазовый конец, приём Морисо-Левре-Ляшапель Рентгеноанатомия мочевыделительной системы
Рентгеноанатомия мочевыделительной системы Обследование мышц языка
Обследование мышц языка Синдромы при поражении почек
Синдромы при поражении почек Классификация опухолей
Классификация опухолей Основные принципы строения мозга. Структурно-функциональная модель мозга (по А. Р. Лурия)
Основные принципы строения мозга. Структурно-функциональная модель мозга (по А. Р. Лурия) Бациллярный ангиоматоз
Бациллярный ангиоматоз