Слайд 2When calculating indicators, not all the general population is often used, but
only some part of it (for example, in a selective study). It is necessary to evaluate the reliability of the results of the study. The measure of the reliability of the indicator is its error - the error of representativeness (representativeness)
. The error shows how much the result obtained in a selective study differs from the result that could be obtained by a continuous examination of the entire population.
Слайд 3Equipment of the lesson.
Multimedia projector
A laptop
Visual material in the form of a

multimedia presentation
Personal Computer
Слайд 4Taking into account that doctors, as a rule, carry out researches on

selective sets, the theory of statistics allows using the mathematical apparatus (formulas) to transfer data from selective research to the general population.
In this case, the doctor should be able not only to use the mathematical formula, but draw a conclusion, corresponding to each method of assessing the reliability of the data.
Слайд 5Applying the method of assessing the reliability of the results of a

study the researcher must be able to choose the correct method of this method.
Слайд 6Among the methods for assessing reliability
Parametric methods
Nonparametric methods

Слайд 7Parametric methods for assessing reliability are called -the application of which requires
a compulsory knowledge of the law of distribution of the studied features in the aggregate and the calculation of their basic parameters.
Nonparametric methods for assessing reliability are the application of which does not require knowledge of the law of distribution of the studied characteristics in the aggregate and the calculation of their basic parameters.
Слайд 8In the final result, a certain numerical value is calculated, which is
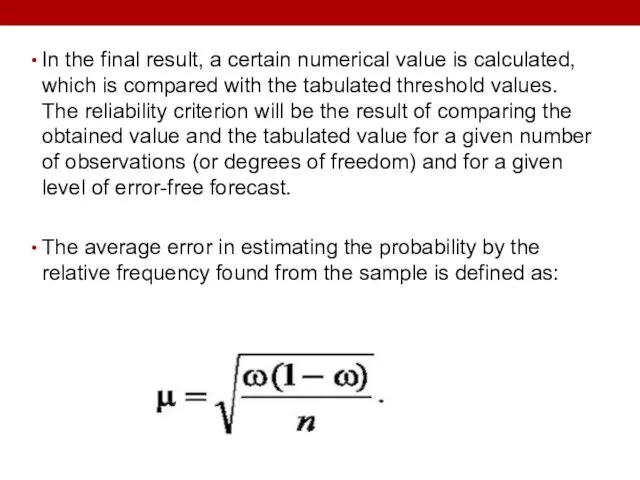
compared with the tabulated threshold values. The reliability criterion will be the result of comparing the obtained value and the tabulated value for a given number of observations (or degrees of freedom) and for a given level of error-free forecast.
The average error in estimating the probability by the relative frequency found from the sample is defined as:
Слайд 9where σ is the standard deviation;
n is the number of observations.
The
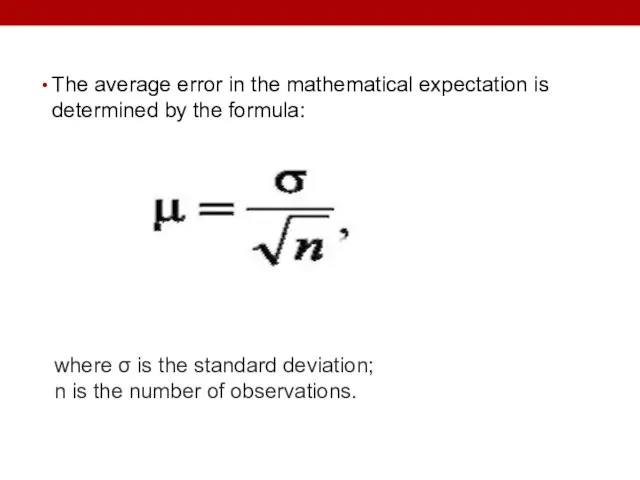
average error in the mathematical expectation is determined by the formula:
Слайд 10With the number of observations less than 30, the mathematical expectation error

and the probability found by the sample are determined by the formulas:









 Болезнь Рандю-Ослера. Гемобластозы. Геморрагические диатезы
Болезнь Рандю-Ослера. Гемобластозы. Геморрагические диатезы Патофизиология нервной системы
Патофизиология нервной системы Туберкулёз
Туберкулёз Вместе против рака
Вместе против рака Оценка состояния нервной системы
Оценка состояния нервной системы Хронический миелобластный лейкоз у детей
Хронический миелобластный лейкоз у детей Менингит. Признаки и симптомы
Менингит. Признаки и симптомы Влияние иммуномодулирующей терапии на течение рассеянного склероза
Влияние иммуномодулирующей терапии на течение рассеянного склероза Оценка скорости резорбции кортикальной кости человека в зависимости от пола и возраста
Оценка скорости резорбции кортикальной кости человека в зависимости от пола и возраста Основы больничной гигиены
Основы больничной гигиены Хромосомные болезни
Хромосомные болезни Рак предстательной железы
Рак предстательной железы Гриппозное поражение нервной системы
Гриппозное поражение нервной системы Развитие медицины в России в XIX веке
Развитие медицины в России в XIX веке ВИЧ-инфекция
ВИЧ-инфекция Сестринский процесс при туберкулёзе лёгких
Сестринский процесс при туберкулёзе лёгких Судорожный синдром у детей
Судорожный синдром у детей Краткий опросник ВОЗ для оценки качества жизни (WHOQOL-BREF)
Краткий опросник ВОЗ для оценки качества жизни (WHOQOL-BREF) Диабет. Лечение
Диабет. Лечение Комбинированное применение лекарственных средств: синергизм, антагонизм, их виды и значение
Комбинированное применение лекарственных средств: синергизм, антагонизм, их виды и значение Оказание первой помощи при функциональных повреждениях
Оказание первой помощи при функциональных повреждениях Уход при ангине
Уход при ангине Травма – основная причина смерти в популяции
Травма – основная причина смерти в популяции Профилактика остеопороза
Профилактика остеопороза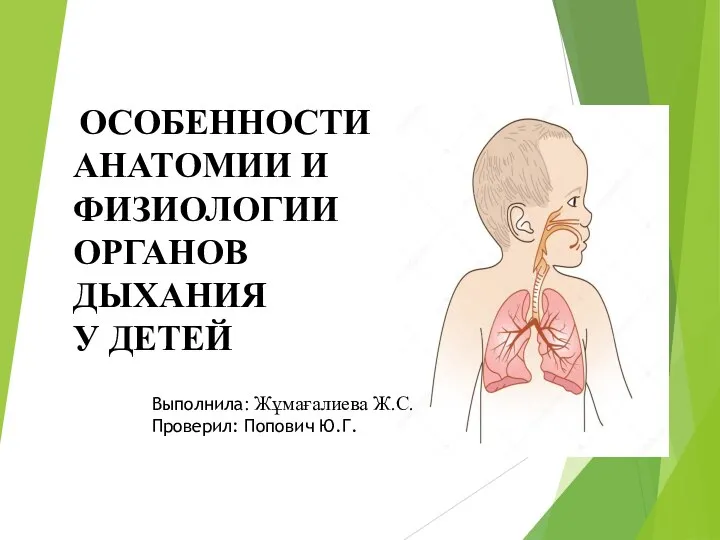 Особенности анатомии и физиологии органов дыхания у детей
Особенности анатомии и физиологии органов дыхания у детей Патогенез приступа стабильной стенокардии
Патогенез приступа стабильной стенокардии Физиология родов и послеродового периода
Физиология родов и послеродового периода Расти и развиваться здоровыми. Почему мы иногда болеем
Расти и развиваться здоровыми. Почему мы иногда болеем