Содержание
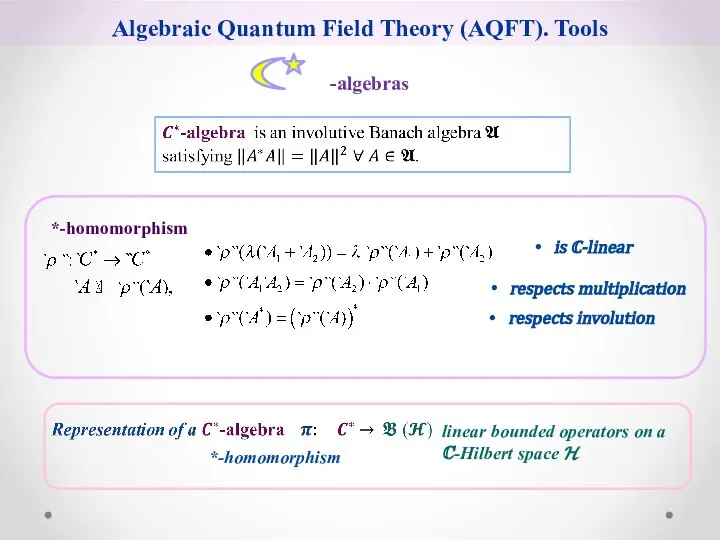
- 2. -algebras *-homomorphism Algebraic Quantum Field Theory (AQFT). Tools
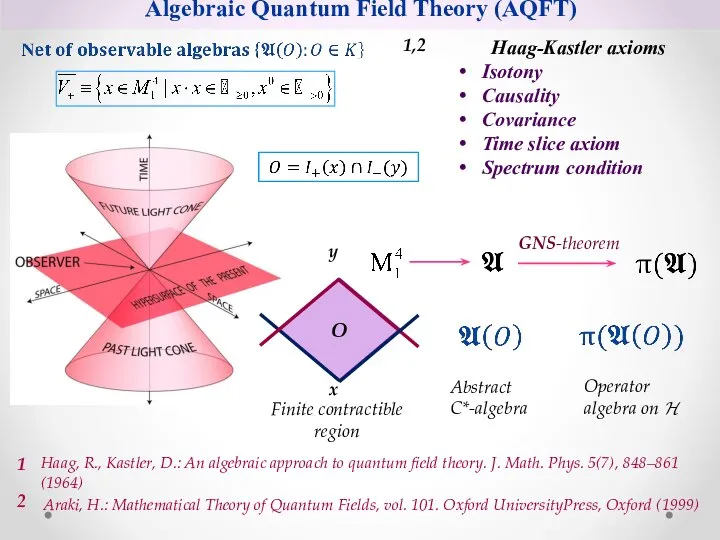
- 4. Algebraic Quantum Field Theory (AQFT) Haag-Kastler axioms Isotony Causality Covariance Time slice axiom Spectrum condition 1,2
- 5. Algebraic Quantum Field Theory (AQFT) Isotony Haag-Kastler axioms
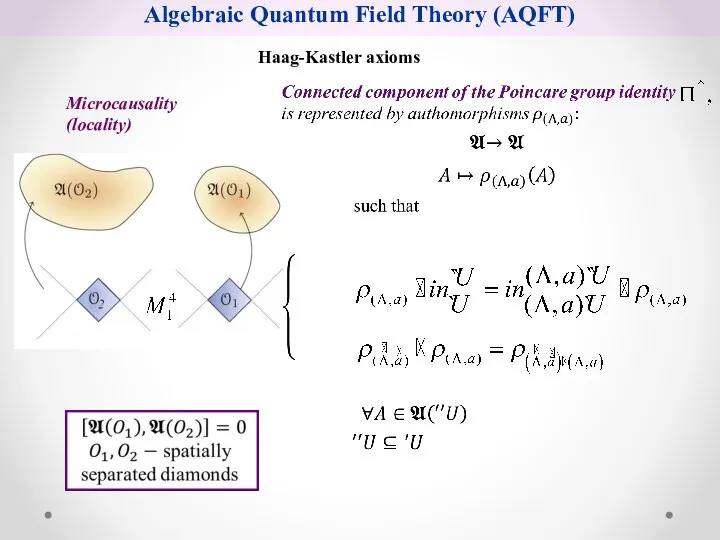
- 6. Microcausality (locality) Algebraic Quantum Field Theory (AQFT) Haag-Kastler axioms
- 7. Algebraic Quantum Field Theory (AQFT) Haag-Kastler axioms
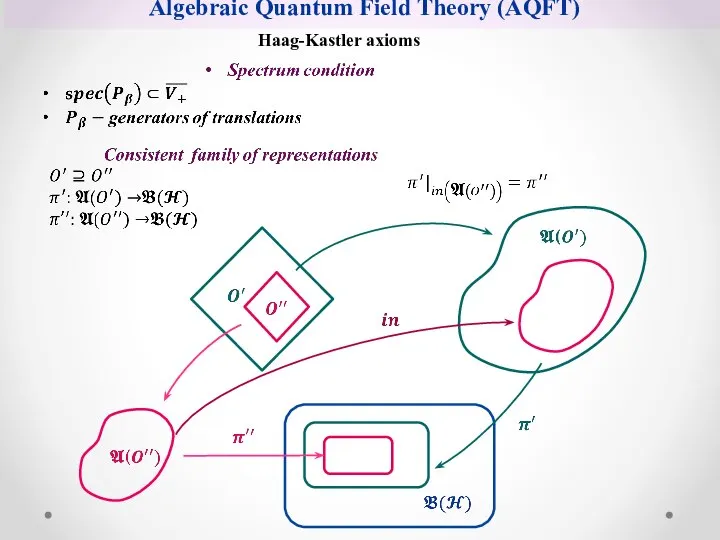
- 8. Algebraic Quantum Field Theory (AQFT) Haag-Kastler axioms
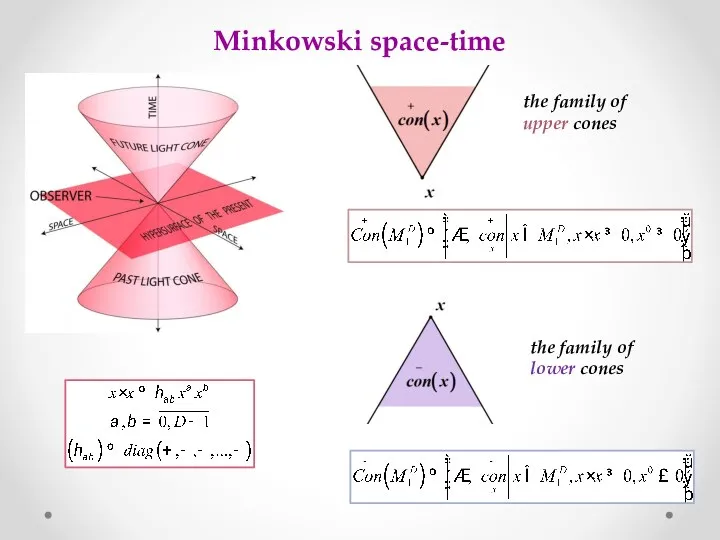
- 9. Minkowski space-time the family of upper cones the family of lower cones
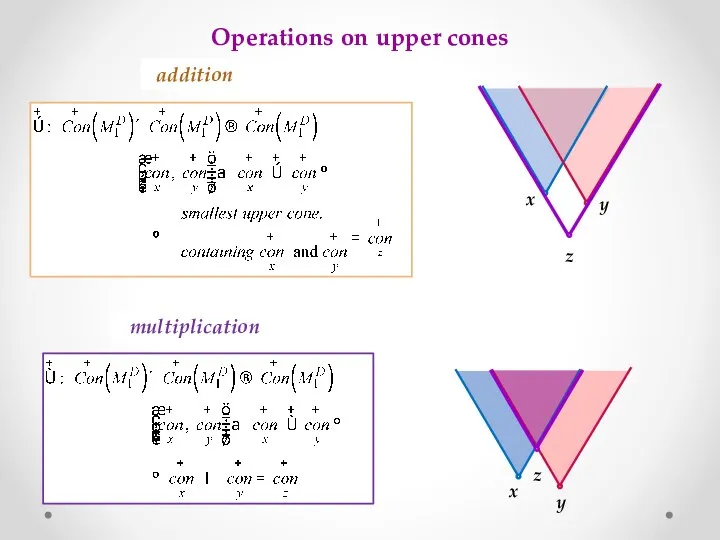
- 10. Operations on upper cones addition multiplication x y z x y z
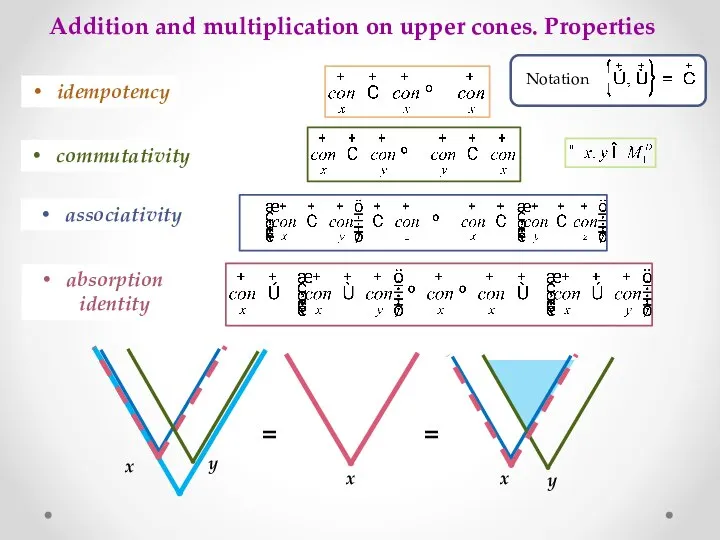
- 11. Addition and multiplication on upper cones. Properties idempotency commutativity associativity absorption identity x y x y
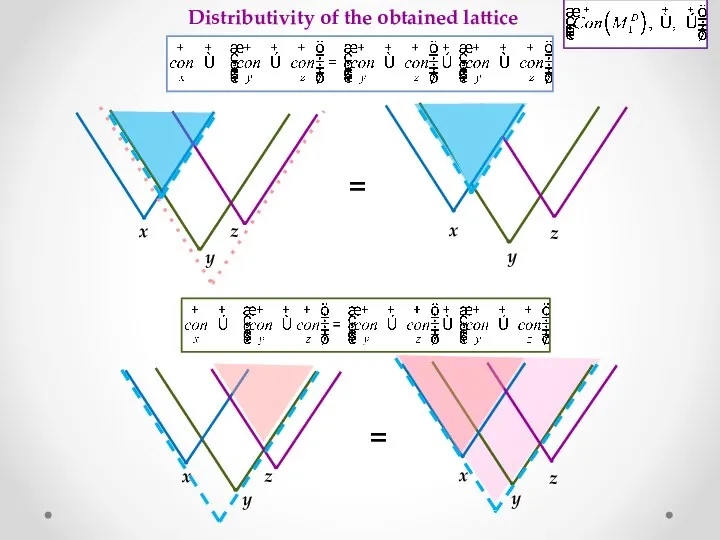
- 12. Distributivity of the obtained lattice = x y z x y z = x y z
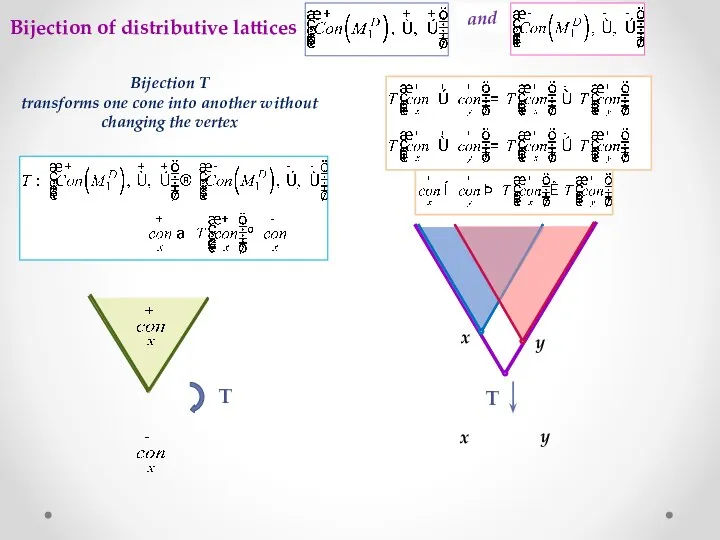
- 13. Bijection of distributive lattices and Bijection T transforms one cone into another without changing the vertex
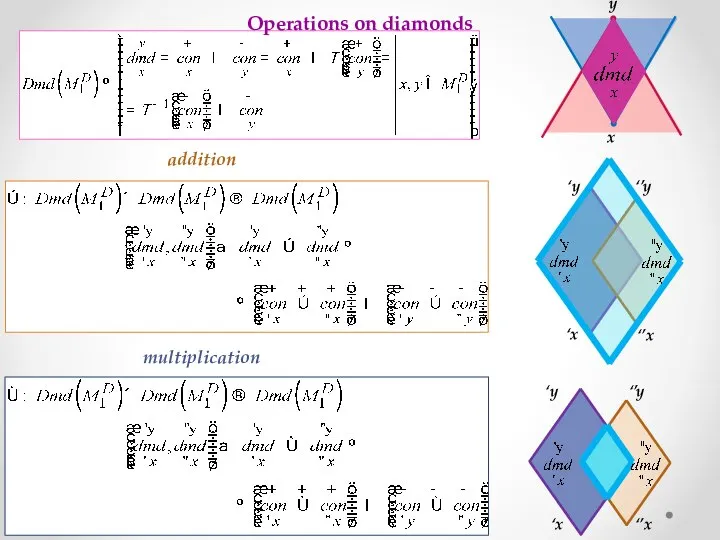
- 14. Operations on diamonds x y addition multiplication ‘x ‘’x ‘y ‘’y ‘x ‘’x ‘y ‘’y
- 15. Addition and multiplication on diamonds. Properties idempotency commutativity associativity absorption identity ‘x ‘’x ‘y ‘’y ‘x
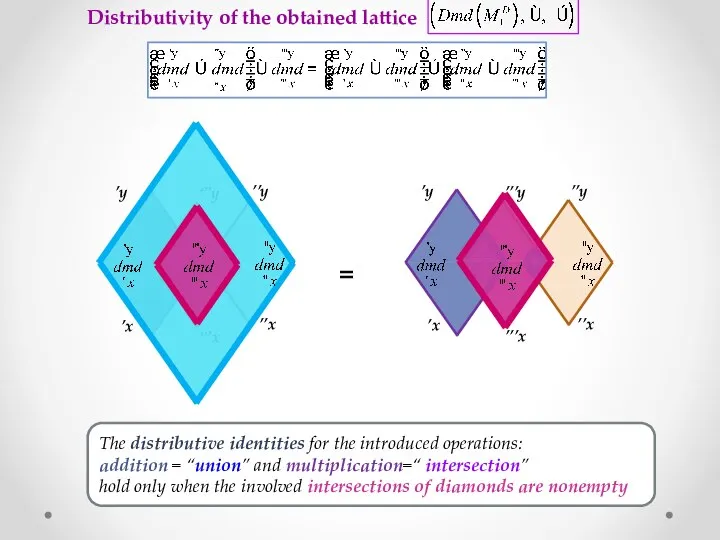
- 16. Distributivity of the obtained lattice ’x ‘’’x ’y ‘’’y ’’x ’’y ’x ’’’x ’y ’’’y ’’x
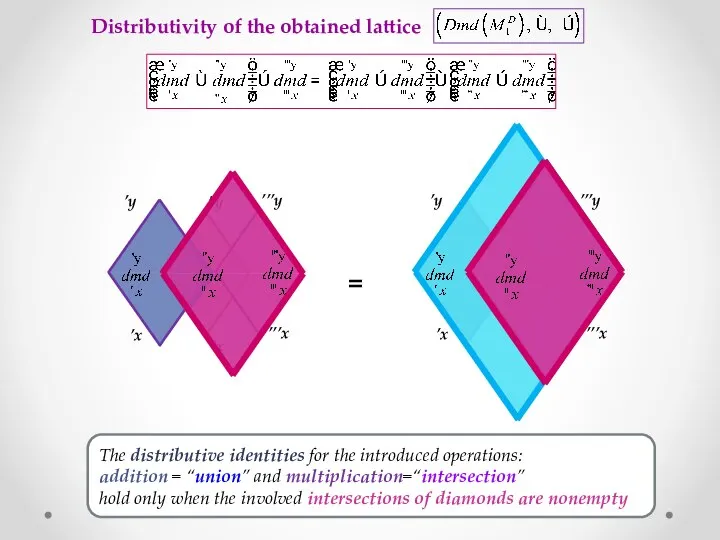
- 17. Distributivity of the obtained lattice ’x ’’x ’y ’’y ’’’x ’’’y ’x ’’x ’y ’’y ’’’x
- 19. Скачать презентацию




 Математический КВН (6 класс)
Математический КВН (6 класс) Математический тренажер
Математический тренажер Площадь трапеции
Площадь трапеции Цепочки. Сравните выражения
Цепочки. Сравните выражения Регрессионный анализ
Регрессионный анализ Координатная плоскость. Прямоугольная система координат
Координатная плоскость. Прямоугольная система координат Пересечение поверхностей
Пересечение поверхностей Презентация на тему Подобные треугольники, решаем задачи по геометрии презентация
Презентация на тему Подобные треугольники, решаем задачи по геометрии презентация  Таблица сложения
Таблица сложения Многогранники. Прямоугольные параллелепипеды
Многогранники. Прямоугольные параллелепипеды Сложение и вычитание многозначных чисел
Сложение и вычитание многозначных чисел Кривые второго порядка
Кривые второго порядка Алгебраическая дробь и её основное свойство. 7 класс
Алгебраическая дробь и её основное свойство. 7 класс Тройные и многократные интегралы
Тройные и многократные интегралы Годовая проверочная работа по математике. 1 класс
Годовая проверочная работа по математике. 1 класс Зачет по теме Параллельность прямых, прямой и плоскости
Зачет по теме Параллельность прямых, прямой и плоскости Приёмы сложения однозначных чисел с переходом через десяток
Приёмы сложения однозначных чисел с переходом через десяток Прямоугольник. Свойства
Прямоугольник. Свойства Технология подготовки учащихся к овладению решения задач с параметрами комбинированными методами. Занятие №5
Технология подготовки учащихся к овладению решения задач с параметрами комбинированными методами. Занятие №5 Курс по математике ОГЭ 2021
Курс по математике ОГЭ 2021 Умножение одночлена на многочлен. Самостоятельная работа
Умножение одночлена на многочлен. Самостоятельная работа Теорема Виета. Устная работа
Теорема Виета. Устная работа Презентация на тему Математика вокруг нас 6 класс для учителя
Презентация на тему Математика вокруг нас 6 класс для учителя  Составные уравнения
Составные уравнения Формулы сокращенного умножения
Формулы сокращенного умножения Уравнения и неравенства с двумя переменными
Уравнения и неравенства с двумя переменными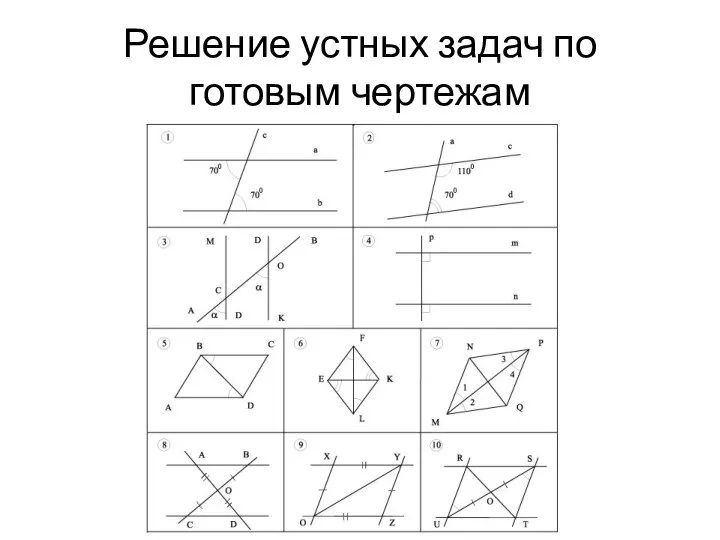 Решение устных задач по готовым чертежам
Решение устных задач по готовым чертежам Декартова система координат в евклидовом пространстве
Декартова система координат в евклидовом пространстве