Содержание
- 2. LECTURE 6 Probability Temur Makhkamov Indira Khadjieva QM Module Leaders [email protected] [email protected] Office hours: by appointment
- 3. Lecture outline The meaning of probability and relevant concepts The basic operations of probability Sets, combination,
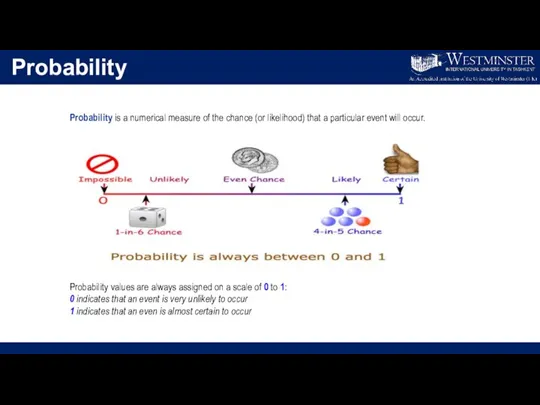
- 4. Probability Probability is a numerical measure of the chance (or likelihood) that a particular event will
- 5. Experiment vs Sample Space The probability is a chance or likelihood of an event to happen
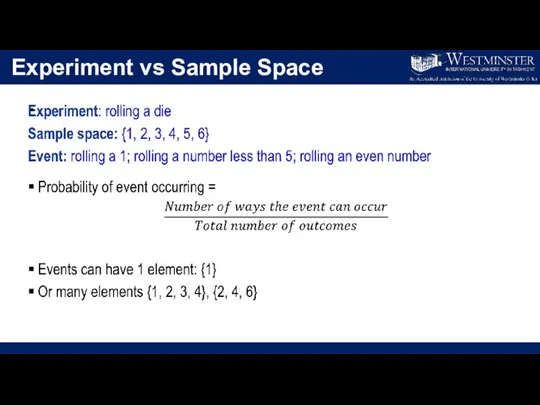
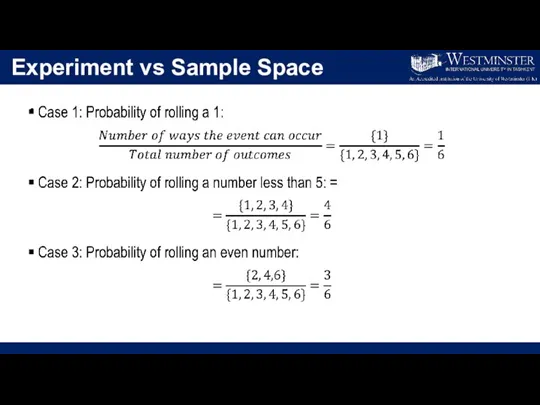
- 6. Experiment vs Sample Space
- 7. Experiment vs Sample Space
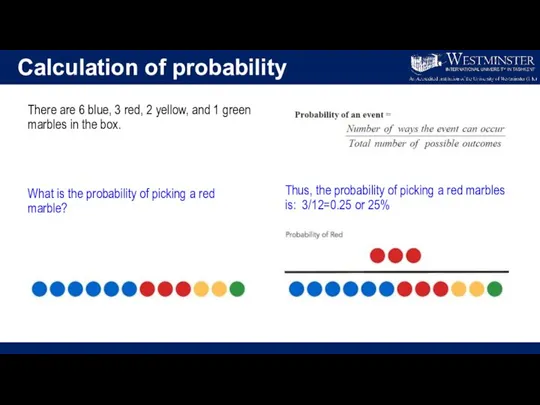
- 8. Calculation of probability There are 6 blue, 3 red, 2 yellow, and 1 green marbles in
- 9. Calculation of probability There are 6 blue, 3 red, 2 yellow, and 1 green marbles in
- 10. Calculation of probability If there are n experimental outcomes, the sum of the probabilities for all
- 12. Types of counting rules Multiple step Combination Permutation
- 13. Multiple step experiment Experiment of a sequence of k steps Total number of experimental outcomes is
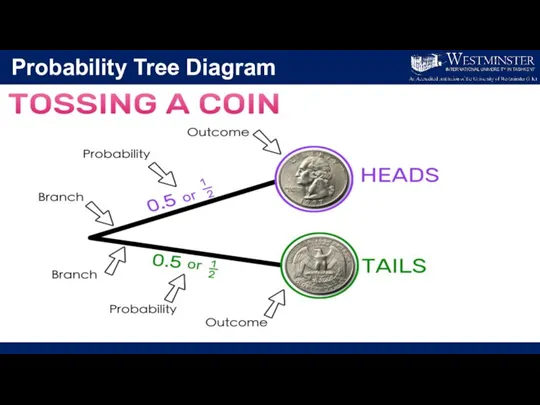
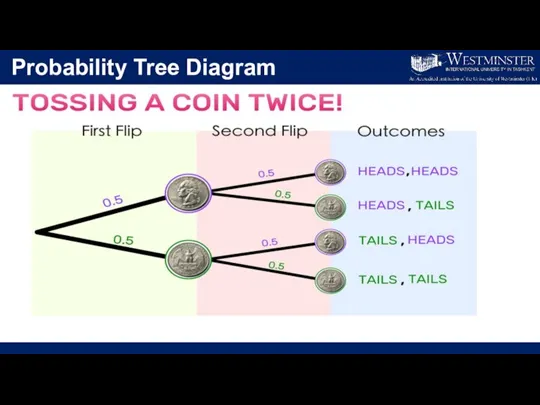
- 14. Probability Tree Diagram
- 15. Probability Tree Diagram
- 16. Probability calculation
- 17. Self exam-training task: Find the number of all possible outcomes for rolling a die three times
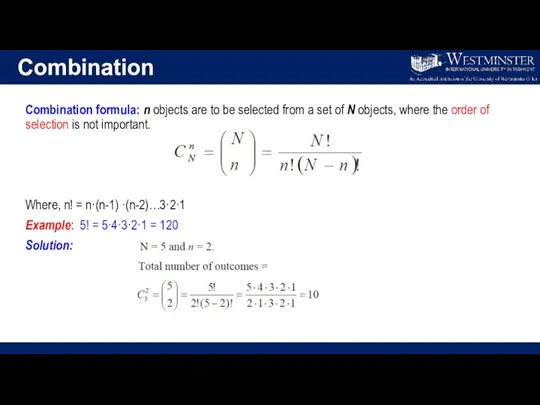
- 18. Combination Example: In this classroom, a lecturer randomly picks two of five students (let’s say Shakhzoda,
- 19. Combination Combination formula: n objects are to be selected from a set of N objects, where
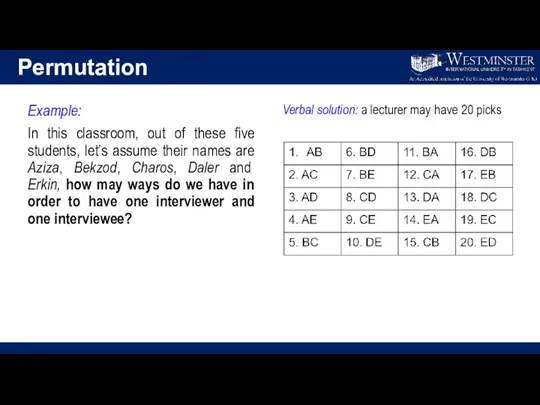
- 20. Permutation Example: In this classroom, out of these five students, let’s assume their names are Aziza,
- 21. Permutation Permutation formula: n objects are to be selected from a set of N objects, where
- 22. What Permutation can tell us ?
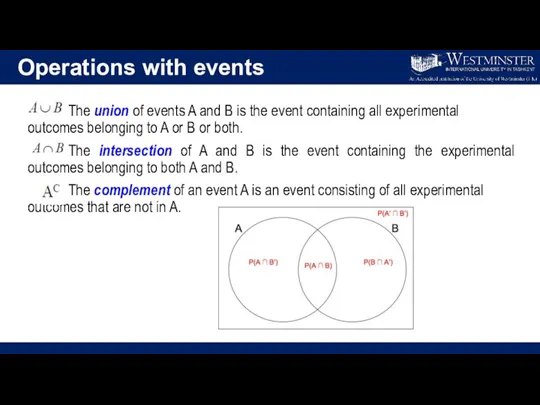
- 23. Operations with events The union of events A and B is the event containing all experimental
- 24. Operations with events Toss a die and observe the number that appears on top S =
- 25. Relationship of events Mutually exclusive events - The events A and B do not have any
- 26. Addition rule for union Question: On Quantitative Methods module for 600 CIFS students at WIUT, 480
- 27. Multiplication rule for intersection
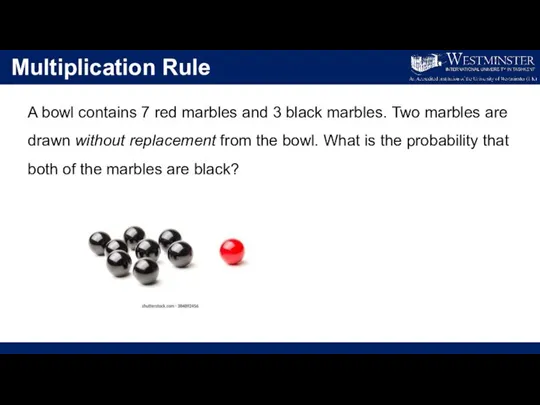
- 28. Multiplication Rule A bowl contains 7 red marbles and 3 black marbles. Two marbles are drawn
- 29. Solution Let A = the event that the first marble is black; let B = the
- 30. Mathematical expectation 365bet.com sent you following offers for coming El-Classico game depending on your betting: If
- 31. Concluding remarks Today, you learned: Basic concepts within probability theory Basic operations of calculating the sample
- 33. Скачать презентацию



















 Сборник задач Во имя тех священных дней
Сборник задач Во имя тех священных дней Делим на равные части
Делим на равные части Геометричний метод розв’язування задач лінійного програмування. Методи оптимізації. Лекція №2
Геометричний метод розв’язування задач лінійного програмування. Методи оптимізації. Лекція №2 Матрицы и действия на матрицами
Матрицы и действия на матрицами Перемещение тела на плоскости (задача)
Перемещение тела на плоскости (задача)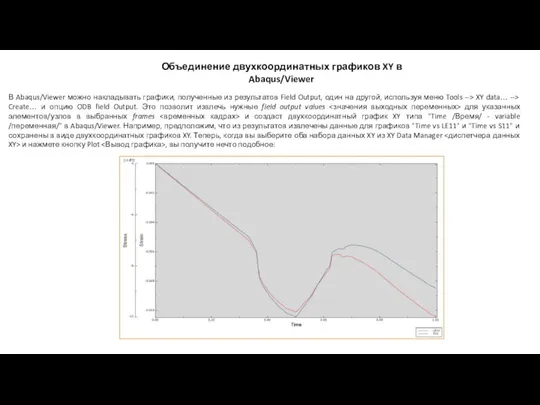 Объединение двухкоординатных графиков XY в Abaqus/Viewer
Объединение двухкоординатных графиков XY в Abaqus/Viewer Свойства параллельных плоскостей
Свойства параллельных плоскостей Свойства степеней с натуральными показателями
Свойства степеней с натуральными показателями Производная функции
Производная функции Решение задач на применение признаков подобия треугольников
Решение задач на применение признаков подобия треугольников Промежутки возрастания, убывания, знакопостоянства и нули функции
Промежутки возрастания, убывания, знакопостоянства и нули функции Геометрическая мозаика из правильных одноимённых многоугольников
Геометрическая мозаика из правильных одноимённых многоугольников Основные правила дифференцирования
Основные правила дифференцирования Геометрия
Геометрия Сложение векторов
Сложение векторов ЦАРСКОЙ ДОРОГИ В МАТЕМАТИКЕ НЕТ.
ЦАРСКОЙ ДОРОГИ В МАТЕМАТИКЕ НЕТ. Razonamiento. Matemático práctica
Razonamiento. Matemático práctica Предел функции
Предел функции Доверительные интервалы и доверительная вероятность. Распределение Стьюдента
Доверительные интервалы и доверительная вероятность. Распределение Стьюдента Тест. Первообразная
Тест. Первообразная Случаи вычитания 12 -
Случаи вычитания 12 - Четные и нечетные функции
Четные и нечетные функции Алгоритмы нахождения независимого множества
Алгоритмы нахождения независимого множества “Збери” задачу
“Збери” задачу Решение задач на одновременное встречное движение
Решение задач на одновременное встречное движение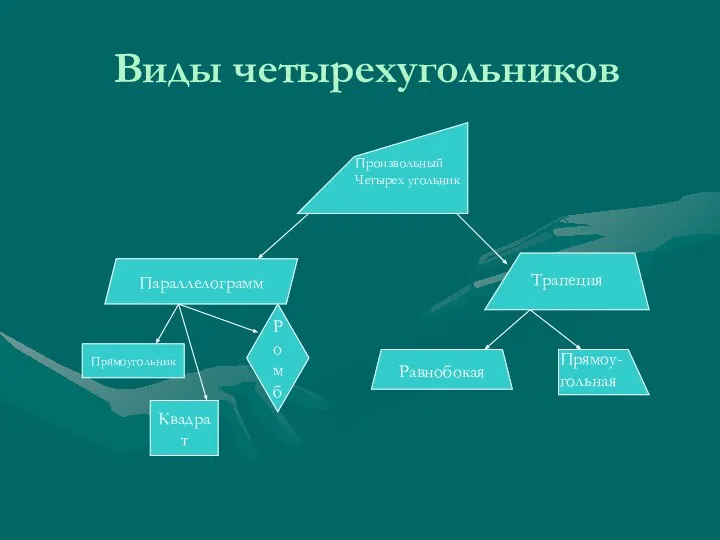 Виды четырехугольников
Виды четырехугольников Числовые последовательности
Числовые последовательности Презентация на тему Применение распределительного свойства умножения 6 класс
Презентация на тему Применение распределительного свойства умножения 6 класс