Содержание
- 2. Points and grades from examination
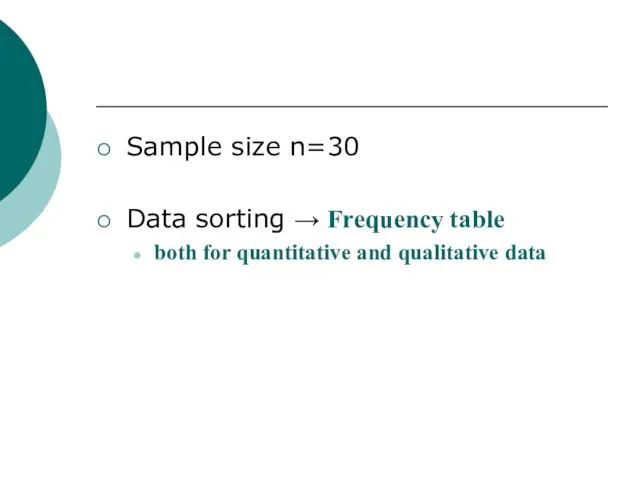
- 3. Sample size n=30 Data sorting → Frequency table both for quantitative and qualitative data
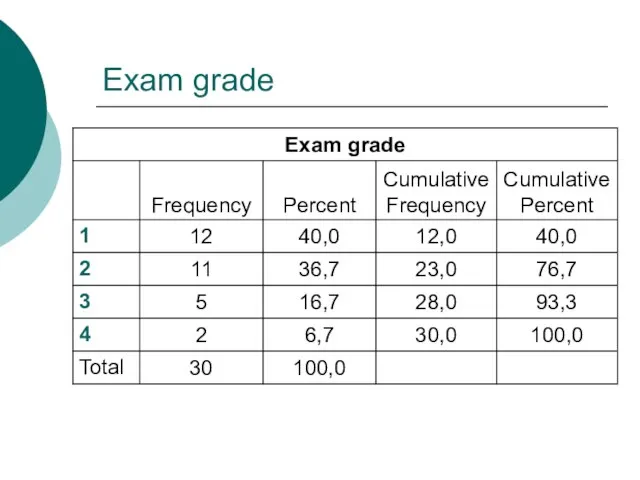
- 4. Exam grade
- 5. Notation Frequency … ni Relative frequency … fi Cumulative Frequency … Ni Cumulative Percent … Fi
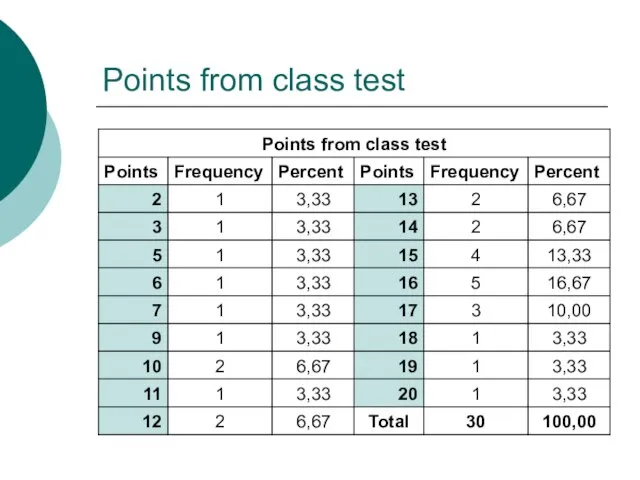
- 6. Points from class test
- 7. Quantitative variables Grouping into class intervals
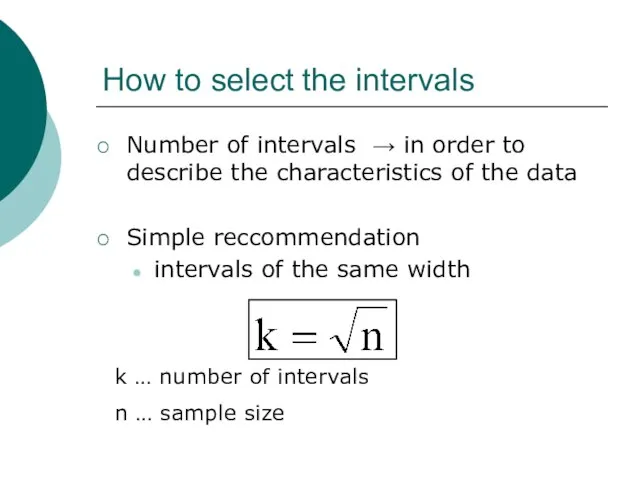
- 8. How to select the intervals Number of intervals → in order to describe the characteristics of
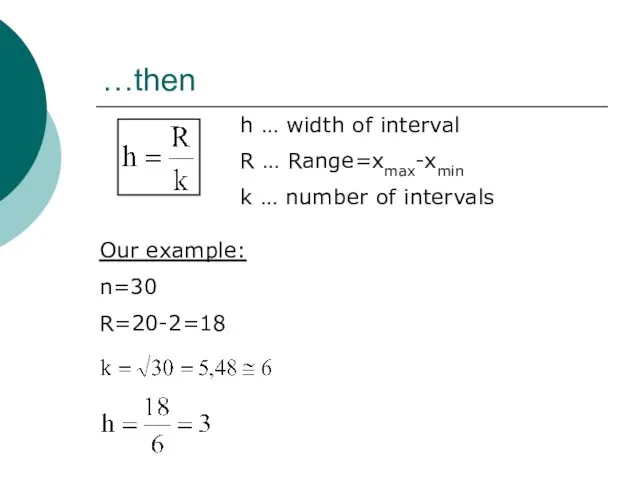
- 9. …then h … width of interval R … Range=xmax-xmin k … number of intervals Our example:
- 10. Points from class test
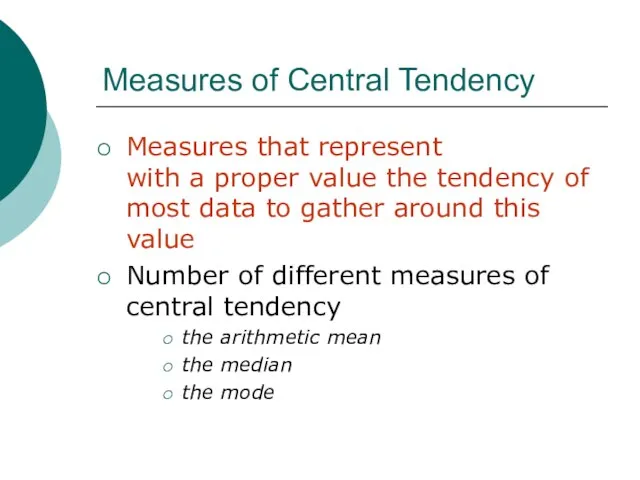
- 11. Measures of Central Tendency Measures that represent with a proper value the tendency of most data
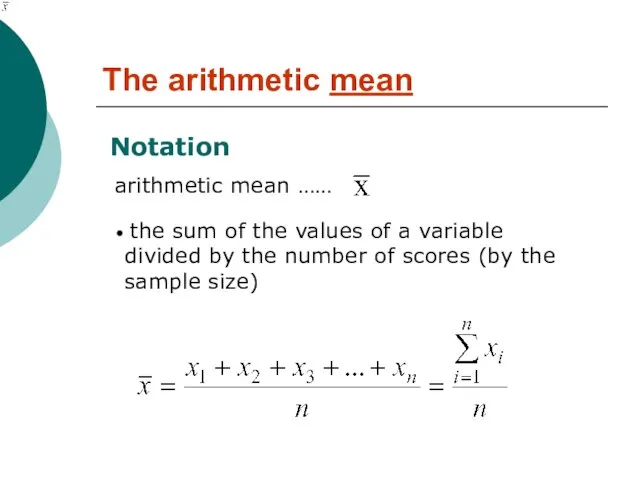
- 12. The arithmetic mean Notation arithmetic mean …… the sum of the values of a variable divided
- 13. Properties of the arithmetic mean it is expressed in the same unit of measure as the
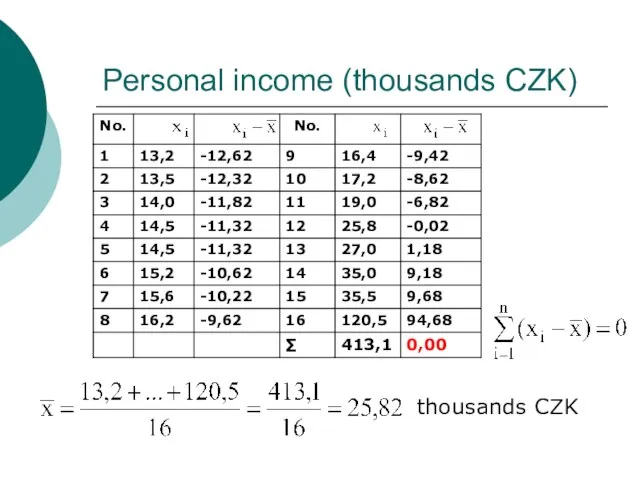
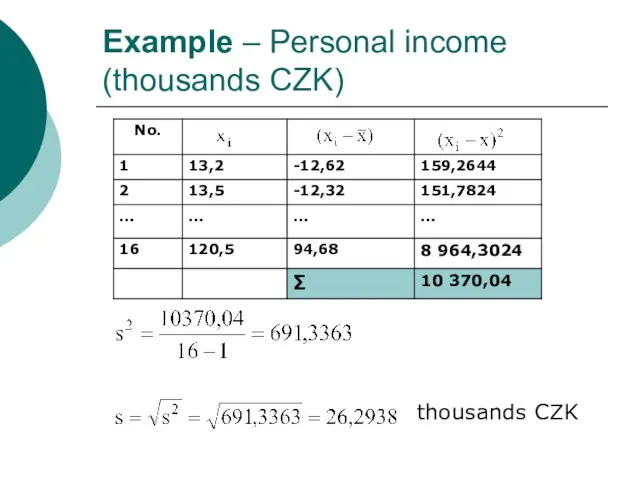
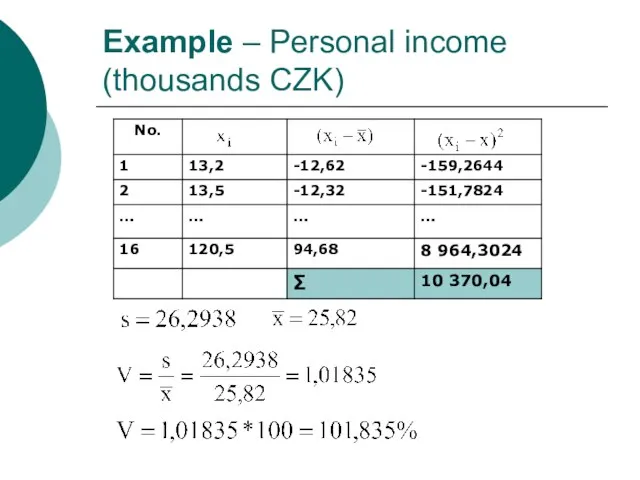
- 14. Personal income (thousands CZK) thousands CZK
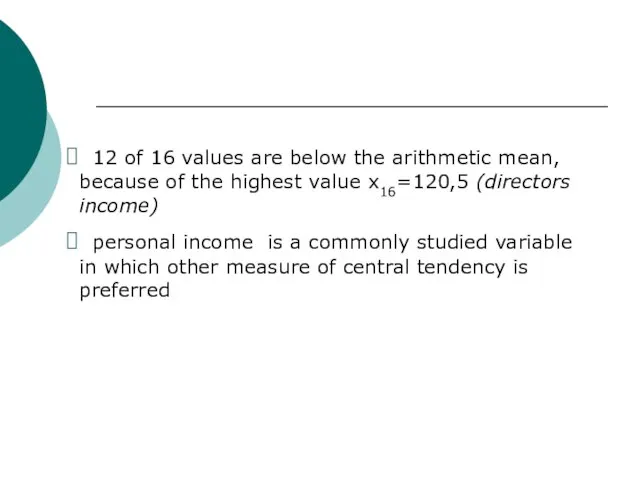
- 15. 12 of 16 values are below the arithmetic mean, because of the highest value x16=120,5 (directors
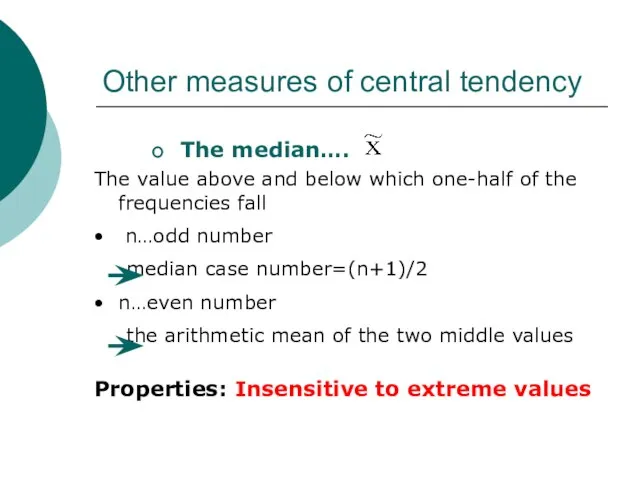
- 16. Other measures of central tendency The median…. The value above and below which one-half of the
- 17. Other measures of central tendency The mode…. The value that occurs with greatest frequency for qualitative
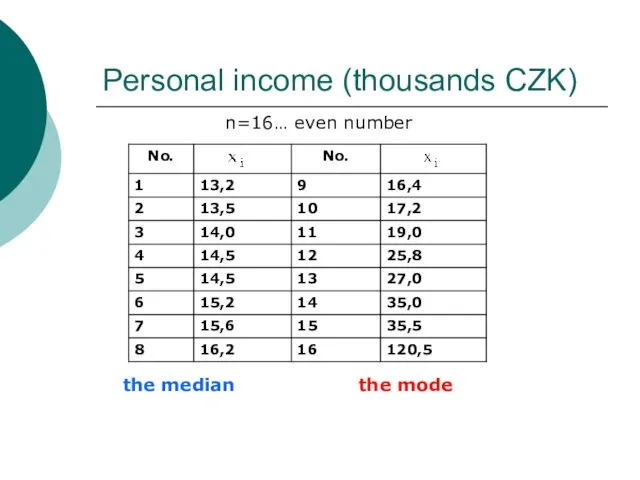
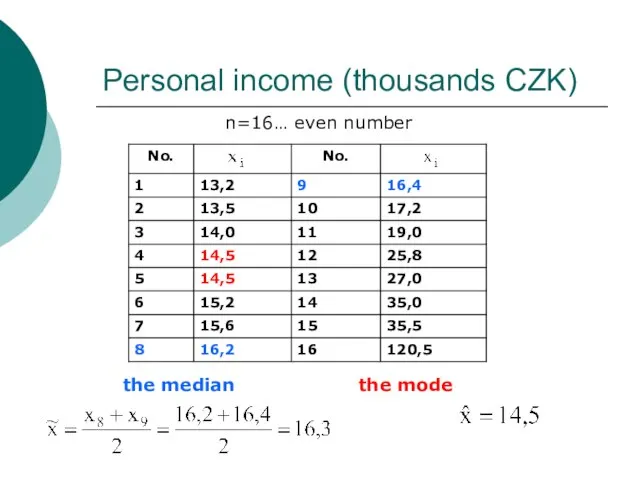
- 18. Personal income (thousands CZK) n=16… even number the median the mode
- 19. Personal income (thousands CZK) n=16… even number the median the mode
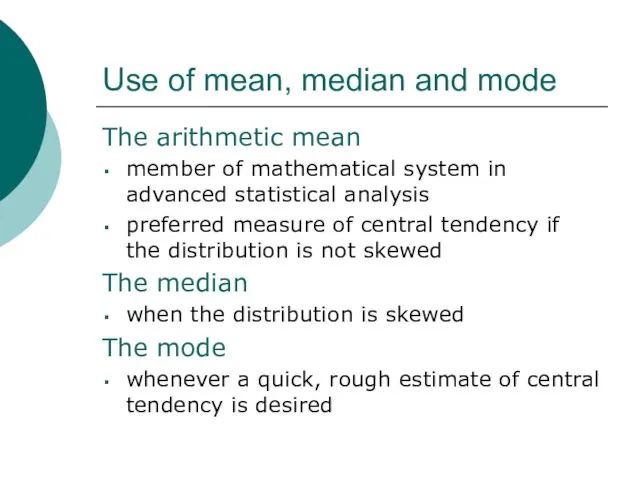
- 20. Use of mean, median and mode The arithmetic mean member of mathematical system in advanced statistical
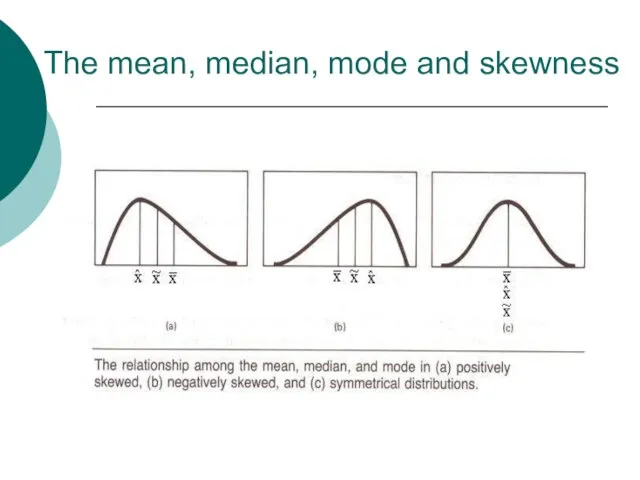
- 21. The mean, median, mode and skewness
- 22. to describe the spread of the data, its variation around a central value we want to
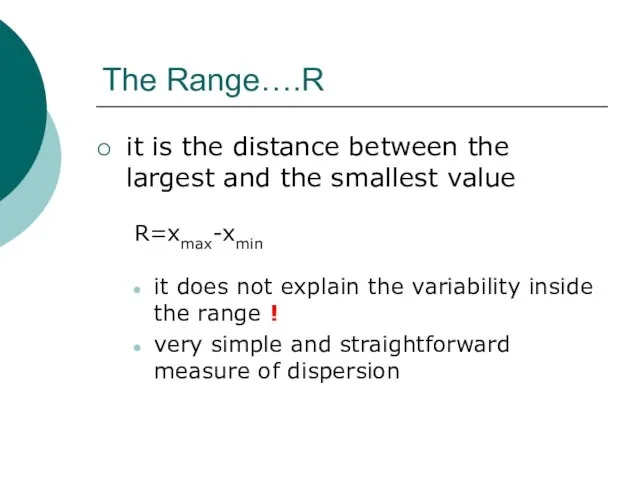
- 24. The Range….R it is the distance between the largest and the smallest value R=xmax-xmin it does
- 25. The Variance…s2 it is an average squared deviation of each value from the mean it is
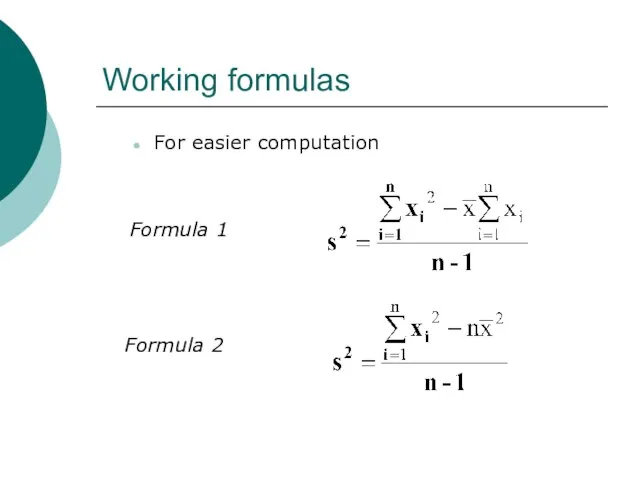
- 26. Working formulas For easier computation Formula 1 Formula 2
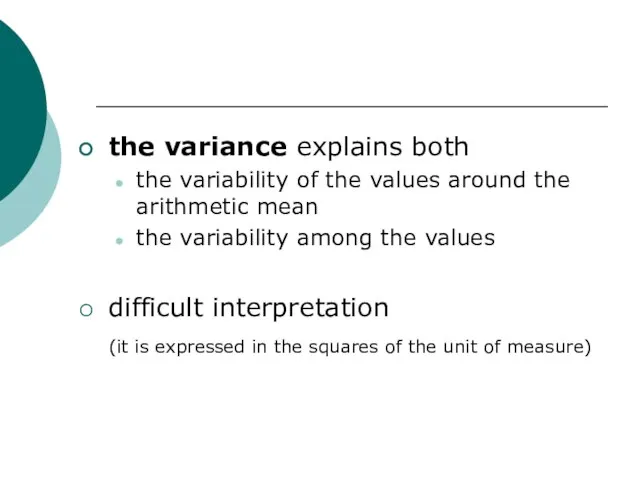
- 27. the variance explains both the variability of the values around the arithmetic mean the variability among
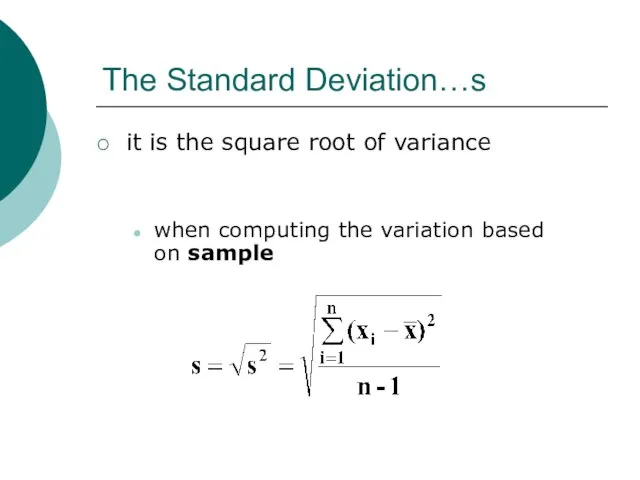
- 28. The Standard Deviation…s it is the square root of variance when computing the variation based on
- 29. it is expressed in the same unit of measure as the observed variable the size of
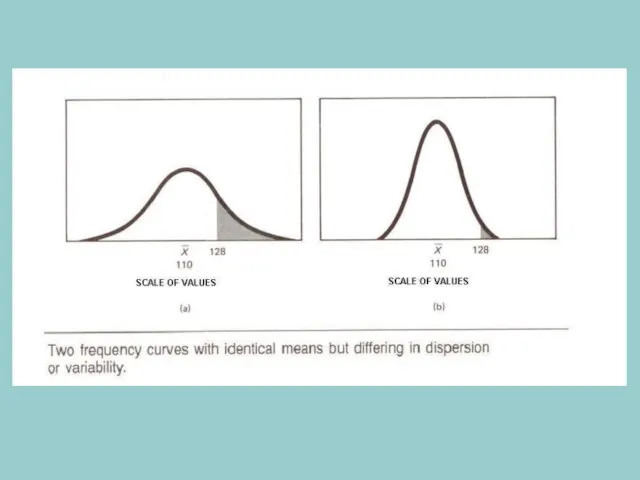
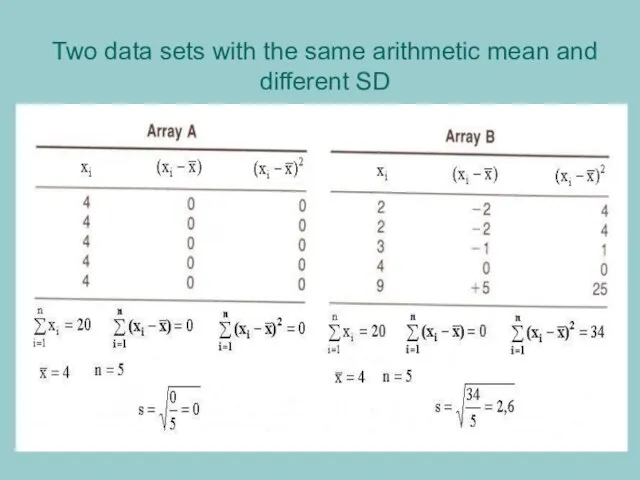
- 30. Two data sets with the same arithmetic mean and different SD
- 31. Example – Personal income (thousands CZK) thousands CZK
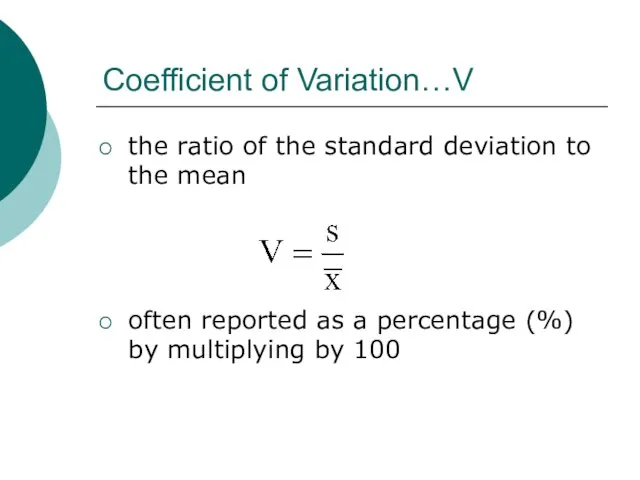
- 32. Coefficient of Variation…V the ratio of the standard deviation to the mean often reported as a
- 33. it is a relative measure of dispersion used when comparing two data sets with different units
- 34. Example – Personal income (thousands CZK)
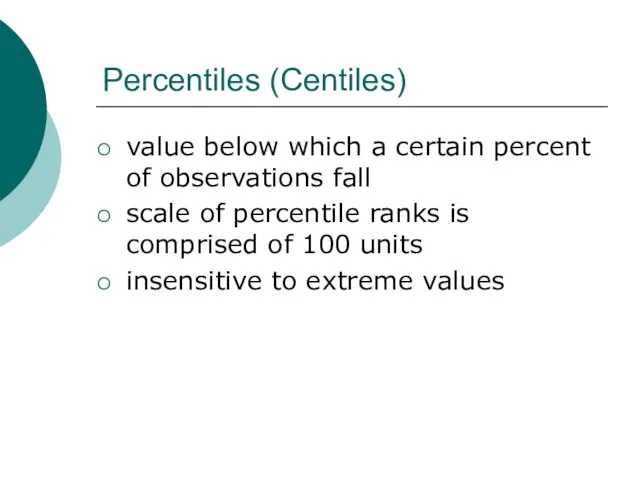
- 35. Percentiles (Centiles) value below which a certain percent of observations fall scale of percentile ranks is
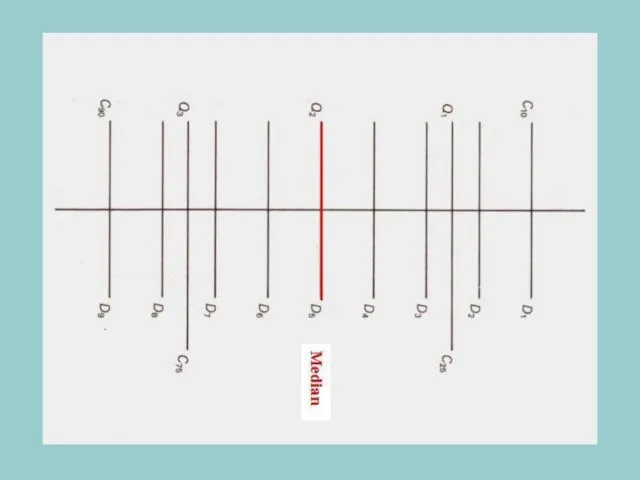
- 36. Deciles divides a distribution into 10 equal parts there are 9 deciles D1 – 1st decile
- 37. divides a distribution into 4 equal parts Q1 - 25 percent of values fall below it
- 39. Graphing Techniques
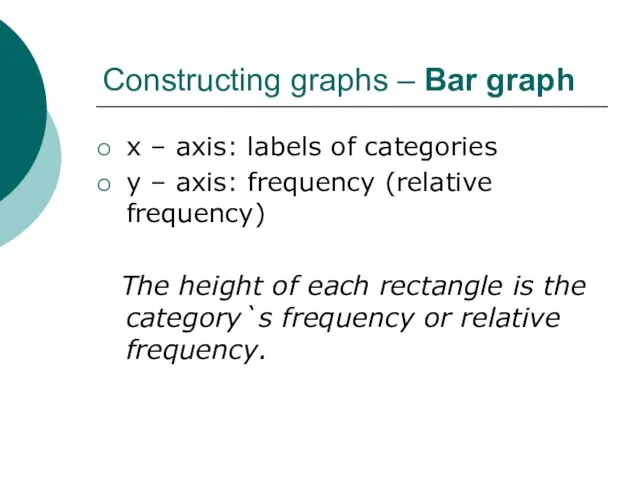
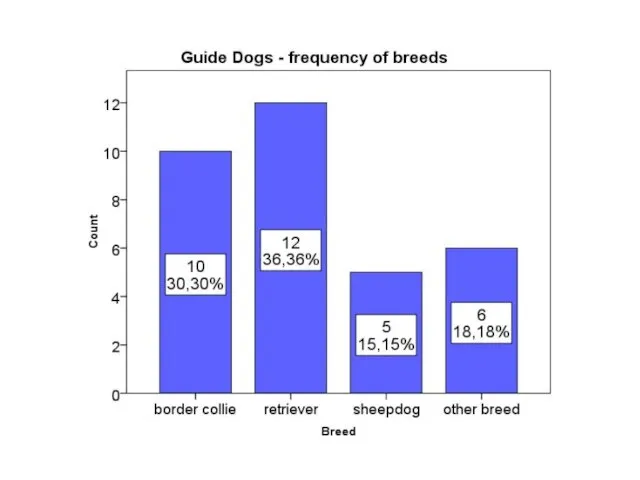
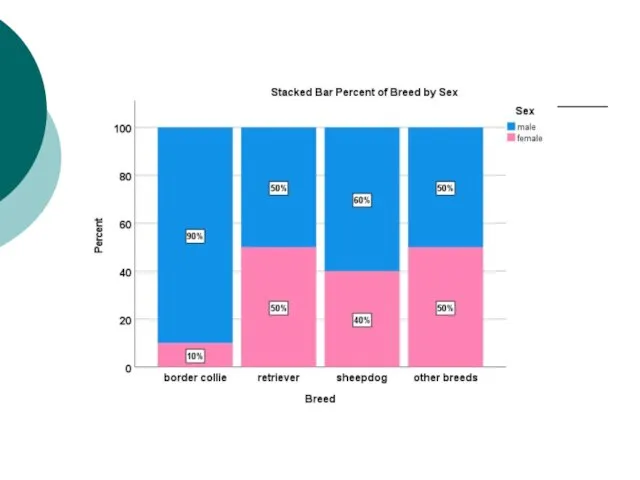
- 40. Constructing graphs – Bar graph x – axis: labels of categories y – axis: frequency (relative
- 41. Arranging the graph nominal variables – we can arrange the categories in any order:alphabetically, decreasing/increasing order
- 45. Constructing graphs – Pie graph Pie chart – a circle divided into sectors each sector represents
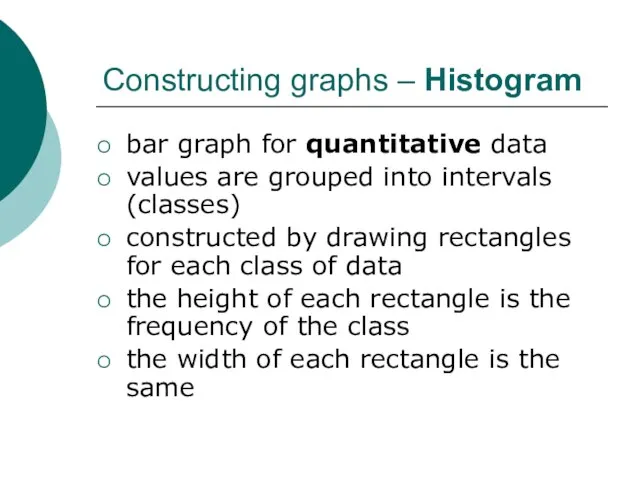
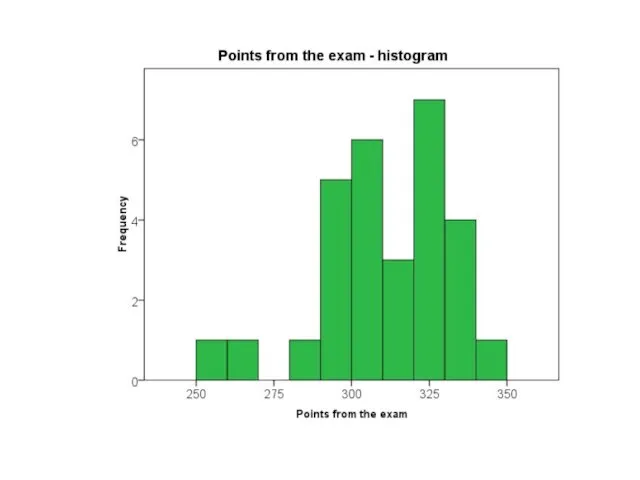
- 47. Constructing graphs – Histogram bar graph for quantitative data values are grouped into intervals (classes) constructed
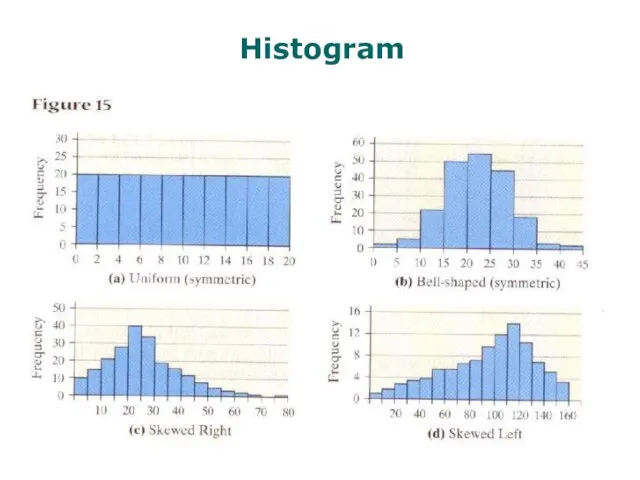
- 49. Histogram
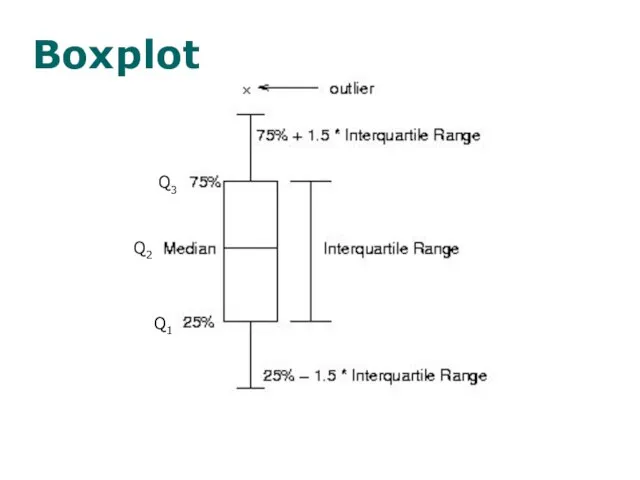
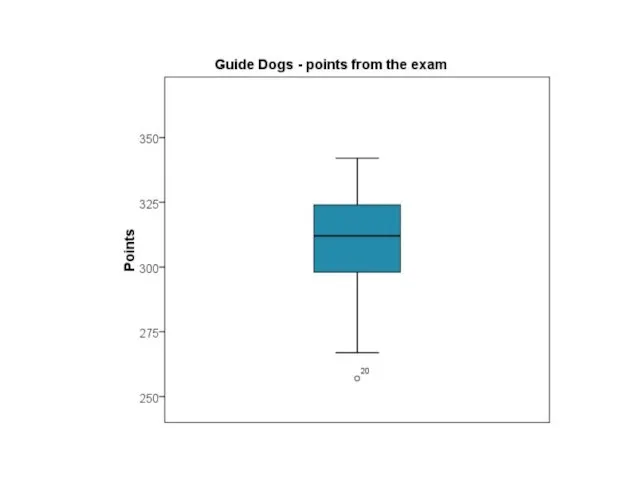
- 51. Constructing graphs – Boxplot box-and-whisker diagram five number summary
- 52. Boxplot Q3 Q1 Q2
- 55. Скачать презентацию















 Комплексные числа
Комплексные числа Обыкновенные дроби
Обыкновенные дроби Преобразование тригонометрических выражений. 10 класс. Часть 3
Преобразование тригонометрических выражений. 10 класс. Часть 3 Умножение числа 1 на однозначные числа
Умножение числа 1 на однозначные числа Определение арифметического корня п-ой степени
Определение арифметического корня п-ой степени Презентация на тему Уравнение прямой на плоскости (9 класс)
Презентация на тему Уравнение прямой на плоскости (9 класс)  Математические методы в психологии. Генеральная совокупность и выборка
Математические методы в психологии. Генеральная совокупность и выборка Сложение и вычитание с переходом через десяток в пределах 10. Интерактивная игра-тренажер по математике Смешарики
Сложение и вычитание с переходом через десяток в пределах 10. Интерактивная игра-тренажер по математике Смешарики Квадратный корень
Квадратный корень Заколдованные цифры
Заколдованные цифры Промежуточная мерка
Промежуточная мерка Решение задачи с использованием циклов
Решение задачи с использованием циклов Число и цифра 8
Число и цифра 8 Ликвидация пробелов в знаниях по теме Соотношения между сторонами и углами треугольника
Ликвидация пробелов в знаниях по теме Соотношения между сторонами и углами треугольника Вычитание векторов
Вычитание векторов вычитание векторов 13.10
вычитание векторов 13.10 Таблица умножения на 2
Таблица умножения на 2 Презентация на тему Модуль числа (6 класс)
Презентация на тему Модуль числа (6 класс)  Галерея великих имён
Галерея великих имён Решение иррациональных уравнений
Решение иррациональных уравнений Сложение с переходом через десяток
Сложение с переходом через десяток Тригонометрические уравнения сtgх=а. 10 класс
Тригонометрические уравнения сtgх=а. 10 класс Сайы. Умумий малюмат
Сайы. Умумий малюмат Треугольник. Первый признак равенства треугольников
Треугольник. Первый признак равенства треугольников Презентация на тему Математические диктанты 3 класс
Презентация на тему Математические диктанты 3 класс  Презентация на тему Круговые диаграммы
Презентация на тему Круговые диаграммы  Параллельность прямых в пространстве
Параллельность прямых в пространстве